UNIT 2
Projections Of Planes
Projection of planes when it is parallel to one & perpendicular to other reference plane
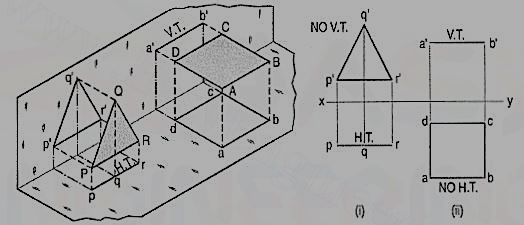
a) Plane, perpendicular to the H.P. And parallel to the V.P. Figure. A triangle PQR is perpendicular to the H.P. And is parallel to the V.P. Its H.T. Is parallel to xy. It has no V.T.
The front view p'q'r' shows the exact shape and size of the triangle. The top view pqr is a line parallel to xy. It coincides with the H.T.
(b) Plane, perpendicular to the V.P. And parallel to the H.P figure. A square ABCD is perpendicular to the V.P. And parallel to the H.P. Its V.T. Is parallel to xy. It has no H.T.
The top view abed shows the true shape and true size of the square. The front view a'b' is a line, parallel to xy. It coincides with the V.T.
Lying in reference plane
Inclined to one & perpendicular to other reference plane
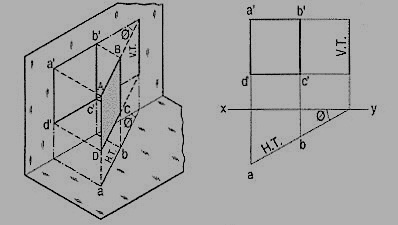
A square ABCD is perpendicular to the H.P. And inclined at an angle φ to the V.P. Its V.T. Is perpendicular to xy. Its H.T. Is inclined at φ to xy.
Its top view ab is a line inclined at φ to xy. The front view a'b'c'd' is smaller than ABCD.
(b) Plane, perpendicular to the V.P. And inclined to the H.P. Figure.
A square ABCD is perpendicular to the V.P. And inclined at an angle θ to the H.P. Its H.T. Is perpendicular to xy. Its V.T. Makes the angle e with xy. Its front view a'b' is a line inclined at θ to xy. The top view abed is a rectangle which is smaller than the square ABCD.
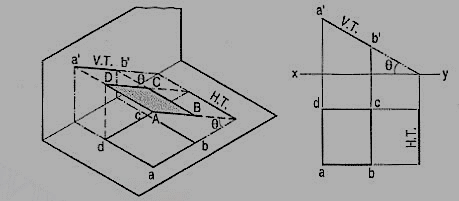
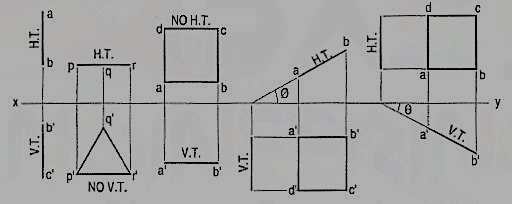
Figure shows the projections and the traces of all these perpendicular planes by third-angle projection method.
A plane is a two dimensional object having length and breadth only. Its thickness is always neglected. Various shapes of plane figures are considered such as square, rectangle, circle, pentagon, hexagon, etc.
PROJECTIONS OF PLANES
- To draw their projections means F.V, T.V. & S.V.
What will be given in the problem?
- Description of the plane figure.
- It’s position with HP and VP.
In which manner it’s position with HP & VP will be described?
- Inclination of its SURFACE with one of the reference planes will be given
- Inclination of one of its EDGES with other reference plane will be given (Hence this will be a case of an object inclined to both reference Planes.)

Solids: cube, tetrahedron, prism, pyramid, cylinder and cone
Solids may be divided into two main groups:
(1) Polyhedra
(2) Solids of revolution.
Polyhedra: A polyhedron is defined as a solid bounded by planes called faces. When all faces are equal and regular, the polyhedron is said to be regular. There are seven regular polyhedra which may be defined as stated below:
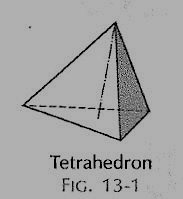
(i) Tetrahedron figure . It has four equal faces, each an equilateral triangle.
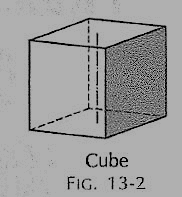
(ii) Cube or hexahedron figure . It has six faces, all equal squares.
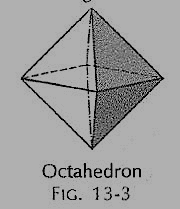
(iii) Octahedron figure . It has eight equal equilateral triangles as faces.
(iv) Dodecahedron figure: It has twelve equal and regular pentagons as faces.
(v) Icosahedron figure: It has twenty faces, all equal equilateral triangles.
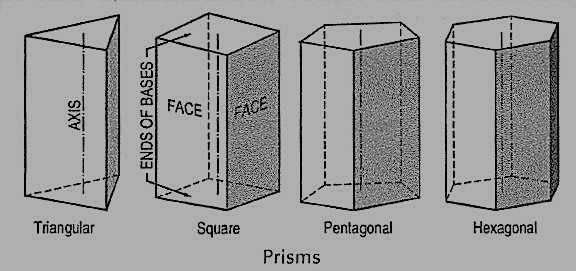
(vi) Prism: This is a polyhedron having two equal and similar faces called its ends or bases, parallel to each other and joined by other faces which are parallelograms. The imaginary line joining the centres of the bases is called the axis. A right and regular prism figure has its axis perpendicular to the bases. All its faces are equal rectangles.
(vii) Pyramid: This is a polyhedron having a plane figure as a base and many triangular faces meeting at a point called the vertex or apex. The imaginary line joining the apex with the centre of the base is its axis.
A right and regular pyramid figure has its axis perpendicular to the base which is a regular plane figure. Its faces are all equal isosceles triangles.

Oblique prisms and pyramids have their axes inclined to their bases. Prisms and pyramids are named according to the shape of their bases, as triangular, square, pentagonal, hexagonal etc.
(viii) Cylinder figure: A right circular cylinder is a solid generated by the revolution of a rectangle about one of its sides which remains fixed. It has two equal circular bases. The line joining the centres of the bases is the axis. It is perpendicular to the bases.
(ix) Cone figure: A right circular cone is a solid generated by the revolution of a right-angled triangle about one of its perpendicular sides which is fixed.
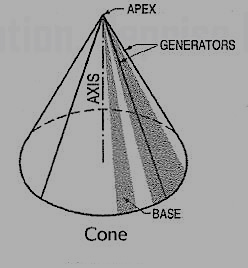
It has one circular base. Its axis joins the apex with the centre of the base to which it is perpendicular. Straight lines drawn from the apex to the circumference of the base-circle are all equal and are called generators of the cone. The length of the generator is the slant height of the cone.
(x) Sphere figure: A sphere is a solid generated by the revolution of a semi-circle about its diameter as the axis. The mid-point of the diameter is the centre of the sphere. All points on the surface of the sphere are equidistant from its centre.
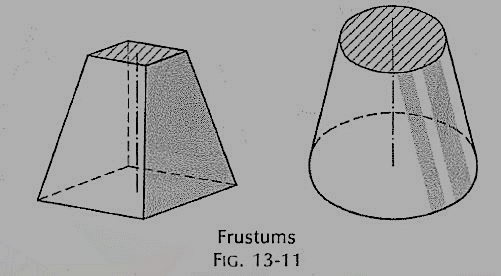
(xi) Frustum: When a pyramid or a cone is cut by a plane parallel to its base, thus removing the top portion, the remaining portion is called its frustum figure.
(xii) Truncated: When a solid is cut by a plane inclined to the base it is said to be truncated.
Projections of above solids when axis perpendicular to one of the reference planes
Axis perpendicular to the H.P.:
Problems:
1. Draw the projections of a triangular prism, base 40 mm side and axis 50 mm long, resting on one of its bases on the H.P. With a vertical face perpendicular to the V.P.
(i) As the axis is perpendicular to the ground i.e. the H.P. Begin with the top view. It will be an equilateral triangle of sides 40 mm long, with one of its sides perpendicular to xy. Name the corners as shown, thus completing the top view. The corners d, e and fare hidden and coincide with the top corners a, b and c respectively
(ii) Project the front view, which will be a rectangle. Name the corners. The line b'e' coincides with a'd'.
2. Draw the projections of a pentagonal pyramid, base 30 mm edge and axis 50 mm long, having its base on the H.P. And an edge of the base parallel to the V.P. Also draw its side view.
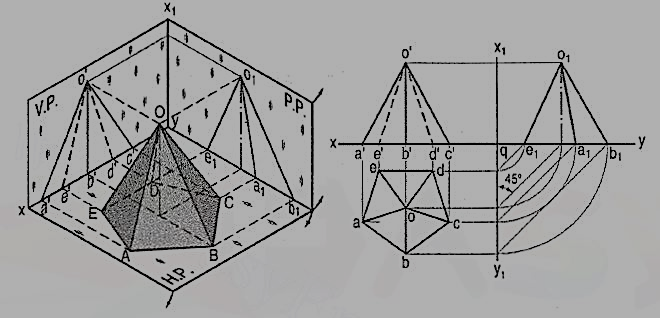
(i) Assume the side DE which is nearer the V.P., to be parallel to the V.P. As shown in the pictorial view.
(ii) In the top view, draw a regular pentagon abcde with ed parallel to and nearer xy. Locate its centre o and join it with the corners to indicate the slant edges.
(iii) Through o, project the axis in the front view and mark the apex o', 50 mm above xy. Project all the corners of the base on xy. Draw lines o'a', o'b' and o'c' to show the visible edges. Show o'd' and o'e' for the hidden edges as dashed lines.
(iv) For the side view looking from the left, draw a new reference line x1y1 perpendicular to xy and to the right of the front view. Project the side view on it, horizontally from the front view as shown. The respective distances of all the points in the side view from x1y1, should be equal to their distances in the top view from xy. This is done systematically as explained below:
(v) From each point in the top view, draw horizontal lines up to x1y1. Then draw lines inclined at 45° to x1y1 (or xy) as shown. Or, with q, the point of intersection between xy and x1y1 as centre, draw quarter circles. Project up all the points to intersect the corresponding horizontal lines from the front view and complete the side view as shown in the figure. Lines o1d1 and o1c1 coincide with o1e1 and o1a1 respectively.
Axis perpendicular to the V.P.:
A hexagonal prism figure has one of its rectangular faces parallel to the H.P. Its axis is perpendicular to the V.P. And 3.5 cm above the ground. Draw its projections when the nearer end is 2 cm in front of the V.P. Side of base 2.5 cm long; axis 5 cm long.
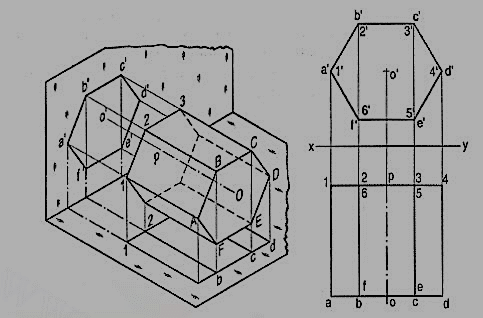
(i) Begin with the front view. Construct a regular hexagon of 2.5 cm long sides with its centre 3.5 cm above xy and one side parallel to it.
(ii) Project down the top view, keeping the line for nearer end, viz. 1-4, 2 cm below xy.
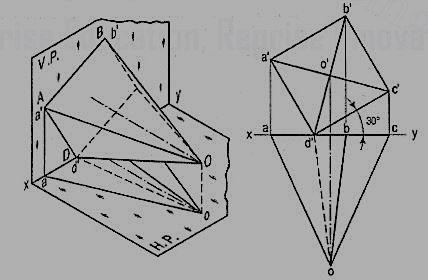
2. A square pyramid figure , base 40 mm side and axis 65 mm long, has its base in the V.P. One edge of the base is inclined at 30° to the H.P. And a corner contained by that edge is on the H.P. Draw its projections.
(i) Draw a square in the front view with the corner d' in xy and the side d'c' inclined at 30° to it. Locate the centre o' and join it with the corners of the square.
(ii) Project down all the corners in xy (because the base is in the V.P.). Mark the apex o on a projector through o'. Draw lines for the slant edges and complete the top view.
Axis inclined to one & parallel to other reference plane
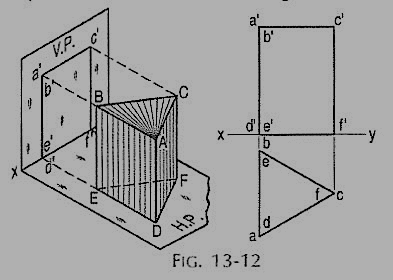
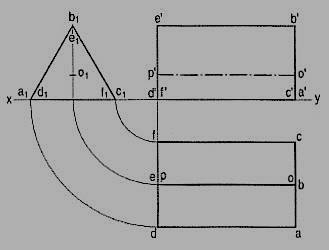
A triangular prism1 base 40 mm side and height 65 mm is resting on the H.P. On one of its rectangular faces with the axis parallel to the V.P. Draw its projections.
As the axis is parallel to both the planes, begin with the side view.
(i) Draw an equilateral triangle representing the side view, with one side in xy.
(ii) Project the front view horizontally from this triangle.
(iii) Project down the top view from the front view and the side view, as shown.
Problems:
Draw the projections of the following solids, situated in their respective positions, taking a side of the base 40 mm long or the diameter of the base 50 mm long and the axis 65 mm long.
1. A hexagonal pyramid, base on the H.P. And a side of the base parallel to and 25 mm in front of the V.P.
2. A square prism, base on the H.P., a side of the base inclined at 30° to the V.P. And the axis 50 mm in front of the V.P.
3. A triangular pyramid, base on the H.P. And an edge of the base inclined at 45° to the V.P.; the apex 40 mm in front of the V.P.
Axis inclined to both the reference planes
When a solid has its axis inclined to one plane and parallel to the other, its projections are drawn in two stages.
(1) In the initial stage, the solid is assumed to be in simple position, i.e. its axis perpendicular to one of the planes.
If the axis is to be inclined to the ground, i.e. the H.P., it is assumed to be perpendicular to the H.P. In the initial stage. Similarly, if the axis is to be inclined to the V.P., it is kept perpendicular to the V.P. In the initial stage.
Moreover
(i) if the solid has an edge of its base parallel to the H.P. Or in the H.P. Or on the ground, that edge should be kept perpendicular to the V.P.; if the edge of the base is parallel to the V.P. Or in the V.P., it should be kept perpendicular to the H.P.
(ii) If the solid has a corner of its base in the H.P. Or on the ground, the sides of the base containing that corner should be kept equally inclined to the V.P.; if the corner is in the V.P., they should be kept equally inclined to the H.P.