UNIT 3
Sections Of Solids
Section planes
Section planes are generally perpendicular planes. They may be perpendicular to one of the reference planes and either perpendicular, parallel or inclined to the other plane. They are usually described by their traces. It is important to remember that the projection of a section plane, on the plane to which it is perpendicular, is a straight line. This line will be parallel, perpendicular or inclined to xy, depending upon the section plane being parallel, perpendicular or inclined respectively to the other reference plane.
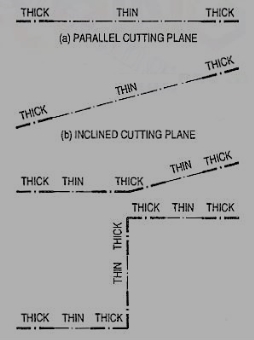
Figure 1
As per latest 8.1.S. Convention (SP: 46-2003), the cutting-plane line should be drawn as shown in fig. 1.
Sectional views
The projection of the section on the reference plane to which the section plane is perpendicular, will be a straight-line coinciding with the trace of the section plane on it. Its projection on the other plane to which it is inclined is called apparent section. This is obtained by
(i) projecting on the other plane, the points at which the trace of the section plane intersects the edges of the solid and
(ii) drawing lines joining these points in proper sequence.
To project sectional views of above solids cut by different section planes (when solid is in simple position and when axis is parallel to one & inclined to other reference plane)
Sections of Prisms:
These are illustrated according to the position of the section plane with reference
To the principal planes as follows:
(1) Section plane parallel to the V.P.
(2) Section plane parallel to the H.P.
(3) Section plane perpendicular to the H.P. And inclined to the V.P.
(4) Section plane perpendicular to the V.P. And inclined to the H.P.
Section parallel to the VP
Problem 1:
A cube of 35 mm long edges is resting on the H.P. On one of its faces with a vertical face inclined at 30° to the V.P. It is cut by a section plane parallel to the V.P. And 9 mm away from the axis and further away from the V.P. Draw its sectional front view and the top view.
In fig.2 (i), the section plane is assumed to be transparent and the cube is shown with the cut-portion removed. Four edges of the cube are cut and hence, the section is a figure having four sides.
Draw the projections of the whole cube in the required position [fig.2(ii)].As the section plane is parallel to the V.P., it is perpendicular to the H.P.; hence, the section will be a line in the top view coinciding with the H.T. Of the section plane.
(i) Draw a line H.T. In the top view (to represent the section plane) parallel to xy and 9 mm from o.
(ii) Name the points at which the edges are cut, viz. Ab at 1, be at 2, gf at 3 and fe at 4.
(iii) Project these points on the corresponding edges in the front view and join them in proper order.
As the section plane is parallel to the V.P., figure 1' 2' 3' 4' in the front view, shows the true shape of the section.
Show the views by dark but thin lines, leaving the lines for the cut-portion fainter.
(iv) Draw section lines in the rectangle for the section.
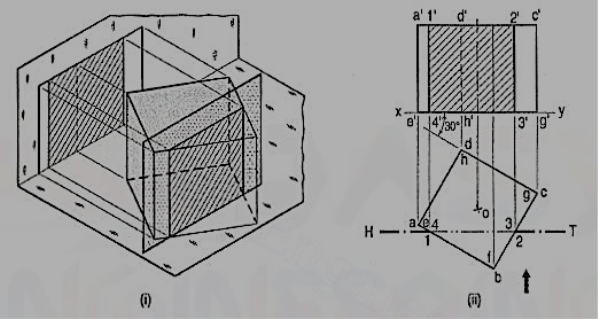
Figure 2
(2) Section plane parallel to the H.P.
Problem 2 (fig. 3): A triangular prism, base 30 mm side and axis 50 mm long, is lying on the H.P. On one of its rectangular faces with its axis inclined at 30° to the V.P. It is cut by a horizontal section plane, at 12 mm above the ground. Draw its front view and sectional top view.
As the section plane is horizontal, i.e. parallel to the H.P., it is perpendicular to the V.P. Hence, the section will be a line in the front view, coinciding with the V. T. Of the section plane.
(i) Therefore, draw a line V.T. In the front view to represent the section plane, parallel to xy and 12 mm above it.
(ii) Name in correct sequence, points at which the edges are cut viz. a'b' at 1 ', a'c' at 2', d'f' at 3' and d'e' at 4'.
(iii) Project these points on the corresponding lines in the top view and complete the sectional top view by joining them in proper order.
As the section plane is parallel to the H.P., the figure 1 2 3 4 (in the top view) is the true shape of the section.
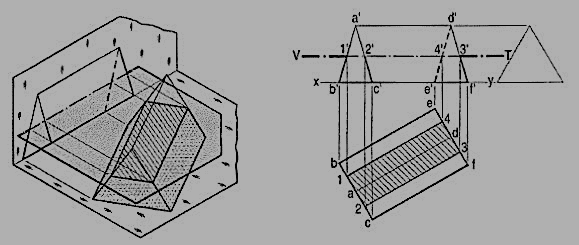
Figure 3
(3) Section plane perpendicular to the H.P. And inclined to the V.P.
Problem 3. (fig. 4): A cube in the same position as in problem 1, is cut by a section plane, inclined at 60° to the V.P. And perpendicular to the H.P., so that the face which makes 60° angle with the V.P. Is cut in two equal halves. Draw the sectional front view, top view and true shape of the section.
The section will be a line in the top view coinciding with the H.T. Of the section plane.
(i) Draw the projections of the cube. Draw a line H.T. In the top view inclined at 60° to xy and cutting the line ad (or be) at its mid-point.
(ii) Name the corners at which the four edges are cut and project them in the front view. As the section plane is inclined to the V.P., the front view of the section viz. 1' 2' 3' 4' does not reveal its true shape. Only the vertical lines show true lengths, while the true lengths of the horizontal lines are seen in the top view. The true shape of the section will be seen when it is projected on an auxiliary vertical plane, parallel to the section plane.
(iii) Therefore, draw a new reference line x1y1 parallel to the H.T. And project the section on it. The distances of the points from x1y1 should be taken equal to their corresponding distances from xy in the front view. Thus 4" and 3" will be on x1y1. 1" 4" and 2" 3" will be equal to 1' 4' and 2' 3' respectively. Complete the rectangle 1" 2" 3"4" which is the true shape of the section and draw section lines in it.
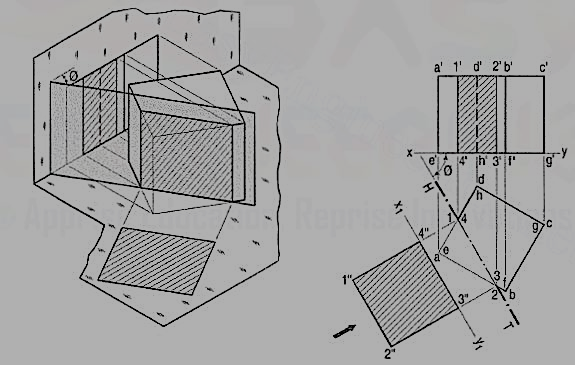
Figure 4
(4) Section plane perpendicular to the V.P. And inclined to the H.P.
Problem 4. (fig. 5): A cube in the same position as in problem 14-1 is cut by a section plane, perpendicular to the V.P., inclined at 45° to the H.P. And passing through the top end of the axis. (i) Draw its front view, sectional top view and true shape of the section. (ii) Project another top view on an auxiliary plane, parallel to the section plane.
The section will be a line in the front view.
(i) Draw a line V.T. In the front view, inclined at 45° to xy and passing through the top end of the axis. It cuts four edges, viz. a' e' at 1 ', a' b' at 2', c'd' at 3' and d'h' at 4'.
(ii) Project the top view of the section, viz. The figure 1 2 3 4. It does not show the true shape of the section, as the section plane is inclined to the H.P. To determine the true shape, an auxiliary top view of the section should be projected on an A.I.P. Parallel to the section plane.
(iii) Assuming the new reference line for the A.I.P. To coincide with the V.T., project the true shape of the section as shown by quadrilateral 11 21 31 41. The distances of all the points from the V.T. Should be taken equal to their corresponding distances from xy in the top view, e.g. 111' = e'1, 414' = h'4 etc.
(iv) To project an auxiliary sectional top view of the cube, draw a new reference line x1 y1, parallel to the V.T. The whole cube may first be projected and the points for the section may then be projected on the corresponding lines for the edges. Join these points in correct sequence and obtain the required top view.
(v) Draw section lines in the cut-surface, in the views where it is seen. Keep the lines for the removed edges thin and fainter.
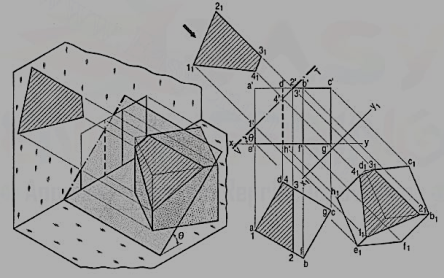
Figure 5
Sections of Pyramids:
The following cases are discussed in details.
(1) Section plane parallel to the base of the pyramid.
(2) Section plane parallel to the V.P.
(3) Section plane perpendicular to the V.P. And inclined to the H.P.
(4) Section plane perpendicular to the H.P. And inclined to the V.P.
(1) Section plane parallel to the base of the pyramid.
Problem 5 (fig. 6): A pentagonal pyramid, base 30 mm side and axis 65 mm long has its base horizontal and an edge of the base parallel to the V.P. A horizontal section plane cuts it at 25 mm above the base. Draw it’s from view and sectional top view.
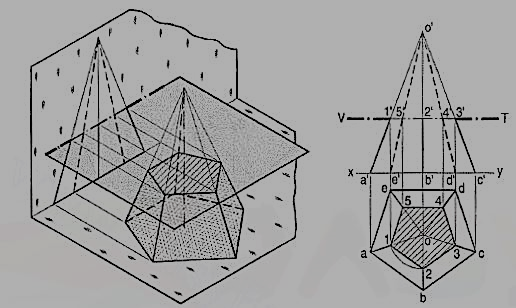
Figure 6
(i) Draw the projections of the pyramid in the required position and show a line V.T. For the section plane, parallel to and 25 mm above the base. All the five slant edges are cut.
(ii) Project the points at which they are cut, on the corresponding edges in the top view. The point 2' cannot be projected directly as the line ob is perpendicular to xy. But it is quite evident from the projections of other points that the lines of the section in the top view, viz. 3-4, 4-5 and 5-1 are parallel to the edges of the base in their respective faces and that the points 1, 3, 4 and 5 are equidistant from o.
(iii) Hence, line 1-2 also will be parallel to ab and o2 will be equal to o1, o3 etc. Therefore, with o as centre and radius o1, draw an arc cutting ob at a point 2 which will be the projection of 2'. Complete the sectional top view in which the true shape of the section, viz. The pentagon 1, 2, 3, 4 and 5 is also seen.
(iv) Hence, when a pyramid is cut by a plane parallel to its base, the true shape of the section will be a figure, like the base; the sides of the section will be parallel to the edges of the base in the respective faces and the corners of the section will be equidistant from the axis.
(2) Section plane parallel to the V.P.
Problem 6 (fig. 7): A triangular pyramid, having base 40 mm side and axis 50 mm long, is lying on the H.P. On one of its faces, with the axis parallel to the V.P. A section plane, parallel to the V.P. Cuts the pyramid at 6 mm from the axis. Draw its sectional front view and the top view.
(i) Draw the projections of the pyramid in the required position and show a line H.T. (for the cutting plane) in the top view parallel to xy and 6 mm from the axis.
(ii) Project points 1, 2 and 3 (at which the edges are cut) on corresponding edges in the front view and join them. Figure 1' 2' 3' shows the true shape of the section.
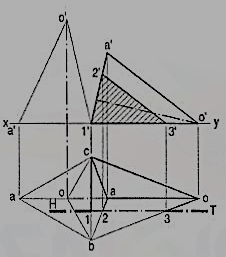
Figure 7
(3) Section plane perpendicular to the V.P. And inclined to the H.P.
Problem 7 (fig. 8): A square pyramid, base 40 mm side and axis 65 mm long, has its base on the H.P. And all the edges of the base equally inclined to the V.P. It is cut by a section plane, perpendicular to the V.P., inclined at 45° to the H.P. And bisecting the axis. Draw its sectional top view, sectional side view and true shape of the section.
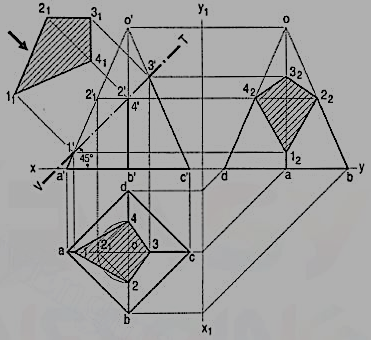
Figure 8
(i) Draw the projections of the pyramid in the required position. The section plane will be seen as a line in the front view. Hence, draw a line V.T. Through the mid-point of the axis and inclined at 45° to xy. Name in correct sequence the points at which the four edges are cut and project them in the top view. Here also, points 2' and 4' cannot be projected directly.
Hence, assume a horizontal section through 2' and draw a line parallel to the base, cutting o' a' at 2'1. Project 2'1 to 21 on oa in the top view. From 21 draw a line parallel to ab and cutting ob at a point 2. Or, with o as centre and radius o 21, draw an arc cutting ob at 2 and ob at 4. Complete the section 1 2 3 4 by joining the points and draw section lines in it.
(ii) Assuming the V.T. To be the new reference line, draw the true shape of the section. Project the side view from the two views. The removed portion of the pyramid may be shown by thin and faint lines.
(4) Section plane perpendicular to the H.P. And inclined to the V.P.
Problem 8 (fig. 9) A pentagonal pyramid has its base on the H.P. And the edge of the base nearer the V.P., parallel to it. A vertical section plane, inclined at 45° to o the V.P., cuts the pyramid at 6 mm from the axis. Draw the top view, sectional front view and the auxiliary front view on an A. V.P. Parallel to the section plane. Base of the pyramid 30 mm side; axis 50 mm long.
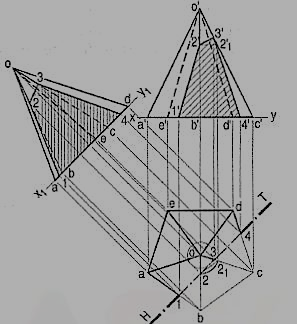
Figure 9
The section plane will be a line in the top view. It is to be at 6 mm from the axis.
i) Draw a circle with o as center and radius equal to 6mm.
Ii) Draw a line H.T. Tangent to this circle and inclined at 45° to xy. It can be drawn in four different positions, of which any one may be selected.
Iii) Project points 1, 2 etc. from the top view to the corresponding edges in the front view. Here again, point 2 cannot be projected directly. With centre o and radius o2 draw an arc cutting any one of the slant edges, say oc at 21. Project 21 to 2'1 on o'c'.
(iv) Through 2'1, draw a line parallel to the base, cutting o' b' at 2'. Then 2' is the required point. Complete the view. It will show the apparent section.
(v) Draw a reference line x1y1 parallel to the H.T. And project an auxiliary sectional front view which will show the true shape of the section also.
Sections of Cylinders:
It consists of following three cases:
(1) Section plane parallel to the base
(2) Section plane parallel to the axis
(1) Section plane parallel to the base:
When a cylinder is cut by a section plane parallel to the base, the true shape of the section is a circle of the same diameter.
(2) Section plane parallel to the axis:
When a cylinder is cut by a section plane parallel to the axis, the true shape of the section is a rectangle, the sides of which are respectively equal to the length of the axis and the length of the section plane within the cylinder (fig. 10). When the section plane contains the axis, the rectangle will be of the maximum size.
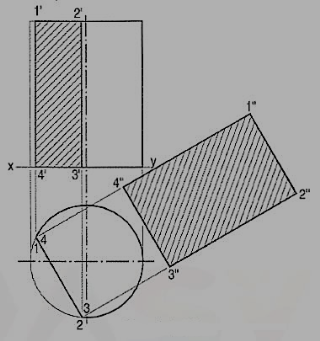
Figure 10
Problem 9 (fig. 11): A cylinder of 40 mm diameter, 60 mm height and havingits axis vertical, is cut by a section plane, perpendicular to the V.P., inclined at 45° tothe H.P. And intersecting the axis32 mm above the base. Drawits front view, sectional top view,sectional side view and true shape of the section.
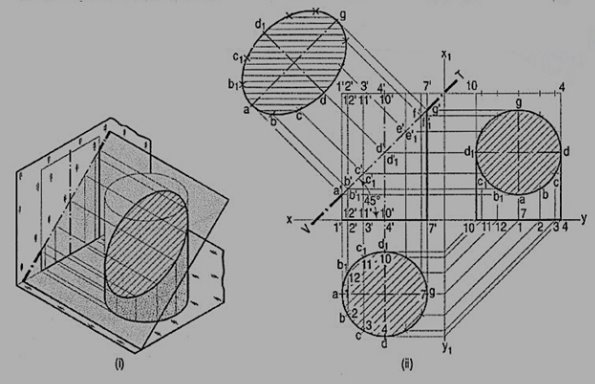
Figure 11
As the cylinder has no edges, many lines representing the generators may be assumed on its curved surface by dividing the base-circle into, say 12 equal parts.
(i) Name the points at which these lines are cut by the V.T. In the top view, these points lie on the circle and hence, the same circle is the top view of the section. The width of the section at any point, say c', will be equal to the length of the chord cc1 in the top view.
(ii) The true shape of the section may be drawn around the center line ag drawn parallel to V.T. As shown. It is an ellipse the major axis of which is equal to the length of the section plane viz. a'g', and the minor axis equal to the diameter of the cylinder viz. Dd1.
(iii) Project the sectional side view as shown. The section will be a circle because the section plane makes 45° angle with xy.
Sections of Cones:
(1) Section plane parallel to the base of the cone.
(2) Section plane passing through the apex of the cone.
(3) Section plane inclined to the base of the cone at an angle smaller than the angle of inclination of the generators with the base.
(4) Section plane parallel to a generator of the cone.
(5) Section plane inclined to the base of the cone at an angle greater than the angle of inclination of the generators with the base.
(1) Section plane parallel to the base of cone:
The cone resting on the H.P. On its base [fig. 12(i)] is cut by a section plane parallel to the base. The true shape of the section is shown by the circle in the top view, whose diameter is equal to the length of the section viz. a'a'. The width of the section at any point, say b', is equal to the length of the chord bb1.
Problem:
Fig. 12 (ii) To locate the position in the top view of any given point p' in the front view of the above cone.
Method I:
(i) Through p', draw a line r'r' parallel to the base.
(ii) With o as center and diameter equal to r'r', draw a circle in the top view.
(iii) Project p' to points p and p1 on this circle. p is the top view of p'. p1 is the top view of another point p'1 on the back side of the cone and coinciding with p'. The chord pp1 shows the width of the horizontal section of the cone at the point p'. This method may be called the circle method.
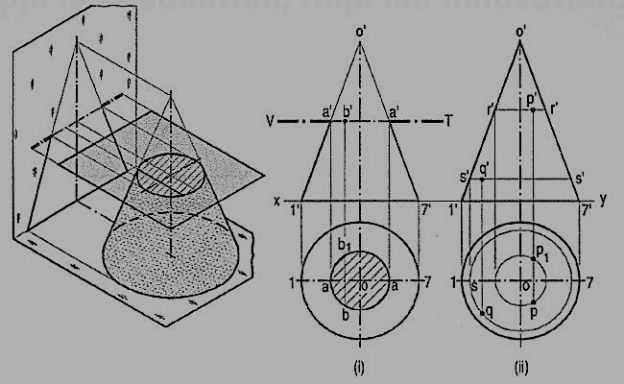
Figure 12
Method II:
When the position of a point in the top view say q is given, its front view q' can be determined by reversing the above process.
(i) With centre o and radius oq, draw a circle cutting the horizontal centre line at s.
(ii) Through s, draw a projector cutting the slant side o'1' at s'.
(iii) Draw the line s's' parallel to the base, intersecting a projector through q at the required point q'.
(2) Section plane passing through the apex of the cone:
Problem
[fig. 13 (i)]: A cone, diameter of base 50 mm and axis 50 mm long is resting on its base on the H.P. It is cut by a section plane perpendicular to the V.P., inclined at 75° to the H.P. And passing through the apex. Draw its front view, sectional top view and true shape of the section.
Draw the projections of the cone and on it, show the line V. T. For the section plane.
Mark many points a', b' etc. on the V.T. And project them to points a, b etc. in the top view by the circle method. It will be found that these points lie on a straight line through o.
Thus, od is the top view of the line or generator o'd' and triangle odd1 is the top view of the section. The width of the section at any point b' on the section is the line bb1, obtained by projecting b' on this triangle. This method is called the generator method.
Project the true shape of the section. It is an isosceles triangle, the base of which is equal to the length of the chord on the base-circle and the altitude is equal to the length of the section plane within the cone.
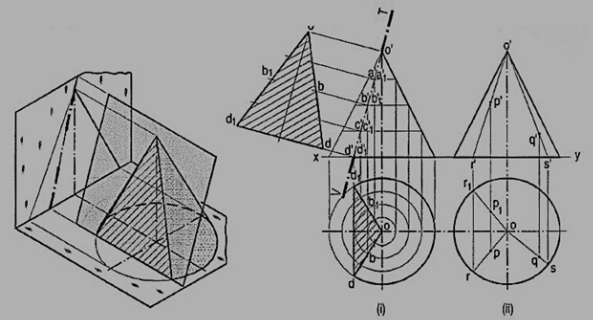
Figure 13
(3) Section plane inclined to the base of the cone at an angle smaller than the angle of inclination of the generators with the base:
Problem 14-24. A cone, base 75 mm diameter and axis 80 mm long is resting on its base on the H.P. It is cut by a section plane perpendicular to the V.P., inclined at 45° to the H.P. And cutting the axis at a point 35 mm from the apex. Draw its front view, sectional top view, sectional side view and true shape of the section.
Draw a line V.T. In the required position in the front view of the cone.
(i) Generator method [fig. 14- and fig. 15]:
(a) Divide the base-circle into many equal parts, say 12. Draw lines (i.e. generators) joining these points with o. Project these points on the line representing the base in the front view.
(b) Draw lines o' 2', o' 3' etc. cutting the line for the section at points b', c' etc. Project these points on the corresponding lines in the top view. For example, point b' on o' 2', also represents point b' 1 on o'-12' which coincides with o'-12'. Therefore, project b' to b on o 2 and to b1 on o'-12'. b and b1 are the points on the section (in the top view).
(c) Similarly, obtain other points. Point d' cannot be projected directly. Hence, the same method as in case of pyramids should be employed to determine the positions d and d1, as shown. In addition to these, two more points for the maximum width of the section at its centre should also be obtained. Mark m', the mid-point of the section and obtain the points m and m1. Draw a smooth curve through these points.
(d) The true shape of the section may be obtained on the V.T. As a new reference line or symmetrically around the centre line ag, drawn parallel to the V.T. As shown. It is an ellipse whose major axis is equal to the length of the section and minor axis equal to the width of the section at its centre.
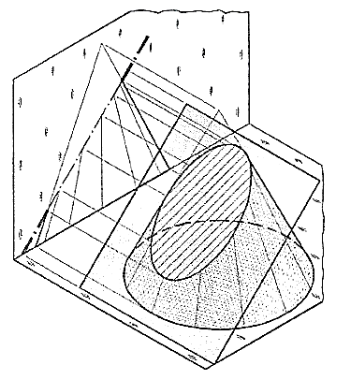
Figure 14
Draw the sectional side view by projecting the points on corresponding generators, as shown.
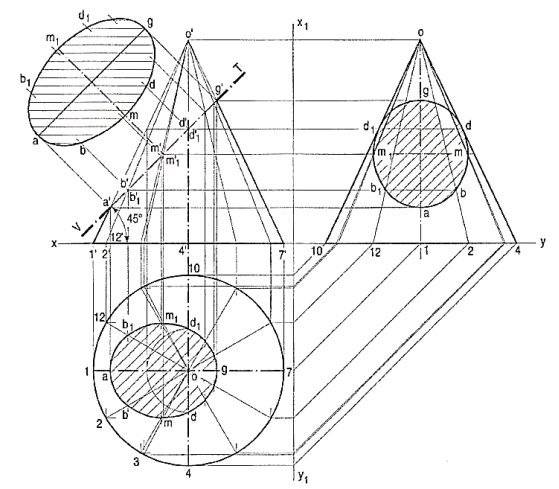
Figure 15
(ii) Circle method (fig. 16):
(a) Divide the line of section into many equal parts. Determine the width of section at, and the position of each division-point in the top view by the circle method. For example, through c', draw a line c"c" parallel to the base.
(b) With o as centre and radius equal to half of c"c", draw an arc. Project c' to c and c1 on this arc. Then c and c1 are the required points. The straight line joining c and c1 will be the width of the section at c'.
(c) Similarly, obtain all other points and draw a smooth curve through them. This curve will show the apparent section. The maximum width of the section will be at the mid-point e'. It is shown in the top view by the length of the chord joining e and e1.
(d) Draw a reference line x1 y1 parallel to the V.T. And project the true shape of the section. In the figure, the auxiliary sectional top view of the truncated cone is shown. It shows the true shape of the section.
The sectional side view may be obtained by projecting all the division-points horizontally and then marking the width of the section at each point, symmetrically around the axis of the cone.

Figure 16
Section plane parallel to a generator of the cone:
Problem (fig. 17): The cone in same position as in fig. 12, is cut bya section plane perpendicular to the V.P. And parallel to and 12 mm away from one ofits end generators. Draw its front view, sectional top view and true shape of the section.
(i) Draw a line V.T. (for the section plane) parallel to and 12 mm away from the generator o' 1 '.
(ii) Draw the twelve generators in the top view and project them to the front view. All the generators except o' 1 ', o' 2' and o'-12' are cut by the section plane. Project the points at which they are cut, to the corresponding generators in the top view. The width of the section at the point where the base is cut will be the chord aa1. Draw a curve through a ...f... a1. The figure enclosed between aa1 and the curve is the apparent section.
(iii) Obtain the true shape of the section as explained in the previous problem.
It will be a parabola.

Figure 17
Section plane inclined to the base of the cone at an angle greater than the angle of inclination of the generators with the base:
Problem
Fig. 18. [fig. 18(i) and fig. 18(ii)]: A cone, base 45 mm diameter and axis 55 mm long is resting on the H.P. On its base. It is cut by a section plane, perpendicular to both the H.P. And the V.P. And 6 mm away from the axis. Drawits front view, top view and sectional side view.
The section will be a line, perpendicular to xy, in both the front viewand the top view. The side view will show the true shape of the section. The widthof the section at any point, say c', will be equal to cc1 obtained by the circlemethod [fig. 18(i)].
(i) Draw the side view of the cone.
(ii) Project the points (on the section) in the side view taking the widths from thetop view. For example, through c' draw a horizontal line. Mark on it points c"and c"1 equidistant from and on both sides of the axis so that c" c"1 = cc1.
(iii) Draw a curve through the points thus obtained. It will be a hyperbola.
Fig. 18 (ii) shows the views obtained by the generator method.
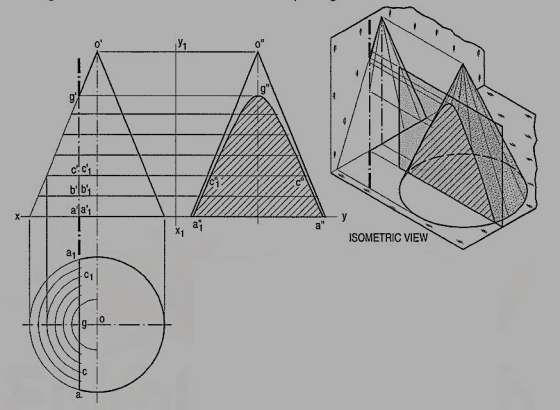
Figure 18 (i)
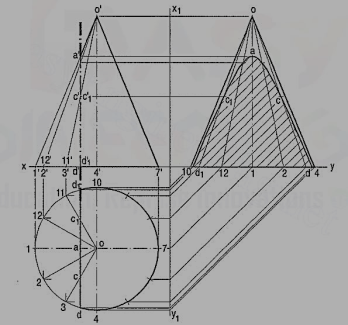
Figure 18 (ii)
Sections of Spheres:
(1) Section plane parallel to the H.P.
(2) Section plane parallel to the V.P.
(3) Section plane perpendicular to the V.P. And inclined to the H.P.
(4) Section plane perpendicular to the H.P. And inclined to the V.P.
(1) Section plane parallel to the H.P.: When a sphere is cut by a plane, the true shape of the section is always a circle.
The sphere in fig. 19 is cut by a horizontal section plane. The true shape of the section (seen in the top view) isa circle of diameter a'a'. The width of the section at any point say b', is equal to the length of the chord bb.
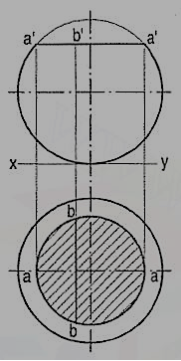
Figure 19
(2) Section plane parallel to the V.P.: When the sphere is cut by a section plane parallel to the V.P. (fig. 20), the true shape of the section, seen in the front view, is a circle of diameter cc. The width of section at any point d is equal to the length of the chord d'd'.
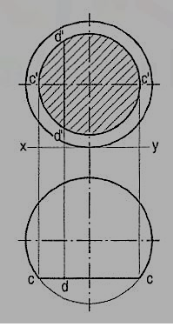
Figure 20
(3) Section plane perpendicular to the V.P. And inclined to the H.P.:
Problem (fig. 21): A sphere of 50 mm diameter is cut by a section plane perpendicular to the V.P., inclined at 45° to the H.P. And at 70mm from its center. Draw the sectional top view and true shape of the section.
Draw a line (for the section plane) inclined at 45° to xy and tangent to the circle of 10 mm radius drawn with o' as center. Mark many points on this line.
Method I:
(i) Find the width of section at each point in the top view as shown in fig. 19. For example, the chord cc is the width of section at the point c'.
(ii) Draw a curve through the points thus obtained. It will be an ellipse. The true shape of the section will be a circle of diameter a'g'.
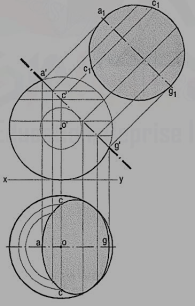
Figure 21
Method II:
It is known that the true shape of the section is a circle of diameter equal to a'g'. The width of section at any point say c' is equal to the chord c1c1 on this circle. Therefore, project c' to points c in the top view so that cc = c1c1. Similarly, obtain other points and draw the ellipse through them. Fig. 21 shows the sectional front view and true shape of the section when the section plane is vertical and inclined to the V.P.
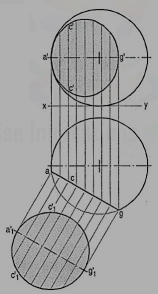
Figure 22
Section plane perpendicular to the H.P. And inclined to the V.P.:
Problem (fig. 23): The projections of a hemisphere 50 mm diameter, placed centrally on the top of a frustum of a hexagonal pyramid, base 32 mm side, top 20 mm side and axis 50 mm long are given. Draw the sectional front view when the vertical section plane H.T. Inclined at 45° to the V.P. And 10 mm from the axis, cuts them. Also draw the- true shapes of the sections of both the solids.
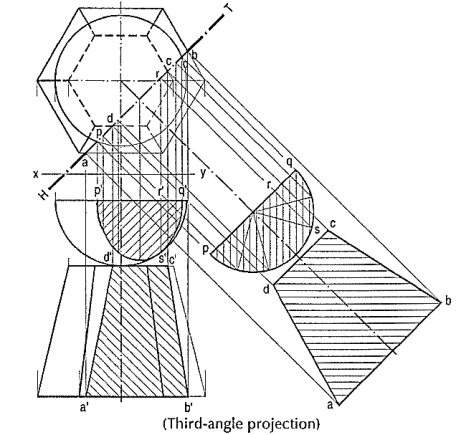
Figure 23
The widths of the section of the sphere at various points are obtained from the semi-circle drawn in the top view.
Problems:
1. A cube of 50 mm long edges is resting on the H.P. With a vertical face inclined at 30° to the V.P. It is cut by a section plane, perpendicular to the V.P., inclined at 30° to the H.P. And passing through a point on the axis, 38 mm above the H.P. Draw the sectional top view, true shape of the section and development of the surface of the remaining portion of the cube.
2. A hexagonal prism, side of base 35 mm and height 75 mm is resting on one of its corners on the H.P. With a longer edge containing that corner inclined at 60° to the H.P. And a rectangular face parallel to the V.P. A horizontal section plane cuts the prism in two equal halves.
(i) Draw the front view and sectional top view of the cut prism.
(ii) Draw another top view on an auxiliary inclined plane which makes an angle of 45° with the H.P.
Applications of development of surfaces
Imagine that a solid is enclosed in a wrapper of thin material, such as paper. If this covering is opened out and laid on a flat plane, the flattened-out paper is the development of the solid. Thus, when surfaces of a solid are laid out on a plane, the figure obtained is called its development.
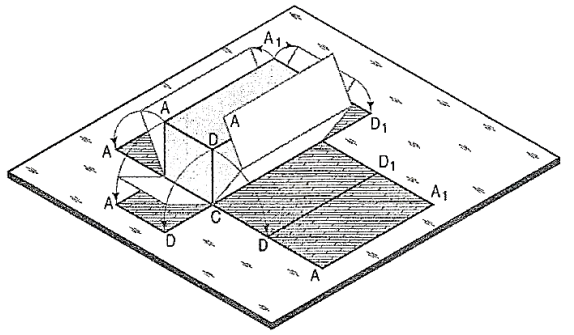
Figure 1
Fig. 1 shows a square prism covered with paper in process of being opened out. Its development (fig. 2) consists of four equal rectangles for the faces and two similar squares for its ends. Each figure shows the true size and shape of the corresponding surface of the prism. The development of a solid, thus represents the actual shape of all its surfaces which, when bent or folded at the edges, would form the solid.
Hence, it is very important to note that every line on the development must be the true length of the corresponding edge on the surface.
The knowledge of development of surfaces is essential in many industries such as automobile, aircraft, ship building, packaging and sheet-metal work. In construction of boilers, bins, process-vessels, hoppers, funnels, chimneys etc., the plates are marked and cut according to the developments which, when folded, form the desired objects. The form of the sheet obtained by laying all the outer surfaces of the solid with suitable allowances for the joints is known as pattern.
Only the surfaces of polyhedra (such as prisms and pyramids) and single curved surfaces (as of cones and cylinders) can be accurately developed. Warped and double-curved surfaces are undevelopable. These can however be approximately developed by dividing them up into many parts.
Methods of development
The following are the principal methods of development:
1. Parallel-line development:
It is employed in case of prisms and cylinders in which stretch-out-line principle is used. Lines A-A and A1-A1in fig.2 are called the stretch-out Iines.
2. Radial-line development:
Itis used for pyramids and cones in which the true length of the slant edge or the generator is used as radius.
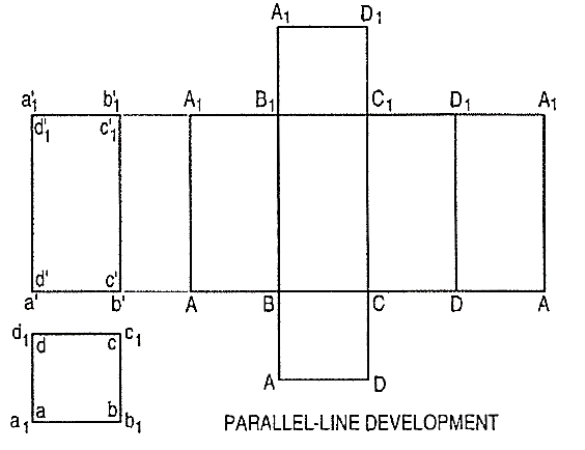
Figure 2
3. Triangulation development:
This is used to develop transition pieces. This is simply a method of dividing a surface into many triangles and transferring them into the development.
4. Approximate method:
It is used to develop objects of double curved or warped surfaces as sphere, paraboloid, ellipsoid, hyperboloid and helicoid.
Developments of lateral surfaces of right solids:
The methods of drawing developments of surfaces of various solids are explained by means of the following typical problems. Only the lateral surfaces of the solids (except the cube) have been developed. The ends or bases have been omitted. They can be easily incorporated if required.
Development of surfaces of above solids
Cube
The development of the surface of a cube consists of six equal squares, the length of the side of the squares being equal to the length of the edge of the cube.
Problem:
1. Draw the development of the surface of the part P of the cube, the front view of which is shown in fig. 3(i).Name all the corners of the cube and the points at which the edges are cut.
(i) Draw the stretch-out lines A-A and E-E directly in line with the front view, and assuming the cube to be whole, draw four squares for the vertical faces, one square for the top and another for the bottom as shown in fig. 3(ii).
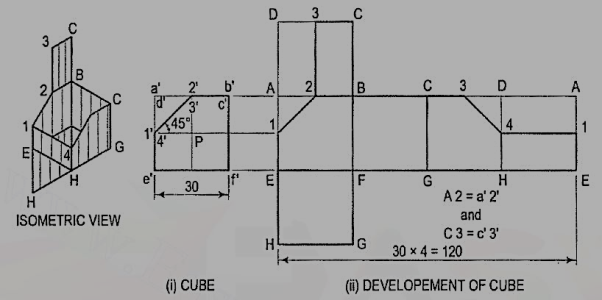
Figure 3
(ii) Name all the corners. Draw a horizontal line through 1' to cut AE at 1 andDH at 4. a' b' is the true length of the edge. Hence, mark a point 2 onAB and 3 on CD such that A 2 = a' 2' and C 3 = c' 3'. Mark the point3 on CD in the top square also.
(iii) Draw lines 1-2, 2-3, 3-4 and 4-1, and complete the development as shown. Keep lines for the removed portion, viz. A1, A2, 3D, D4 and DA thin and fainter.
2. Draw the development of the surface of the part P of the cube shown in two views in fig. 4(i).
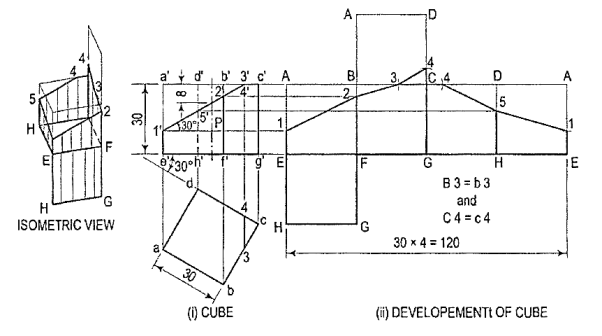
Figure 4
(i) Draw horizontal lines through points 1 ', 2' and 5' to cut AE in 1, BF in 2and DH in 5 respectively. Lines b'c' and c'd' do not show the true lengths of the edges. The sides of the square in the top view show the true length. Therefore, mark points 3 in BC and 4 in CD such that 83 = b3 and C4 = c4.
(ii) Draw lines joining 1, 2, 3 etc. in correct sequence and complete the required development. Keep the lines for the removed part fainter.
Prisms:
Development of the lateral surface of a prism consists of the same number of rectangles in contact as the number of the sides of the base of the prism. One side of the rectangle is equal to the length of the axis and the other side equal to the length of the side of the base.
Problem:
3. Draw the development of the lateral surface of the part P of the pentagonal prism shown in fig. 3 (i).
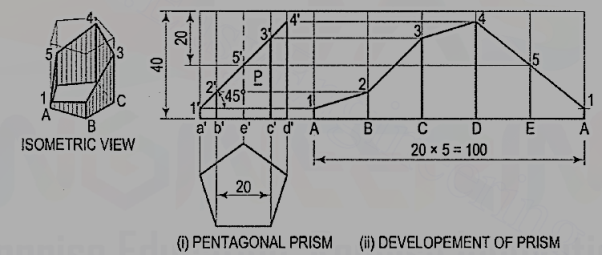
Figure 5
Name the corners of the prism and the points at which the edges are cut.
(i) Draw the development assuming the prism to be whole [fig. 5(ii)]. It is made up of five equal rectangles.
(ii) Draw horizontal lines through points 1’, 2’ etc. to cut the lines for the corresponding edges in the development at points 1, 2 etc.
(iii)Draw lines joining these points and complete the development as shown.
4. Draw the development of the lateral surface of the part P of the hexagonal prism shown in fig. 6 (i).
Name the points at which the edges are cut and draw the development assuming the prism to be whole [fig. 6(ii)].
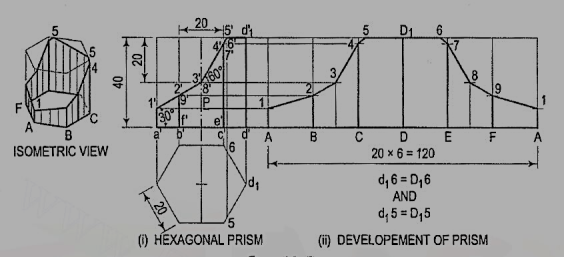
Figure 6
(i) Obtain all the points except 5 and 6 by drawing horizontal lines. Note that points 3 and 8 lie on vertical lines drawn through the mid-points of BC and EF.
(ii) Mark points 5 and 6 such that SD1 = 5d1 and D16 = d16.
(iii) Draw lines joining points 1, 2, 3 etc. in correct sequence and complete the required development as shown.
Cylinders
The development of the lateral surface of a cylinder is a rectangle having one side equal to the circumference of its base-circle and the other equal to its length.
Problem
1. Develop the lateral surface of the truncated cylinder shown in fig. 7(i).
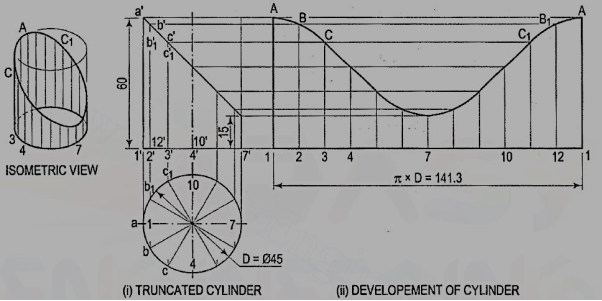
Figure 7
(i) Divide the circle in the top view into twelve equal parts. Project the division points to the front view and draw the generators. Mark points a', b' andb'1, c' and c'1 etc. in which the generators are cut.
(ii) Draw the development of the lateral surface of the whole cylinder along with the generators [fig. 7(ii)]. The length of the line 1-1 is equal to π x D (circumference of the circle). This length can also be marked approximately by stepping off with a bow divider, twelve divisions, each equal to the chord-length ab. (The length thus obtained is about 1 %shorter than the exact length; but this is permitted in drawing work.)
(iii) Draw horizontal lines through points a', b' and b'1 etc. to cut the corresponding generators in points A, B and B1 etc. Draw a smooth curve through the points thus obtained. The figure 1-A-A-1 is the required development.
Pyramids
The development of the lateral surface of a pyramid consists of many equal isosceles triangles in contact. The base and the sides of each triangle are respectively equal to the edge of the base and the slant edge of the pyramid.
Method of drawing the development of the lateral surface of a pyramid:
(i) With any point O as centre and radius equal to the true length of the slant edge of the pyramid, draw an arc of the circle. With radius equal to the true length of the side of the base, step-off (on this arc) the same number of divisions as the number of sides of the base.
(ii) Draw lines joining the division-points with each other in correct sequence and with the centre for the arc. The figure thus formed (excluding the arc) is the development of the lateral surface of the pyramid.
Problem:
1. Draw the development of the lateral surface of the part P of the triangular pyramid shown in fig. 8 (i). The line o'1' in the front view is the true length of the slant edge because it is parallel to xy in the lop view. The true length of the side of the base is seen in the top view.
(i) Draw the development of the lateral surface of the whole pyramid [fig. 8(ii)]as explained above. On 01 mark a point A such that OA = o'a'. o'2' (with which o'3' coincides) is not the true length of the slant edge.
(ii) Hence, through b', draw a line parallel to the base and cutting o' a' at b".o'b" is the true length of o'b' as well as o'c'. Mark a point B in 02 and C in 03 such that OB = OC = o'b".
(iii) Draw lines AB, BC and CA and complete the required development as shown. Keep the arc and the lines for the removed part fainter.
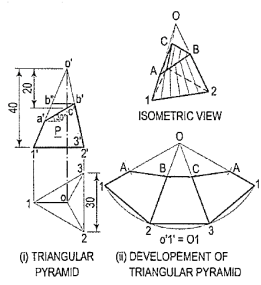
Figure 8
2. Draw the development of the lateral surface of the frustum of the square pyramid shown in fig. 9 (i).
(i) Determine the position of the apex. None of the lines in the front view shows the true length of the slant edge. Therefore, draw the top view and make any one line (for the slant edge) horizontal, i.e. parallel to xy and determine the true length o'1 '1. Through a', draw a line parallel to the base and obtain the true length o'a".
(ii) With O as centre and radius o'1 '1, draw an arc and obtain the development of the lateral surface of the whole pyramid [fig. 9(ii)].
(iii) With centre O and radius o' a", draw an arc cutting O1, O2 etc. at points A, B etc. respectively.
(iv) Draw lines AB, BC, CD and DA and complete the required development. Note that these lines are respectively parallel to lines 1-2, 2-3 etc.
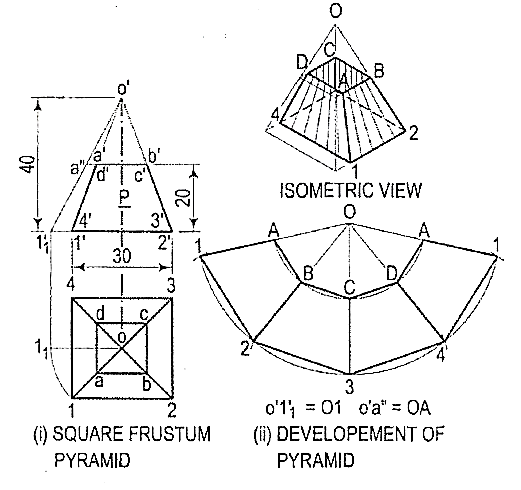
Figure 9
3.A frustum of a square pyramid has its base 50 mm side; top 25 mm side and height 75 mm. Draw the development of its lateral surface.
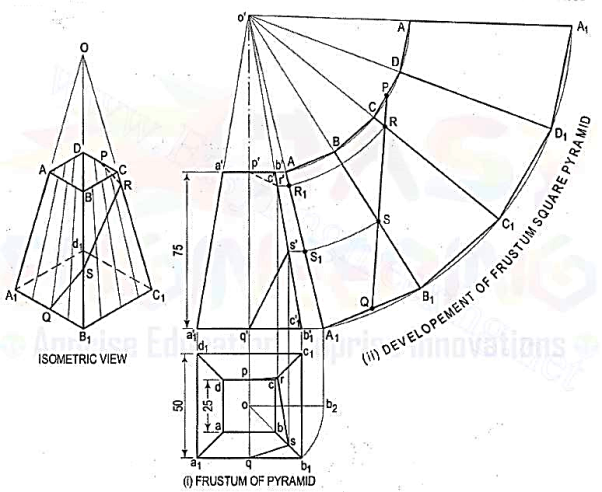
Figure 10
(i) Mark the mid-point P of CD and Q of A1B1. Draw a line joining P and Q and cutting CC1 at R and BB1 at S. Transfer these points to the front view and the top view. For example, with o' as centre and radius o'R, draw an arc cutting o' A1 at R1. Through R1, draw a line parallel to the base and cutting c'c'1 at r'. Project r' to r on cc1 in the top view. r' and r are the projections of R.
(ii) Similarly, obtain s' and s on b'b'1 and bb1 respectively. Draw lines pr, rs and sq which will show the top view of the line PQ. p'r's'q' will be the path of the line PQ in the front view.
Cone
The development of the curved surface of a cone is a sector of a circle, the radius and the length of the arc of which are respectively equal to the slant height and the circumference of the base-circle of the cone.
Problem:
1. Draw the development of the lateral surface of the truncated cone shown in fig. 11 (i).

Figure 11
Assuming the cone to be whole, let us draw its development.
(i) Draw the base-circle in the top view and divide it into twelve equal parts.
(ii) With any point O as centre and radius equal to o'1' or o'7', draw an arc of the circle [fig. 15-24(ii)]. The length of this arc should be equal to the circumference of the base circle. This can be determined in two ways.
(iii) Calculate the subtended angle θ by the formula,
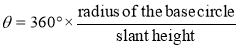
Cut-off the arc so that it subtends the angle θ at the center and divide it into twelve equal parts.
(iv) Step-off with a bow-divider, twelve equal divisions on the arc, each equal to one of the divisions of the base-circle.
(This will give an approximate length of the circumference. Note that the base-circle should not be divided into less than twelve equal parts.)
(v) Join the division-points with 0, thus completing the development of the whole cone with twelve generators shown in it [fig. ,11(ii)].
(vi) The truncated portion of the cone may be deducted from this development by marking the positions of points at which generators are cut and then drawing a curve through them. For example, generators o'2' and o'12' in the front view are cut at points b' and b'1 which coincide with each other. The true length of o' b' may be obtained by drawing a line through b', parallel to the base and cutting o'7' at b". Then o'b" is the true length of o'b'.
(vii) Mark points B and 81 on generators 02 and 0-12 respectively, such that OB = 0B1 = o' b". Locate all points in the same way and draw a smooth curve through them. The figure enclosed this curve and the arc is the development of the truncated cone.
2. Draw the development of the lateral surface of the part P ofthe cone shown in fig. 12

Figure 12
Draw the development as explained in problem [fig. 12 (ii)]. For the points at which the base of the cone is cut, mark points A and A1 on the arcs2-3 and 11-12 respectively, such that A2 = A1 12 = a2. Draw the curve passing through the points A, B, C etc. The figure enclosed between this curve and the arcA-A1 is the required development.
3. Draw the projections of a cone resting on the ground on its base and show on them, the shortest path by which a point P, starting from a point on the circumference of the base and moving around the cone will return to the same point. Base of cone 61 mm diameter; axis 75 mm long.

Figure 13
(i) Draw the projections and the development of the surface of the cone showing all twelve generators (fig. 13). The development may be drawn attached to o'1 '.
(ii) Assume that P starts from the point 1 (i.e. point 1' in the front view). Draw a straight line 1 '1' on the development. This line shows the required shortest path. Let us take a point P4 at which the path cuts the generator o’4. Mark a point P"4 on o'1' such that o'P"4 = o'P4. This can be done by drawing an arc with o' as centre and radius equal to o'P 4 cutting o11'at P"4. Through P"4, draw a line parallel to the base cutting o'4' at P'4.Then p'4 is the position of the point p4 in the front view. Similarly, transfer all the points to the front view and draw the required curve through them. The curve at the back will coincide with the front curve.
(iii) Project these points to the top view on the respective generators. p'4 andp'10 cannot be projected directly. Hence, project p"4 to a point q on o1.With o as centre and radius equal to oq, draw an arc cutting o4 at p4 ando-10 at p10. Thus op4 = op10 = oq. A curve drawn through the points thus obtained will show the path in the top view.
Development of surfaces of cut solids. (No reverse development)
Pipes are used in many industries to convey hot or cold fluids. When two different sizes and shapes of pipes are joined using special pipe joint which is known as transition piece. In most cases, transition pieces are composed of plane surfaces and conical surfaces, the latter being developed by triangulation.
Problem:
1. In air-conditioning system a rectangular duct of 100 mm x 50 mm connects another rectangular duct of 50 mm x 25 mm through the transition piece as shown in fig. 14(i). Neglecting thickness of a metal sheet, develop the lateralsurface of the transition piece as shown in fig. 14(ii).
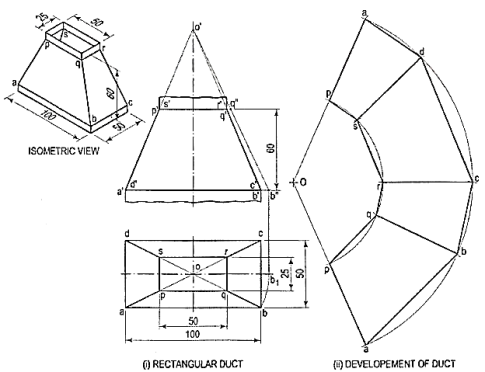
Figure 14
The transition piece is a frustum of a rectangular pyramid.
(i) Determine the position of the apex of the pyramid by extending a'p' and b'q'as shown. None of the lines in the front view shows the true length of theslant edge. Therefore, draw the top view and make any slant line parallel toxy and determine its true length o'b".
(ii) With O as centre and radius o'b", draw an arc and obtain the development ofa whole pyramid as shown.
(iii) With O as centre and radius o'q", draw an arc cutting oa, ob, oc at points p, q, etc. respectively. Join them in sequence and complete the development as shown.
2. An air-conditioning duct of a square cross-section70 mm x 70 mm connects a circular pipe of 40 mm diameter through the transition piece. Draw the projections and develop the lateral surface of the transition piece.

Figure 15
(i) Draw the front view and the top view as shown in fig. 15 (i).
(ii) Divide the top view of circle into some convenient divisions, say 16 parts as shown.
(iii) Note that the transition piece is composed of four isosceles triangles andfour conical surfaces. The seam is a long line 1-P.
(iv) Begin the development from the seam line 1-P (1 '-P'). As shown in fig. 15(ii)draw the right-angle triangle 1-P-b, whose base pb is equal to half the side ab and whose hypotenuse 1-b is equal to the true length 1 '-b' of side 1-b.
(v) The conical surfaces are developed by the triangulation method as follows.(vi) In the top view, join division of the circle 1, 2, 3 etc. with the corner a,b, c and d. Project them in the front view as shown. Obtain the true length of sides of each triangle as shown.
(vii) With b as centre and 2'b' (true length) radius draw an arc, cutting the arc drawn with 1' as centre and 1 '2' as radius. Similarly, obtain the points 3', 4', 5' etc. Join them in the proper order as shown.
References:
1. N. D. Bhatt, Engineering Drawing, Charotar Publishing House, 46th Edition, 2003.
2. K. V. Nataraajan, A text book of Engineering Graphic, Dhanalakshmi Publishers, Chennai, 2006.
3. K. Venugopal and V. Prabhu Raja, Engineering Graphics, New Age International (P) Ltd, 2008.
4. Dhananjay A. Jolhe, Engineering Drawing with an Introduction to Autocad,
McGraw Hill Education, 2017