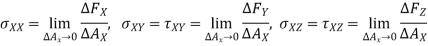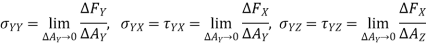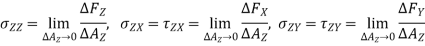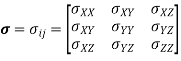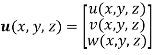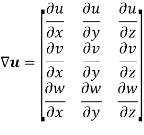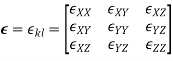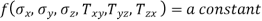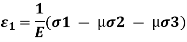Unit-2
Generalized state of stress and strain
Consider a body placed in a 3D coordinate system.
There are a number of forces acting on this body in different directions but the net force acting on the body is 0.
Consider a part of body sliced in yz-plane normal to X-axis.
Consider a small area on this plane and call it
△Ax = △Ax x*
The force acting on this small area is given as
△F = △Fx + △Fy + △Fz = △Fx x* + △Fy y* + △Fz z*
As this is in accordance with the area considered, as the area changes, the value of force also changes.
Now,
|
The first subscript is for the plane and second one for the direction. Similarly, we can also write,
|
The six scalars arranged in an 3x3 matrix form the stress tensor
|
Normal strain is change in dimension in a particular direction divided by the original dimension in that direction.
Shear strain is the complement of angle that is created due to distortion of body by application of force.
On application of force to a solid body, translatory and rotary motion maybe produced along with deformation.
The vector function defining the difference between original and final position of each particle of the body is given by,
|
Taking gradient of the above function,
|
This is the Strain tensor
For small strains, ∇u + ∇uT = 2ɛ, where
|
Yield point is the point where the stresses are strains cease to be proportional to each other.
This is specifically observed in the elastic material.
Yielding is defined the process of increase in deformation without any increase in the loading or stresses acting on the body.
In actual engineering applications, complex and multiaxial loads and stresses are present and under such situation, it is necessary to know when a material will yield.
The relations between the yield point under uniaxial tensile test and yield strength under complex situations are known as yield criteria.
Thus, yield criterion is defined as relationship between yield strength under uniaxial tensile load and yielding under multiaxial complex stress situation.
It is used to accurately predict if and when the yielding will occur in elastic material under the action of complex force system.
The general form of equation defining yield criterion in terms of stresses is
|
Maximum Principal Stress Theory (Rankine's Theory)
According to this theory, the failure of material will occur when the maximum principal stress in the complex stress system attains the value of the maximum stress at the elastic limit in simple tension or the minimum principal stress (i.e., maximum compressive stress) attains the elastic limit in simple compression. Thus, in this theory, maximum or minimum principal stress is the criterion of failure.
Let, σx, σy and τ be the direct and shear stresses acting on the body.
And σ1 be the maximum principal stress
Then,
|
This theory is accurate for brittle materials only.
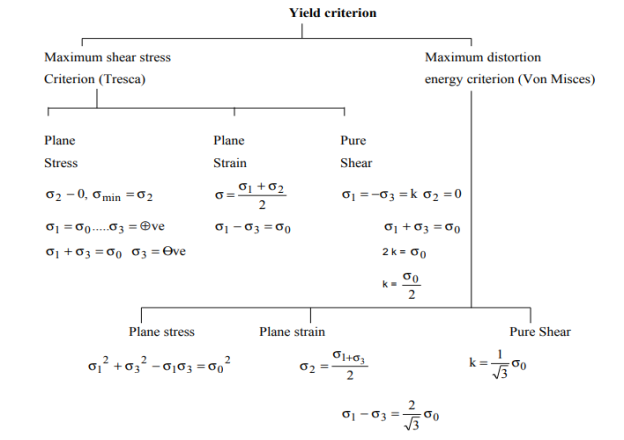
Maximum Shear Stress Theory (Guest's and Tresca's Theory)
This theory states that the failure occurs when the maximum shear stress in the complex system attains the value of the maximum shear stress at the elastic limit in simple tension. The maximum value of shear stress in terms of principal stresses σ1 and σ2,
τ = ½ (σ1 - σ2) = ½ √{(σy - σx)2 + 4 τ2}
|
This theory is accurate for ductile materials like mild steel.
Maximum Principal Strain Theory (St. Venant's Theory)
According to this theory, the maximum principal strain in the complex stress system must be less than the elastic limit in simple tension if there is to be no failure.
|
This theory is not much used since it gives accurate results only in some cases.
Maximum Strain Energy Theory (Haigh's Theory)
This theory is based on the principle that the work done in bringing a body to a particular state is independent of the method applied to bring the body to that state. According to this theory, the failure takes place when the strain energy per unit volume of a body reaches the value of strain energy at elastic limit in simple tension.
σ12 + σ22 + σ32 - 2µ (σ1 σ2 + σ2 σ3 + σ3 σ1) = σ2
|
This theory gives good results for ductile materials.
Maximum Shear Strain Energy Theory (Mises' and Henkey's Theory)
This theory states that the failure takes place when the shear strain energy in a complex system becomes equal to that in simple tension
Shear strain energy in a complex system
1 / 12G {(σ1 - σ2)2 + (σ2 - σ3)2 + ( σ3 - σ1)2}
|
Tresca found that the metal starts yielding when value of tangential stress crosses a certain limit.
If the body is subjected to stresses σ1 σ2 σ3 and σ1 > σ2 > σ3
The maximum shear stress is given by,
Tmax = (σ1 - σ3) / 2
|
According to Von Mises criteria, yielding begins when strain energy per volume reaches a certain value.
In terms of three principal stresses,
e = 1 / σ G {(σ1 - σ2)2 + (σ2 - σ3)2 + ( σ3 - σ1)2} |
|
In mechanics, Haigh-Westergaard's stress space or simply stress space is the 3D representation where each axis represents the principal stress acting on the body in respective direction.
In a 2D model, it becomes a plane.
|
The figure represents the triaxial stresses acting on a body. This is a Haigh-Westergaard Stress space example.
References:
1. Norris, C.H. and Wilber, J. B. and Utku, S. “Elementary Structural Analysis” Mc Graw Hill, Tokyo, Japan.
2. Timoshenko, S. and Young, D. H., “Elements of Strength of Materials”, DVNC, New York, USA.
3. Kazmi, S. M. A., ‘Solid Mechanics” TMH, Delhi, India.
4. Hibbeler, R. C. Mechanics of Materials. 6th ed. East Rutherford, NJ: Pearson Prentice Hall, 2004
5. Crandall, S. H., N. C. Dahl, and T. J. Lardner. An Introduction to the Mechanics of Solids. 2nd ed. New York, NY: McGraw Hill, 1979
6. Gere, J. M., and S. P. Timoshenko. Mechanics of Materials. 5th ed. Boston: PWS Kent Publishing, 1970.
7. Ashby, M. F., and D. R. H. Jones. Engineering Materials, An Introduction to their Properties and Applications. 2nd ed. Butterworth Heinemann.
8. Collins, J. A. Failure of Materials in Mechanical Design. 2nd ed. John Wiley & Sons, 1993.
9. Courtney, T. H. Mechanical Behavior of Materials. McGraw-Hill, 1990.
10. Hertzberg, R. W. Deformation and Fracture Mechanics of Engineering Materials. 4th ed. John Wiley & Sons, 1996.
11. Nash, W. A. Strength of Materials. 3d ed. Schaum's Outline Series, McGraw-Hill, 1994.