Unit 6
Three-phase voltage source inverter
For high power applications, three phase voltage source inverters are preferred to
Provide three phase voltage source in addition to that the magnitude, phase and frequency of voltages should be controlled.
The typical three-phase VSI topology is shown in fig below, and middle points of the inverter legs are connected to three phase RL load. There are the eight valid switch states which are given in Table. The switches of any leg of the inverter (S1and S4, S3 and S6 or S5 and S2) cannot be switched-on simultaneously. Because it would result in short circuit across the DC link voltage supply. Similarly, the switches of any leg of the inverter cannot be switched off simultaneously to avoid undefined states in the VSI and thus undefined ac output line voltages.
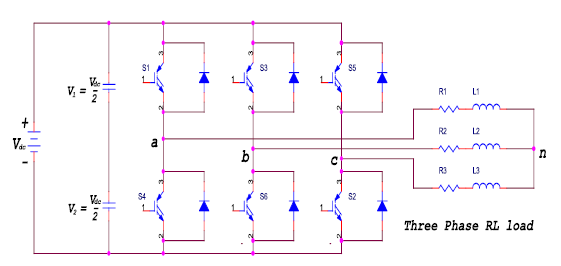
Fig: power circuit of three phase voltage source inverter with RL load
Two of eight valid states (7 and 8) are called as zero switch states to produce zero AC line voltages. In this case, the AC line currents freewheel through either the upper or lower components. The remaining states (1 to 6 in Table) are called as non-zero switch states to produce non-zero AC output voltages. The resulting AC output line voltages consist of discrete values of voltages that are Vdc , 0, and - Vdc for the topology shown in waveform figure below.
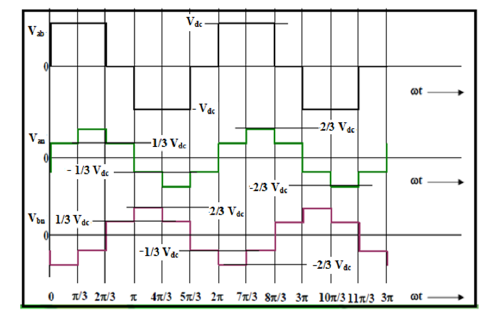
Fig: output voltage waveform of three phase VSI
The pole voltages and output voltage waveforms obtained with respectto switching states from three phase VSI are shown in waveform. Analysis of three phase VSI is carried out in either 1200 mode or 1800 mode of conduction.
1800 conduction Mode
In this mode each switch turned on at every600.Conduction of switches in each switching states, pole voltages measured at ‘a’ and ‘b’ and load voltage (Vab) are noted in the Table below.
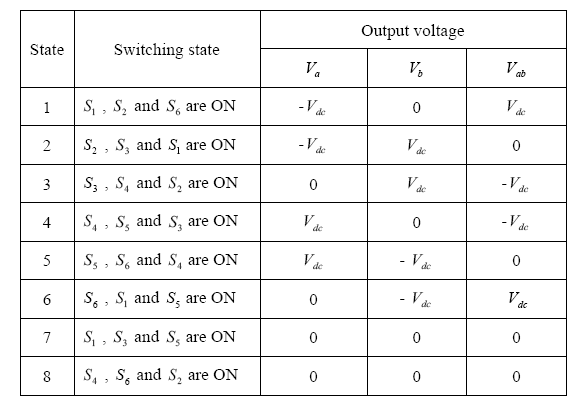
Table: switching states and output voltages of three phase VSI
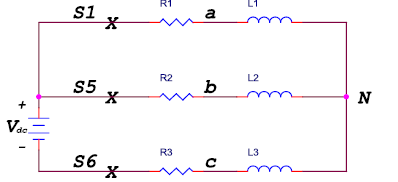
Fig: equivalent circuit representation at 0≤ωt≤π/3
With reference to abovefigure it is clear that for 0≤ωt≤π/3, thepower switches S6 ,S1 and 5S conduct and it will represent the equivalentinverter and load circuit during the above time interval. In such cases, when the load is a balanced one, it is easy to find thephase voltages for each phase and that can be given as
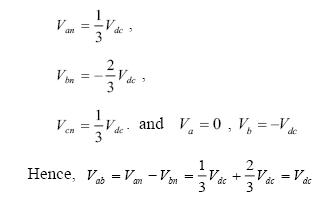
1200 conduction mode
In this type of control, only two transistors conduct at the same time such that each transistor conducts for 120˚ and remains OFF for 240˚. This means that only two transistors remain “ON” at any instant of time.
The conduction sequence of the transistors is : 1,6-1,2-2,3-3,4-4,5-1,6-1,2-2,3
During mode-1 for 0 ≤ ωt≤π/3 transistor 1and 6 conduct:
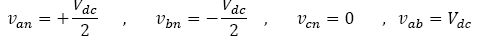
During mode-2 for π/3 ≤ ωt≤ 2π/3 transistor 1and 2 conduct:
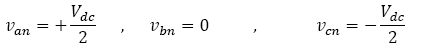
During mode-3 for 2π/3 ≤ ωt ≤ π transistor 3 and 2 conduct:
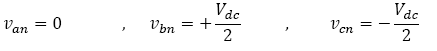
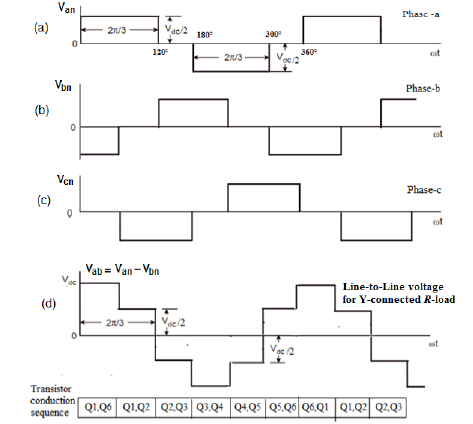
Fig: Voltage waveform in 1200 conduction mode
Mathematical analysis of 120˚ Mode inverter
The rms value of the output voltage waveform of 120˚ mode inverter for phase-a can be obtain as,
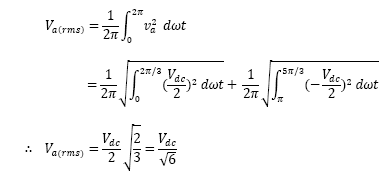
The line-to-line voltages for star-connected load can be found as:
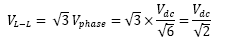
The voltage source inverter that use PWM switching techniques have a DC input voltage (VDC = VS) that is usually constant in magnitude. There are several techniques of Pulse Width Modulation (PWM).The efficiency parameters of an inverter such as switching losses and harmonic reduction are principally depended on the modulation strategies used to control the inverter.
The Sinusoidal Pulse Width Modulation (SPWM) technique has been used for controlling the inverter as it can be directly controlled the inverter output voltage and output frequency according to the sine functions.
SPWM techniques are characterized by constant amplitude pulses with different duty cycles for each period. The width of these pulses are modulated to obtain inverter output voltage control and to reduce its harmonic content.
In SPWM technique three sine waves and a high frequency triangular carrier wave are used to generate PWM signal.
Generally, three sinusoidal waves are used for three phase inverter. The sinusoidal waves are called reference signal and they have 1200 phase difference with each other.
The carrier triangular wave is usually a high frequency (in several KHz) wave. The switching signal is generated by comparing the sinusoidal waves with the triangular wave. The comparator gives out a pulse when sine voltage is greater than the triangular voltage and this pulse is used to trigger the respective inverter switches. In order to avoid undefined switching states and undefined AC output line voltages in the VSI, the switches of any leg in the inverter cannot be switched off simultaneously.
The phase outputs are mutually phase shifted by 1200 angles The ratio between the triangular wave & sine wave must be an integer N, the number of voltage pulses per half-cycle, such that, 2N= fc /fs.
Current Source Inverter
The current source inverter (CSI) is a device that converts the input direct current into an alternating current. It is also called current fed inverter in which the output current is maintained constant irrespective of load on the inverter. This means that, the magnitude and nature of the load current depends on the nature of load impedance. The output voltage of the inverter is independent of the load. The major advantage of current source inverter is its reliability.
In the case of current source inverter a commutation failure in the same leg does not occur due to the presence of a large inductance Ld connected in series with the voltage source.
Compared with a VSI system, the output current of a CSI system is not influenced by the supply voltage, so its output current has low THD and high PF.
Therefore, due to its advantages, the current source inverters are used in many industrial applications such as
Induction heating,
Static var compensators (SVC) ,
Variable speed a.c. Motors etc.
The freewheeling diodes that are used for voltage source inverters become useless if an inverter is supplied from a d.c. Current source, this is because the current in any half-leg of the inverter cannot change its polarity, hence it can only flow through the power semiconductor switches. Therefore, absence of the freewheeling diodes reduces the size and weight of the inverter circuit.
Single-Phase Current Source Inverter
The following Fig(a) shows the circuit of a single-phase current source inverter. A constant current source is used here with a large value of inductance Ld in series with the current limited d.c. Voltage source Vdc.
The thyristors of the inverter circuits are, T1 ,T3, T2 and T4 , are alternatively turned ON to obtain a nearly square wave current waveform. Two commutating capacitors – C1 in the upper half, and C2 in the lower half, are used.
Four diodes, D1, D2, D3 and D4 are connected in series with each thyristor to isolate the commutating capacitors from the load and prevent them from discharging through the load.
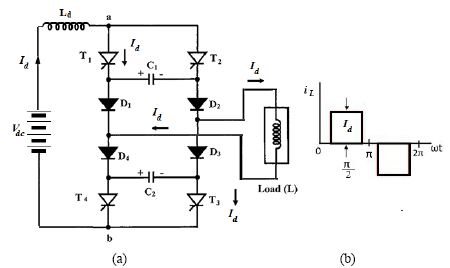
Fig (a) circuit of single phase CSI (b) output current waveform
Working
The output frequency of the inverter is controlled by varying the half time period, (T/2), at which the thyristors in pair are triggered by pulses being fed to the respective gates by the control circuit, to turn them ON. The operation of the circuit can be described as :
In the circuit of fig(a), two thyristors must trigger simultaneously to allow current to flow. For example, T1 and T3 must be triggered, while in reverse, T2 and T4 must trigger at the same time. The output current waveform is shown in fig(b) for the case of inductive load.
When T1 andT3 conduct, capacitors C1 and C2 would be charged with the polarity as shown. When T2 andT4 are turned on, thyristorsT1 and T3 are reversed biased by the capacitors C1 and C2 respectively to commutate them. At this instant, the load current flows through T2 – C1 – D1 – load – D3 – C2 – T4 charging capacitors C1 and C2 with opposite polarity and are ready now to commutate T2 and T4 , while the current in the load changes its direction and the cycle is repeated.
Three-Phase Current Source Inverter
The circuit of a three-phase current source inverter is shown in Fig. As in the circuit of a single-phase CSI, the input is also a constant current source. In this circuit, six thyristors, two in each of three arms, are used, as in a three-phase VSI. Also, six diodes D1, D2, D3, D4, D5 and D6, each one in series with the respective thyristor, are needed here, as used for single-phase CSI.
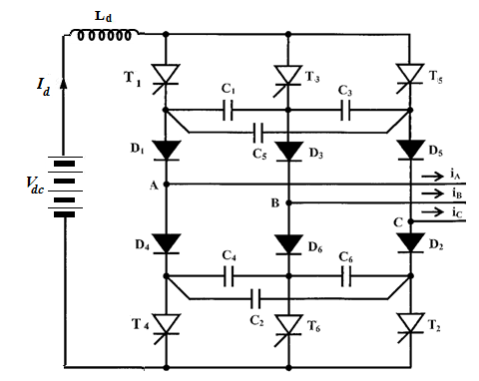
Fig: circuit of three phase CSI
Six capacitors, three each in two (top and bottom) halves, are used for commutation. The six capacitors are equal, i.e. C1= C2 = C3.....= C6. The six diodes are needed in CSI, so as to prevent the capacitors from discharging through the load. Three Phase inverters are normally used for high power applications.
Modes of Operations
The three phase output can be obtained from a configuration of six switches and six diodes. Two types of control signals can be applied to the switches: 180°conduction or 1200 conduction.
1800 Conduction Mode
In these inverters each switch conducts for a duration of 1800. Three switches remain on, at any instant of time. When switch-1 is switched on, terminal 'a' is connected to the positive terminal of the DC input voltage.
When switch-4 is switched on, terminal 'b' is connected to the negative terminal of the DC source. There are six modes of operation in a cycle and the duration of each mode is 600 .The switches are numbered in the sequence of gating the switches 1-2-3, 2-3-4, 3-4-5, 4-5-6, 5-6-1, 6-1 -2. The gating signals are shifted from each other by 600 to obtain three phase balanced voltages.
1200 Conduction Mode
In this conduction mode each switch conducts for 120". Only twoswitches remain on at any instant of time. The conduction sequence of switchesis 6-1, 1-2, 2-3,3-4,4-5, 5-6, and 6-1. There are three modes of operation in a half cycle and the equivalent circuits.
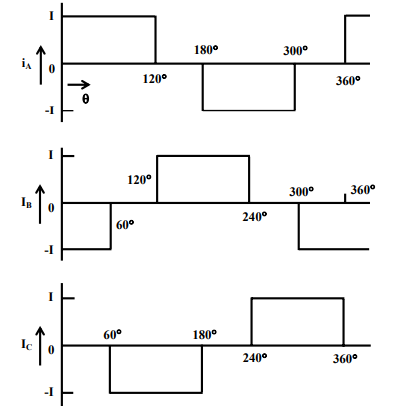
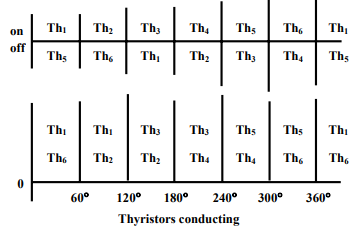
Fig: phase current waveform
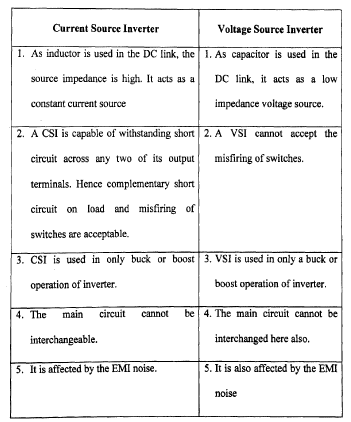
Comparison between VSI and CSI
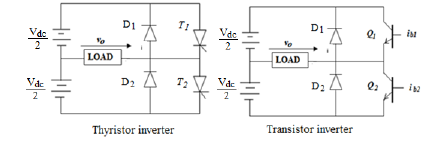
Ex.1 The single-phase half-bridge transistor inverter shown in Fig.below has a resistive load of R = 3Ω and the d.c. Input voltage Vdc= 60 V. Determine:
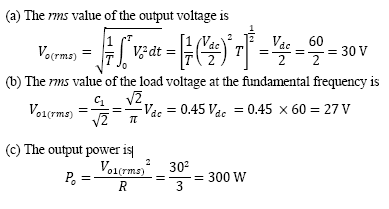
(a) The rmsvalue of the output voltage.
(b) The rmsvalue of the load voltage at the fundamental frequency .
(c) The output power.
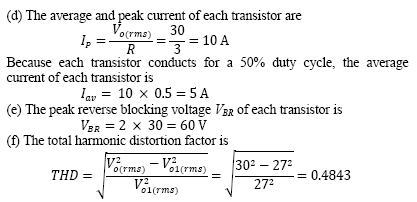
(d) The average and peak current of each transistor.
(e) The peak reverse blocking voltage VBR of each transistor.
(f) The total harmonic distortion factor.
Ans:
Ex.2 For the single-phase MOSFET bridge inverter circuit shown below, the source Vdc=125 V, load resistance R =10 Ω and output voltage frequency fo= 50 Hz.
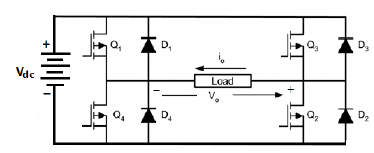
a) Draw the output voltage and load current waveforms.
(b) Derive the rms value of the output voltage waveform and hence calculate the output power Po in terms of the output voltage.
(c) Analyse the amplitude of the Fourier series terms of the output voltage waveform by considering up to the 7th order harmonic. Determine the value of the rms output voltage in terms of harmonics rms values.
(d) Calculate the average and peak currents of each transistor.
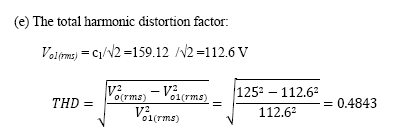
(e) Estimate the total harmonic distortion factor THD of the circuit.
Ans:
(a)
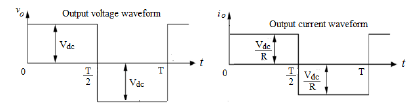
(b) The rmsvalue of the output voltage is
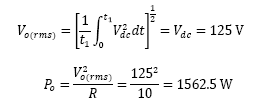
(c) The Fourier series of the output voltage is
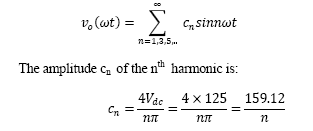
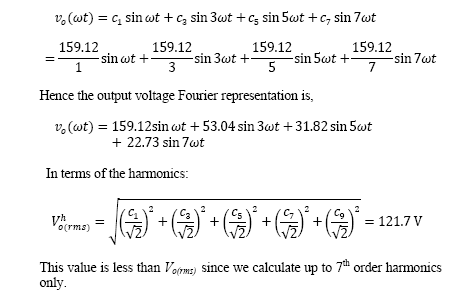
(d) Since the duty cycle of each transistor is 0.5, the current waveform is as shown below
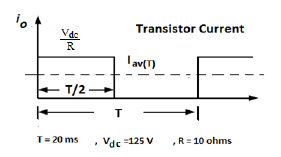

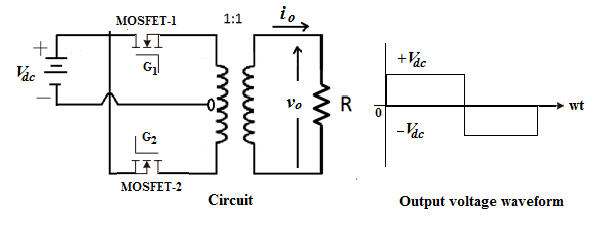
Ex.3 A single-phase MOSFET parallel inverter has a supply d.c. Voltage of 100V supplying a resistive load with R =10 Ω via a center-tap transf- ormer with 1:1 ratio. The output frequency is 50 Hz.
(a) Draw the circuit diagram and the output voltage waveform of the inverter.
(b) Determine the rms value of the output voltage waveform.
(c) Determine the amplitude of the Fourier series terms for the square output voltage waveform up to 9th order harmonics.
(d) Calculate the rms value of the output voltage in terms of harmonic components that obtained in (b).
(e) Determine the power absorbed by the load consider up to 9th order harmonic.
(f) Draw the frequency spectra of the output voltage waveform.
(g) Calculate the total harmonic distortion factor THD.
Ans: (a) the circuit diagram and the output voltage waveform of the inverter
(b) The rmsvalue of the output voltage is
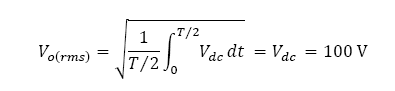
(c) the Fourier series of the output voltage is
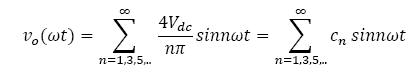
The amplitude cnof the n th order harmonic is:
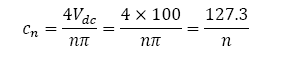

Hence the output voltage Fourier representation is,
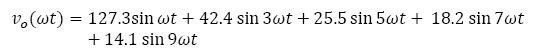
(d) In terms of the harmonics , the rmsvalue of the output voltage is

(e) To calculate the power we most calculate the rmsvalue of the current for each harmonic the amplitude of the nth harmonic current
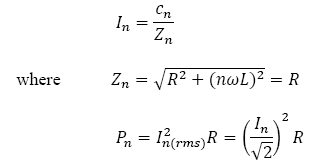
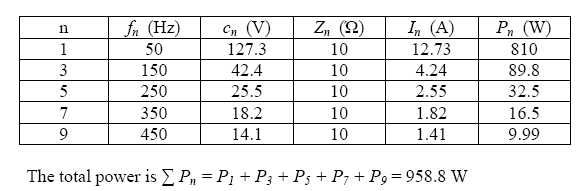
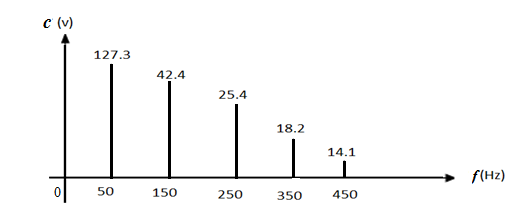
(f) The frequency spectrum is given in Fig.
(g) The total harmonic distortion factor
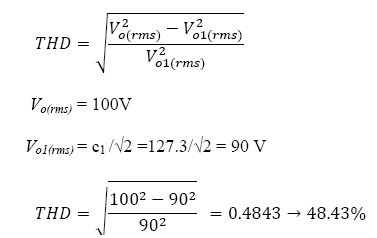
This is very high THD , the practical value of THD is about (3-10)% hence we need to use low-pass filter at the output to filter out most of the undesirable harmonic component and to produce nearly sinusoidal output waveform.
Ex.4 The three-phase inverter in Fig. Shown used to feed a Y-connected resistive load with R =15 Ω per-phase. The d.c. Input to the inverter Vdc= 300 V and the output frequency is 50 Hz. If the inverter is operating with 120˚ conduction mode, calculate : (a) The peak and rms value of the load current IL, (b) The output power, and the average and rms values of the current of each transistor.
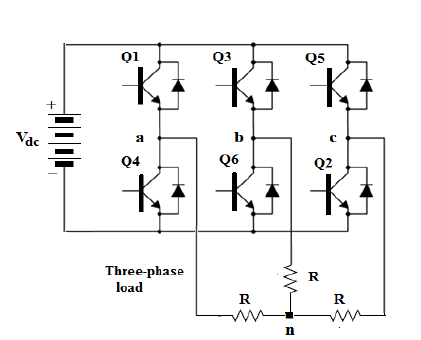
Ans:
(a) For 120ᵒ conduction mode, at any time the load resistances of two phases are connected in series, hence, peak value of load current is
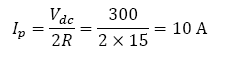
Therms value of the phase voltage is
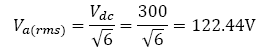
Hence the rms value of the load current is
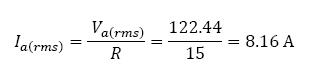
(b) The load power is
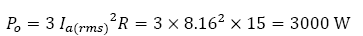
(c) For 120ᵒ conduction mode, each transistor carries current for (1/3)rd of a cycle, hence the average transistor current is
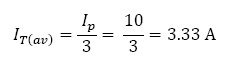
The rmsvalue of the thyristor current is
