Unit - 3
Intermolecular forces and properties of gases
The properties like melting point and boiling point can be a measure to show how strong the attractive forces exist between molecules are individual molecules. We can also call these as intermolecular forces. The general principle being as bons become more polarized, the charges on the atoms become greater, subsequently the intermolecular attraction increases, which leads to higher boiling point.
The three intermolecular forces are as follows
Are the interactions between charged molecules or atoms (ions).
Positively charged ions, are Na (+), Li (+), and Ca (+), they are called as Cations, on the other hand the negatively charged ions are Cl (-), Br (-), Ho (-) they are Anions. The attractive forces between ions that are oppositely charged is described under the Coulomb’s Law, as the law says the force increases with charge and decreases as the distance between these ions increases. The highly polarised or charged nature of the ionic molecules is reflected in the nature of their melting point. For e.g., NaCl has a melting point of 801oc. The ionic molecules also show the property of high solubility in water.
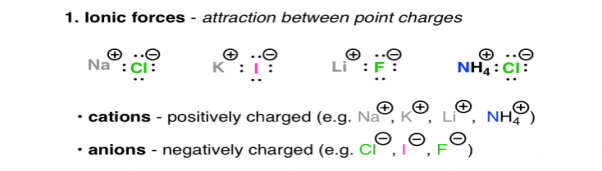
Fig. 1: Ionic forces – attraction between point charges
Hydrogen bonding occurs in molecules that are highly electromagnetic in nature, elements like F, O or N are directly bound to hydrogen. As hydrogen has an electronegativity of 2.2, they are not as polarized as ionic bonds and have some covalent character. But still the hydrogen bond is polarized and possess a dipole.
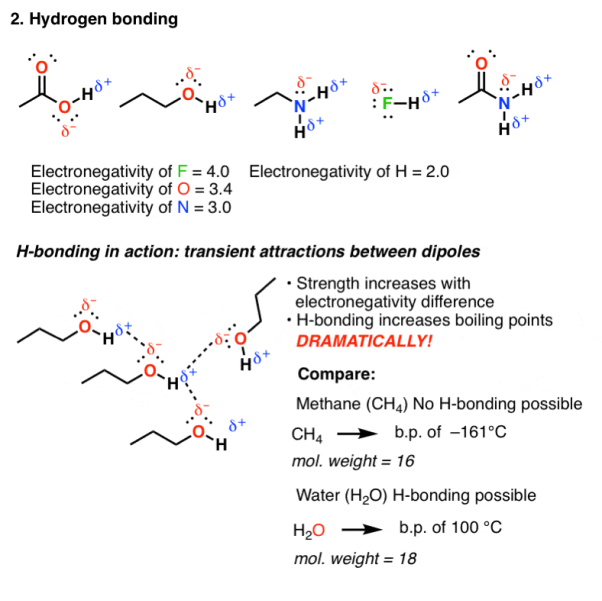
Fig. 2: Hydrogen bonding
Hydrogen bonding is an attractive interaction that occurs when the dipole of one molecule can align with the dipole from another molecule. Since the molecular motion of these molecules are rapid in a solution, these bonds are short lived therefore HO and NH are bonding molecules containing these functional groups that tend to have high boiling point.
Other groups other than hydrogen can be involved in covalent bonding with strong electronegative atoms. For example, in the figure given below each of the molecules contain a dipole:
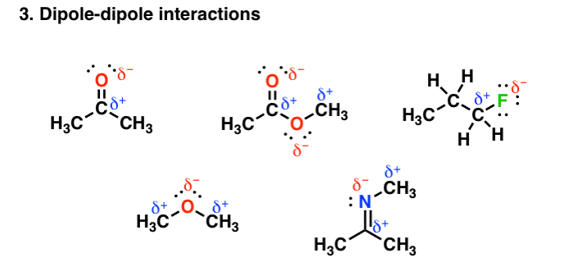
Fig. 3: Dipole-dipole interaction
These dipoles interact with each other in a very unique and attractive manner and thereby increase the boiling point. But the main aspect is the bonding depends on the electronegativity differences. The electronegativity of carbon =2.5, but that of Oxygen and Nitrogen is less than hydrogen whose electronegativity is=2.2, therefore the polar interaction is not so very strong. So, on an average these forces tend to be weaker than in hydrogen bonding
To sum up, boiling points are a measure of intermolecular forces and boiling point increases with molecular weight and surface area. The intermolecular forces increase with the increase in polarization of bonds.
The ideal gas law is one of the simplest equations of state, although it is accurate for gases at low pressure and high temperatures, but becomes relatively inaccurate at high pressure and low temperatures. The ideal gas law is used in various fields like science and engineering, due to its simple equation.
Ideal Gas Law: is an equation state of an ideal gas, it combines many gas laws together (Daltons law, Boyles law, Avagadro’s law)
P=Ρrt
Here p is the pressure of gas; is the density of the gas equal to m/V; m is the mass of the gas occupying volume V; V the volume it occupies, R the gas constant for the given gas; and T the temperature of the gas In SI units, the pressure is expressed in pascals, the volume in cubic meters, and the temperature in degrees Kelvin. The ideal gas law generalizes the three classical gas laws.
The equation is valid only for an ideal gas. Real gases obey this equation only approximately but its validity increases as the density of the gas tends to zero.
Ideal gas
Ideal gas (also called perfect gas) is a hypothetical gas which obeys the gas laws exactly. An ideal gas consists of molecules which occupy negligible space and among which negligible forces exist, except at collisions. All collisions between molecules or between molecules and the wall of a container are perfectly elastic, as the molecules have no means of storing energy except as translational kinetic energy. Ideal gas is a good approximation for diluted real gases.
At lower temperatures and higher densities, it may be necessary to treat quantum-mechanical effects statistics even before those of interactions. In such cases, of an ideal gas is extended to that of an ideal Fermi gas or Bose gas.
Van der Waals Equation Derivation
In a real gas the molecular volume is not negligible, also cohesive or repulsive intermolecular forces mean that the pressure applied on the containing vessel is less than that of an Ideal gas. Therefore, the equation of state needs that the pressure p is increased by a quantity proportional to the density or, by the quantity inversely proportional to the volume. The van der Waals equation has played an important role in describing fluids, i.e., both liquids and gases.
Van der Waals equation is also known as Van der Waals equation of state for real gases and is not applicable or follows ideal gas law. According to the state of ideal gas law, PV = nRT where P is the pressure, V is the volume, n is the number of moles, T is the temperature and R is the universal gas constant. The Van der Waals Equation derivation is explained below.
Derivation of Van der Waals equation
For the state of real gas, using Van der Waals equation, the volume of a real gas is given as (Vm – b), where b is volume occupied by per mole.
Therefore, ideal gas law when substituted with V = Vm – b is given as:
P(Vm−b) =nRT
Because of intermolecular attraction P was modified as below
(P+a/Vm2) (Vm−b) =RT
(P+an2/V2) (V−nb) =nRT
Where,
Vm: molar volume of the gas
R: universal gas constant
T: temperature
P: pressure
V: volume
Thus, Van der Waals equation can be reduced to ideal gas law as PVm = RT.
The kinetic molecular theory has two predictions for real gases that cause problems in low temperatures and high pressures (as in real gases deviate from this idea behaviour). The kinetic molecular theory assumes that gas particles will only take up a minute fraction of the total volume of the gas.
The behaviour of real gases usually agrees with the assumptions of the ideal gas equation to within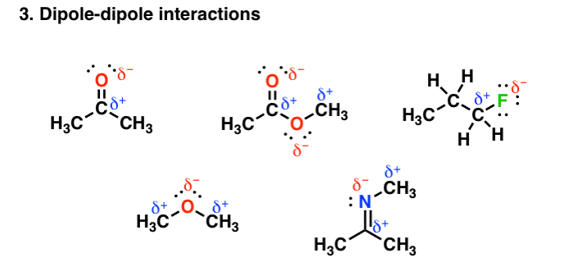 
5% at normal temperatures and pressures. At low temperatures or high pressures, real gases deviate significantly from ideal gas behaviour. In 1873, while searching for a way to link the behaviour of liquids and gases, the Dutch physicist Johannes van der Waals developed an explanation for these deviations and an equation that was able to fit the behaviour of real gases over a much wider range of pressures.
Van der Waals realized that two of the predictions of the kinetic molecular theory was a big question. The kinetic theory notifies that gaseous particle occupy a very minute fraction of the total volume of the gas. It also assumes that the force of attraction between gas molecules is negligible or zero.
The first assumption or prediction of the theory works at pressures close to 1 atmosphere. But something happens to the effectiveness of this assumption as the gas is compressed. At this moment the atoms or molecules in a gas were all clustered in one corner of a cylinder, as shown in the figure below. At normal pressures, the volume occupied by these gaseous particles is a very minute fraction of the total volume of the gas. But at high pressures, this does not hold true. So subsequently, real gases are not as compressible or suppressed at high pressures as an ideal gas. The volume of a real gas is thus larger than expected from the ideal gas equation at high pressures.
Van der Waals stated the fact that the volume of a real gas is too large at high pressures by deleting a term from the volume of the real gas before we substitute or add it into the ideal gas equation. He therefore introduced a constant
constant (b) into the ideal gas equation that was equal to the volume actually occupied by a mole of gaseous particles. because the volume of the gas particles depends on the number of moles of gaseous particles in the container, the term that is subtracted from the real volume of the gas is equal to the number (n) of moles of gaseous particles times b.
P (V - nb) = nRT
When the pressure is relatively less, and the volume is reasonably large, the nb term is very minute to make any difference in the calculation. But at relatively high pressures, when the volume of the gas is less, the nb term corrects for the fact that the volume of a real gas is larger than expected from the ideal gas.
Real gas is a theoretical gas that is composed of randomly moving, non-interacting particles. The ideal gas concept completely obeys the ideal gas law, which approximates that behaviour of gases under varying conditions.
The van der Waals Equation of state approaches the ideal gas law PV=nRT as the values of these constants are nil or zero. The constant provides a correction a for the intermolecular forces. The constant b is a correction for finite or limited molecular size and its value is the volume of one mole of the atoms or molecules. The ideal Gas equation predicts that a plot of PV versus for a gas would be a horizontal line because PV is a constant. Experimental data for PV versus P for H2 and N2 gas at 0oc and CO2 at 40c are given below.
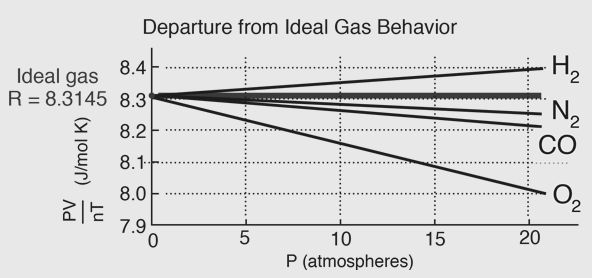
Fig. 5: Departure from Ideal gas behaviour
Values of the van der Waals constants for these and other gases are given in the table below.
Van der Waals Coefficients | ||
Gas | a (pa m6) | b (m3/mol) |
Helium | 3.46 x 10-3 | 23.71 x 10-6 |
Neon | 2.12 x 10-2 | 17.10 x 10-6 |
Hydrogen | 2.45 x 10-2 | 26.61 x 10-6 |
Carbon dioxide | 3.96 x 10-1 | 42.69 x 10-6 |
Water proof | 5.47 x 10-1 | 30.52 x 10-6 |
Fig. 6: Van der waals coefficients
The volume (V) occupied by n moles of any gas has a pressure (P) at temperature (T) in kelvin. The relationship for these variables,
PV=nRT
Where R is known as the gas constant, is called the Gas Law or Equation of State.
References:
