Unit - 4
Use of Free Energy in Chemical Equilibria and Water Chemistry
The branch which deals with the movement of energy from one form to the other and the relationship that exists between heat and temperature with energy and the work done is called as thermodynamics. Thermodynamics is a branch of science that deals with the different forms of energy and work done in a system. Thermodynamics is only confined to large scale response of a system which we are observed and measured in experiments
Energy:
It referred to the energy content that is present within the system. The energy represents the overall energy contained in the system and may include many forms of energy such as potential energy, kinetic energy etc. In a chemical reaction, we know the energy transformations and basic thermodynamics gives us information regarding energy change associated with the particles present in a system.
Factors affecting the internal energy
The internal energy of a system may change when:
Work:
Work done by a system is defined as the amount of energy exchanged between a system and its surroundings. Work is completely determined by external factors such as an external force, pressure or volume or change in temperature etc.
Heat:
Heat in thermodynamics is defined as the kinetic energy of the molecules of the substance or material. Heat and thermodynamics together form the basics which help process designers and engineers to optimize their processes and harness the energy associated with chemical reactions economically.
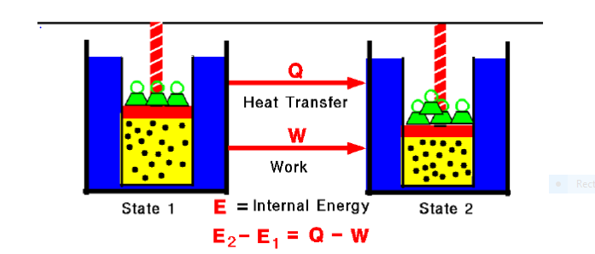
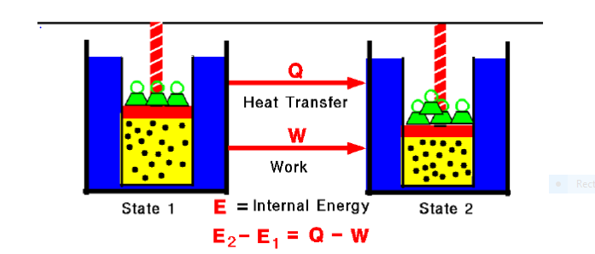
Fig. 1:
Any thermodynamic system in an equilibrium state possesses a state variable called the internal energy (E). Between any two equilibrium states, the change in internal energy is equal to the difference of the heat transfer into the system and work done by the system.
Enthalpy:
Enthalpy a process or activity takes place at constant pressure, the heat released or absorbed is equal to the Enthalpy change. Enthalpy is occasionally called or known as “heat content”, “enthalpy” is derived from the Greek word which means “warming”. Enthalpy(H) is the sum of the internal energy(U) and the result of pressure(P) and volume(V).
Enthalpy H is written as,
H = U + PVm
Where, H = is the Enthalpy of the system
U = is the Internal energy of the system and is entirely dependent on the state functions T, p and U.
Enthalpy can also be written as
ΔH=ΔU+ΔPV.
P = is the Pressure of the system
V = Volume of the system Enthalpy is not directly measured, but the change in enthalpy (ΔH) is measured, which is the heat lost or added by the system,
Entropy:
It was Introduced by the German Physicist Rudolf Clausius in 1850, and is a highlight of 19th century Physics. The word “entropy” is derived from the Greek word, which means “turning”. It was derived to provide a quantitative measure for the spontaneous changes and Clausius introduced the concept of entropy as a precise way of expressing the Second Law of Thermodynamics, Clausius form of the second law states that spontaneous change for an irreversible process in an isolated system is a measure of randomness or irregularity or disorder of the system?
The more or the randomness, higher is the entropy.
Solid state has the lowest or least entropy, the gaseous state has the highest entropy and the liquid state has the entropy that lies between the two.
Entropy is a state function. The changes in its value during any process, is called the entropy change.
ΔS = S2 -S1 = ∑S products – ∑S reactants
1) When a system absorbs heat, the molecules begin to move faster because the kinetic energy increases. therefore, disorder increases. Greater the heat absorbed, greater will be the disorder.
2) For equal amount of heat absorbed at low temperature, the disorder will be more than at high temperature. This proves that entropy change is inversely proportional to temperature.
ΔS = eve / T
Entropy change during a process is defined as the quantity of heat (q) absorbed isothermally and reversibly divided by the absolute Temperature (T) at which the heat is absorbed.
Free Energy
Gibbs Free Energy is a measure of the potential for reversible or maximum work that may be done by a system at constant temperature and pressure. It is a thermodynamic function that was discovered in 1876 by Josiah Willard Gibbs to assume if a process will occur spontaneously at constant temperature and pressure. Gibbs free energy G is defined as
G = H - TS
where H, T, and S are the Enthalpy, temperature, and entropy. The SI unit for Gibb’s energy is the kilojoule.
Gibbs free energy combines both the enthalpy and entropy into a single value.
Gibbs free energy is the energy t with a that associates itself with a chemical reaction. It equals the enthalpy minus the temperature of the product and the entropy of the system.
G=H-TS
At constant temperature
ΔG = ΔH – TΔS
ΔG predicts the direction of a chemical reaction. If ΔG value is negative, then the corresponding reaction is spontaneous. If ΔG value is positive then reaction is non-spontaneous.
ΔGº=ΔHº-TΔSº
Where
ΔGº = Gibbs free energy (J or KJ)
ΔHº=enthalpy
T=Temperature
ΔSº=Entropy
Gibbs free energy is the energy that is available to do quality work.
A reaction will spontaneously occur if ΔG<0 (exergonic reaction)
A reaction will not spontaneously occur if ΔG>0 (endergonic reaction).
if ΔG value is less than zero, there is a thermodynamic force for the reaction or it drives the process in the forward direction.
When ΔG is positive, then reactants are favoured, when ΔG=0 system is at equilibrium.
EMF
Electromotive force, or, as it is often written, e.m.f., is described as that source of energy which enables electrons movement around an electric circuit.
For any object to move from rest, there has to be some energy change. To ensure electrons movement round an electrical circuit, they should receive energy from a source of e.m.f. which usually is a battery or a generator.
For every coulomb of electricity to move completely around an electrical circuit, a certain amount of electrical energy is needed, which depends on the particular circuit. The e.m.f. is expressed in volts and is numerically the number of joules of energy given by the source of e.m.f. to each coulomb to enable movement around the circuit. The symbol for volt is the capital letter V.
Thus
Joule’s coulombs=volts.
It follows that:
joules=volts × coulombs = volts × ampers × seconds.
A 12-volt (12-V) battery is able to give 12 joules (12 J) of energy to each coulomb to enable movement around an electrical circuit.
The symbol for e.m.f. is the capital letter E.
Example:
Calculate the energy supplied by a 12v battery when a current of 4 A flows for 10 minutes.
Energy supplied = Volts x amperes x seconds
= 12 x 4 x (10 x 60) Joules
= 28,800 J
Cell Potential
(1) “The difference in potentials of the two half – cells of a cell known as electromotive force (emf) of the cell or cell potential.”
The potential difference of the two half – cells of a cell arise because of the flow of electrons from anode to cathode and flow of current from cathode to anode.

(2) The emf of the cell or cell potential can be calculated from the values of electrode potentials of two half – cells constituting the cell. The following three methods are in use
(i) When oxidation potential of anode and reduction potential of cathode are taken into consideration
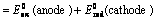
(ii) When reduction potentials of both electrodes are taken into consideration
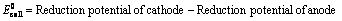
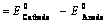
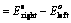
(iii) When oxidation potentials of both electrodes are taken into consideration
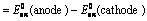
Nernst Equation
Any change in the Gibbs free energy G directly correspond to changes in free energy for processes at constant temperature and pressure, change is the maximum non-expansion work obtainable under these conditions in a closed system; ΔG is negative for spontaneous process, positive for nonspontaneous process, and zero for processes at equilibrium.
It takes into consideration the values of the standard electrode potentials, temperature, activity and the reaction quotient for the calculation of cell potential. For any cell reaction, that occurs Gibbs free energy can be related to standard electrode potential as:
ΔG =-nFE
Where, n = number of electrons transferred in the reaction, ΔG= Gibbs free energy, E= cell potential F = Faraday’s constant (96,500 C/mol) and. Under standard conditions, the above equation can be written as,
ΔGo =-nFEo
According to the theory of thermodynamics, Gibbs free energy under general conditions can be related to Gibbs free energy under standard condition and the reaction quotient as:
ΔG=ΔGo + RT lnQ
Where, Q= reaction quotient, R= universal gas constant and T= temperature in Kelvin. Incorporating the value of ΔGo and ΔG, from the first two equations, we get the equation:
-nFE = -nFE0 + RT lnQ
E = E0 – (RT/nF) lnQ
By conversion of Natural log to log10, the above equation is called as the Nernst equation. Here, it shows the relation of the reaction quotient and the cell potential. Special cases of Nernst equation:
E = Eo − (2.303RT/nF) log10Q
At standard temperature, T= 298K:
E = Eo − (0.0592V/n) log10Q
At standard temperature T = 298 K, the 2.303RTF, term equals 0.0592 V.
Under Equilibrium Condition
As the redox reaction in the cell progresses, the concentration of reactants decreases while the concentration of products increases. This process goes on until equilibrium is achieved. At equilibrium, ΔG = 0. Hence, cell potential, E = 0. Thus, the Nernst equation can be modified to:
E0 – (2.303RT/nF) log10Keq = 0
E0 = (2.303RT/nF) log10Keq
Where, Keq = equilibrium constant and F= faradays constant. Therefore, the above equation gives us a relation between standard electrode potential of the cell where the reaction takes place and the equilibrium constant.
Applications of Nernst Equation
One of the major applications of Nernst equation is in determining ion concentration
Corrosion is the disintegration of a metal due to the chemical reactions between the metal and the surrounding environment. Both the types of metal and the environmental conditions, particularly gasses that come in contact with the metal, determine the form and rate of the metal.
All metals can corrode. Some metals, like pure iron, deteriorate very fast. Stainless steel, and, metals that combines with iron and other alloys, is slower to corrode and is therefore used more efficiently.
All small group of metals, are called the Noble Metals, and show much less reaction than others. As a result, they deteriorate rarely. They are, in fact, the only metals that are found in nature in their purest form. The Noble Metals, not unexpectedly, are often very valuable. They include gold, rhodium, silver, palladium, and platinum.
Types of Corrosion
There are different reasons for metal corrosion. Some can be prevented from corrosion by adding alloys to a pure metal. Others can be avoided by carefully combining metals or management of the metal's environment. Some of the most common types of corrosion are described below.
- Pitting -- it involves the creation of small holes in the surface of a metal.
- Crevice corrosion – it is the corrosion that occurs in stagnant locations such as those found under gaskets.
- Filiform corrosion –they are the corrosions that occurs when water gets under a coating such as paint.
Corrosion Prevention
An effective prevention system begins in the design stage with a proper understanding of the environmental conditions and metal properties. Engineers who work with metallurgical experts should select the proper metal or alloy for every situation. They should also be aware of possible chemical interactions between metals used for surfaces, fittings, and fastenings.
H.G.T Ellingham proposed the Ellingham diagram to predict the spontaneity of reduction of metal oxides. Ellingham diagram was basically a curve which related the Gibbs energy value with temperature.
Ellingham diagram for reduction of oxides
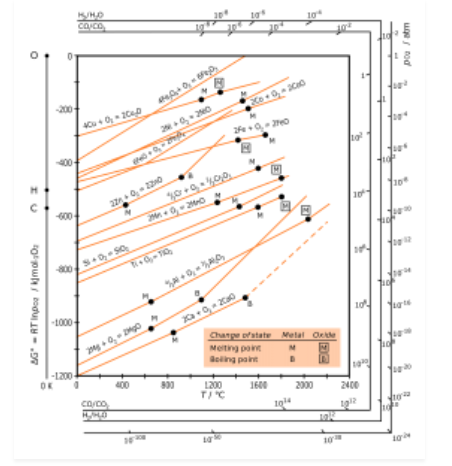
Fig. 2: Ellingham diagram for reduction of oxides
Ellingham diagram is a plot between ΔfGo and T for the formation of oxides of metals. A general reaction expressing oxidation is given by
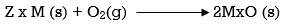
It is evident from the reaction that the gaseous amount of reactant decreased from left to right, as the product formed is solid metal therefore molecular randomness all decreases from left to right, thus ΔS is negative. Hence for most reactions the formation of MxO(s) curve is positive.
Except for the process, where change of phase takes place, each plot is a straight line. The temperature at which change of phase takes place is indicated by a positive increase in the slope. For E.g. The melting is indicated by an abrupt change in the curve in Zn, ZnO plot.
The metal oxide (MxO) is stable at a point in a curve, below ΔG is negative.
The feasibility of reduction of the oxides of the upper line by the element is represented by the lower line and is determined by the difference in the two ΔrGº after the point of intersection in Ellingham diagram.
The solubility product constant (Ksp) is the equilibrium constant for a solid that dissolves in an aqueous solution. All of the rules for determining equilibrium constants continue to apply. An equilibrium constant is the ratio of the concentration of the products of a reaction divided by the concentration of the reactants once the reaction has reached equilibrium. Consider this reaction:
AgCl(s)→Ag+(aq)+Cl−(aq)AgCl(s)→Ag+(aq)+Cl−(aq)
The equilibrium expression for the reaction is:
Keq=[Ag+] [Cl−][AgCl]Keq=[Ag+] [Cl−][AgCl]
Because the AgCl is a solid, its concentration before and after the reaction is the same. The equilibrium equation can therefore be rearranged as:
Ksp=[Ag+] [Cl−]Ksp=[Ag+] [Cl−]
For substances in which the ions are not in a 1:1 ratio, the stoichiometric coefficients of the reaction become the exponents for the ions in the solubility-product expression:
PbCl2⇌Pb2++2Cl− gives Ksp=[Pb2+] [Cl−]2PbCl2⇌Pb2++2Cl− gives Ksp=[Pb2+] [Cl−]
Ba3(PO4)2⇌3Ba2++2PO42− gives Ksp=[Ba2+]3[PO42−]2Ba3(PO4)2⇌3Ba2++2PO42− gives Ksp=[Ba2+]3[PO42−]2
Calculating the Solubility Product
At a certain temperature, the solubility of Fe (OH)2 in water is 7.7 x 10-6 mol/L (M).
Its Ksp can be calculated based on the equilibrium equation:
Fe (OH)2⇌Fe2++2OH−Fe (OH)2⇌Fe2++2OH−
Therefore, the solubility product expression is:
Ksp=[Fe2+] [OH−]2Ksp=[Fe2+] [OH−]2
One mole of dissolved Fe (OH)2 produces one mole of Fe2+ and two moles of OH–, so therefore:
Ksp=x(2x)2Ksp=x(2x)2 where x = 7.7 x 10-6
[Fe2+] =7.7×10−6[Fe2+] =7.7×10−6
[OH−]=2×7.7×10−6=1.54×10−5[OH−]=2×7.7×10−6=1.54×10−5
Ksp=[Fe2+] [OH−]2Ksp=[Fe2+] [OH−]2
Ksp=7.7×10−6× (1.54×10−5)2Ksp=7.7×10−6× (1.54×10−5)2
Ksp=1.83×10−15Ksp=1.83×10−15
Uses of Solubility Product
Solubility products are useful in predicting whether a precipitate will form under specified conditions. It is also helpful in choosing conditions under which two chemical substances in solution can be separated by fractional precipitation. The solubility product of a number of substances have been experimentally determined and can be used to predict solubility at a specified temperature.
Water Chemistry
Water is a chemical compound consisting of two hydrogen atoms and one Oxygen atom. The name water typically refers to the liquid state of the compound. The solid phase is called as ice and the gas phase is called as steam. Under specific conditions, water also forms a supercritical fluid. Water is the main compound found in living organisms. Approximately 62 percent of the human: body contains water. The word "water" comes from the Old English word water or from the Proto-Germanic water or German Wasser. All of which mean "water" or "wet." The boiling point of water is 99.98 degrees C (211.96 degrees F; 373.13 K).
Water is amphoteric. Which means, it can act as both an acid and as a base.
Hard and soft water:
Hard water: is water that contains a required quantity of dissolved minerals (like calcium and magnesium) As rainwater falls, it is naturally soft. However, as water flows through the ground and into waterways, it picks up minerals like chalk, lime and mostly calcium and magnesium and becomes hard water. Since hard water contains essential minerals, it is essentially used as drinking water. Not only because of the health benefits, but also the flavour. Water that does not produce lather with soap readily is called hard water. Water hardness is usually measured as calcium hardness in milligrams per litre (mg/l) OR parts per million (ppm) OR in grains per gallon (GPG).
For e.g.: sea water, river water, spring water, lake water and well water.
Soft water: Water that shows the absence of dissolved salts of such metals as magnesium, iron, or calcium, which are known to form insoluble deposits such as appear as scale in boilers or soap curds in bathtubs and laundry equipment, soft water is neither healthy nor desirable to drink. water that readily produces lather with soap is called soft water.
For e.g.: Rain water, distilled water, demineralised water.
There are three types of water quality parameters physical, biological and chemical.
The Physical parameters of water quality include:
Turbidity:
is the cloudiness present in water. It is a measure of the ability of light to pass through water. It is caused by suspended materials such as silt, clay, plankton, organic material, and other particulate materials present in water.
Turbidity in drinking water is aesthetically unacceptable, which makes the water look unappetizing. The impact of turbidity can be summarized in the following points:
It can increase the cost of water treatment for various uses.
The particulates can shelter harmful microorganisms and thereby protect them from the disinfection process.
Suspended materials can clog or damage fish gills, decreasing its resistance to diseases, reducing its growth rates, affecting egg and larval maturing, and affecting the efficiency of fish catching method.
Suspended particles provide a medium of adsorption for heavy metals such as cadium, lead, chromium, mercury, and many hazardous organic pollutants such as polychlorinated biphenyls (PCBs), polycyclic aromatic hydrocarbons (PAHs), and many types pesticides.
The amount of available food is reduced because higher turbidity raises water. Consequently, the concentration of the dissolved oxygen (DO) can be decreased since warm water carries less dissolved oxygen compared to cold water.
Turbidity can be measured by an instrument called Nephelometric turbidimeter, the instrument expresses turbidity in terms of NTU or TU. A TU is equivalent to 1 mg/L of silica in suspension.
Turbidity that is more than 5 NTU can be visible to the average person while turbidity in muddy water, it exceeds 100 NTU. Groundwater normally has very low turbidity because of the natural filtration that occurs as the water penetrates through the soil.
Temperature
Palatability, viscosity, solubility, odours, and chemical reactions are influenced by temperature. Thereby, the chlorination and sedimentation, processes and biological oxygen demand (BOD) are dependent on temperature.
Colour
Materials decayed from organic matter, such as any, vegetation and inorganic matter namely stones, soil, and rocks impart colour to water, which is objectionable for aesthetic reasons, not for health reasons.
Colour is measured by comparing the water sample with standard colour solutions or coloured glass disks. One unit of colour is equivalent to the colour produced by a 1 mg/L solution of platinum (potassium chloroplatinate (K2PtCl6)).
The colour of a water sample can be reported as follows:
Apparent colour is the entire water sample colour and consists of both dissolved and suspended components colour.
True colour of the water sample is measured after filtering the water sample to remove all suspended particles.
Colour is graded on scale of 0 (clear) to 70 colour units. Pure water is colourless, which is equivalent to 0 colour units.
Taste and odour
Taste and odour in water can be caused by foreign materials such as organic materials, inorganic compounds, or dissolved gasses. These materials arise from natural, domestic, or agricultural sources.
The numerical value of odour or taste is determined quantitatively by measuring a volume of sample A and diluting it with a volume of sample B of an odour-free distilled water so that the odour of the resulting mixture is just detectable at a total mixture volume of 200 ml. The unit of odour or taste is expressed in terms of a threshold number as follows:
TON or TTN = (A + B)/A
where TON is the threshold odour number and TTN is the threshold taste number.
Solids
Solids exist in water in either forms, a solution or in suspension. The two types of solids can be identified by using a glass fibre filter through which water sample passes through. By definition, the suspended solids are retained on the top of the filter and the dissolved solids pass through the filter with the water.
when the filtered portion of the water sample is placed in a small dish and then evaporated, the solids form as a residue. This material is called total dissolved solids or TDS.
Total solid (TS) = Total dissolved solid (TDS) + Total suspended solid (TSS)
Water can be classified by the amount of TDS per litre as follows:
The residue of TSS and TDS after heating to dryness for a specified period of time and at a specific temperature is defined as fixed solids. Volatile solids are those solids lost on ignition (heating to 550°C).
These necessary measures help the operators of the wastewater treatment plant because they roughly approximate the amount of organic matter that exists in the total solids of Industrial waste, activated sludge, and waste water. Fig. 3 describes the interrelationship of solids found in water. They are calculated as follows:
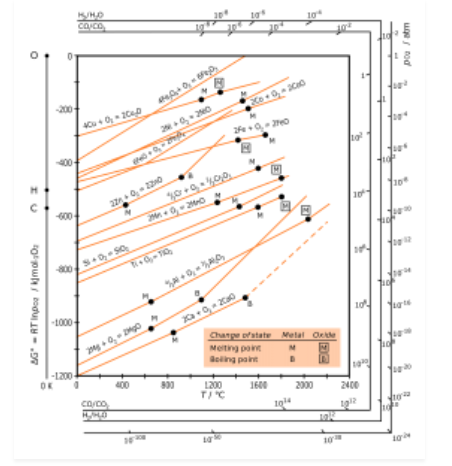
Figure 3: Interrelationship of solids found in water.
Total solids(mg/L) = [(TSA – TSB)] × 1000/sample(mL)
One of the prime factors that establishes the quality of a water supply is its degree of hardness. Hardness is defined as amount of calcium and magnesium ion content present in water. Since most experiments do not distinguish between Ca2+ and Mg2+, and most hardness is caused by carbonate mineral deposits, hardness is usually derived as parts per million (ppm) of calcium carbonate (by weight). if water is supplied with a hardness of 100 ppm that consists an equivalent of 100 g of CaCO3 in 1 million g of water or 0.1 g in 1 L of water.
Water hardness can be readily determined by titration method with the chelating agent, EDTA (ethylenediaminetetraacetic acid) (Greek χηλή, chelè, meaning claw). This reagent is a weak acid that can lose four H (in bold) on complete neutralization;
The four acid oxygen sites and the two nitrogen atoms have unshared pair of electrons, which can form bonds with a metal ion forming a complex ion or coordination compound. The complex that is formed is quite stable, and the conditions of its formation can easily be controlled so that it is ready for selection for a particular metal ion. When a titration to determine the concentration of a metal ion is carried out, the added EDTA quantitatively combines with the cation to form the complex. The endpoint is reached when essentially all of the cation has reacted. In this analysis a solution of EDTA will be standardize by titration against a standard solution made from calcium carbonate, CaCO3. The EDTA solution can then be used to determine the hardness of an unknown water sample. Since both EDTA and Ca2+ are colourless, therefore a special indicator is used to detect the end point of the titration. The indicator mostly used is called Eriochrome Black T, which forms a very stable wine-red complex, MgIn–, with the magnesium ion. A small amount of this complex will be present in the solution during the titration. As EDTA is added, the complex free Ca2+ and Mg2+ ions, leaving the MgIn– complex alone until essentially all of the calcium and magnesium are converted to chelates. At this point EDTA concentration will increase marginally to displace Mg2+ from the complex indicator; the indicator reverts to its uncombined form, which is sky blue, thus establishing the end point of the titration. The titration is carried out at a pH of 10, in a NH3/NH4 + buffer, the equations for the reactions which occur during the titration are:


 Titration reaction: HY3–(aq) + Ca2+(aq) CaY2–(aq) + H+ (aq) (also for Mg2+) End point reaction: HY3–(aq) + MgIn– (aq) MgY2–(aq) + HIn2–(aq)
Titration reaction: HY3–(aq) + Ca2+(aq) CaY2–(aq) + H+ (aq) (also for Mg2+) End point reaction: HY3–(aq) + MgIn– (aq) MgY2–(aq) + HIn2–(aq)
As the indicator requires a trace of Mg2+ to operate properly, a little magnesium ion will be added to each solution. The effect of the added Mg2+ can be subtracted by titrating a blank.
A simple way to calculate the hardness in water.
A sample of hard water contains 1mg cacl2 and 1 mg mgcl2 per
litre. calculate the hardness of water in terms of caco3 present in per 106 parts of water.
Hardness of water in terms of Caco3= (molar mass of hardness causing substance*molecular
weight of Caco3)/ (molecular weight of hardness causing substance.
Thus, cacl2 hardness = 1mg*100/111 = 0.900ppm for Mgcl2
Hardness = 1mg*100/95=1.052ppm
Total hardness of water in terms of Caco3 = 0.90+1.052= 1.952ppm
Alkalinity
Alkalinity is the measure of all bases present in the water and assumed as the buffering capacity of water, or its ability to resist change in pH. The most common and important base is carbonate. Total alkalinity is expressed as parts per million (ppm) of calcium carbonate (CaCO3) or milligrams per litre (mg/L). Alkalinity can be referred to as "carbonate hardness" or "KH," which is usually measured in degrees (dKH) than in mg/L or ppm. One dKH is equal to 17.9 mg/L or 17.9 ppm.
Waters that have moderate to high levels (50 mg/L or greater) of total alkalinity and total hardness usually have a neutral to slightly basic pH. At this stage the pH is more stable and does not change greatly throughout the day because the presence of carbonates and bicarbonates neutralize, or "buffer," the carbon dioxide and other acids in the water.
Alkalinity is a measure of the capacity of water to neutralize hydrogen ions or acids. Alkalinity is otherwise to as "Carbonate hardness". Alkalinity acts as a buffer when any changes are made to the water's pH value. The Alkalinity in the water will help keep the pH of water stabilized. The drinking water and all water should be a pH of 7 which neutral.
Procedure of Alkalinity Test
The titration tube up is filled up to the 5ml line with water.
Removal of hardness of water by lime soda
Surface water hardly exceed hardness level above 200 mg/1 and softening is not at all required in most of the cases, unless the water is being polluted by some effluent sources. In case of groundwater, hardness level of more than 1000 mg/1 are quite common. Since, soft water is corrosive, therefore public water supply is usually not softened below 30 to 50 mg/1. The most accepted and commonly used water softening methods are cat ion exchange and precipitation method. In order to obtain maximum profit, the factors to be considered are a good choice of a softening process, quality of the raw water, the cost of softening chemicals and the cost of disposing of waste streams.
Precipitation methods
The principle that follows the precipitation method is to bind calcium cations Ca and magnesium cations Mg, with ions of CO3 and OH. The precipitate CaC03 and Mg (OH)2 formed are removed from the water. slake lime Ca (OH)2, Quick lime CaO, soda ash NaC03 and sodium hydroxide (caustic soda) NaOH, are reagents that are commonly used in water softening. Depending upon the quality of initial water, the following main precipitation methods are determined. a) Lime softening b) Lime - Soda softening c) Sodium Hydroxide softening Lime affects the carbonate hardness (alkalinity) and therefore can be used in order to decrease the carbonate hardness present in the initial water. This method however does not result in deep softening. Magnesium is removed from water if there is excess of OH” present. Water dissolved carbon dioxide is removed, total solids in the treated water diminishes and the total hardness in the lime treated water also reduces. But the pH increases to 10 or beyond. When lime is added to the hard water following reactions occurs, In the above reactions,
Lime Addition:
Hardness Lime Precipitate
CO2 + Ca (OH)2 -- > CaCO3 + H2O Ca (HCO3)2 + Ca (OH)2 -- > 2CaCO3 + 2H2O Mg (HCO3)2 + Ca (OH)2 -- > CaCO3 + MgCO3 + 2H2O MgCO3 + Ca (OH)2 -- > CaCO3 + Mg (OH)2 CO2 the insoluble products do not contribute to the hardness, but it reacts with the lime, and thereby uses up some lime before the lime can start removing the hardness in water. Lime - Soda softening method is commonly practiced in most of the public water supply. (Belan1984) The method is universal as water of almost any composition is treated with lime and soda. In this treatment, two reagents are used namely lime and soda ash. Lime as earlier discussed, decreases the carbonate hardness, (Mg2+) and removes C02 from the water.
Soda therefore reduces the non - carbonate hardness, mainly due to Ca2+, that shows after reaction with lime and the reaction occurs after the addition of soda ash is as follows.
Lime and Soda ash Addition: - Lime Precipitate MgSO4 + Ca (OH)2 -- > Mg (OH)2 + CaSO4 Soda ash Precipitate CaSO4 + Na2CO3 -- > CaCO3 + Na2SO4 2.
Ion exchange method including Zeolite method
Ion Exchange Process
Ion exchange process to soften water, using cations or anions. This is done by exchanging cations or anions with the calcium and magnesium ions in hard water. This process involves a reversible chemical reaction. However, we can use this technique only in dilute solutions. The equipment that we use for this purpose is ion exchangers.
Types
There are two types:
The materials used in cation exchangers include either weak acids or strong acids. Strong acid cation exchangers mainly contain sulfate functional groups. Weak acid cation exchangers mainly contain carboxyl groups. The materials that are used in anion exchangers include either weak bases or strong bases. Moreover, there are several categories of ion exchange process used for water softening, Dealkalization and demineralization. The ions that are part of the exchange process (the ions that exchange with the calcium and magnesium cations in hard water) include sodium ions, hydrogen cations, chloride anions and hydroxyl anions.
Zeolite process
Zeolite process is a process of softening hard water thru ion exchange technique using a chemical compound zeolite. It possesses a chemical compound that has hydrated sodium aluminosilicate. Thus, the name of the process is called as zeolite process. Zeolite compound can exchange its sodium cations reversibly with calcium and magnesium ions in the water softening process.
There are two types of zeolites used in this process they include natural and synthetic zeolite. The natural form is found to be porous and synthetic form is a non-porous zeolite. however synthetic form possesses a high exchange capacity per unit weight than the natural form.
Figure: Cylinder Containing the Zeolite Bed
Process
In the water softening process, hard water is passed through a bed of zeolite (inside a cylinder) at a specified rate. Then the cations that cause the water-hardening will remain on the zeolite bed because these cations exchange with the sodium cations of zeolite. Therefore, the water coming out of this cylinder contains sodium cations rather than calcium and magnesium cations.
After some time, the zeolite bed gets exhausted, the water flow is stopped and treat the bed is treated with concentrated brine solution (10%) in order to regenerate the zeolite. The bed is treated with a brine solution, as it washes away all the calcium and magnesium ions, by exchanging them with sodium ions in a brine solution. hence, this treatment regenerates the zeolite.
References: