Unit - 6
Stereochemistry
A three-dimensional (3-D) structure of organic molecules can be represented on paper by using certain conventions. A solid () wedge is used to indicate a bond projecting out of the plane of the paper, towards the observer. A dashed () wedge is used to indicate a bond projecting out of the plane of the paper, away from the observer.
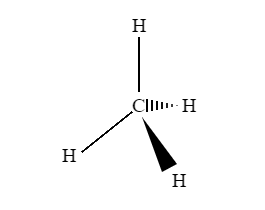
Fig 1: An example of a 3D representation of organic molecules
Isomerism
Two or more compounds having the same molecular formula but different physical and chemical properties are called isomers and the phenomenon is known as isomerism. There are two types of isomerism
Structural isomerism
Those compounds that have the same molecular formula but have different structures are called as structural isomers. Structural isomerism is further divided into six subcategories.
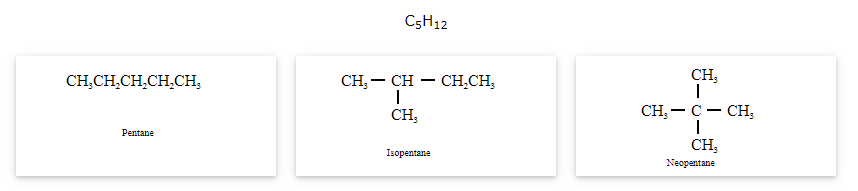
The compounds that have same molecular formula but differ in carbon skeletons are called as chain isomers and the phenomenon is known as Chain isomerism.
Position isomerism
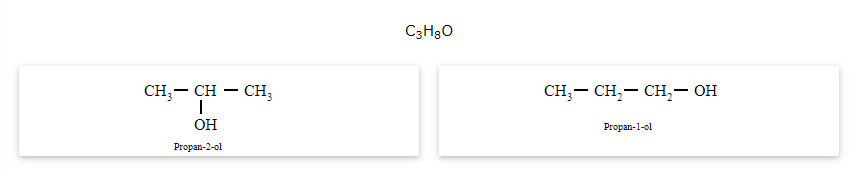
The compounds that have same molecular formula but are different with respect to the position of the multiple bond (double or triple bond) or functional group are called as position isomers and the phenomenon is known as position isomerism.
Functional isomerism
The compounds that have same molecular formula but differ in their functional groups are called functional isomers and the phenomenon is called functional isomerism.
General formula for ether is R-O-R' where R and R' are different (or similar) carbon chains.
Metamerism
The compounds that have same formula but differ in alkyl chains on either side of the functional group are called as metamers and the phenomenon is known as metamerism.
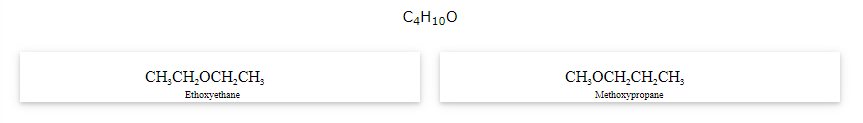
Notice that the above compounds are also called as position isomers.
Tautomerism
Tautomerism occurs due to the 1,3-migration of a hydrogen atom within the same molecule. The best example of tautomerism is keto-enol tautomerism, where one form contains the keto group (>C=O) and the other form contains the enol (en + ol) group.
Acetaldehyde and ethanol
You may note that in keto-enol tautomerism, enol form is usually negligible.
Ring-chain isomerism
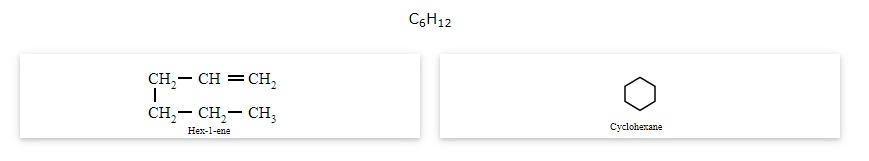
The compounds that have the same molecular formula but one possesses an open chain while the others possess cyclic structures are called ring-chain isomers and the phenomenon is known as ring-chain isomerism.
Stereoisomerism
The compounds that have the same formula but are different in spatial arrangement of one atoms or group of atoms in space are known as stereoisomers and the phenomenon is called stereoisomerism. Stereoisomerism has the following two sub categories:
Conformational isomers are those isomers where the relative positions of few atoms differ in the molecule in the three-dimensional space because of the rotation about sigma bonds.
Configurational isomerism is caused due to remaking and breaking of covalent bonds. They are further divided into:
Configuration
In the context of stereochemistry, the term is restricted to the arrangements of atoms of a molecular entity in space that distinguishes stereoisomers, the isomerism between which is not due to confirmation differences.
It is the spatial arrangement of the atoms of a chiral
molecular entity (or group) and its stereochemical description e.g., R or S.
The configuration of any stereo genic (asymmetric) centre with respect to any other stereo genic centre contained within the same molecular entity. Unlike
Absolute configuration, relative configuration is a reflection-invariant. Relative configuration, distinguishing diastereoisomers, may be denoted by the configurational descriptors R*R*, R*R* (or l) and R*R*, S*S* (or u) which means, respectively, that the two centres have similar or opposite configurations. For molecules that have more than two
asymmetric centres the prefix rel- may be used in front of the name of one
enantiomer where R and S have been used. If any centres have known
absolute configuration then only R*R* and S*S* can be used for the relative configuration.
The symmetry of a molecule describes how its different parts relate to one another geometrically. symmetry plays an important role in many areas of chemistry, with effects on:
Symmetry Element: It is an axis, point or plane about which a symmetry operation is performed.
Point Group: It is a symbol that identifies all the symmetry elements present in a molecule.
Symmetry Elements and Operations
Element | Operation | Symbol | Notes |
None | identity | E |
|
proper | rotate by | Cn |
|
mirror plane | Reflection | Σ |
|
inversion | Inversion | I |
|
improper | rotate by 360º/n, then reflect ^ to axis | Sn |
|
Effects on Molecular Properties
For a molecule to be chiral, both the functions Inversion (i) and Reflection(σ) must be absent. * Those Molecules that belong to groups Cn (including E) and Dn are chiral. * This is a small simplification. The most accurate requirement for chirality is the lack of any Sn element, as the appearance of these is difficult to figure out, however there are a series of increasingly precise shortcuts used to Spotting a Chiral Compound.
There are two types of STEREOISOMERS, enantiomers and diastereomers.
Enantiomers contain chiral centers that are non-superimposable & mirror images. They only come in pairs!
Diastereomers contain chiral centers which are non-superimposable but are NOT mirror images. There can be more than 2 depending on the number of stereo centers.
An easy way to remember enantiomers from diastereomers is to analyse and study the picture given below. In cases where there are 2 chiral centers, 4 stereoisomers are possible formed. Only the exact opposites (diagonal arrows) are enantiomers and they therefore have a mirror image that is not superimposable. The molecules with only one stereocenter that differs (parallel arrows) are diastereomers.
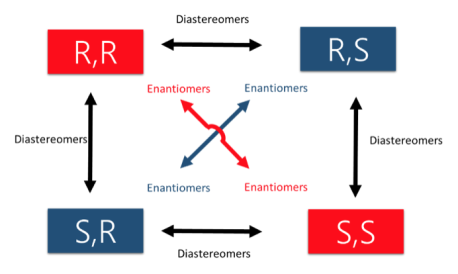
Fig.2: The picture shows two chiral centres, resulting in the formation of 4 stereoisomers. The diagonal arrows are enantiomers, their images are not superimposable though they are mirror images formed, the parallel arrows are diastereomers with only one stereocentre.
A biological example of this property can be viewed in, saccharide (or sugar) and below are the enantiomers and diastereomers of threose molecule.
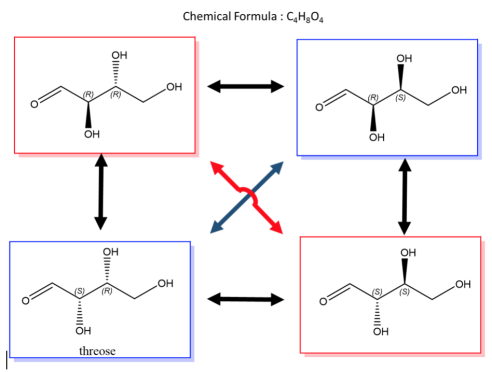
Fig.3: The picture shows a molecule of 5-DHT,an active form of Testosterone and has 7 stereocentres, Using the formula, 128 possible combinations are formed, only one is an enantiomer the rest are stereoisomers.
While enantiomers are found to only come in pairs, many diastereomers can exist without for a given molecule. Let’s take, 5-DHT for example, this compound is metabolically active form of testosterone and has 7 stereocenters, using the 2N rule for determining the number of stereoisomers, we get 128 possible combinations from the given formula. But only one of them is the enantiomer of 5DHT! The rest are diastereomers.
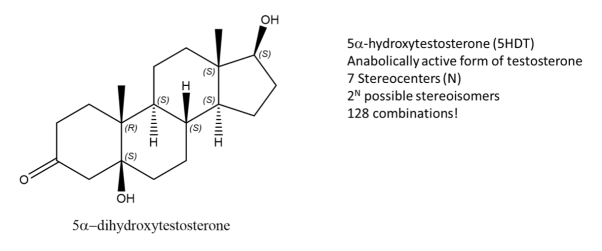
Fig.4: Structure of Testosterone, using the 2n rule, 128 combinations are formed.
The optical activity of a substance, is the tendency of a substance to rotate along the plane of polarization of a beam of light that is passed through it. (In a plane-polarized light, the vibrations of the electric field are confined to a single plane.) The intensity of optical activity of a substance is expressed in terms of a quantity, called specific rotation. The specific rotation is defined by an equation that is related to the angle through which the plane is rotated, the length of the light path through the sample, and the density of the sample (or its concentration if it is present in a solution). As the specific rotation depends upon the temperature and upon the wavelength of the light, these quantities also must be specified. The rotation is assigned a positive value if it is clockwise with respect to an observer facing the light source, negative if counter clockwise. A substance with a positive specific rotation is described as dextrorotatory and denoted by the prefix d or (+); a substance with a negative specific rotation is levorotatory, designated by the prefix l or (-).
Optical activity was first discovered in quartz, crystals in by a French physicist in 1811, Francois Arago. Another French physicist, Jean-Baptiste Biot, found in 1815 that liquid solutions of tartaric acid or of sugar are optically active, as are liquid or vaporous turpentine. however, Louis Pasteur was the first to recognize that optical activity appears from the dissymmetric arrangement of atoms in the crystalline structures or also in individual molecules of certain compounds.
Configuration can be defined as an arrangement of elements or parts in a particular figure, form, or found in any combination. It refers to the spatial arrangement of the atoms of a particular chiral molecular entity (or group) and its stereochemical description.
e.g., R or S, which refer to as Rectus, or Sinister,
respectively. Absolute configurations for a chiral molecule (in pure form) are most
often obtained through X-ray crystallography.
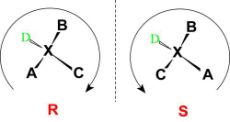
Fig:5: The figure shows the R and S configuration to assign the absolute configuration to a structure.
Conformation Analysis is related to any of the spatial arrangements of the atoms in a molecule these atoms may adopt and freely convert between, especially by rotation about individual single bonds.
It’s a branch of stereochemistry that studies the conformations of molecules and their relations to the chemical and physical properties of substances. The Dutch chemist J. H. Van’t Hoff based on the stereochemical hypothesis he formulated (1874–75) on two principal postulates: (1) the valences of the saturated carbon atom are directed in space toward the vertices of the tetrahedrons, and (2) the atoms or groups of atoms (substituents) in the molecule can freely rotate about single bonds without being broken (unlike double bonds whose rigidity causes the formation of geometric isomers). Subsequently, the tetrahedral model of the carbon atom was confirmed by direct X-X-ray analysis. The supposition regarding the free rotation about single bonds was subjected to review, as it is already established that rotation about single bonds is not entirely “free”; during such rotation, energetically unequal geometric forms—conformations—or rotational isomers are formed, some of which are energetically more favourable than others, will emerge. Most of the atoms present in molecules are present primarily in one or several stable (preferred) conformations. Different conformation of the same substance that are separated by energy barriers, are usually equal to 20.9–62.7 kilojoules per mole (5–15 kilocalories per mole); individual conformations constantly convert into one another. The investigations performed by the British chemist D. Barton on the conformations in the cyclohexane series were of singular importance; Barton also introduced the term “conformational analysis” in (1950).
In the paraffin hydrocarbon series, the necessity of conformational analysis is another evidence in the case of ethane, that have two possible conformations: the eclipsed (or even) and staggered (or odd). These are formed during the rotation of one methyl group with respect to another (Figure 1).
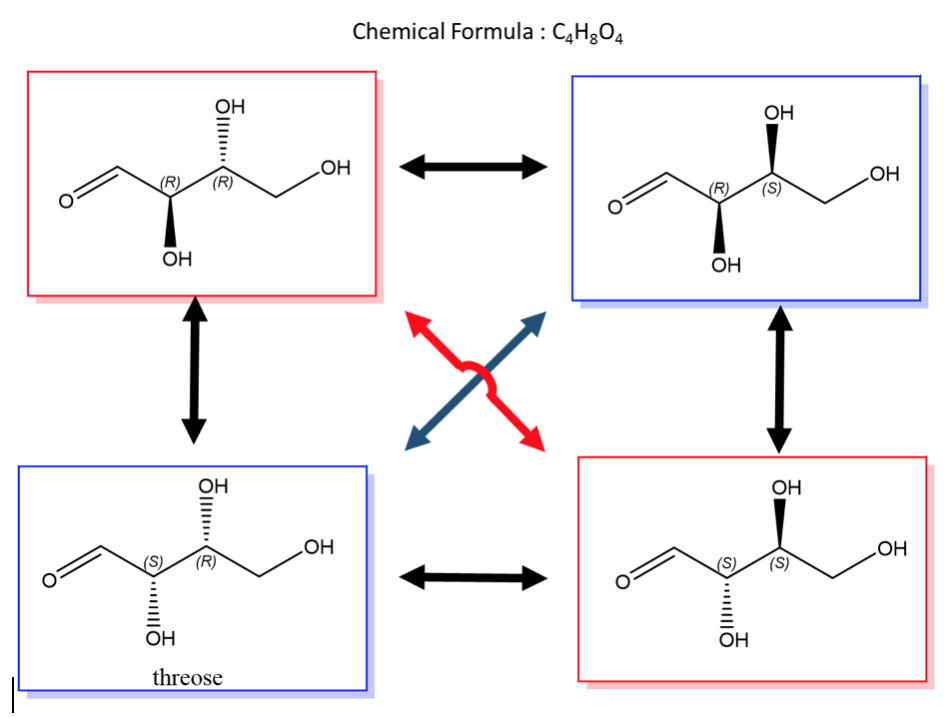
Figure 6. (a) Perspective formulas and (b) Newman’s projection (view of molecule from “above” along the C—C bond) formulas, depicting the even or eclipsed (I) and staggered or odd in (II) conformation of Ethane. ø is the angle between the substituents also known as dihedral angle
Conformational representations have great importance in the chemistry of synthetic and natural macromolecular compounds, as well as in the field of physiologically active substances.
References:
Unit - 6
Stereochemistry
A three-dimensional (3-D) structure of organic molecules can be represented on paper by using certain conventions. A solid () wedge is used to indicate a bond projecting out of the plane of the paper, towards the observer. A dashed () wedge is used to indicate a bond projecting out of the plane of the paper, away from the observer.
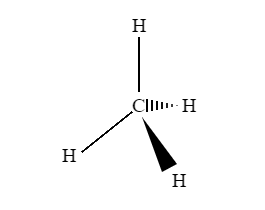
Fig 1: An example of a 3D representation of organic molecules
Isomerism
Two or more compounds having the same molecular formula but different physical and chemical properties are called isomers and the phenomenon is known as isomerism. There are two types of isomerism
Structural isomerism
Those compounds that have the same molecular formula but have different structures are called as structural isomers. Structural isomerism is further divided into six subcategories.
The compounds that have same molecular formula but differ in carbon skeletons are called as chain isomers and the phenomenon is known as Chain isomerism.
Position isomerism
The compounds that have same molecular formula but are different with respect to the position of the multiple bond (double or triple bond) or functional group are called as position isomers and the phenomenon is known as position isomerism.
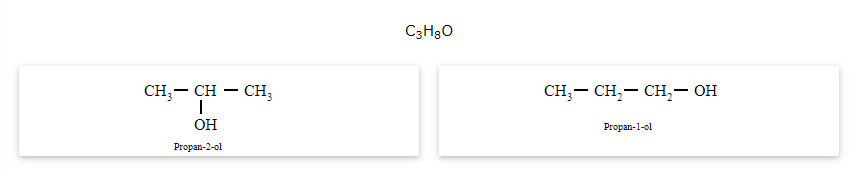Functional isomerism
The compounds that have same molecular formula but differ in their functional groups are called functional isomers and the phenomenon is called functional isomerism.
General formula for ether is R-O-R' where R and R' are different (or similar) carbon chains.
Metamerism
The compounds that have same formula but differ in alkyl chains on either side of the functional group are called as metamers and the phenomenon is known as metamerism.
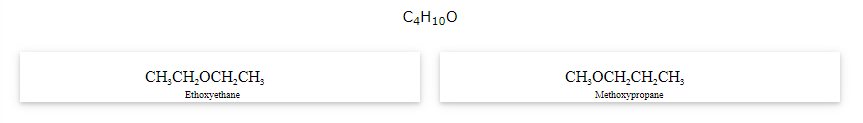
Notice that the above compounds are also called as position isomers.
Tautomerism
Tautomerism occurs due to the 1,3-migration of a hydrogen atom within the same molecule. The best example of tautomerism is keto-enol tautomerism, where one form contains the keto group (>C=O) and the other form contains the enol (en + ol) group.
Acetaldehyde and ethanol
You may note that in keto-enol tautomerism, enol form is usually negligible.
Ring-chain isomerism
The compounds that have the same molecular formula but one possesses an open chain while the others possess cyclic structures are called ring-chain isomers and the phenomenon is known as ring-chain isomerism.
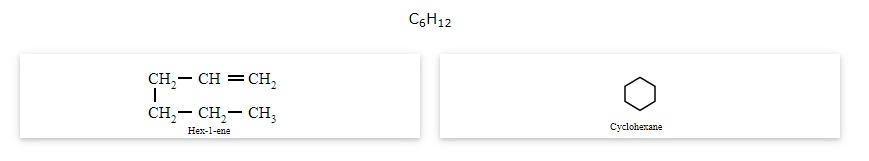
Stereoisomerism
The compounds that have the same formula but are different in spatial arrangement of one atoms or group of atoms in space are known as stereoisomers and the phenomenon is called stereoisomerism. Stereoisomerism has the following two sub categories:
Conformational isomers are those isomers where the relative positions of few atoms differ in the molecule in the three-dimensional space because of the rotation about sigma bonds.
Configurational isomerism is caused due to remaking and breaking of covalent bonds. They are further divided into:
Configuration
In the context of stereochemistry, the term is restricted to the arrangements of atoms of a molecular entity in space that distinguishes stereoisomers, the isomerism between which is not due to confirmation differences.
It is the spatial arrangement of the atoms of a chiral
molecular entity (or group) and its stereochemical description e.g., R or S.
The configuration of any stereo genic (asymmetric) centre with respect to any other stereo genic centre contained within the same molecular entity. Unlike
Absolute configuration, relative configuration is a reflection-invariant. Relative configuration, distinguishing diastereoisomers, may be denoted by the configurational descriptors R*R*, R*R* (or l) and R*R*, S*S* (or u) which means, respectively, that the two centres have similar or opposite configurations. For molecules that have more than two
asymmetric centres the prefix rel- may be used in front of the name of one
enantiomer where R and S have been used. If any centres have known
absolute configuration then only R*R* and S*S* can be used for the relative configuration.
The symmetry of a molecule describes how its different parts relate to one another geometrically. symmetry plays an important role in many areas of chemistry, with effects on:
Symmetry Element: It is an axis, point or plane about which a symmetry operation is performed.
Point Group: It is a symbol that identifies all the symmetry elements present in a molecule.
Symmetry Elements and Operations
Element | Operation | Symbol | Notes |
None | identity | E |
|
proper | rotate by | Cn |
|
mirror plane | Reflection | Σ |
|
inversion | Inversion | I |
|
improper | rotate by 360º/n, then reflect ^ to axis | Sn |
|
Effects on Molecular Properties
For a molecule to be chiral, both the functions Inversion (i) and Reflection(σ) must be absent. * Those Molecules that belong to groups Cn (including E) and Dn are chiral. * This is a small simplification. The most accurate requirement for chirality is the lack of any Sn element, as the appearance of these is difficult to figure out, however there are a series of increasingly precise shortcuts used to Spotting a Chiral Compound.
There are two types of STEREOISOMERS, enantiomers and diastereomers.
Enantiomers contain chiral centers that are non-superimposable & mirror images. They only come in pairs!
Diastereomers contain chiral centers which are non-superimposable but are NOT mirror images. There can be more than 2 depending on the number of stereo centers.
An easy way to remember enantiomers from diastereomers is to analyse and study the picture given below. In cases where there are 2 chiral centers, 4 stereoisomers are possible formed. Only the exact opposites (diagonal arrows) are enantiomers and they therefore have a mirror image that is not superimposable. The molecules with only one stereocenter that differs (parallel arrows) are diastereomers.
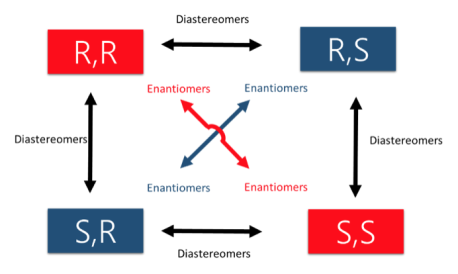
Fig.2: The picture shows two chiral centres, resulting in the formation of 4 stereoisomers. The diagonal arrows are enantiomers, their images are not superimposable though they are mirror images formed, the parallel arrows are diastereomers with only one stereocentre.
A biological example of this property can be viewed in, saccharide (or sugar) and below are the enantiomers and diastereomers of threose molecule.
Fig.3: The picture shows a molecule of 5-DHT,an active form of Testosterone and has 7 stereocentres, Using the formula, 128 possible combinations are formed, only one is an enantiomer the rest are stereoisomers.
While enantiomers are found to only come in pairs, many diastereomers can exist without for a given molecule. Let’s take, 5-DHT for example, this compound is metabolically active form of testosterone and has 7 stereocenters, using the 2N rule for determining the number of stereoisomers, we get 128 possible combinations from the given formula. But only one of them is the enantiomer of 5DHT! The rest are diastereomers.
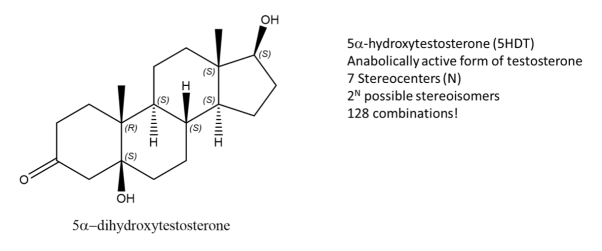
Fig.4: Structure of Testosterone, using the 2n rule, 128 combinations are formed.
The optical activity of a substance, is the tendency of a substance to rotate along the plane of polarization of a beam of light that is passed through it. (In a plane-polarized light, the vibrations of the electric field are confined to a single plane.) The intensity of optical activity of a substance is expressed in terms of a quantity, called specific rotation. The specific rotation is defined by an equation that is related to the angle through which the plane is rotated, the length of the light path through the sample, and the density of the sample (or its concentration if it is present in a solution). As the specific rotation depends upon the temperature and upon the wavelength of the light, these quantities also must be specified. The rotation is assigned a positive value if it is clockwise with respect to an observer facing the light source, negative if counter clockwise. A substance with a positive specific rotation is described as dextrorotatory and denoted by the prefix d or (+); a substance with a negative specific rotation is levorotatory, designated by the prefix l or (-).
Optical activity was first discovered in quartz, crystals in by a French physicist in 1811, Francois Arago. Another French physicist, Jean-Baptiste Biot, found in 1815 that liquid solutions of tartaric acid or of sugar are optically active, as are liquid or vaporous turpentine. however, Louis Pasteur was the first to recognize that optical activity appears from the dissymmetric arrangement of atoms in the crystalline structures or also in individual molecules of certain compounds.
Configuration can be defined as an arrangement of elements or parts in a particular figure, form, or found in any combination. It refers to the spatial arrangement of the atoms of a particular chiral molecular entity (or group) and its stereochemical description.
e.g., R or S, which refer to as Rectus, or Sinister,
respectively. Absolute configurations for a chiral molecule (in pure form) are most
often obtained through X-ray crystallography.
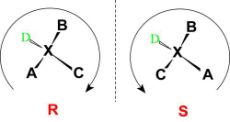
Fig:5: The figure shows the R and S configuration to assign the absolute configuration to a structure.
Conformation Analysis is related to any of the spatial arrangements of the atoms in a molecule these atoms may adopt and freely convert between, especially by rotation about individual single bonds.
It’s a branch of stereochemistry that studies the conformations of molecules and their relations to the chemical and physical properties of substances. The Dutch chemist J. H. Van’t Hoff based on the stereochemical hypothesis he formulated (1874–75) on two principal postulates: (1) the valences of the saturated carbon atom are directed in space toward the vertices of the tetrahedrons, and (2) the atoms or groups of atoms (substituents) in the molecule can freely rotate about single bonds without being broken (unlike double bonds whose rigidity causes the formation of geometric isomers). Subsequently, the tetrahedral model of the carbon atom was confirmed by direct X-X-ray analysis. The supposition regarding the free rotation about single bonds was subjected to review, as it is already established that rotation about single bonds is not entirely “free”; during such rotation, energetically unequal geometric forms—conformations—or rotational isomers are formed, some of which are energetically more favourable than others, will emerge. Most of the atoms present in molecules are present primarily in one or several stable (preferred) conformations. Different conformation of the same substance that are separated by energy barriers, are usually equal to 20.9–62.7 kilojoules per mole (5–15 kilocalories per mole); individual conformations constantly convert into one another. The investigations performed by the British chemist D. Barton on the conformations in the cyclohexane series were of singular importance; Barton also introduced the term “conformational analysis” in (1950).
In the paraffin hydrocarbon series, the necessity of conformational analysis is another evidence in the case of ethane, that have two possible conformations: the eclipsed (or even) and staggered (or odd). These are formed during the rotation of one methyl group with respect to another (Figure 1).
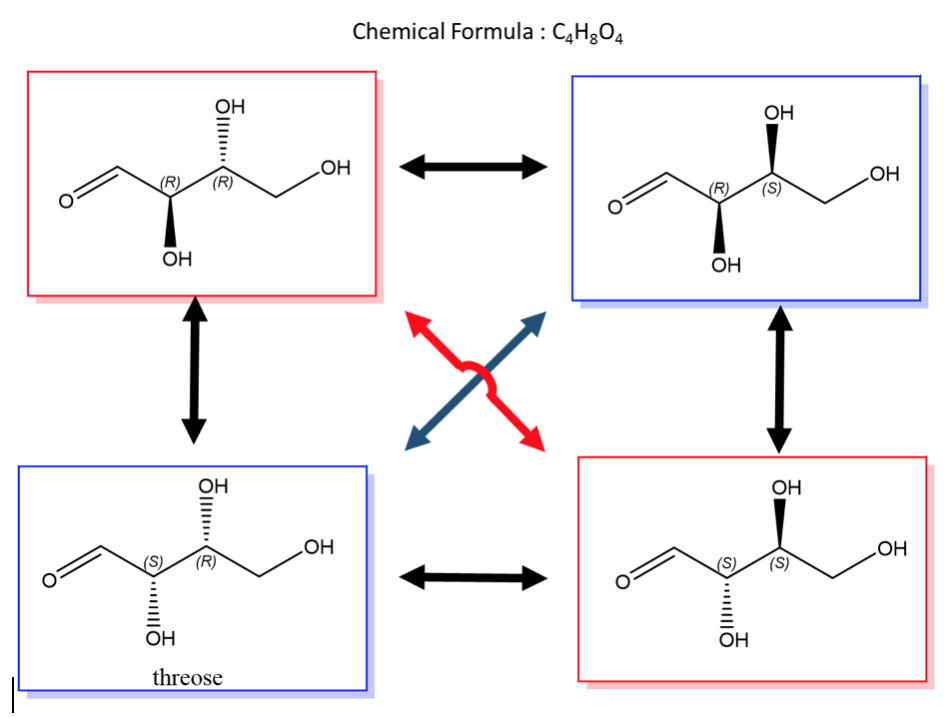
Figure 6. (a) Perspective formulas and (b) Newman’s projection (view of molecule from “above” along the C—C bond) formulas, depicting the even or eclipsed (I) and staggered or odd in (II) conformation of Ethane. ø is the angle between the substituents also known as dihedral angle
Conformational representations have great importance in the chemistry of synthetic and natural macromolecular compounds, as well as in the field of physiologically active substances.
References:
