Unit - 1
Calculus
Definition- The locus of centres of curvature of a given curve is called the evolute of that curve.
The locus of the center of curvature C of a variable point P on a curve is called the evolutes of the curve and the curve itself is called involute of the evolute.
Evolute is nothing but an equation of the curve.
Method to find the evolute-
1. If a curve equation is given and we need to prove left hand side is equal to right hand side (L.H.S = R.H.S), then
First of all, find the curvature C (X, Y), where
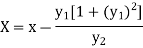
And
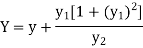
Then take left hand side and put X in place of x and Y in place of y.
2. When the curve is given and we need to find the evolute, then-
Find the curvature first and then re-write as x in terms of X and y in terms of Y and then put in the given curve
Properties of envelope and evolute -
1. The normal at any point of a curve is a tangent to its evolute touching at the corresponding centre of curvature.
2. The evolute is one only but there can be infinite involutes.
3. The difference between the radii of curvature at two points of a curve is equal to the length of the arc of the evolute between the two corresponding points.
Example: Prove that the evolute of parabola 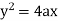 is given by
is given by 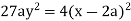
Sol. It is given that-

If (X, Y) are the coordinates of the centre of curvature at any point P (x, y) on the curve y = f(x), then X and Y are given as-
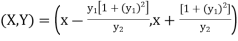 …………….. (1)
…………….. (1)
Now consider the equation of parabola (given)
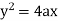
On differentiating w.r.t x-
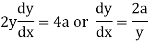
Again differentiating w.r.t. x-
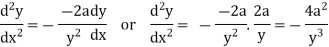
Put these derivatives in (1), we get-
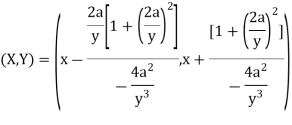
Now consider X,
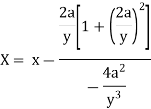
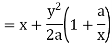
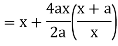
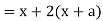
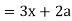
Here we get-
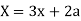
Now consider,
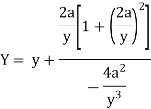
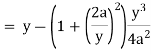
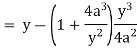
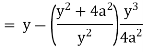
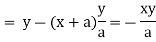
Here we get-

Now
Taking L.H.S of 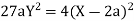
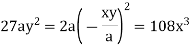
Taking R.H.S of 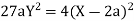
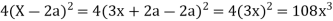
Hence proved.
Example: Find the evolute of the ellipse  .
.
Sol. It is given that-
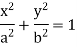
The parametric equations are 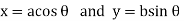
Now,
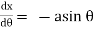 and
and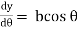
So that-
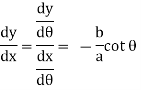
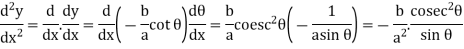
Which gives,
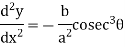
Co-ordinates of centre of curvature are (X, Y)
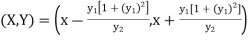 …………….. (1)
…………….. (1)
Consider X,
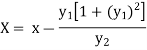
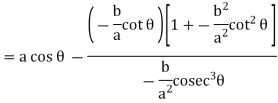
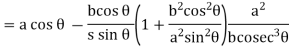
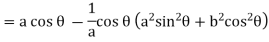
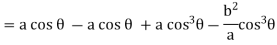
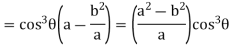
We get-
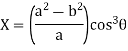
Now consider Y,

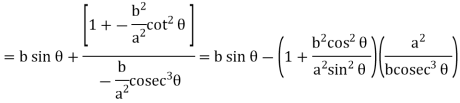
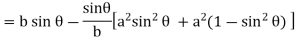
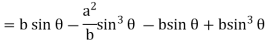
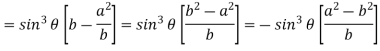
So that we get-
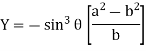
Eliminating 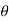 from X and Y, we get,
from X and Y, we get,
 and
and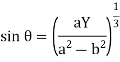
We know that
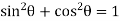
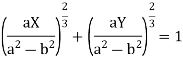
which gives on solving-
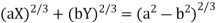
which is the required evolute.
When we apply limits in indefinite integrals are called definite integrals.
If an expression is written as 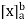 , here ‘b’ is called upper limit and ‘a’ is called lower limit.
, here ‘b’ is called upper limit and ‘a’ is called lower limit.
If f is an increasing or decreasing function on interval [a, b], then
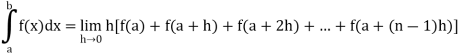
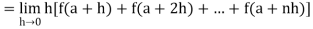
Where 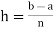
Properties-
1. The definite integral applies only if a<b, but it would be appropriate to include the case a = b and a>b as well, in that case-
If a = b, then
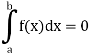
And if a>b, then
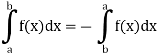
2. Integral of a constant function-
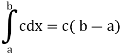
3. Constant multiple property-
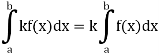
4. Interval union property-
If a < c < b, then
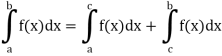
5. Inequality-
If c and d are constants such that 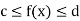 for all x in [a, b], then
for all x in [a, b], then
c (b – a)
Note- if a function f: [a, b] →R is continuous, then the function ‘f’ is always Integrable.
Example-1: Evaluate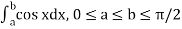 .
.
Sol. Here we notice that f:x→cos x is a decreasing function on [a, b],
Therefore, by the definition of the definite integrals-
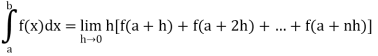
Then
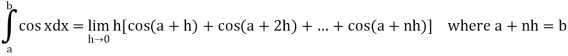
Now,
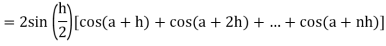
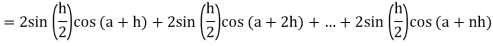
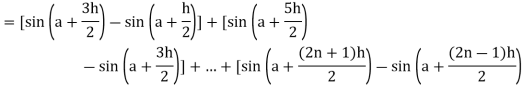
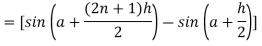
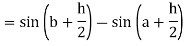
Here 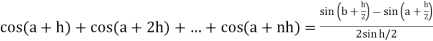
Thus
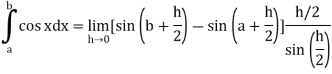
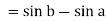
Example-2: Evaluate 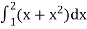
Sol. Here  is an increasing function on [1, 2]
is an increasing function on [1, 2]
So that,
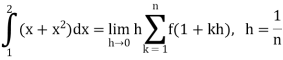
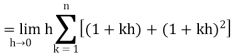
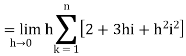
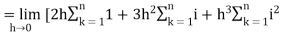 …. (1)
…. (1)
We know that-
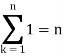

And 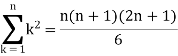
Then equation (1) becomes-
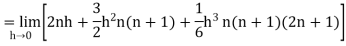
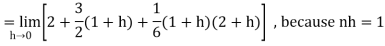
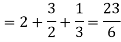
Note- we can find the definite integral directly as-
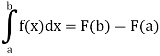
Example-3: Evaluate-
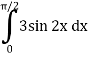 Sol.
Sol. 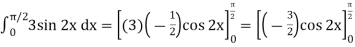
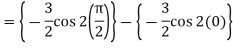
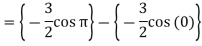
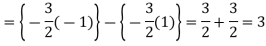
Improper integrals
(1) Let f is function defined on [a, ∞) and it is integrable on [a, t] for all t >a, then
If 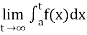 exists, then we define the improper integral of f over [a, ∞) as follows-
exists, then we define the improper integral of f over [a, ∞) as follows-
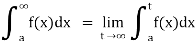
(2) Let f is function defined on (-∞, b] and it is integrable on [t, b] for all t >b, then
If 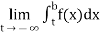 exists, then we define the improper integral of f over (-∞, b] as follows-
exists, then we define the improper integral of f over (-∞, b] as follows-
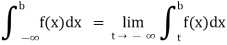
(3) Let f is function defined on (-∞, ∞] and it is integrable on [a, b] for every closed and bounded interval [a, b] which is the subset of R., then
If 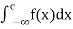 and
and 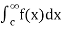 exist for some c belongs to R, then we define the improper integral of f over (-∞, ∞) as follows-
exist for some c belongs to R, then we define the improper integral of f over (-∞, ∞) as follows-
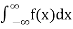 =
= 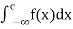 +
+ 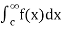
(4) Let f is function defined on (a, ∞) and 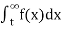 exists for all t>a, then
exists for all t>a, then
If  exists, then we define the improper integral of f over (a ∞) as follows-
exists, then we define the improper integral of f over (a ∞) as follows-
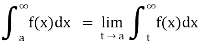
(5) Let f is function defined on (-∞, b) and 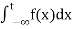 exists for all t<b, then
exists for all t<b, then
If 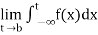 exists, then we define the improper integral of f over (-∞, b) as follows-
exists, then we define the improper integral of f over (-∞, b) as follows-
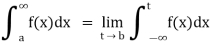
Improper integrals over finite intervals-
(1) Let f is function defined on (a, b] and 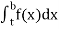 exists for all t ∈ (a, b), then
exists for all t ∈ (a, b), then
If 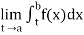 exists, then we define the improper integral of f over (a, b] as follows-
exists, then we define the improper integral of f over (a, b] as follows-
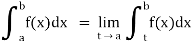
(2) Let f is function defined on [a, b) and 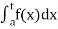 exists for all t ∈ (a, b), then
exists for all t ∈ (a, b), then
If 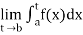 exists, then we define the improper integral of f over [a, b) as follows-
exists, then we define the improper integral of f over [a, b) as follows-
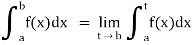
(3) Let f is function defined on [a, c) and (c, b]. if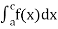 and
and  exist
exist
then we define the improper integral of f over [a, b] as follows-
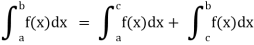
When we apply limits in indefinite integrals are called definite integrals.
If an expression is written as 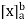 , here ‘b’ is called upper limit and ‘a’ is called lower limit.
, here ‘b’ is called upper limit and ‘a’ is called lower limit.
If f is an increasing or decreasing function on interval [a, b], then
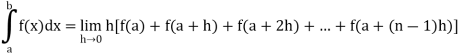
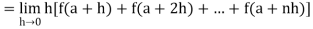
Where 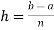
Properties-
1. The definite integral applies only if a<b, but it would be appropriate to include the case a = b and a>b as well, in that case-
If a = b, then
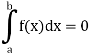
And if a>b, then

2. Integral of a constant function-
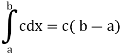
3. Constant multiple property-
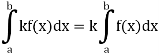
4. Interval union property-
If a < c < b, then
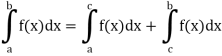
5. Inequality-
If c and d are constants such that 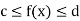 for all x in [a, b], then
for all x in [a, b], then
c (b – a)
Note- if a function f: [a, b] →R is continuous, then the function ‘f’ is always Integrable.
Example-1: Evaluate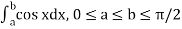 .
.
Sol. Here we notice that f:x→cos x is a decreasing function on [a, b],
Therefore, by the definition of the definite integrals-
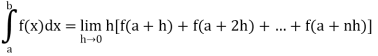
Then
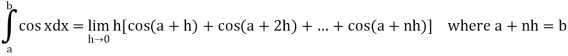
Now,
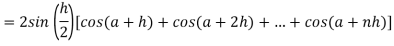
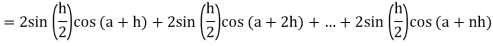
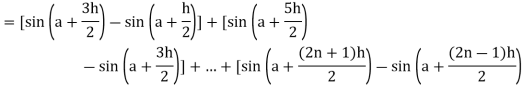
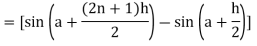
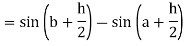
Here 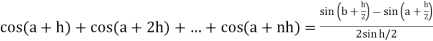
Thus
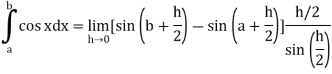
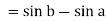
Example-2: Evaluate 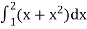
Sol. Here 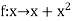 is an increasing function on [1, 2]
is an increasing function on [1, 2]
So that,

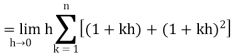
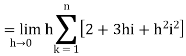
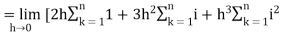 …. (1)
…. (1)
We know that-
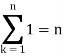
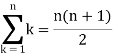
And 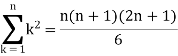
Then equation (1) becomes-
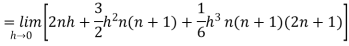
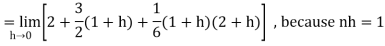
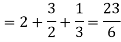
Note- we can find the definite integral directly as-

Example-3: Evaluate-

Sol. 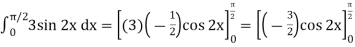
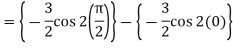
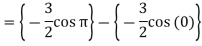
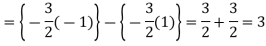
Improper integrals
(1) Let f is function defined on [a, ∞) and it is integrable on [a, t] for all t >a, then
If 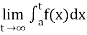 exists, then we define the improper integral of f over [a, ∞) as follows-
exists, then we define the improper integral of f over [a, ∞) as follows-
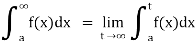
(2) Let f is function defined on (-∞, b] and it is integrable on [t, b] for all t >b, then
If 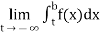 exists, then we define the improper integral of f over (-∞, b] as follows-
exists, then we define the improper integral of f over (-∞, b] as follows-
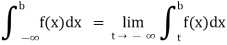
(3) Let f is function defined on (-∞, ∞] and it is integrable on [a, b] for every closed and bounded interval [a, b] which is the subset of R., then
If 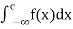 and
and 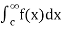 exist for some c belongs to R, then we define the improper integral of f over (-∞, ∞) as follows-
exist for some c belongs to R, then we define the improper integral of f over (-∞, ∞) as follows-
 =
= 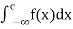 +
+ 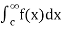
(4) Let f is function defined on (a, ∞) and 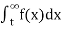 exists for all t>a, then
exists for all t>a, then
If 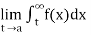 exists, then we define the improper integral of f over (a, ∞) as follows-
exists, then we define the improper integral of f over (a, ∞) as follows-
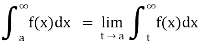
(5) Let f is function defined on (-∞, b) and 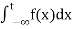 exists for all t<b, then
exists for all t<b, then
If 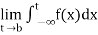 exists, then we define the improper integral of f over (-∞, b) as follows-
exists, then we define the improper integral of f over (-∞, b) as follows-
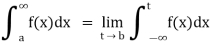
Improper integrals over finite intervals-
(1) Let f is function defined on (a, b] and 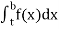 exists for all t ∈ (a, b), then
exists for all t ∈ (a, b), then
If 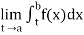 exists, then we define the improper integral of f over (a, b] as follows-
exists, then we define the improper integral of f over (a, b] as follows-
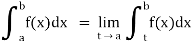
(2) Let f is function defined on [a, b) and 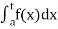 exists for all t ∈ (a, b), then
exists for all t ∈ (a, b), then
If 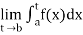 exists, then we define the improper integral of f over [a, b) as follows-
exists, then we define the improper integral of f over [a, b) as follows-
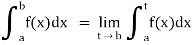
(3) Let f is function defined on [a, c) and (c, b]. if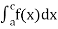 and
and 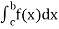 exist
exist
then we define the improper integral of f over [a, b] as follows-
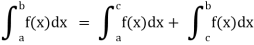
The beta and gamma functions are defined as-
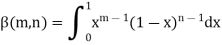
And

These integrals are also known as first and second Eulerian integrals.
Note- Beta function is symmetrical with respect to m and n.
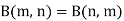
Some important results-

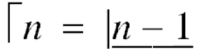
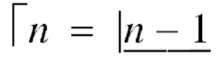
Ex.1: Evaluate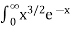 dx
dx
Solution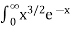 dx =
dx = 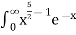 dx
dx
= Γ (5/2)
= Γ (3/2+ 1)
= 3/2 Γ (3/2)
= 3/2. ½ Γ (½)
= 3/2. ½ π
= ¾ π
Ex. 2: Find γ(-½)
Solution: (-½) + 1= ½
Γ (-1/2) = Γ (-½ + 1) / (-½)
= - 2 Γ (1/2)
= - 2 π
Ex. 3: Show that 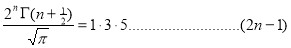

Solution:
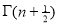 =
= 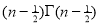
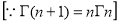
= 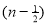
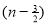
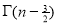
= 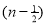
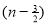
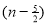
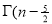 ) .......................
) .......................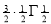
= 
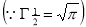
= 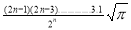
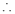
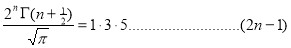
Ex. 4: Evaluate 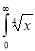
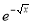 dx.
dx.
Solution: Let 
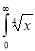
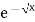 dx
dx
X | 0 | 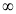 |
t | 0 | 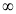 |
Put 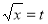 or
or 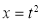 ;dx =2t dt
;dx =2t dt
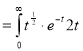 dt
dt
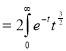 dt
dt
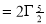
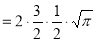
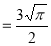
Ex. 5: Evaluate  dx.
dx.
Solution: Let 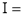
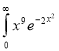 dx.
dx.
x | 0 | 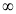 |
t | 0 | 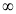 |
Put 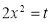 or
or 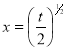 ; 4x dx = dt
; 4x dx = dt
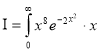 dx
dx
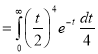
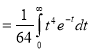
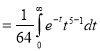
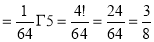
Evaluation of beta function 𝛃 (m, n)-
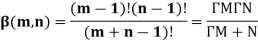
Here we have-
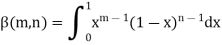
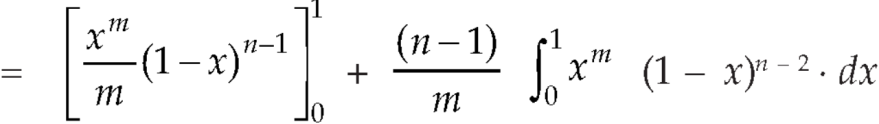
Or
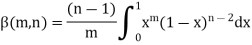
Again, integrate by parts, we get-
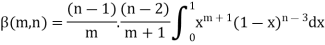
Repeating the process above, integrating by parts we get-
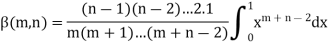
Or
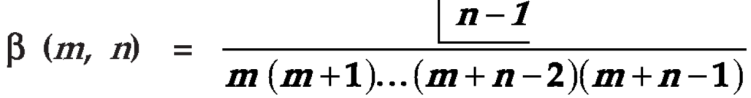
Evaluation of gamma function-

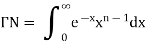
Integrating by parts, we take 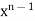 as first function-
as first function-
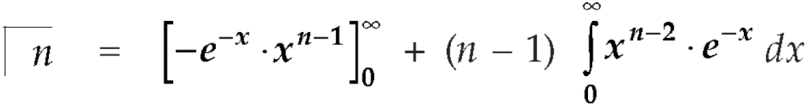
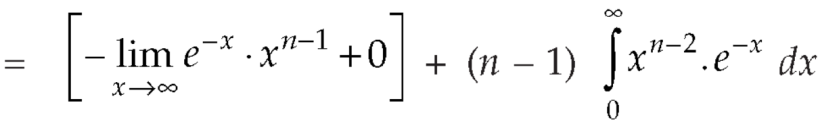
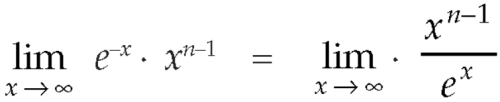
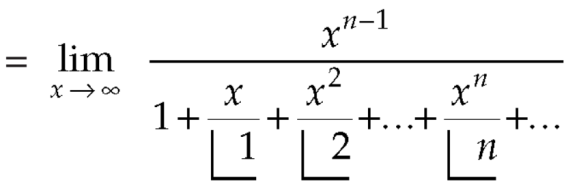
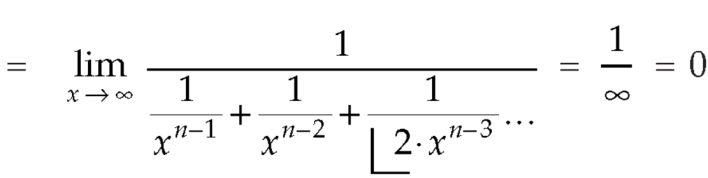
We get-
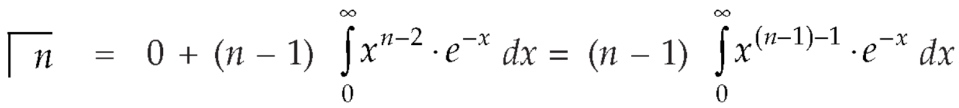
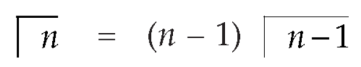
Replace n by n+1,
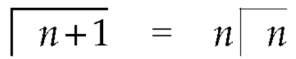
Relation between beta and gamma function-
We know that-
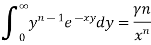
 ………… (1)
………… (1)
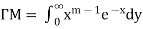 …………………..(2)
…………………..(2)
Multiply equation (1) by 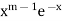 , we get-
, we get-

Integrate both sides with respect to x within limits x = 0 to x = 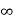 , we get-
, we get-
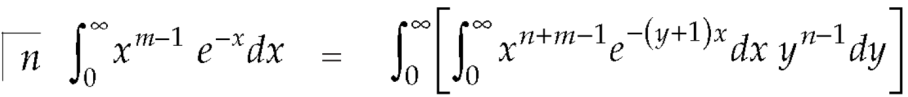
But
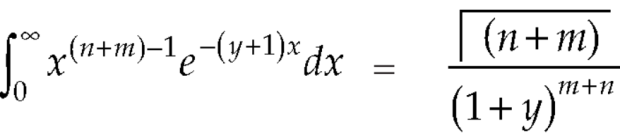
By putting λ = 1 + y and n = m + n
We get by using this result in (2)-
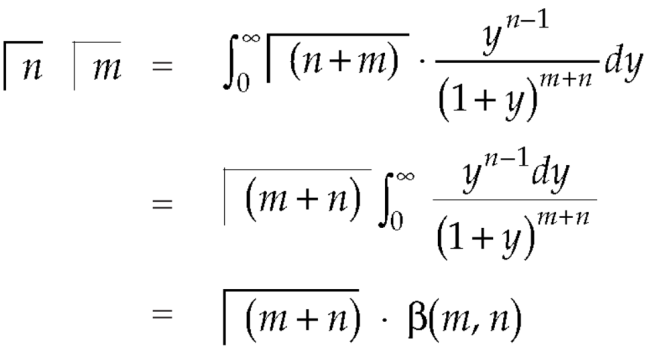
So that-
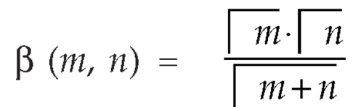
Definition: Beta function
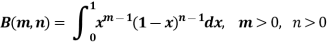
Properties of Beta function:
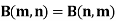
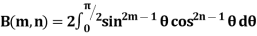
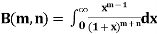

Example (1): Evaluate I = 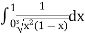
Solution:
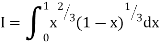
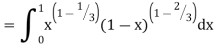
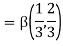
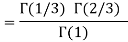
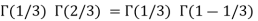
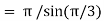
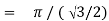
= 2 π/3
Example (2): Evaluate: I = 02 x2 / (2 – x). dx
Solution:
Letting x = 2y, we get
I = (8/2) 01 y2 (1 – y) -1/2dy
= (8/2). B (3, 1/2) = 642 /15
Beta Function More Problems
Relation between Beta and Gamma functions:
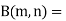
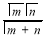
Example (1): Evaluate: I = 0a x4 (a2 – x2). dx
Solution: Letting x2 = a2 y, we get
I = (a6 / 2) 01 y 3/2 (1 – y )1/2dy
= (a6 / 2). B (5/2, 3/2)
= a6 /3 2
Example (2): Evaluate: I = 02 x (8 – x3). dx
Solution: Let x3 = 8y
I = (8/3) 01 y-1/3 (1 – y) 1/3. dy
= (8/3) B (2/3, 4/3)
= 16 π / (9 3)
Example (3): Prove that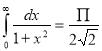
Solution: Let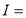
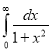
Put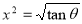 or
or 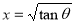 ,
, 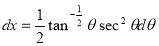
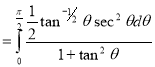
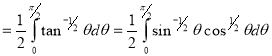
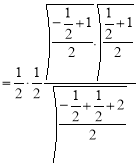
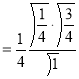

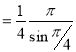
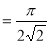
Example (4): Evaluate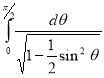
Solution: Let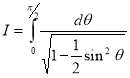
Put 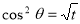 or
or 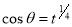 ,
,
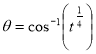 ,
,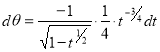
When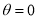 ,
,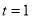 ;
;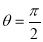 ,
,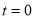
o
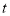
1
0
Also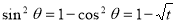
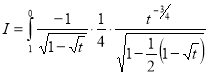
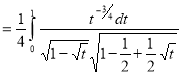
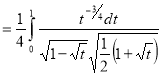
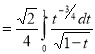
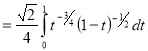
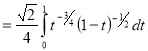
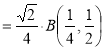
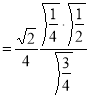
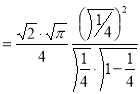
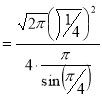
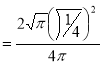
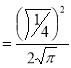
Example (5): Show that
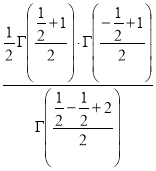
Solution: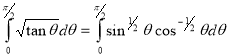
=
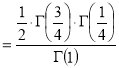

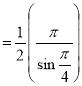
 (
(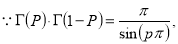 0<p<1)
0<p<1)
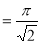
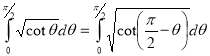
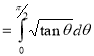
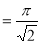 (By above result)
(By above result) 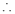
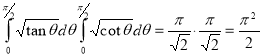
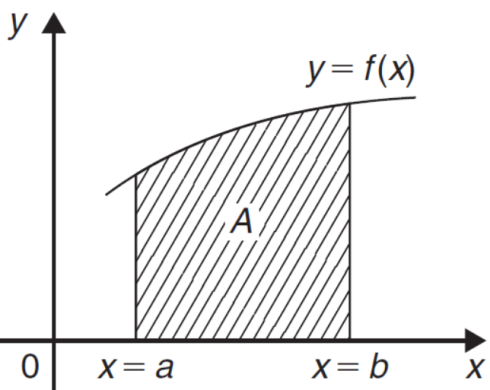
Area under and between the curves-
Total shaded area will be as follows of the given figure (by using definite integrals)-
Total shaded area = 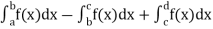

Example-1: Determine the area enclosed by the curves-
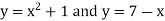
Sol. We know that the curves are equal at the points of interaction, thus equating the values of y of each curve-
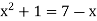
Which gives-

By factorization,
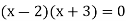
Which means,
x = 2 and x = -3
By determining the intersection points the range the values of x have been found-
x | -3 | -2 | -1 | 0 | 1 | 2 |
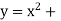 | 10 | 5 | 2 | 1 | 2 | 5 |
And
x | -3 | 0 | 2 |
y = 7 - x | 10 | 7 | 5 |
We get the following figure by using above two tables-
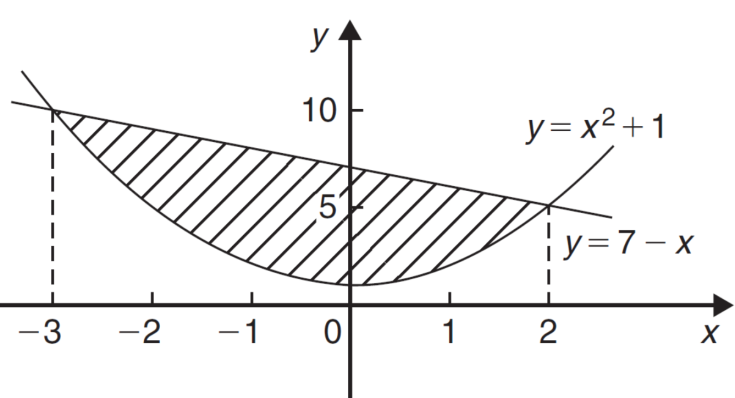
Area of shaded region = 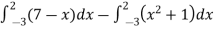
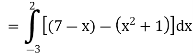
= 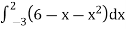
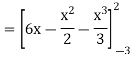
= (12 – 2 – 8/3) – (-18 – 9/2 + 9)
= 
= 125/6 square unit
Example-2: Determine the area bounded by three straight lines y = 4 – x, y = 3x and 3y = x
Sol. We get the following figure by using the equations of three straight lines-
y = 4 – x, y = 3x and 3y = x
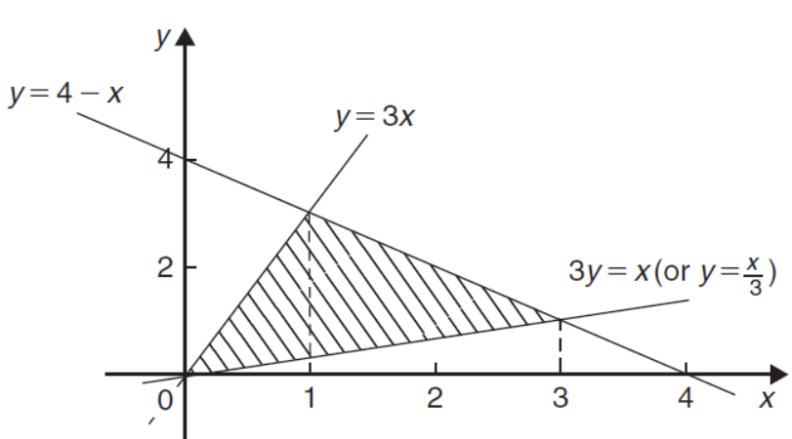
Area of shaded region-
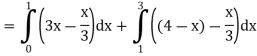
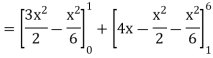
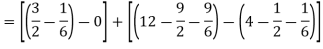
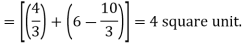
Example-3: Find the area enclosed by the two functions-
 and g(x) = 6 – x
and g(x) = 6 – x
Sol. We get the following figure by using these two equations
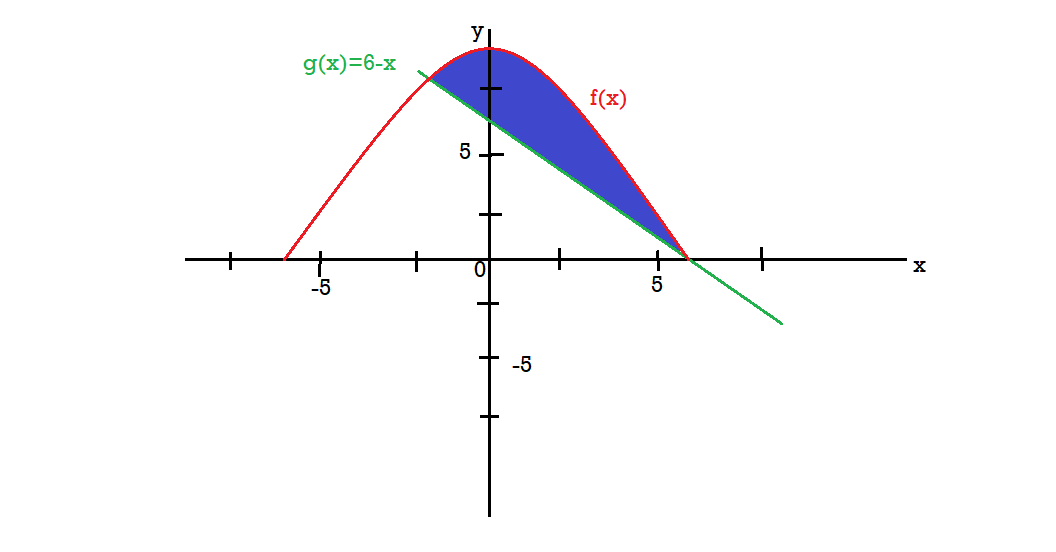
To find the intersection points of two functions f(x) and g(x)-
f(x) = g(x)
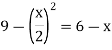
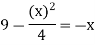
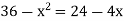
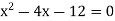
On factorizing, we get-
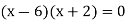
x = 6, -2
Now
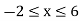
Then, area under the curve-
A = 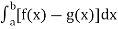
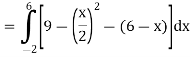
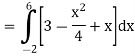
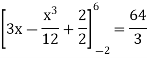
Therefore, the area under the curve is 64/3 square unit.
Areas and volumes of revolutions
The volume of revolution (V) is obtained by rotating area A through one revolution about the x-axis is given by-
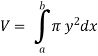
Suppose the curve x = f(y) is rotated 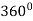 about y-axis between the limits y = c and y = d, then the volume generated V, is given by-
about y-axis between the limits y = c and y = d, then the volume generated V, is given by-
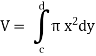
Example-1: Find the area enclosed by the curves 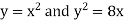 and if the area is rotated
and if the area is rotated 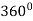 about the x-axis, then determine the volume of the solid of revolution.
about the x-axis, then determine the volume of the solid of revolution.
Sol. We know that, at the point of intersection the coordinates of the curve are equal. So that first we will find the point of intersection-
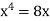
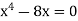
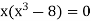
We get,
x = 0 and x = 2
The curve of the given equations will look like as follows-
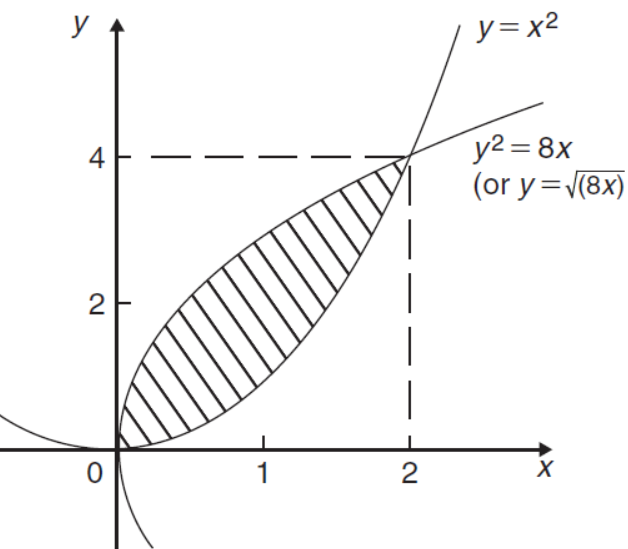
Then,
The area of the shaded region will be-
A = 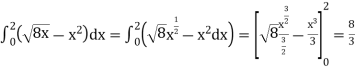
So that the area will be 8/3 square unit.
The volume will be
= (volume produced by revolving 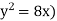 – (volume produced by revolving
– (volume produced by revolving 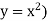
= 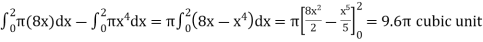
Method of cylindrical shells-
Let f(x) be a continuous and positive function. Define R as the region bounded above by the graph f(x), below by the x-axis, on the left by the line x = a and on the right x = b, then the volume of the solid of revolution formed by revolving R around the y-axis is given by
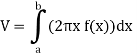
Example-2: Find the volume of the solid of revolution formed by revolving R around y-axis of the function f(x) = 1/x over the interval [1, 3].
Sol. The graph of the function f(x) = 1/x will look like-

The volume of the solid of revolution generated by revolving R (violet region) about the y-axis over the interval [1, 3]
Then the volume of the solid will be-
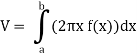
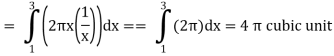
Example-3: Find the volume of the solid of revolution formed by revolving R around y-axis of the function f(x) = 2x - x² over the interval [0, 2].
Sol. The graph of the function f(x) = 2x - x² will be-
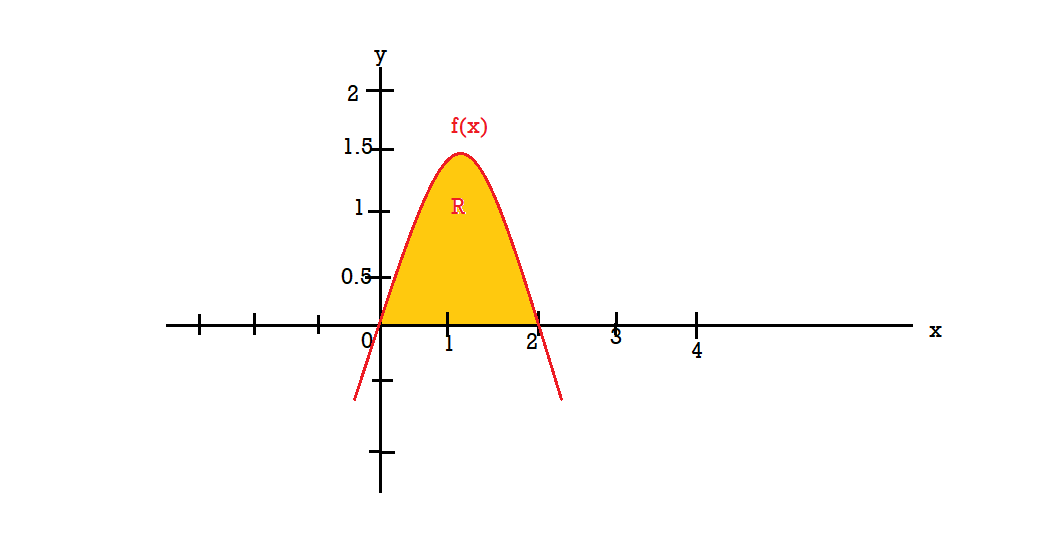
The volume of the solid is given by-
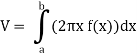
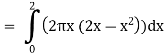
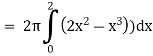
= 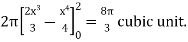
Important definitions-
Continuity- suppose that a function f(x) is defined in the interval I, then it is said to be continuous at x=a, if
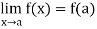
Differentiability- A function f(x) is said to be differentiable at x=a if
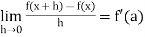 exists where ‘a’ belongs to I
exists where ‘a’ belongs to I
Rolle’s theorem-
Suppose f(x) is a function defined on [a, b] and it satisfies the following conditions
1. f(x) is continuous in [a, b]
2. f(x) is differentiable in (a, b)
3. f(a) = f(b)
Then there exists at least a point c ϵ (a, b), where a<b, such that f’(c) = 0
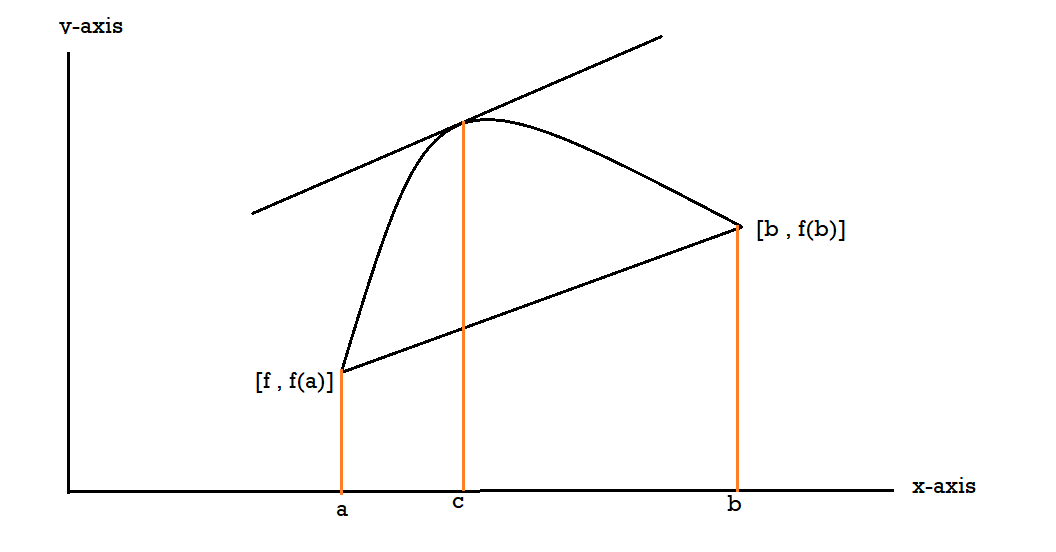
Proof: suppose y = f(x) is a function and A (a, f(a)), B (b, f(b)) be two points on the curve f(x) and a, b are two end points. Now conditions for Rolle’s theorem-
1.f(x) is a continuous function in [a, b], from the figure without breaks in between A & B on y = f(x).
2. f(x) is differentiable in (a, b), because joining A and B we get a line AB.
Slope of the line AB=0 then a point C at P also a tangent at P, or Q, R, S is parallel to x –axis.
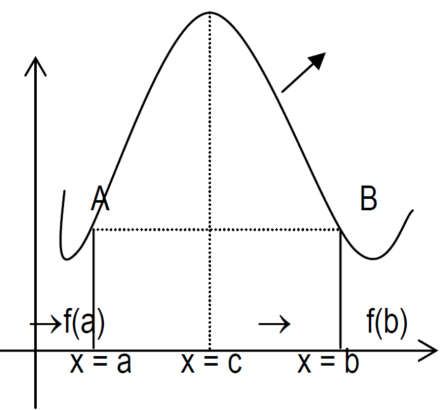
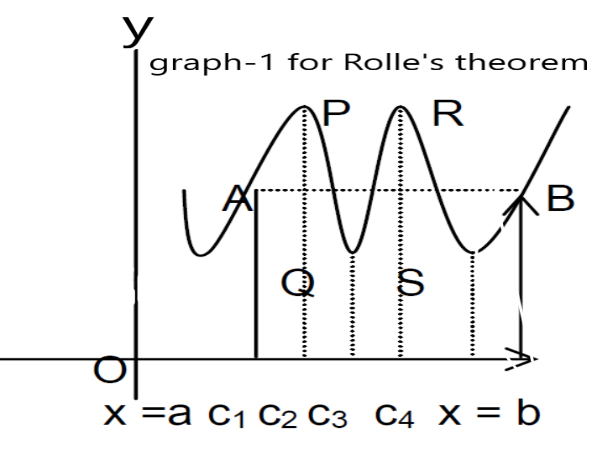
Slope of the tangent at P or Q, R, S, will be 0, even the curve y = f(x) decreases or increases, that means f(x) is constant.
Derivative of f(x),
f’(c) = 0
That’s why, f’(c) = 0
3. The slope of the line AB is equal to zero, that means the line AB is parallel to x-axis.
So that, f(a) = f(b)
Example 1
Verify Rolle’s theorem for the function f(x) = x2 for 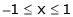
Solution:
Here f(x) = x2; 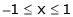
i) Since f(x) is algebraic polynomial which is continuous in [-1, 1]
ii) Consider f(x) = x2
Diff. w.r.t. x we get
f'(x) = 2x
Clearly f’(x) exists in (-1, 1) and does not become infinite.
iii) Clearly
f (-1) = (-1)2 = 1
f (1) = (1)2 = 1
 f (-1) = f (1).
f (-1) = f (1).
Hence by Rolle ’s Theorem, there exist 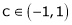 such that
such that
f’(c) = 0
i.e., 2c = 0
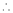 c = 0
c = 0
Thus  such that
such that
f'(c) = 0
Hence Rolle’s Theorem is verified.
Example 2Verify Rolle’s Theorem for the function f(x) = ex (sin x – cos x) in
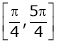
Solution:
Here f(x) = ex (sin x – cos x); 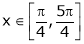
i) Ex is an exponential function continuous for every 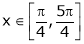 also sin x and cos x are Trigonometric functions Hence (sin x – cos x) is continuous in
also sin x and cos x are Trigonometric functions Hence (sin x – cos x) is continuous in 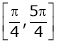 and Hence ex (sin x – cos x) is continuous in
and Hence ex (sin x – cos x) is continuous in 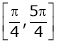 .
.
ii) Consider
f(x) = ex (sin x – cos x)
diff. w.r.t. x we get
f’(x) = ex (cos x + sin x) + ex (sin x + cos x)
= ex [2sin x]
Clearly f’(x) is exist for each 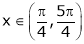 & f’(x) is not infinite.
& f’(x) is not infinite.
Hence f(x) is differentiable in 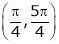 .
.
iii) Consider
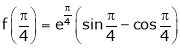
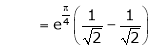
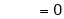
Also,
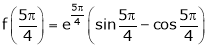
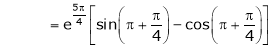
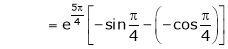
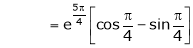
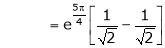

Thus 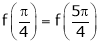
Hence all the conditions of Rolle’s theorem are satisfied, so there exist 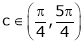 such, that
such, that
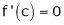
i.e., 
i.e., sin c = 0

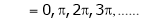
But
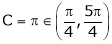
Hence Rolle’s theorem is verified.
Example-3
Verify whether Rolle’s theorem is applicable or not for
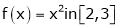
Solution:
Here f(x) = x2; 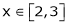
i) X2 is an algebraic polynomial hence it is continuous in [2, 3]
ii) Consider
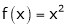

F’(x) exists for each 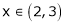
iii) Consider
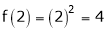
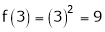
Thus 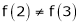 .
.
Thus, all conditions of Rolle’s theorem are not satisfied Hence Rolle’s theorem is not applicable for f(x) = x2 in [2, 3]
Example-4:
Example: Verify Rolle’s theorem for the given functions below-
1. f(x) = x³ - 6x²+11x-6 in the interval [1,3]
2. f(x) = x²-4x+8 in the interval [1,3]
Sol. (1)
As we know that every polynomial is continuous and differentiable for all points, so that the given function is continuous and differentiable in the interval [1,3]
Also, f (1) = f (3) = 0
Now we find f’(x) = 0
3x² - 12x +11 = 0
We get, x = 2+ 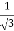 and 2 -
and 2 - 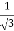
Hence both of them lie in (1,3).
Hence the theorem holds good for the given function in interval [1,3]
(2) As we know that every polynomial is continuous and differentiable for all points, so that the given function is continuous and differentiable in the interval [1,3]
Also, f (1) = 1 -4 +8 = 5 and f (3) = 9 – 12 + 8 = 5
Hence f (1) = f (3)
Now the first derivative of the function,
f’(x) = 0
2x – 4 = 0, gives
X = 2
We can see that 1<2<3, hence there exists 2 between 1 and 3. And f’ (2) = 0.
This means that the Rolle’s theorem holds good for the given function and given interval.
Lagrange’s Mean value Theorem:
Suppose that f(x) be a function of x such that,
1. if it is continuous in [a, b]
2. if it is differentiable in (a, b)
Then there at least exists a value cϵ (a, b)
f’(c) = 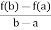
Proof:
L=Lets define a function g(x),
g(x) = f(x) – Ax ………………. (1)
Here A is a constant which is to be determined,
So that, g(a) = g(b)
Now,
g(a) = f(a) – Aa
g(b) = f(b) – Ab
so,
g(a) = g(b),
f(a) – Aa = f(b) – Ab,
Which gives,
A = 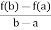 …………………. (2)
…………………. (2)
As right-hand side of eq. (1) is continuous in [a, b], so that g(x) is continuous.
And right-hand side of eq. (1) is differential in (a, b), so that g(x) is differentiable in (a, b).
And g(a) = g(b), because of the choice of A.
Hence g(x) satisfies all the conditions of Rolle’s theorem.
So that,
There exists a value c such that a<c<b at which g’(c) = 0
Now, differentiate eq. (1) with respect to x, we get
g’(x) = f’(x) – A
Here we know that, x = c,
g’(c) = f’(c) – A
As g’(c) = 0, then
f’(c) – A =0
So that, f’(c) = A,
from equation (2), we get
f’(c) = 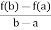
Hence proved.
Example-1:
Verify the Lagrange’s mean value theorem for
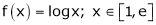
Solution:
Here 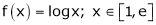
i) Clearly f(x) = log x is logarithmic function. Hence it is continuous in [1, e]
ii) Consider f(x) = log x.
Diff. w.r.t. x we get,
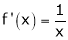
Clearly f’(x) exists for each value of 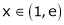 & is finite.
& is finite.
Hence all conditions of LMVT are satisfied Hence at least 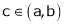
Such that
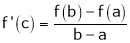
i.e., 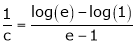
i.e., 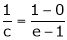
i.e., 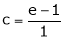
i.e., 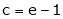
since e = 2.7183
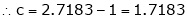
Clearly c = 1.7183 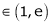
Hence LMVT is verified.
Example-2:
Verify mean value theorem for f(x) = tan-1x in [0, 1]
Solution:
Here 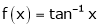 ;
; 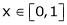
i) Clearly 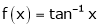 is an inverse trigonometric function and hence it is continuous in [0, 1]
is an inverse trigonometric function and hence it is continuous in [0, 1]
ii) Consider 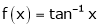
Diff. w.r.t. x we get,
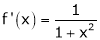
Clearly f’(x) is continuous and differentiable in (0, 1) & is finite
Hence all conditions of LMVT are satisfied, thus there exist 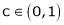
Such that
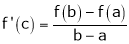
i.e., 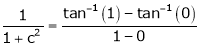
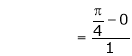
i.e., 
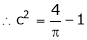
i.e., 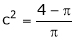
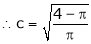
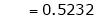
Clearly 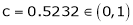
Hence LMVT is verified.
Example-4
Verify Lagrange’s mean value theorem for f(x) = (x-1) (x-2) (x-3) in [0,4].
Sol. As we see that the given function is a polynomial and we know that the polynomial is continuous in [0,4] and differentiable in (0,4).
f(x) = (x-1) (x-2) (x-3)
f(x) = x-6x²+11x-6
Now at x = 0, we get
f (0) = -6 and
at x = 4, we get.
f (4) = 6
Diff. the function w.r.t. x, we get
f’(x) = 3x²-6x+11
Suppose x = c, we get
f’(c) = 3c²-6c+11
By Lagrange’s mean value theorem,
f’(c) = 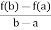 =
= 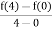 =
= 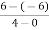 = 3
= 3
Now we get,
3c²-6c+11 = 3
3c²-6c+8 = 0
On solving the quadratic equation, we get
C = 2 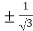
Here we see that the value of c lies between 0 and 4
Therefore, the given function is verified.
Meaning of sign of Derivative:
Let f(x) satisfied LMVT in [a, b]
Let x1 and x2 be any two points laying (a, b) such that x1< x2
Hence by LMVT, 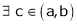 such that
such that 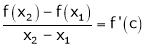
i.e., 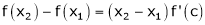 … (1)
… (1)
Cast I:
If 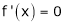
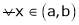 then
then 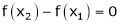
i.e., 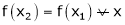
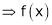 is constant function
is constant function
Case II:
If 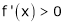
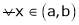 then from equation (1)
then from equation (1)
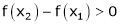
i.e., 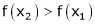
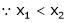 means x2 - x1> 0 and
means x2 - x1> 0 and 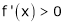
Thus, for x2> x1
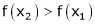
Thus f(x) is increasing function is (a, b)
Case III:
If 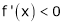
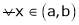
Then from equation (1)
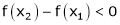
i.e., 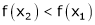
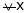
since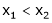 and
and 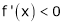 then
then 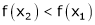
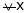 hence f(x) is strictly decreasing function.
hence f(x) is strictly decreasing function.
Example-5:
Prove that
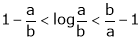
And hence show that
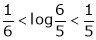
Solution:
Let
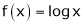 ;
; 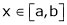
i) Clearly 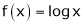 is a logarithmic function and hence it is continuous also
is a logarithmic function and hence it is continuous also
ii) Consider 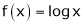
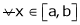
Diff. w.r.t. x we get,

Clearly f’(x) exist and finite in (a, b) Hence f(x) is continuous and differentiable in (a, b). Hence by LMVT 
Such that
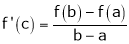
i.e., 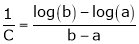
i.e., 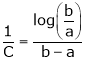
Since
a < c < b
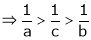
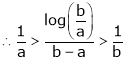
i.e., 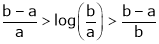
i.e., 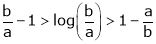
i.e., 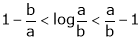
i.e., 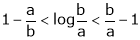
Hence the result
Now put a = 5, b = 6 we get
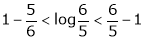
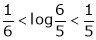
Hence the result
Example-6:
Prove that 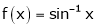 ,
, 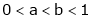 use mean value theorem to prove that,
use mean value theorem to prove that,
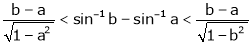
Hence show that
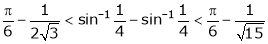
Solution:
i) Let f(x) = sin-1x; 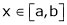
ii) Clearly f(x) is inverse trigonometric function and hence it is continuous in [a, b]
iii) Consider f(x) = sin-1x
diff. w.r.t. x we get,
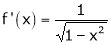
Clearly f’(x) is finite and exists for 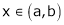 . Hence by LMVT,
. Hence by LMVT, 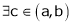 such that
such that
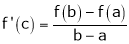
i.e., 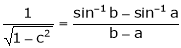
since a < c < b
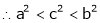
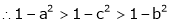
i.e., 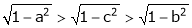
i.e., 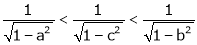
i.e., 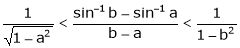
i.e., 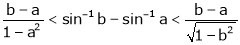
Hence the result
Put 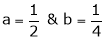 we get
we get
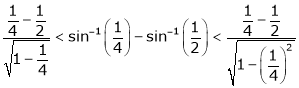
i.e., 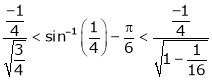
i.e., 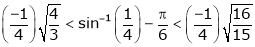
i.e., 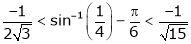
i.e., 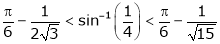
Hence the result
Cauchy’s Mean Value Theorem:
Suppose we have two functions f(x) and g(x) of x, such that,
1. both functions are continuous in [a, b]
2. both functions are differentiable in (a, b)
3. g’(x) ≠ 0 for any x ϵ (a, b)
These three exists at least, x = c ϵ (a, b), at which
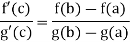
Proof: suppose, we define a function,
h(x) = f(x) – A.g(x) ……………………. (1)
so that h(a) = h(b) and A is a constant to be determined.
Now,
h(a) = f(a) – Ag(a)
h(b) = f(b) – A.g(b)
So that,
f(a) – Ag(a) = f(b) – A.g(b), which gives
A = 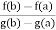 ……………………………. (2)
……………………………. (2)
Now, h(x) is continuous in [a, b] as RHS of eq. (1) is continuous in [a, b] and h(x) is diff. in (a, b) as RHS of eq. (1) is diff. in (a, b)
Also,
h(a) = h(b)
Therefore, all the conditions of Rolle’s theorem are satisfied then there exists a value x = cϵ (a, b)
So that h’(c) = 0
Differentiate eq. (1) w.r.t. x, we get
h’(x) = f’(x) – A.g’(x)
At x = c
h’(c) = f’(c) – A.g’(c)
0 = f’(c) – A.g’(c)
A = 
So that, we get
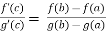 where a<c<b
where a<c<b
Hence the Cauchy’s mean value theorem is proved.
Example-7:
Verify Cauchy mean value theorems for 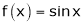 &
&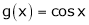 in
in 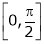
Solution:
Let 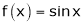 &
&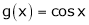 ;
; 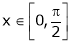
i) Clearly f(x) and g(x) both are trigonometric functions. Hence continuous in 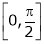
ii) Since 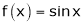 &
&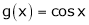
diff. w.r.t. x we get,
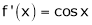 &
&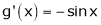
Clearly both f’(x) and g’(x) exist & finite in 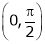 . Hence f(x) and g(x) are derivable in
. Hence f(x) and g(x) are derivable in 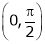 and
and
iii) 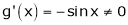
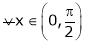
Hence by Cauchy mean value theorem, there exist at least 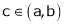 such that
such that
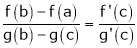
i.e., 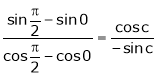
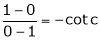
i.e., 1 = cot c

i.e., 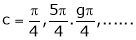
Clearly
Hence Cauchy mean value theorem is verified.
Example-8:
Considering the functions ex and e-x, show that c is arithmetic mean of a & b.
Solution:
i) Clearly f(x) and g(x) are exponential functions Hence they are continuous in [a, b].
ii) Consider 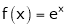 &
&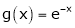
Diff. w.r.t. x we get
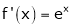 and
and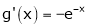
Clearly f(x) and g(x) are derivable in (a, b)
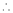 By Cauchy’s mean value theorem
By Cauchy’s mean value theorem 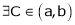 such that
such that
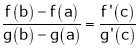
i.e., 
i.e., 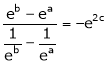
i.e., 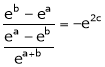
i.e., 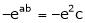
i.e., 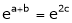
i.e., 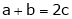
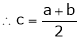
Thus 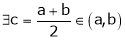
i.e., c is arithmetic mean of a & b.
Hence the result
Example-9
Show that 
Prove that if 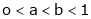
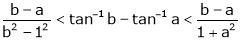 and hence show that
and hence show that
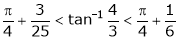
Verify Cauchy’s mean value theorem for the function x2 and x4 in [a, b] where a, b > 0
If for 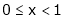 then prove that,
then prove that,
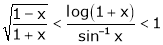
[Hint: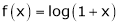 ,
, 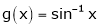 ]
]
Example-10:
Verify Cauchy’s mean value theorem for the function f(x) = x⁴ and g(x) = x² in the interval [1,2]
Sol. We are given, f(x) = x⁴ and g(x) = x
Derivative of these functions,
f’(x) = 4x³ and g’(x) = 2x
Put these values in Cauchy’s formula, we get
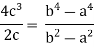
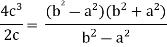
2c² = 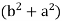
c² = 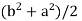
c = 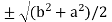
now put the values of a = 1 and b = 2, we get
c = 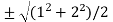 =
=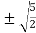 =
= 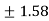 (approx)
(approx)
Hence the Cauchy’s theorem is verified.
Taylor’s Series Expansion:
a) The expansion of f(x+h) in ascending power of x is
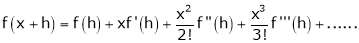
b) The expansion of f(x+h) in ascending power of h is
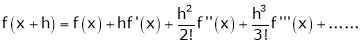
c) The expansion of f(x) in ascending powers of (x-a) is,
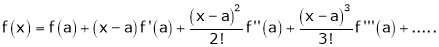
Using the above series expansion, we get series expansion of f(x+h) or f(x).
Expansion of functions using standard expansions-
Example-1:
Expand 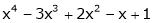 in power of (x – 3)
in power of (x – 3)
Solution:
Let 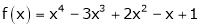
Here a = 3
Now by Taylor’s series expansion,
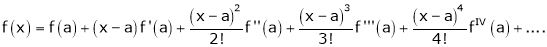 … (1)
… (1)
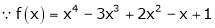
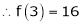
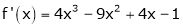

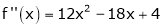

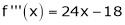
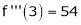
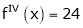
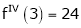
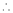 equation (1) becomes.
equation (1) becomes.
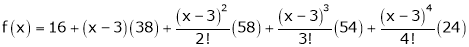
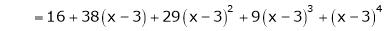
Example-2:
Using Taylors series method expand
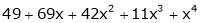 in powers of (x + 2)
in powers of (x + 2)
Solution:
Here 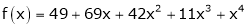
a = -2
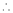 By Taylors series,
By Taylors series,
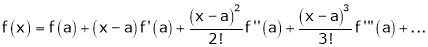 … (1)
… (1)
Since
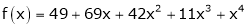
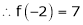
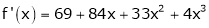
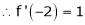
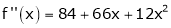
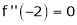
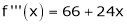
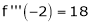
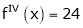
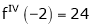 ,
, 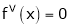 , …..
, …..
Thus equation (1) becomes
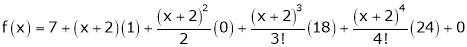
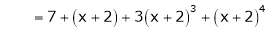
Example-3:
Expand 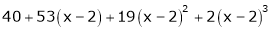 in ascending powers of x.
in ascending powers of x.
Solution:
Here
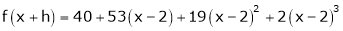
i.e., 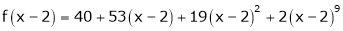
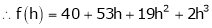
Here h = -2
 By Taylors series,
By Taylors series,
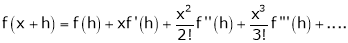 … (1)
… (1)
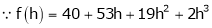
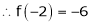
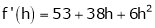
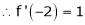

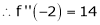
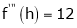
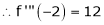
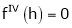
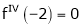
 equation (1) becomes,
equation (1) becomes,
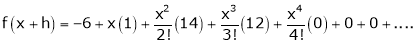
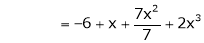
Thus

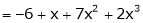
Example-4:
Expand 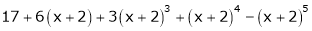 in powers of x using Taylor’s theorem,
in powers of x using Taylor’s theorem,
Solution:
Here
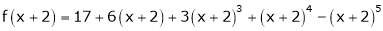
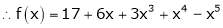
i.e., 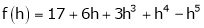
Here
h = 2
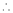 By Taylors series
By Taylors series
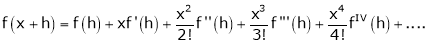 … (1)
… (1)
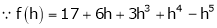
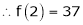
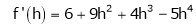
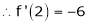
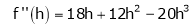
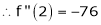
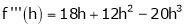

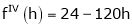
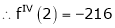
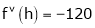
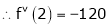
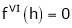
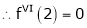
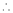 By equation (1)
By equation (1)
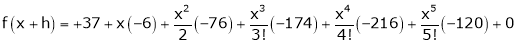
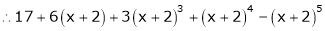
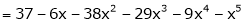
Let we have two functions f(x) and g(x) and-
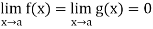
Then-
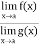 is an expression of the form
is an expression of the form  , in that case we can say that f(x)/g(x) is an indeterminate for of the type
, in that case we can say that f(x)/g(x) is an indeterminate for of the type  at x = a.
at x = a.
Now, let we have two functions f(x) and g(x) and-
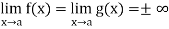
Then-
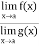 is an expression of the form
is an expression of the form  , in that case we can say that f(x)/g(x) is an indeterminate for of the type
, in that case we can say that f(x)/g(x) is an indeterminate for of the type  at x = a.
at x = a.
Some other indeterminate forms are 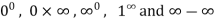
L’Hospital’s rule for  form-
form-
Working steps-
1. Check that the limits f(x)/g(x) is an indeterminate form of type  .
.
(Note- we cannot apply L’Hospital rule if it is not in indeterminate form)
2. Differentiate f and g separately.
3. Find the limits of the derivatives. If the limit is finite,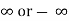 then it is equal to the limit of f(x)/g(x).
then it is equal to the limit of f(x)/g(x).
Example-1: Evaluate 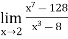
Sol. Here we notice that it is an indeterminate form of .
.
So that, we can apply L’Hospital rule-
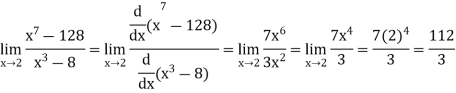
Example-2: Evaluate  .
.
Sol. Let f(x) = 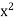 and g(x) =
and g(x) = 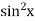 .
.
Here we see that this is the indeterminate form of 0/0 at x = 0.
Now by using L’Hospital rule, we get-
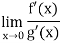
= 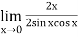
= 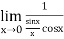
= 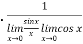 = 1
= 1
Note- Suppose we get an indeterminate form even after finding first derivative, then in that case, we use the other form of L’Hospital’s rule.
If we have f(x) and g(x) are two functions such that 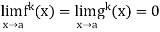
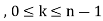 .
.
If 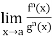 exist or (∞, -∞), then
exist or (∞, -∞), then
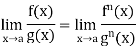
Example-3: Evaluate 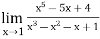
Sol. Let f(x) = 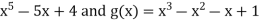 , then
, then
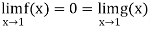
And
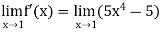 = 0
= 0
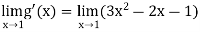 = 0
= 0
But if we use L’Hospital rule again, then we get-
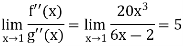
Example-4: Evaluate 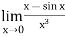
Sol. We can see that this is an indeterminate form of type 0/0.
Apply L’Hospital’s rule, we get
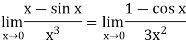
But this is again an indeterminate form, so that we will again apply L’Hospital’s rule-
We get
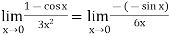 =
= 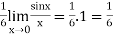
L’Hospital’s rule for  form-
form-
Let f and g are two differentiable functions on an open interval containing x = a, except possibly at x = a and that
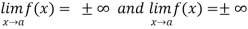
If 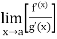 has a finite limit, or if it is
has a finite limit, or if it is 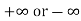 , then
, then
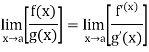
Theorem- If we have f(x) and g(x) are two functions such that 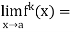
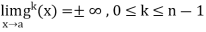 .
.
If 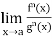 exist or (∞, -∞), then
exist or (∞, -∞), then
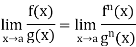
Example-5: Find 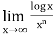 , n>0.
, n>0.
Sol. Let f(x) = log x and g(x) = 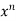
These two functions satisfied the theorem that we have discussed above-
So that,
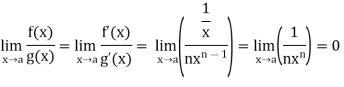
Example-6: Evaluate 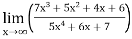
Sol. Apply L’Hospital rule as we can see that this is the form of 
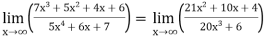 =
= 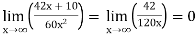
Example-7:
Note- In some cases like above example, we cannot apply L’Hospital’s rule.
Other types of indeterminate forms-
Example-8: Evaluate 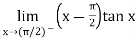
Sol. Here we find that-

So that this limit is the form of 0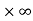 .
.
Now,
Change 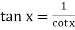 to obtain the limit-
to obtain the limit-
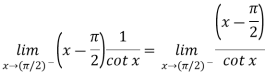
Now this is the form of 0/0,
Apply L’Hospital’s rule-
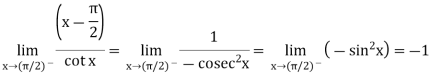
If f(x) is a single valued function defined in a region R then
Maxima is a maximum point 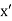 if and only if
if and only if 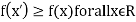
Minima is a minimum point 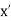 if and only if
if and only if 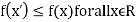
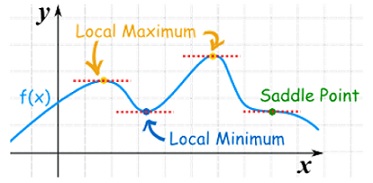

 Maxima and Minima of a function of two independent variables
Maxima and Minima of a function of two independent variables
Let 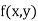 be a defined function of two independent variables.
be a defined function of two independent variables.
Then the point  is said to be a maximum point of
is said to be a maximum point of  if
if
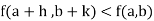
Or  =
= 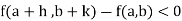
For all positive and negative values of h and k.
Similarly, the point 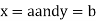 is said to be a minimum point of
is said to be a minimum point of 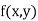 if
if
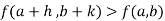
Or 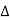 =
= 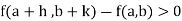
For all positive and negative values of h and k.
Saddle point: Critical points of a function of two variables are those points at which both partial derivatives of the function are zero. A critical point of a function of a single variable is either a local maximum, a local minimum, or neither. With functions of two variables there is a fourth possibility - a saddle point.
A point is a saddle point of a function of two variables if
![2 2 [ 2 ] 2
@f-= 0, @f--= 0, and @-f- @-f-- -@-f- < 0
@x @y @x2 @y2 @x @y](https://glossaread-contain.s3.ap-south-1.amazonaws.com/epub/1643029727_781974.png)
at the point.
Stationary Value
The value 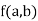 is said to be a stationary value of
is said to be a stationary value of 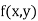 if
if
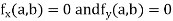
i.e., the function is a stationary at (a, b).
Rule to find the maximum and minimum values of 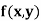
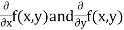
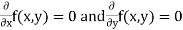

4. (a) If 
(b) If 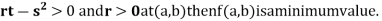
(c) If 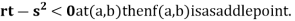
(d) If 
Example1 Find out the maxima and minima of the function
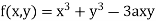
Given  …(i)
…(i)
Partially differentiating (i) with respect to x we get
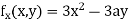 …. (ii)
…. (ii)
Partially differentiating (i) with respect to y we get
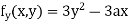 …. (iii)
…. (iii)
Now, form the equations 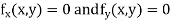
Using (ii) and (iii) we get
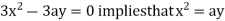
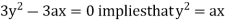
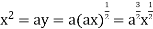 using above two equations
using above two equations
Squaring both side we get

Or 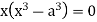
This show that 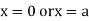
Also, we get 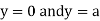
Thus, we get the pair of value as 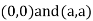
Now, we calculate
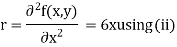
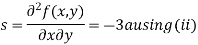
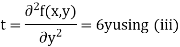
Putting above values in

At point (0,0) we get
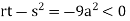
So, the point (0,0) is a saddle point.
At point 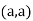 we get
we get
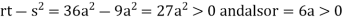
So, the point 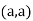 is the minimum point where
is the minimum point where 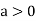
In case 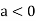
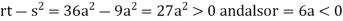
So, the point 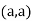 is the maximum point where
is the maximum point where 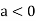
Example2 Find the maximum and minimum point of the function
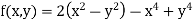
Partially differentiating given equation with respect to and x and y then equate them to zero
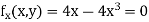
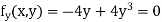
On solving above, we get 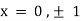
Also 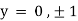
Thus, we get the pair of values (0,0), (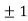 ,0) and (0,
,0) and (0,
Now, we calculate
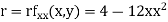
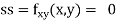
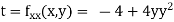
At the point (0,0)
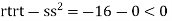
So, function has saddle point at (0,0).
At the point (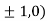
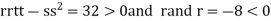
So, the function has maxima at this point (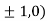 .
.
At the point (0,
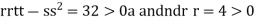
So, the function has minima at this point (0,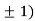 .
.
At the point (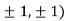
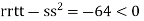
So, the function has a saddle point at (
Example3 Find the maximum and minimum value of 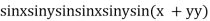
Let 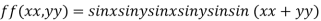
Partially differentiating given function with respect to x and y and equate it to zero
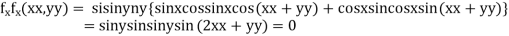 ...(i)
...(i)
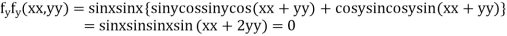 ...(ii)
...(ii)
On solving (i) and (ii) we get

Thus, pair of values are 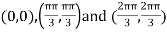
Now, we calculate
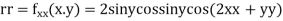
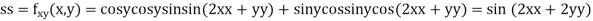
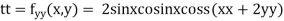
At the point (0,0)
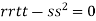
So further investigation is required
On the x axis y = 0, f(x,0) =0
On the line y=x, 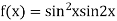
At the point 

So that the given function has maximum value at 
Therefore, maximum value of given function
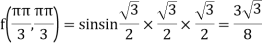
At the point 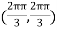
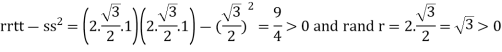
So that the given function has minimum value at 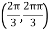
Therefore, minimum value of the given function

References:
1. G.B. Thomas and R.L. Finney, “Calculus and Analytic Geometry”, Pearson, 2002.
2. T. Veerarajan, “Engineering Mathematics”, McGraw-Hill, New Delhi, 2008.
3. B. V. Ramana, “Higher Engineering Mathematics”, McGraw Hill, New Delhi, 2010.
4. N.P. Bali and M. Goyal, “A Text Book of Engineering Mathematics”, Laxmi Publications, 2010.
5. B.S. Grewal, “Higher Engineering Mathematics”, Khanna Publishers, 2000.