Unit - 4
Multivariable calculus: Integration
Double integral –
Before studying about multiple integrals, first let’s go through the definition of definition of definite integrals for function of single variable.
As we know, the integral
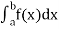
Where is belongs to the limit a ≤ x ≤ b
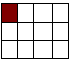
This integral can be written as follows-
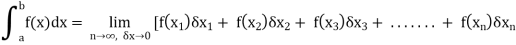
Now suppose we have a function f (x, y) of two variables x and y in two-dimensional finite region Rin xy-plane.
Then the double integration over region R can be evaluated by two successive integrations
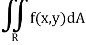
Evaluation of double integrals-
If A is described as 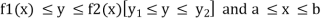
Then,
 ]dx
]dx
Let do some examples to understand more about double integration-
Example-1: Evaluate 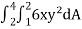 , where dA is the small area in xy-plane.
, where dA is the small area in xy-plane.
Sol. Let, I = 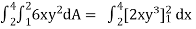
= 
= 
= 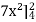
= 84 sq. unit.
Which is the required area.
Example-2: Evaluate 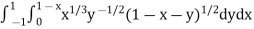
Sol. Let us suppose the integral is I,
I = 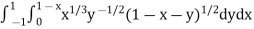
Put c = 1 – x in I, we get
I = 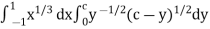
Suppose, y = ct
Then dy = c
now we get,
I = 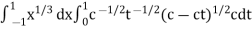
I = 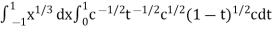
I = 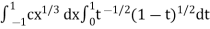
I = 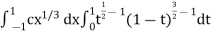
I =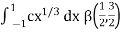
As we know that by beta function,
Which gives,
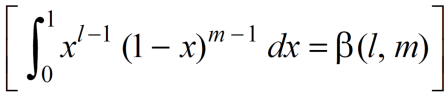
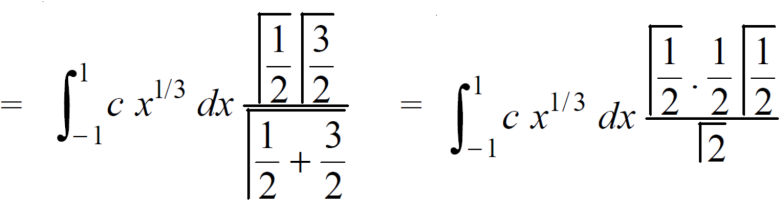
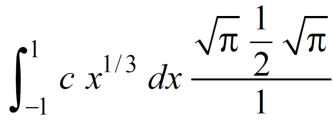
Now put the value of c, we get
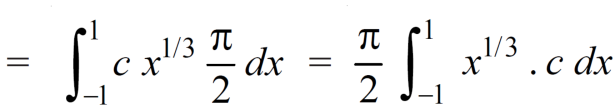
Example-3: Evaluate the following double integral,
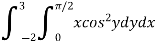
Sol. Let,
I = 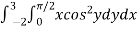
On solving the integral, we get
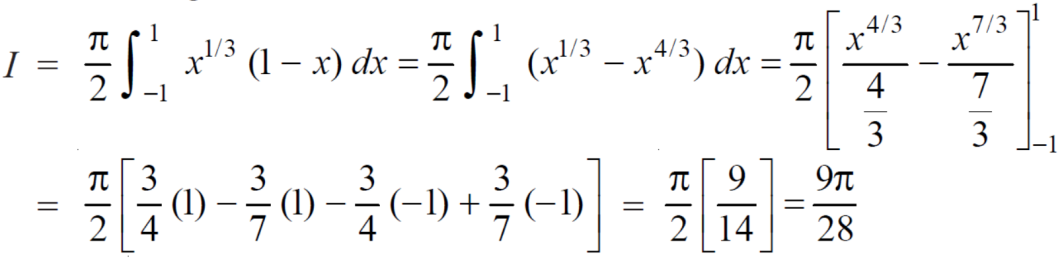
Double integration in polar coordination
In polar coordinates, we need to evaluate
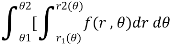
Over the region bounded by θ1 and θ2.
And the curves r1(θ) and r2(θ)
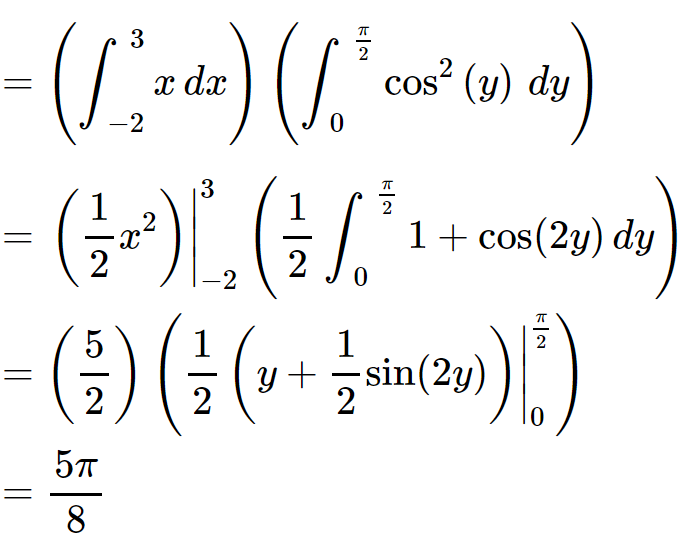
Example-1: Evaluate the following by changing to polar coordinates,
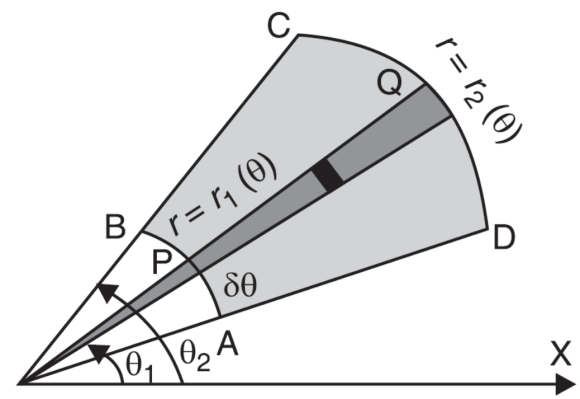
Sol. In this problem, the limits for y are 0 to 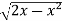 and the limits for are 0 to 2.
and the limits for are 0 to 2.
Suppose,
y = 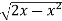
Squaring both sides,
y² = 2x - x²
x² + y² = 2x
But in polar coordinates,
We have,
r² = 2r cosθ
r = 2 cosθ
From the region of integration, r lies from 0 to 2 cosθ and θ varies from 0 to π / 2.
As we know in case of polar coordinates,
Replace x by r cosθ and y by r sinθ, dy dx by r drdθ,
We get,

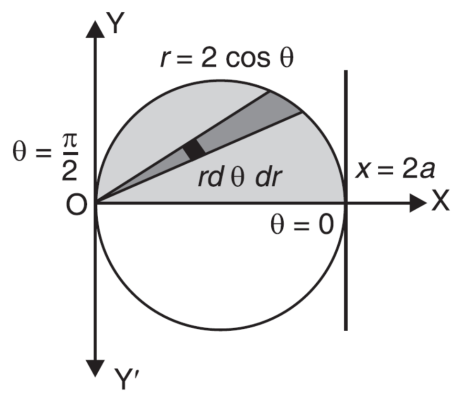
Example-2: Evaluate the following integral by converting into polar coordinates.
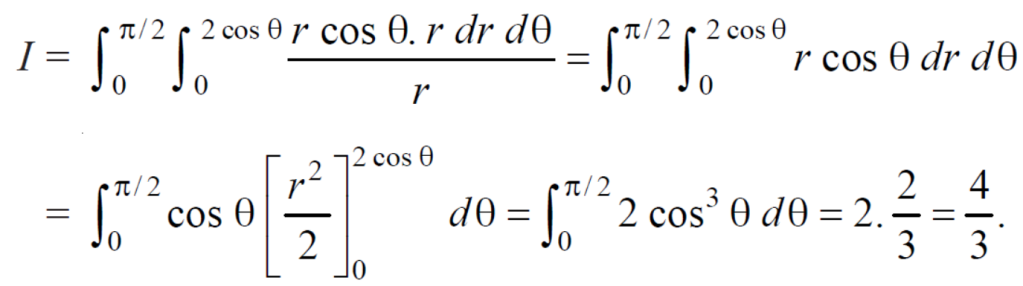
Sol. Here limits of y,
y = 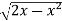
y² = 2x - x²
x² + y² = 2x
x² + y² - 2x = 0 ……………… (1)
Eq. (1) represent a circle whose radius is 1 and centre is (1, 0)
Lower limit of y is zero.
Region of integration in upper half circle,
First, we will convert into polar coordinates,
By putting
x by r cosθ and y by r sinθ, dy dx by r drdθ,
Limits of r are0 to 2 cosθ and limits of θ are from 0 to π / 2.
Example-3: Evaluate 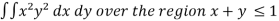
Sol. Let the integral,
I = 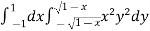
=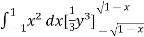
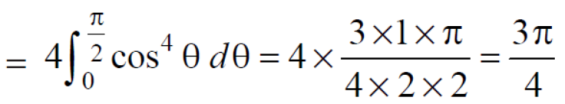
Put x = sinθ
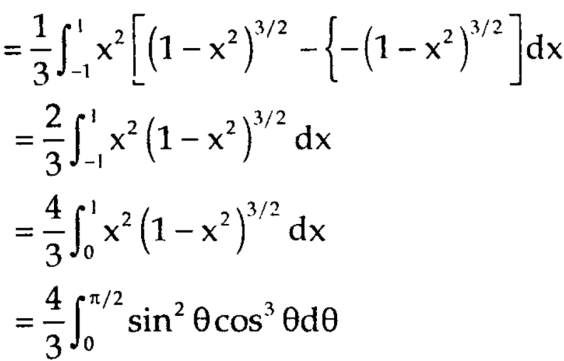
= π / 24 ans.
Triple integrals
Definition: Let f (x, y, z) be a function which is continuous at every point of the finite region (Volume V) of three-dimensional space. Divide the region V into n sub regions of respective volumes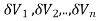 . Let (
. Let (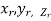 ) be a point in the
) be a point in the 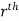 sub region then the sum:
sub region then the sum:
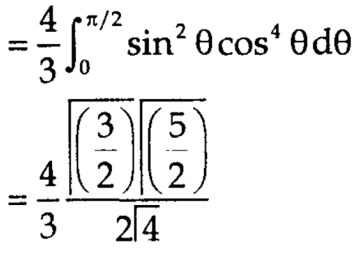 =
= 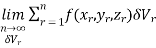
is called triple integration of f (x, y, z) over the region V provided limit on R.H.S of above Equation exists.
Spherical Polar Coordinates
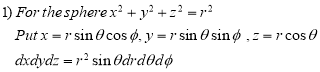
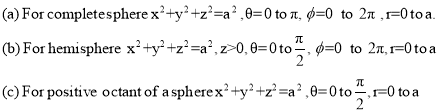
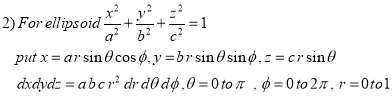
Where the integral is extended to all positive values of the variables subjected to the condition 
Ex.1: Evaluate
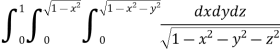
Solution: Let
I = 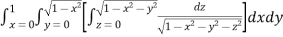
= 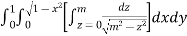
(Assuming m = 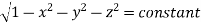 )
)
= 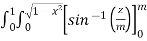 dxdy
dxdy
= 
=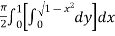
= 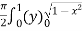 dx
dx
= 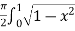 dx
dx
= 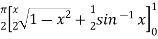
=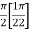
I =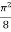
Ex.2: Evaluate 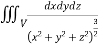 Where V is annulus between the spheres
Where V is annulus between the spheres 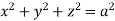
and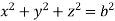 (
(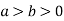 )
)
solution: It is convenient to transform the triple integral into spherical polar co-ordinate by putting
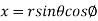 ,
, 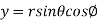 ,
, 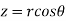
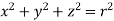 , dxdydz=
, dxdydz=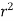 sin
sin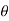 drd
drd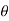 d
d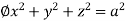 ,
,
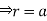 and
and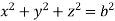
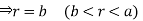
For the positive octant, r varies from r =b to r =a, 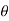 varies from
varies from 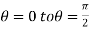
and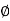 varies from
varies from 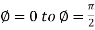
I= 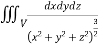
= 8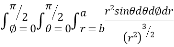
=8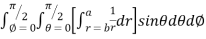
=8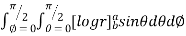
=8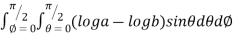
=8 log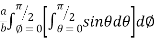
= 8 log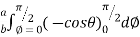
I= 8 log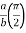 I = 4 log
I = 4 log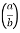
Ex.3: Evaluate 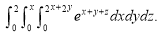
Solution: -
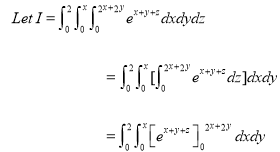
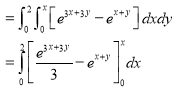
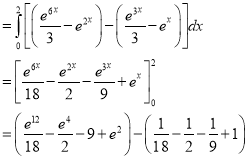
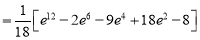
Ex.4: Evaluate
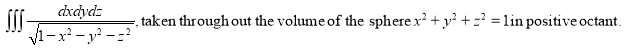 Solution:
Solution:

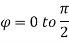
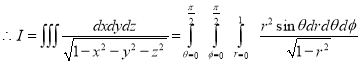
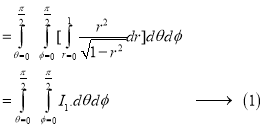
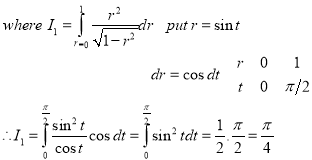
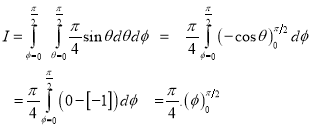
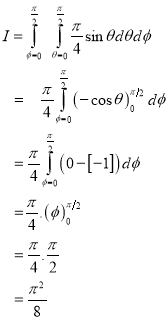
NOTES:
The volume of solid is given by
Volume =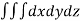
In Spherical polar system
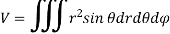
In cylindrical polar system
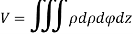
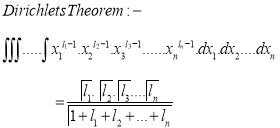
Ex.1: Find Volume of the tetrahedron bounded by the co-ordinate’s planes and the plane
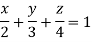
Solution: Volume =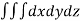 ………. (1)
………. (1)
Put 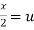 ,
, 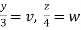
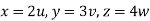
From equation (1) we have
V = 
=24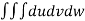
=24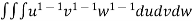 (u+v+w=1) By Dirichlet’s theorem.
(u+v+w=1) By Dirichlet’s theorem.
=24 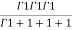
= =
=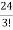 = 4
= 4
Volume =4
Ex.2: Find volume common to the cylinders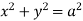 ,
,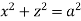 .
.
Solution: For given cylinders,
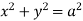 ,
, 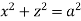 .
.
Z varies from
Z=- to z =
to z = 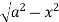
Y varies from
y= -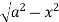 to y =
to y = 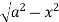
x varies from x= -a to x = a
By symmetry,
Required volume= 8 (volume in the first octant)
=8 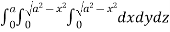
=8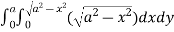
= 8 dx
dx
=8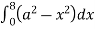
=8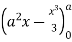
=8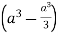
Volume = 16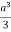
Ex.3: Evaluate
1. 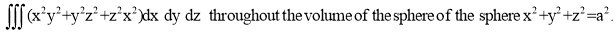 Solution: -
Solution: -
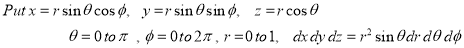
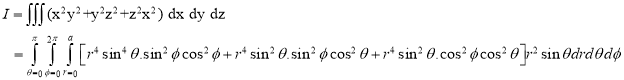
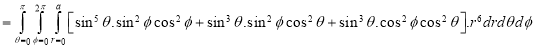
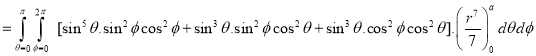
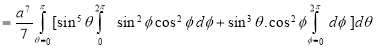
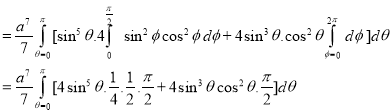
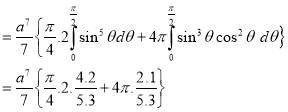
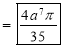
Ex.4: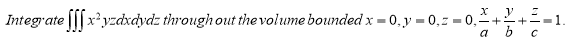
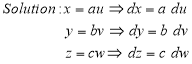
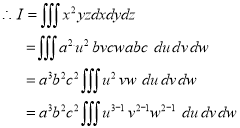
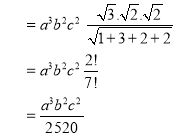
Ex.5: Evaluate
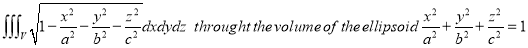
Solution: Put
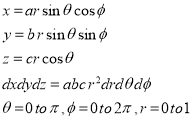
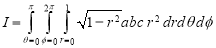

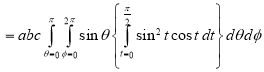
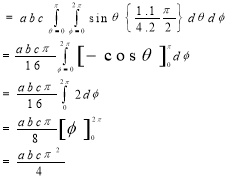
The limits of integration change when we change the order of integration.
We draw the rough diagram of the region of integration to find the new limits.
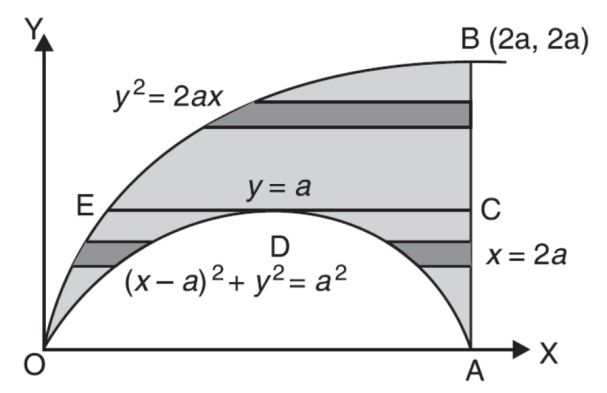
Example-1: Change the order of integration in the double integral-
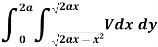
Sol.
Limits are given-
x = 0, x = 2a
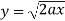
And
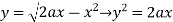
and

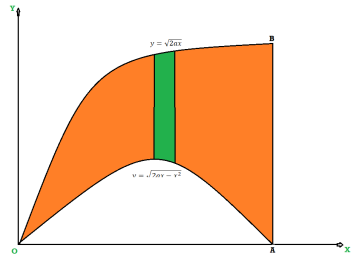
The area of integration is the shaded portion of OAB. On changing the order of integration first we will integrate with respect to x, the area of integration has three portions BCE, ODE and ACD,

Now-
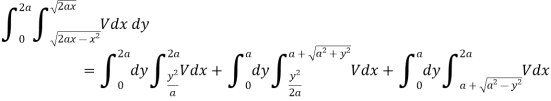
Which is the required answer.
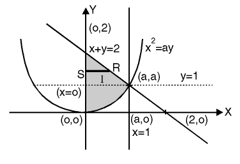
Example-2: Change the order of integration for the integral 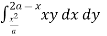 and evaluate the same with reversed order of integration.
and evaluate the same with reversed order of integration.
Sol:
Given, I = 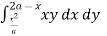 … (1)
… (1)
In the given integration, limits are
y =  , y = 2a – x and x = 0, x = a
, y = 2a – x and x = 0, x = a
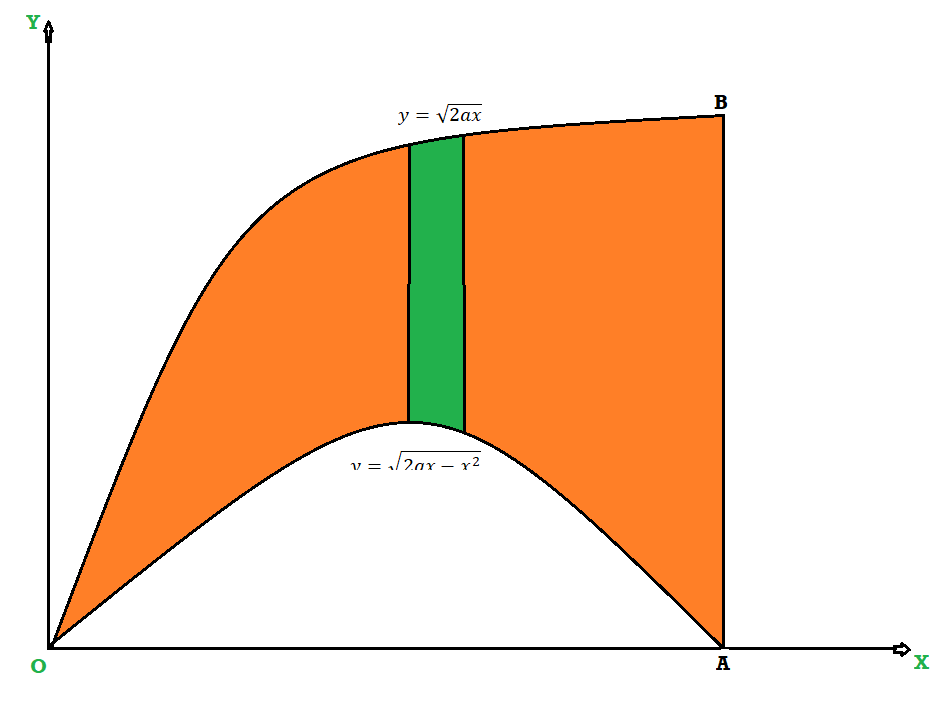
The region bounded by x2 = ay, x + y = 2a
And x = 0, x = a is as shown in Fig.
Here we have to change order of Integration. Given the strip is vertical.
Now take horizontal strip SR.
To take total region, divide region into two parts by taking line y = a.
1st Region:
Along strip, y constant and x vary from x = 0 to x = 2a – y. Slide strip parallel to x-axis therefore y varies from y = a to y = 2a.
I1 = dyxy dx …(2)
2nd Region:
Along strip, y constant and x vary from x = 0 to x= . Slide strip parallel to x-axis therefore x-varies from y = 0 to y = a.
I2 = dyxy dx … (3)
From Equation (1), (2) and (3),
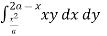 = dyxy dx + dyxy dx
= dyxy dx + dyxy dx
= 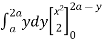 + y dy
+ y dy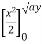
=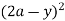 dy + (ay) dy = y (4a2 – 4ay + y2) dy + ay2dy
dy + (ay) dy = y (4a2 – 4ay + y2) dy + ay2dy
= (4a2 y – 4ay2 + y3) dy + y2dy
= 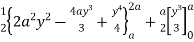
= 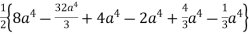 +
+ 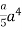
= a4
Example-3: Evaluate
I = 
Soln.:
Given: I = 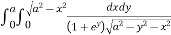 … (1)
… (1)
In the given integration, limits are
x = 0, x = a, y = 0, y = 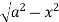
The bounded region is as shown in Fig. **.
In the given, strip is vertical. Now take horizontal strip SR. along strip y constant and x vary from x = 0 to
x = 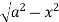 . Slide strip IIel to X-axis therefore y varies from y = 0 to y = a.
. Slide strip IIel to X-axis therefore y varies from y = 0 to y = a.
I = dy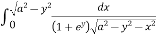
= 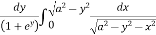
Put a2 – y2 = b2
I = 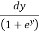
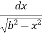
= 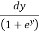 =
= 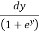
= 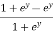 dy =
dy = 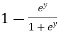 dy
dy
= 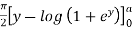
=
= 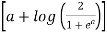
= 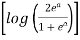 [∵ a = a loge]
[∵ a = a loge]
I = dy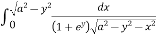 =
= 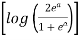
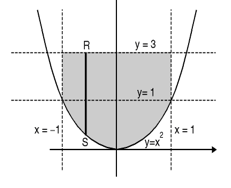
Example-4: Express as single integral and evaluate dy dx + dy dx.
Soln.:
Given: I = dy dx + dy dx
I = I1 + I2
The limits of region of integration I1 are
x = – ; x = and y = 0, y = 1 and I2 are x = – 1,
x = 1 and y = 1, y = 3.
The region of integration is as shown in Fig.
To consider the complete region take a vertical strip SR along the strip y varies from y = x2 to
y = 3 and x varies from x = –1 to x = 1.
I = 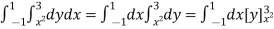
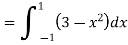
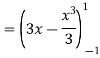
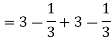
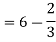
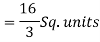
We can solve the double integration by changing independent variables.
Let the double integral is-
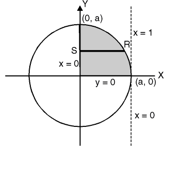
It’s to be changed by the new variables-u and v
The relationship between x, y and u, v is-
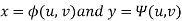
Then the double integration is converted into-
Where-
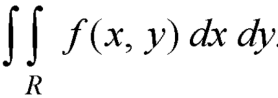
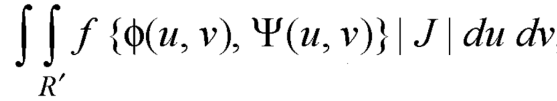
Example-: Evaluate-

Where-
R is the region bounded by a parallelogram- x + y = 0, x + y = 2, 3x – 2y = 0, 3x – 2y = 3.
Sol.
By changing the variables x, y to the new variables u and v, by the substitution x + y = u, 3x – 2y = v, the given parallelogram R reduces to a rectangle 
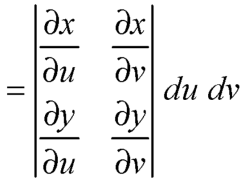
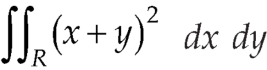

The required Jacobian is-

Since u = x + y and u = x + y = 2, here u varies from 0 to 2 while v varies from 0 to 3.
Since-
3x – 2y = v = 0, 3x – 2y = v = 3
Therefore, the integral will be in new variables-
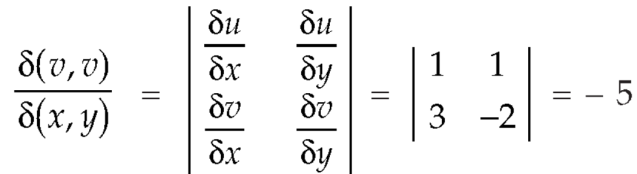
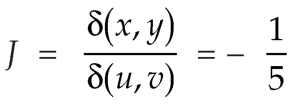
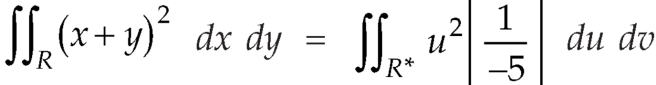
Which is the required answer.
Example: Evaluate  the transformation is x = v and y = uv.
the transformation is x = v and y = uv.
Sol.
Here the region of integration R is the triangle which is bounded by y = 0, x = 1 and y = x
Here put,
X = u and y = uv, we get-

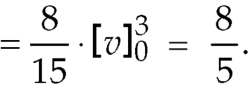
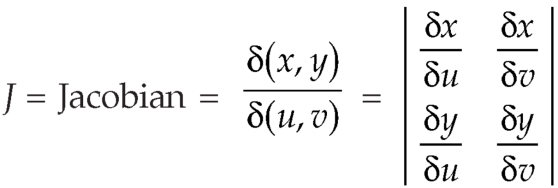
Here x varies from 0 to 1 while y varies from 0 to x.
Since u = x so u varies from 0 to 1
Here, similarly, since 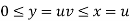 , so that v varies from 0 to 1. Thus-
, so that v varies from 0 to 1. Thus-
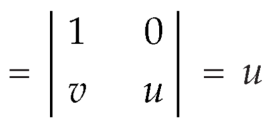
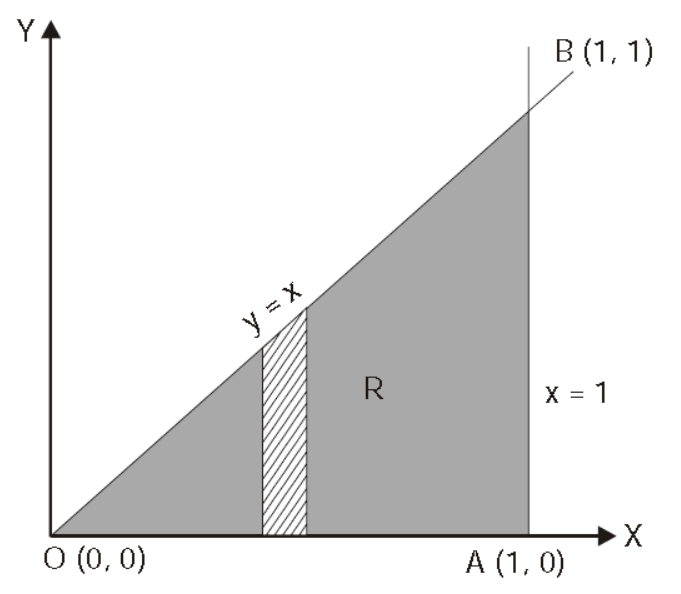
4.4 Applications: Areas and Volumes By (Double Integration)
Area of double integration
Area in Cartesian coordinates-
Example-1: Find the area enclosed by two curves using double integration.
y = 2 – x and y² = 2 (2 – x)
Sol. Let,
y = 2 – x ………………. (1)
and y² = 2 (2 – x) ………………. (2)
On solving eq. (1) and (2)
We get the intersection points (2,0) and (0,2),
We know that,
Area = 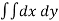
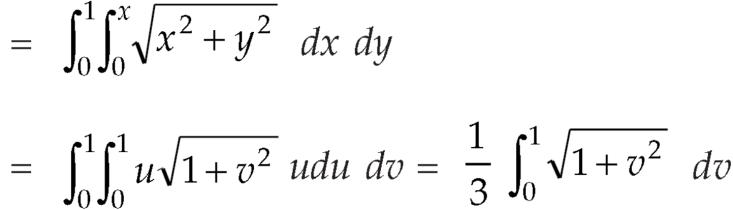
Here we will find the area as below,
Area = 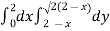
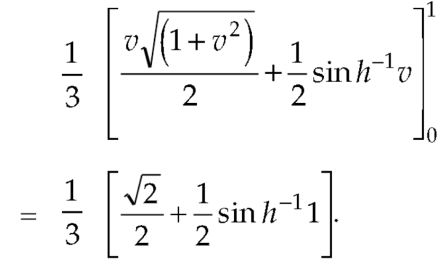
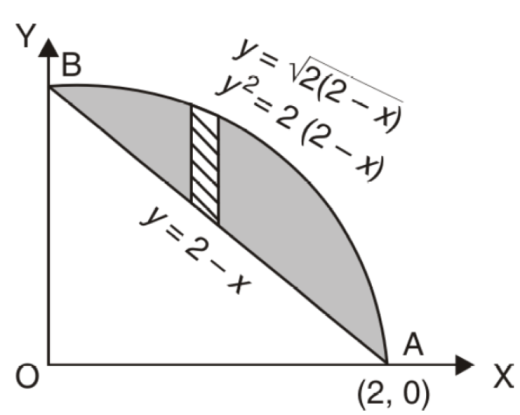
Which gives,
= (- 4 + 4 /2) + 8 / 3 = 2 / 3.
Example-2: Find the area between the parabola y ² = 4ax and another parabola x² = 4ay.
Sol. Let,
y² = 4ax ………………. (1)
and
x² = 4ay…………………. (2)
then if we solve these equations, we get the values of points where these two curves intersect
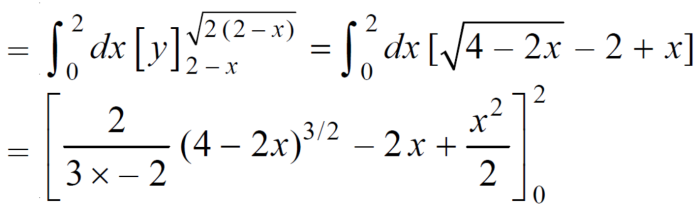
x varies from y²/4a to 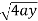 and y varies from o to 4a,
and y varies from o to 4a,
Now using the concept of double integral,
Area = 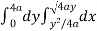
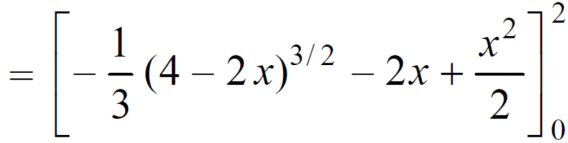
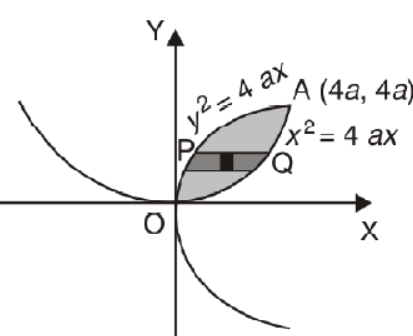
Area in polar coordinates-
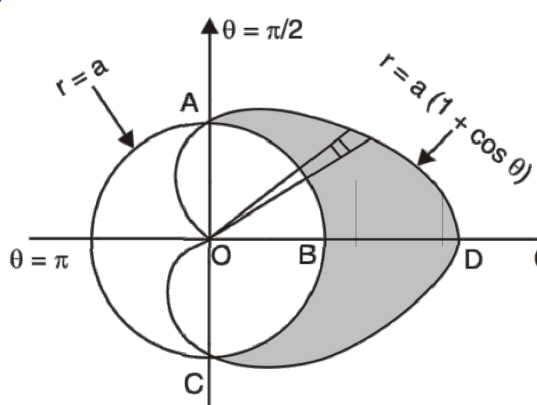
Example-3: Find the area lying inside the cardioid r = a(1+cosθ) and outside the circle r = a, by using double integration.
Sol. We have,
r = a(1+cosθ) ……………………. (1)
And
r = a ………………………………. (2)
On solving these equations by eliminating r, we get
a(1+cosθ) = a
(1+cosθ) = 1
cosθ = 0
Here a θ varies from – π/2 to π/2
Limit of r will be a and 1+cosθ)
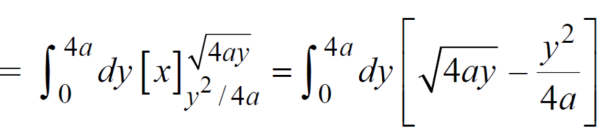
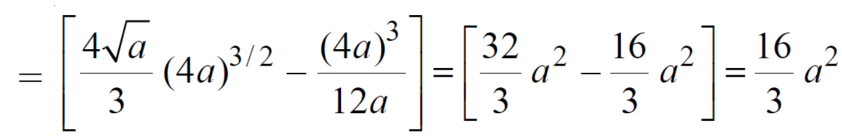
Which is the required area.
Example-4: Find the are lying inside a cardioid r = 1 + cosθ and outside the parabola r (1 + cosθ) = 1.
Sol. Let,
r = 1 + cosθ ……………………. (1)
r (1 + cosθ) = 1……………………. (2)
solving these equations, we get
(1 + cosθ) (1 + cosθ) = 1
(1 + cosθ) ² = 1
1 + cosθ = 1
cosθ = 0
θ = ±π / 2
So that, limits of r are,
1 + cosθ and 1 / 1 + cosθ
The area can be founded as below,
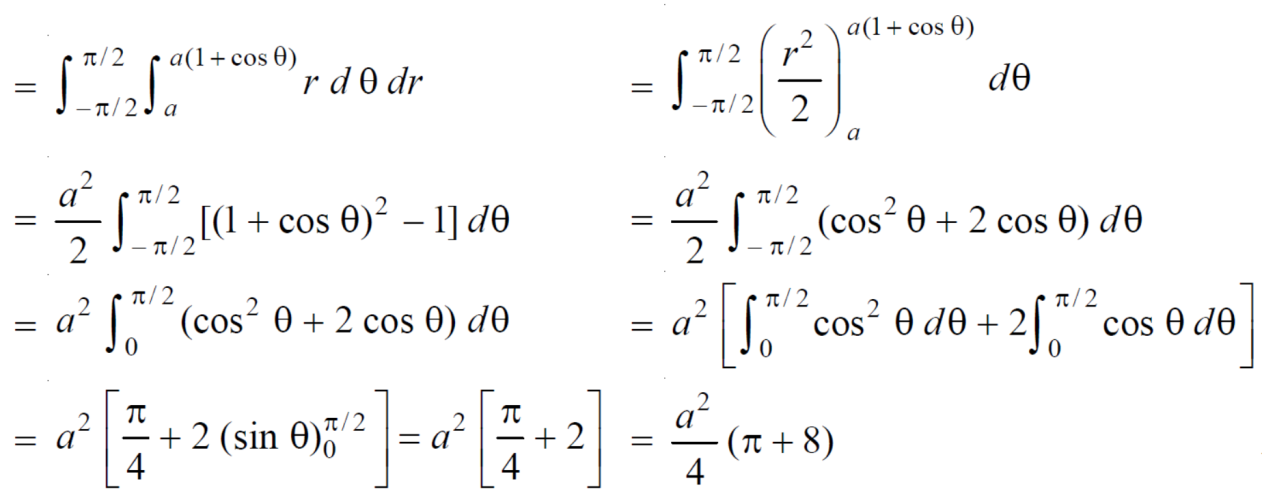
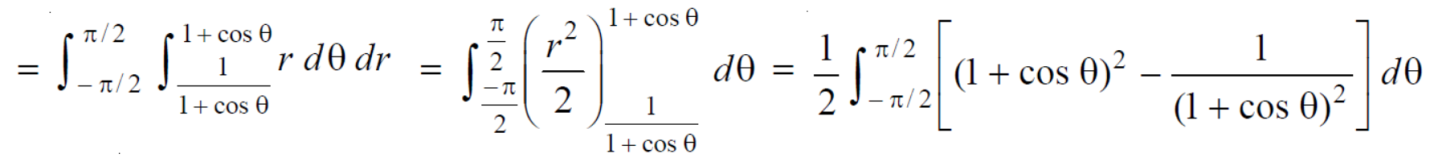
Double integrals as volumes
Suppose we have a curve y = f(x) is revolved about an axis, then a solid is generated, now we need to find out the volume of the solid generated,
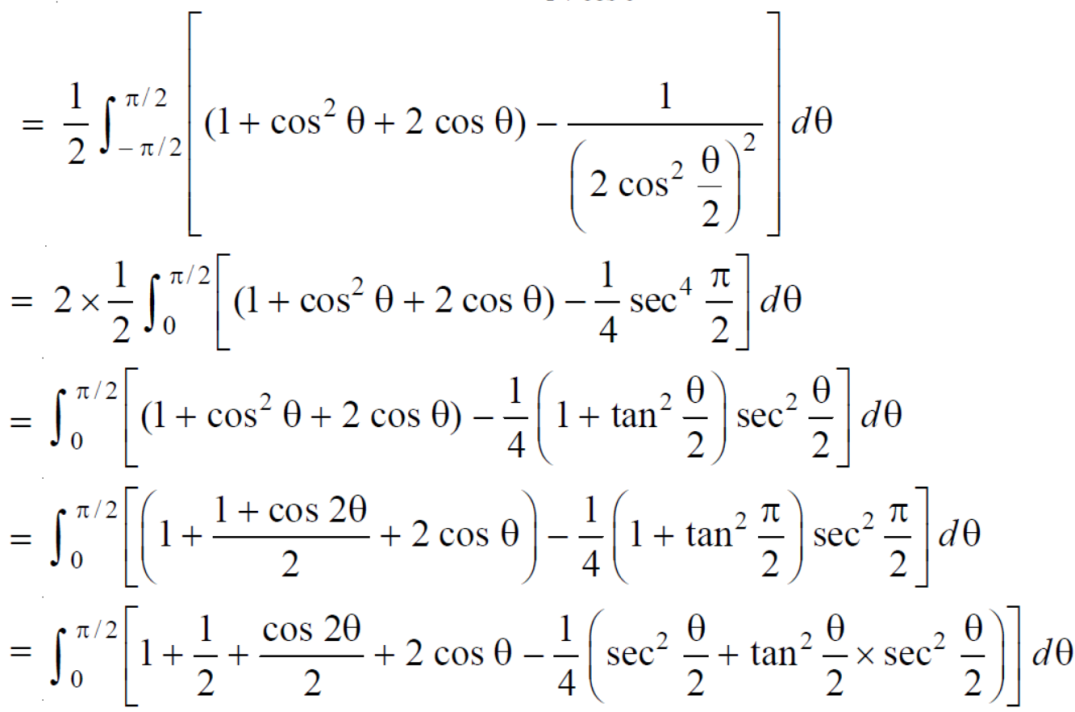
The formula for volume of the solid generated about x-axis,
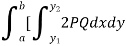
Example-1: Calculate the volume generated by the revolution of a cardioid,
r = a (1 – cosθ) about its axis
Sol. Here, r = a (1 – cosθ)
Volume =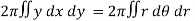
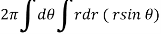
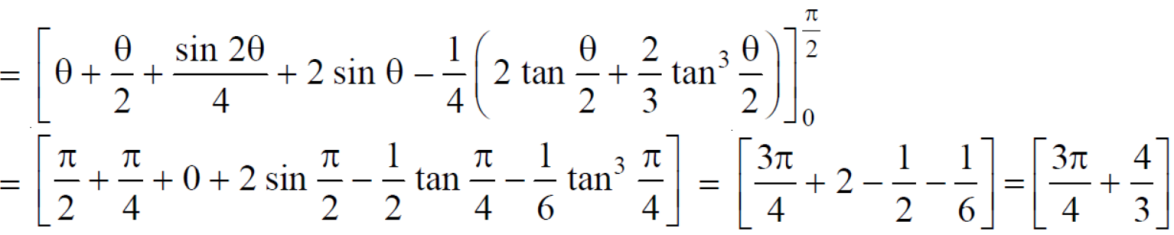
=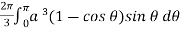
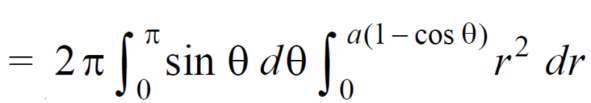
Which is the volume of generated by cardioid.
Example-2: Find the volume generated by revolving a circle x ² + y² = 4 about the line x= 3.
Sol. We know that,
Volume = 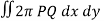
Here , PQ = 3 – x,
= 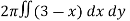
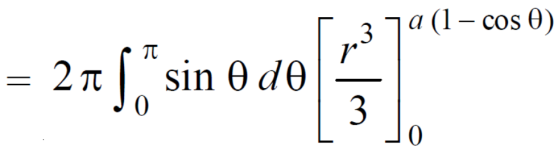
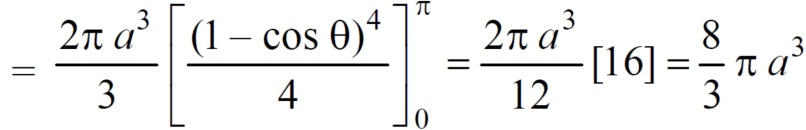
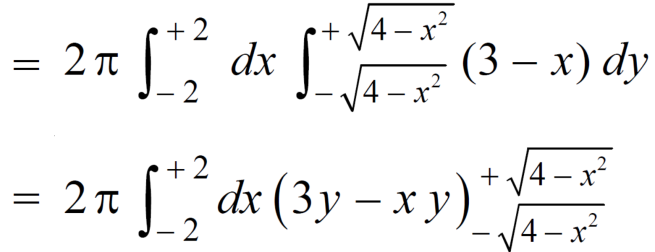
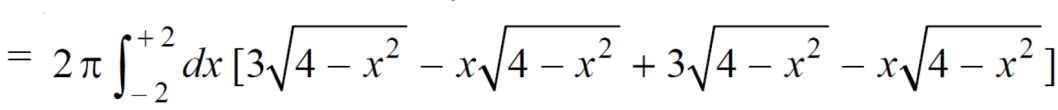
The volume is 24π².
Example-3: Calculate the volume under the surface z = 3 + x² - 2y over the region R defined as 0≤ x ≤ 1 and -x ≤ y ≤ x
Sol. The is a double integral of z = 3 + x² - 2y over the region R
Volume will be,
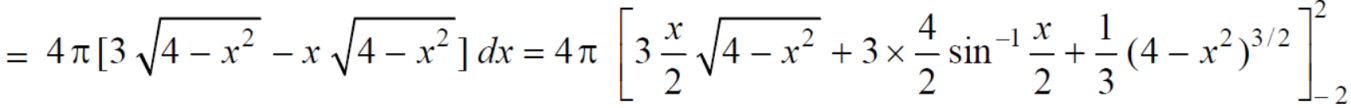
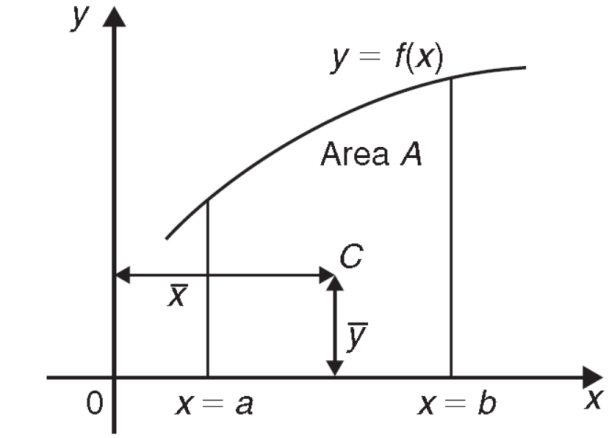
The centre of gravity of a lamina is the point where it balances perfectly which means the lamina’s centre of mass.
If 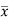 and
and 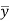 are the coordinates of the centroid C of area A, then-
are the coordinates of the centroid C of area A, then-
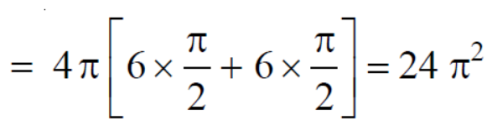
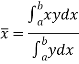
And
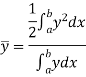
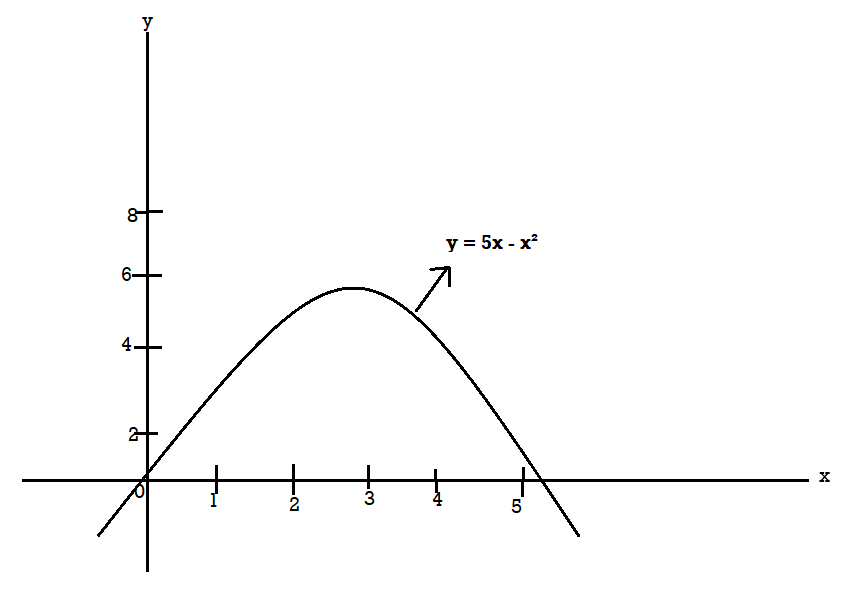
Example: Determine the coordinates of the centroid of the area lying between the curve y = 5x - x² and the x-axis.
Sol.
Here y = 5x - x²when y = 0, x = 0 or x = 5
Therefore, the curve cuts the x-axis at 0 and 5 as in the figure-
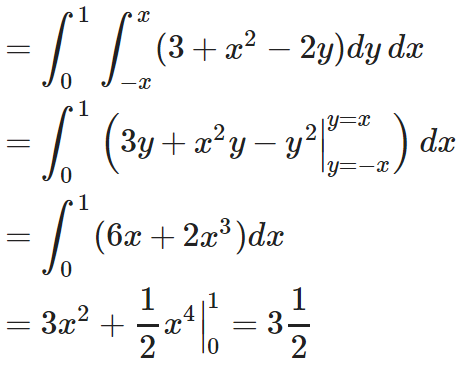
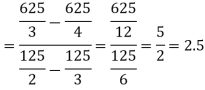
Now
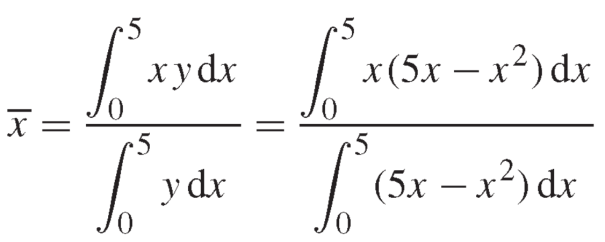
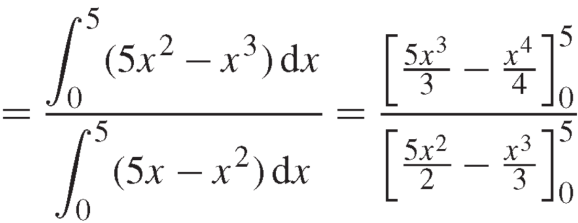
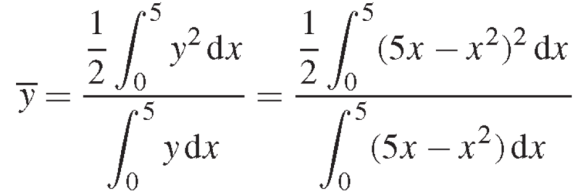
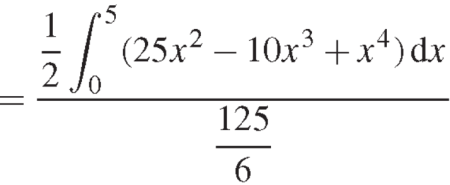
= 2.5
Therefore, the centroid of the area lies at (2.5, 2.5)
Green’s theorem in a plane
If C be a regular closed curve in the xy-plane and S is the region bounded by C then,
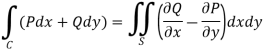
Where P and Q are the continuously differentiable functions inside and on C.
Green’s theorem in vector form-
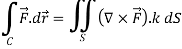
Example-1: Apply Green’s theorem to evaluate 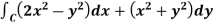 where C is the boundary of the area enclosed by the x-axis and the upper half of circle
where C is the boundary of the area enclosed by the x-axis and the upper half of circle 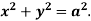
Sol. We know that by Green’s theorem-
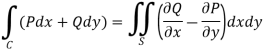
And it is given that-
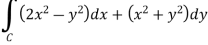
Now comparing the given integral-
P = 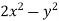 and Q =
and Q = 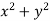
Now-
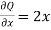 and
and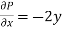
So that by Green’s theorem, we have the following integral-
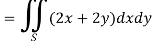
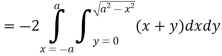
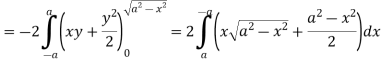
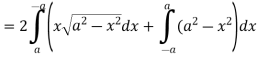
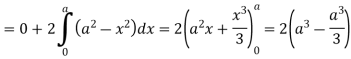
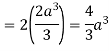
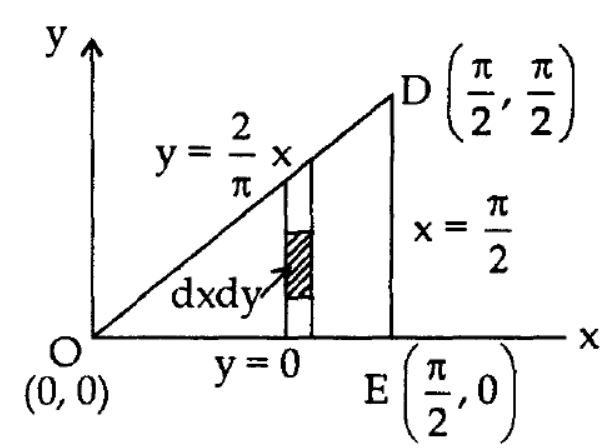
Example-2: Evaluate 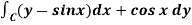 by using Green’s theorem, where C is a triangle formed by
by using Green’s theorem, where C is a triangle formed by 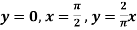
Sol. First we will draw the figure-
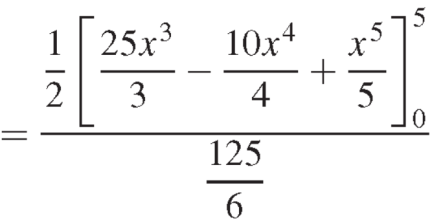
Here the vertices of triangle OED are (0,0), (
Now by using Green’s theorem-
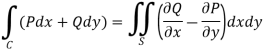
Here P = y – sinx, and Q =cosx
So that-
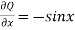 and
and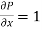
Now-
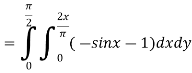
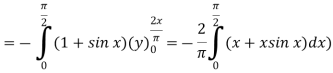
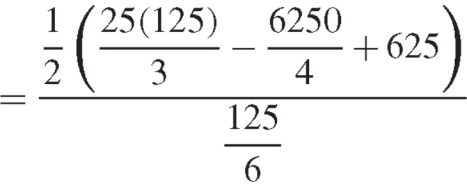
= 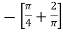
Which is the required answer.
Example-3: Verify green’s theorem in xy-plane for 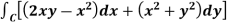 where C is the boundary of the region enclosed by
where C is the boundary of the region enclosed by 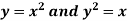
Sol.
On comparing with green’s theorem,
We get-
P = 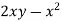 and Q =
and Q = 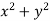
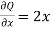 and
and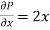
By using Green’s theorem-
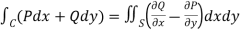 ………….. (1)
………….. (1)
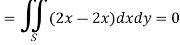
And left-hand side= 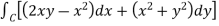
 ………….. (2)
………….. (2)
Now,
Along 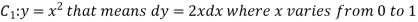
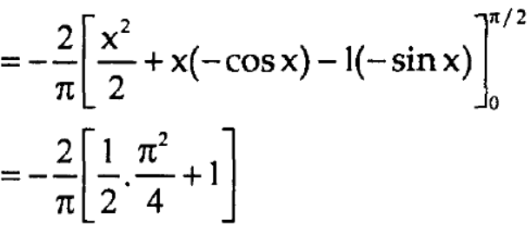
Along 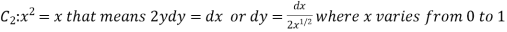
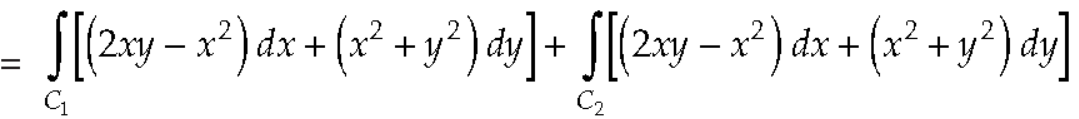
Put these values in (2), we get-
L.H.S. = 1 – 1 = 0
So that the Green’s theorem is verified.
Stoke’s theorem (without proofs) and their verification-
If 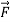 is any continuously differentiable vector point function and S is a surface bounded by a curve C, then-
is any continuously differentiable vector point function and S is a surface bounded by a curve C, then-
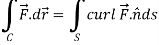
Example-1: Verify stoke’s theorem when 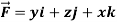 and surface S is the part of sphere
and surface S is the part of sphere 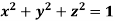 , above the xy-plane.
, above the xy-plane.
Sol.
We know that by stoke’s theorem,
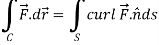
Here C is the unit circle- 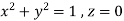
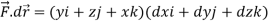
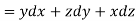
So that-
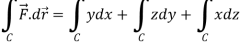
Now again on the unit circle C, z = 0
dz = 0
Suppose, 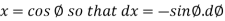
And 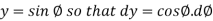
Now
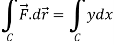
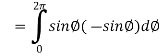
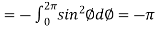 ……………… (1)
……………… (1)
Now-
Curl 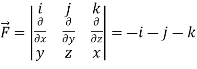
Using spherical polar coordinates-

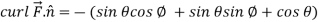
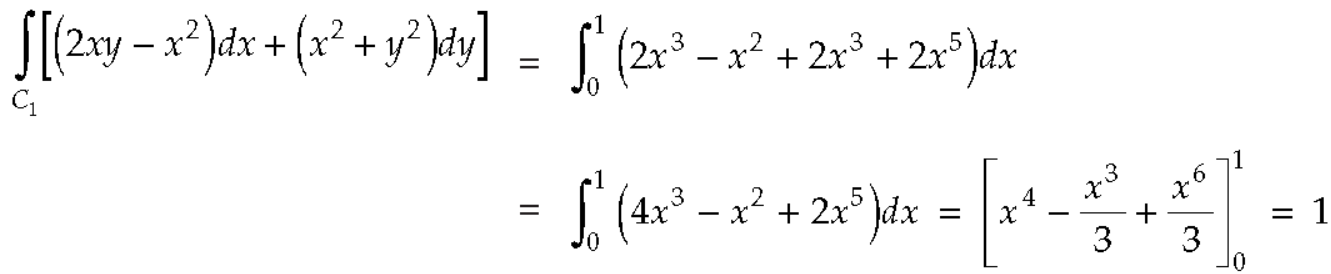
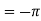 ………………… (2)
………………… (2)
From equation (1) and (2), stoke’s theorem is verified.
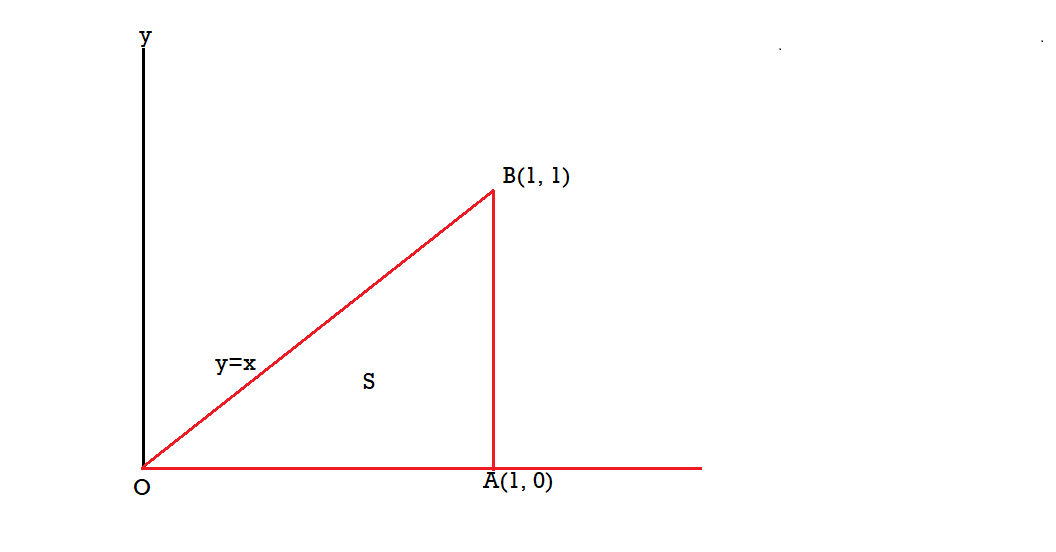
Example-2: If 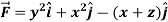 and C is the boundary of the triangle with vertices at (0, 0, 0), (1, 0, 0) and (1, 1, 0), then evaluate
and C is the boundary of the triangle with vertices at (0, 0, 0), (1, 0, 0) and (1, 1, 0), then evaluate 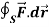 by using Stoke’s theorem.
by using Stoke’s theorem.
Sol. here we see that z-coordinates of each vertex of the triangle is zero, so that the triangle lies in the xy-plane and 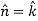
Now,
Curl 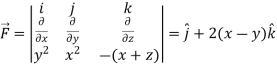
Curl 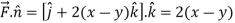
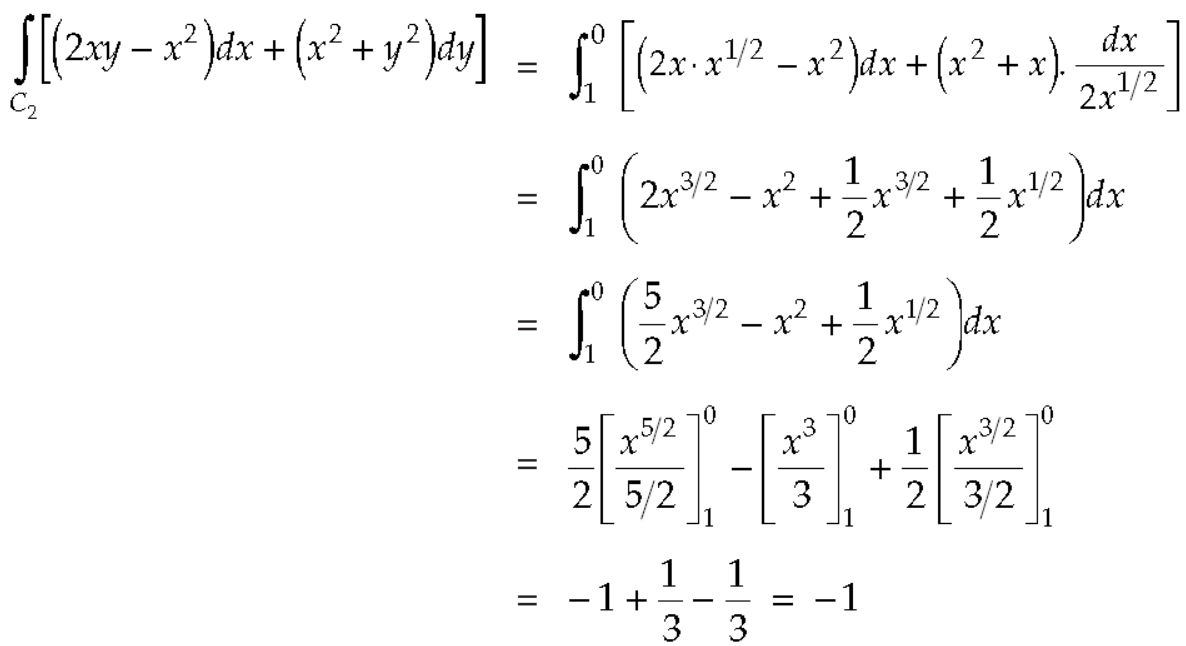
The equation of the line OB is y = x
Now by stoke’s theorem,
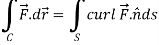

Example-3: Verify Stoke’s theorem for the given function-
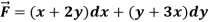
Where C is the unit circle in the xy-plane.
Sol. Suppose-
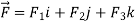
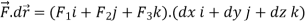
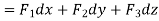
Here 
We know that unit circle in xy-plane-
Or
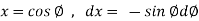
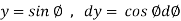
So that,
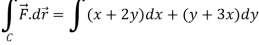
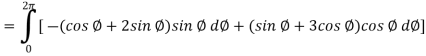
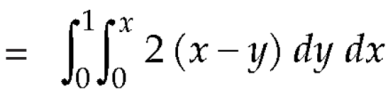
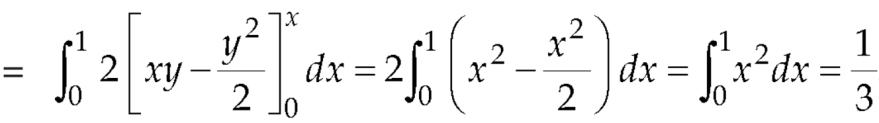
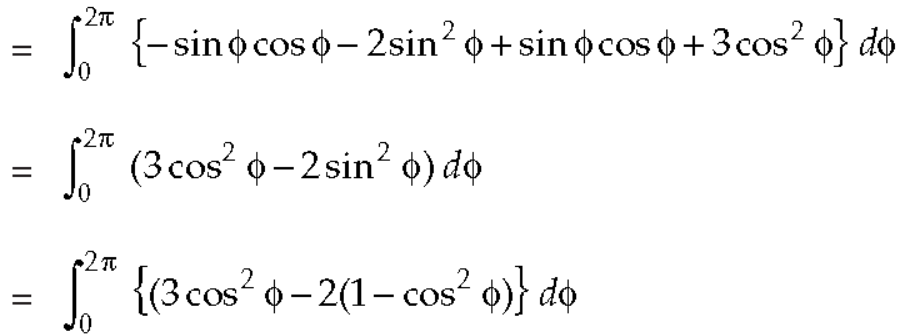
Now
Curl 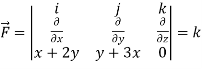
Now,
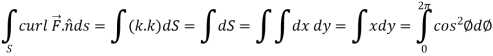
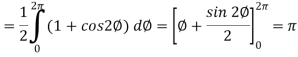
Hence the Stoke’s theorem is verified.
Gauss divergence theorem
If V is the volume bounded by a closed surface S and 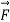 is a vector point function with continuous derivative-
is a vector point function with continuous derivative-
Then it can be written as-
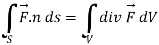
Where 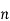 unit vector to the surface S.
unit vector to the surface S.
Example-1: Prove the following by using Gauss divergence theorem-
1. 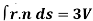
2. 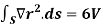
Where S is any closed surface having volume V and 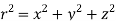
Sol. Here we have by Gauss divergence theorem-
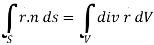
Where V is the volume enclose by the surface S.
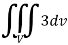
We know that-

= 3V
2.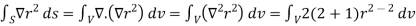
Because 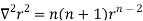
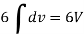
Example – 2 Show that 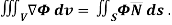
Sol
By divergence theorem, 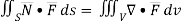 ...… (1)
...… (1)
Comparing this with the given problem let
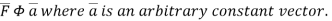
Hence, by (1)
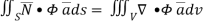 …………. (2)
…………. (2)
Now,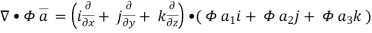
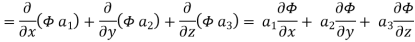
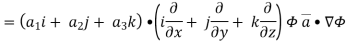
Hence, from (2), We get,
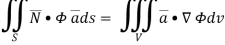
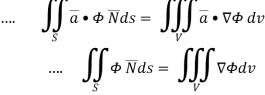
Example Based on Gauss Divergence Theorem
1. Show that
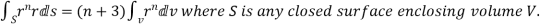
Soln. We have Gauss Divergence Theorem
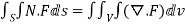
By data, F=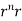

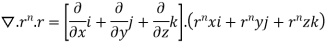
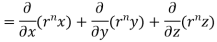
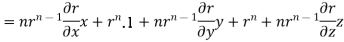
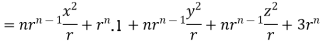
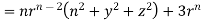
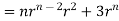
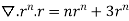
=(n+3)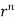

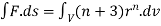
2 Prove that 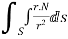 =
=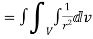
Soln. By Gauss Divergence Theorem,
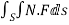 =
=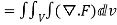
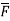 =
=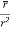 =
=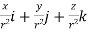
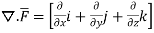 .[
.[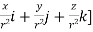
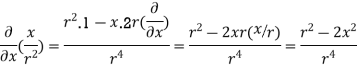
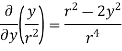

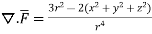 =
= 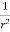
Suppose the rectangular coordinates (x, y, z) of any point be expressed as functions of u, v, w so that-
X = x (u, v, w), y = y (u, v, w), z = z (u, v, w) ……… (1)
Suppose that (1) can be solved for u, v, w in terms of x, y, z, so that-
U = u (x, y, z), v = v (x, y, z), w = w (x, y, z) ………. (2)
Here we assume that the functions in (1) and (2) are single-valued and continuous partial derivative so that the correspondence between (x, y, z) and (u, v, w) is unique, then (u, v, w) are called curvilinear coordinates of (x, y, z).
Each u, v, w, has a level surface through an arbitrary point. The surface u = 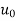 , v =
, v = 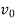 ,w =
,w = 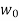 are called coordinate surfaces through P (
are called coordinate surfaces through P (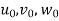 )
)
Each pair of these coordinate surfaces intersect in curves called the coordinate curves.
The curve of intersection of 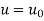 and
and 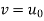 will be called the w-curve, for only w changes along this curve,
will be called the w-curve, for only w changes along this curve,
Similarly, u and v-curves are defined.
In vector notation, equation (1) can be written as-
R = x (u, v, w) I +y (u, v, w) J+z (u, v, w) K
So that-
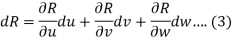
Then 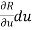 is a tangent vector to the u-curve at P. if
is a tangent vector to the u-curve at P. if 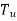 is a unit vector at P in this direction then
is a unit vector at P in this direction then 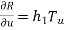 where
where 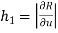
Same as if 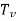 and
and 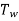 be unit tangent vectors to v and u-curves at P, then-
be unit tangent vectors to v and u-curves at P, then-
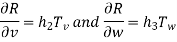
Where 
Equation (3) can be written as-
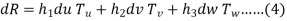
Since  is normal to the surface u =
is normal to the surface u = 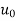 at P, so that a unit vector in this direction is given by
at P, so that a unit vector in this direction is given by
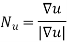
And
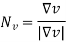
And
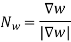
Thus, at each point P of a curvilinear coordinate system there exists two triads of unit vector-
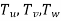 tangents to u, v, w-curves and
tangents to u, v, w-curves and 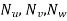 normals to the coordinate surfaces.
normals to the coordinate surfaces.
In particular, when the coordinate surfaces intersect at right angles, the three coordinate curves are also mutually orthogonal and u, v, w are called the orthogonal curvilinear coordinates.
Multiplying equation (3) scalarly by 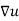 , we get-
, we get-
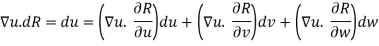
Whence-
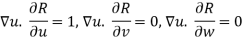
Similarly-
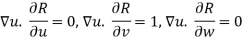
And
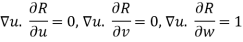
This relation shows that the sets 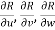 and
and 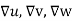 constitute reciprocal system of vectors.
constitute reciprocal system of vectors.
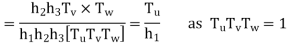
Example: Evaluate-
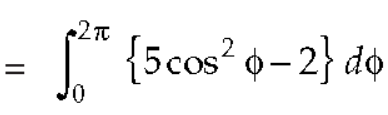
Where
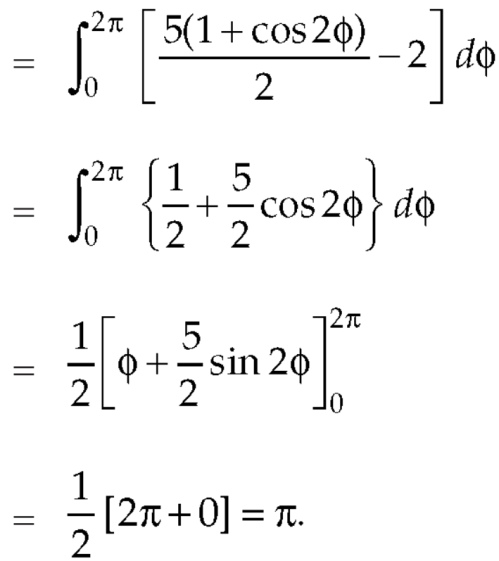
And S is the surface of the cube bounded by x = 0, x = 1, y = 1, z = 0, z = 1.
Sol.
By the Divergence theorem-
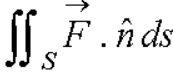
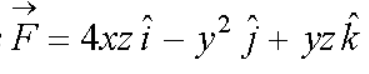

Example: By using divergence theorem to evaluate-
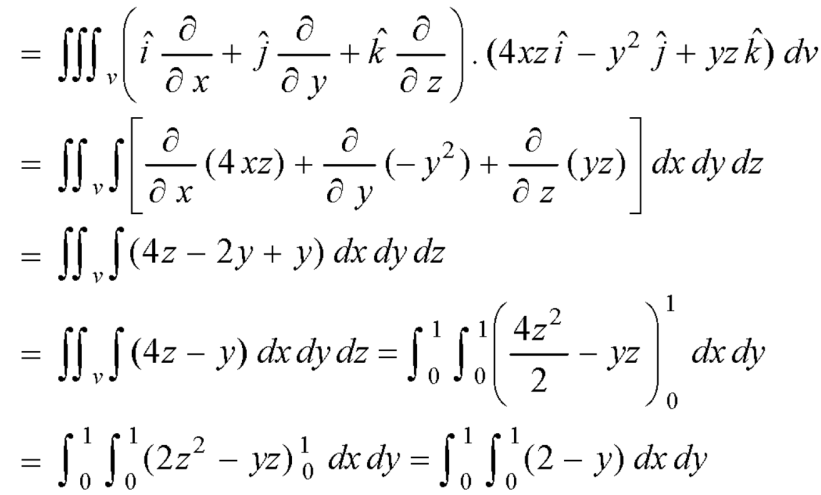
Where-
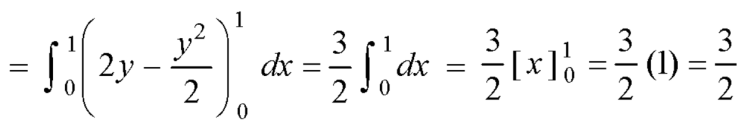
And S is the surface of the sphere 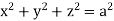 .
.
Sol.
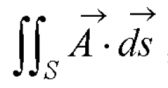
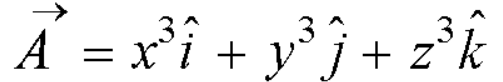
On putting x = 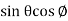 , y =
, y = 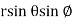 , z =
, z =  , we get-
, we get-
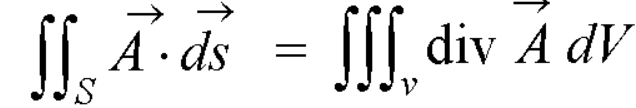
Example: Use the divergence theorem to evaluate-
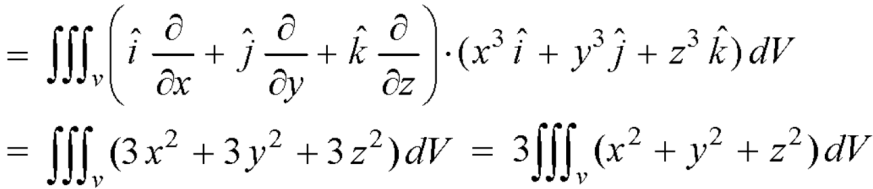
Over the surface of a sphere of radius ‘a’.
Sol.
Here we have-
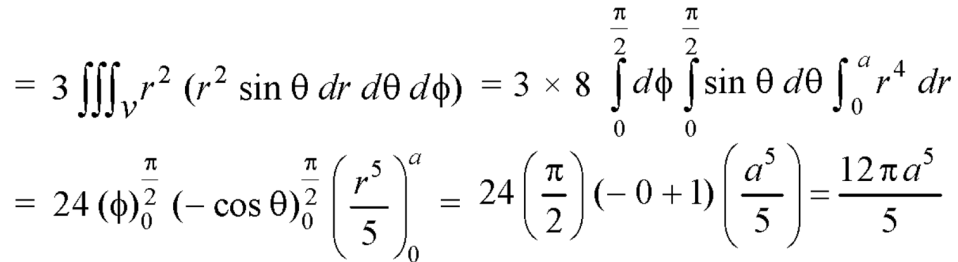
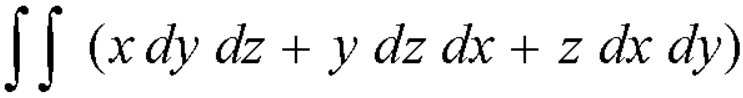

= 3 × Volume of the sphere
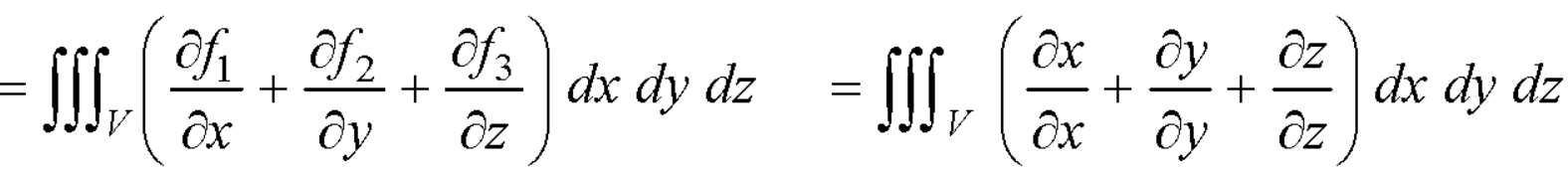
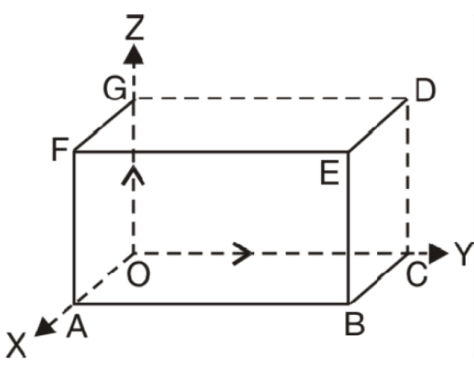
Example: Verify the Gauss divergence theorem for-
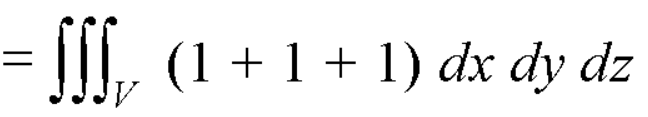
Taken over the rectangular parallelopiped
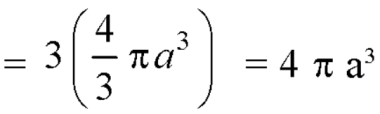
Sol.
We have-
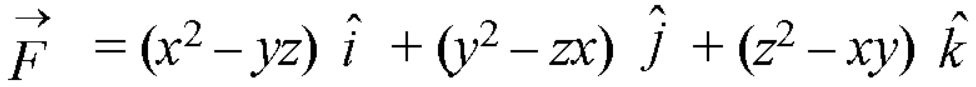
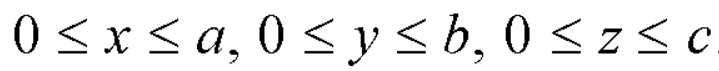
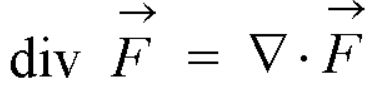
Volume integral-
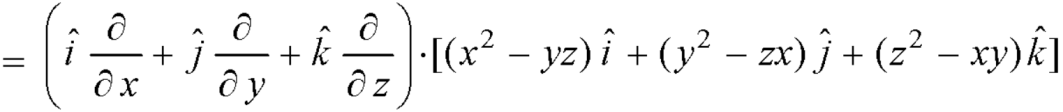
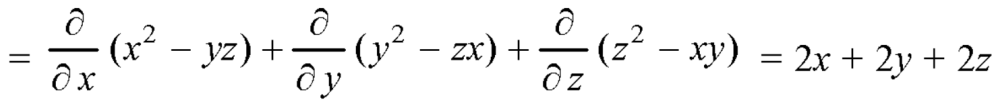
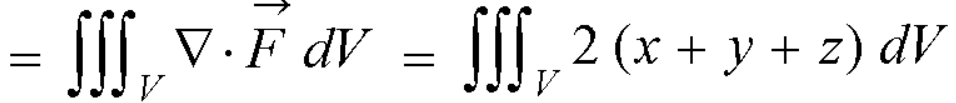
To evaluate 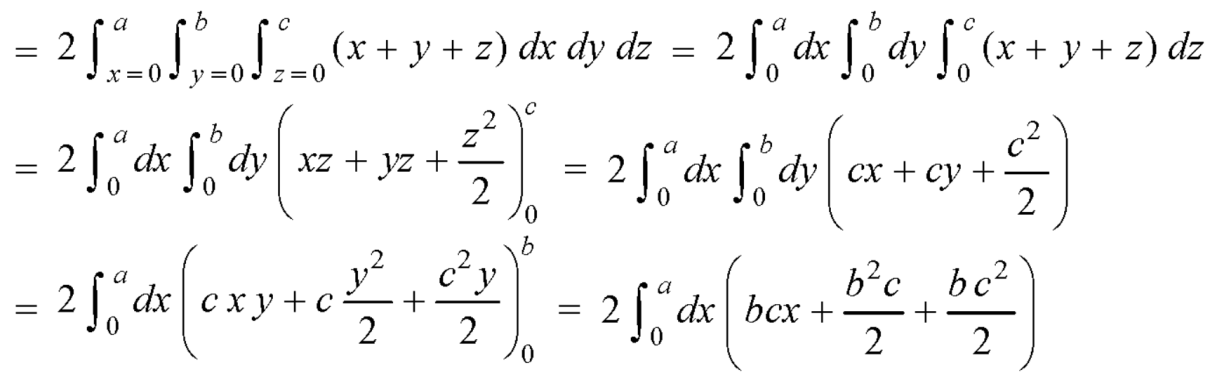 , where S consists of six plane surfaces-
, where S consists of six plane surfaces-
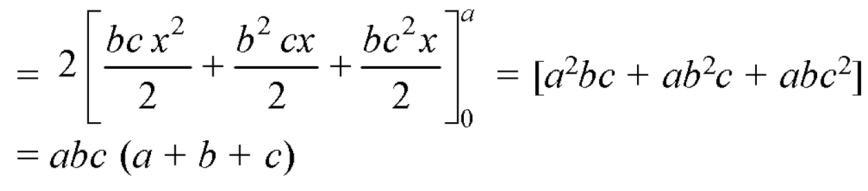

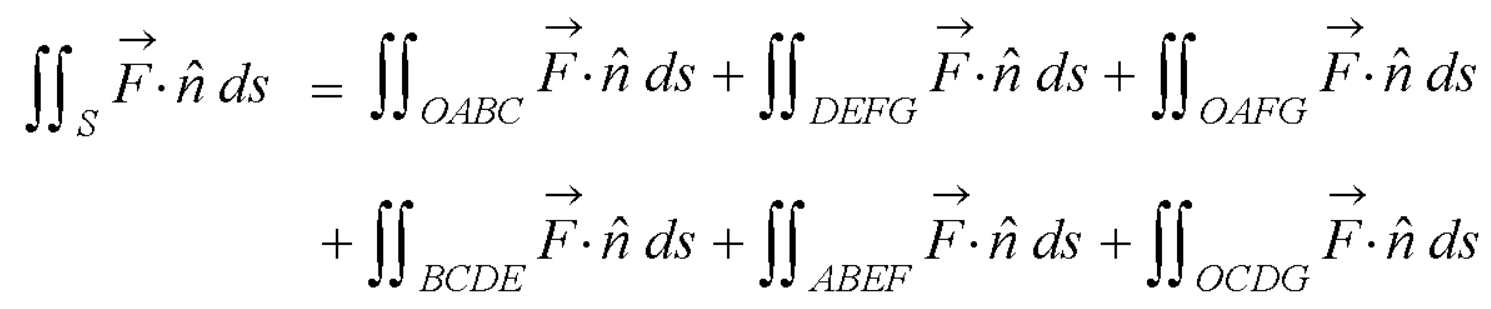
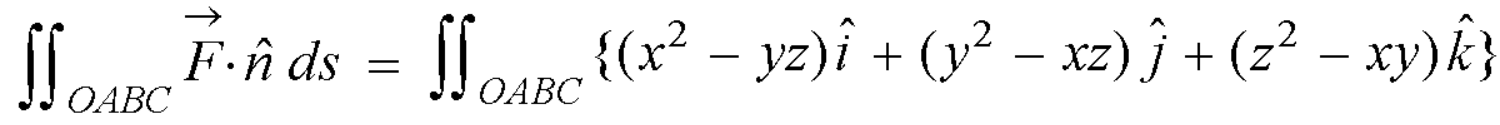
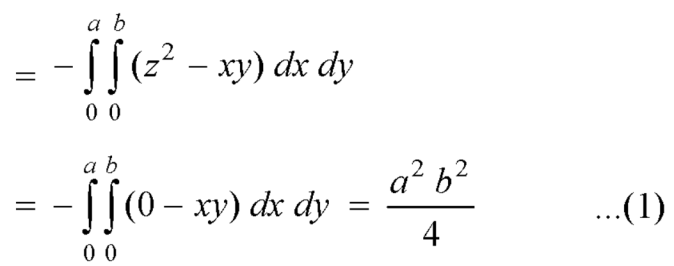
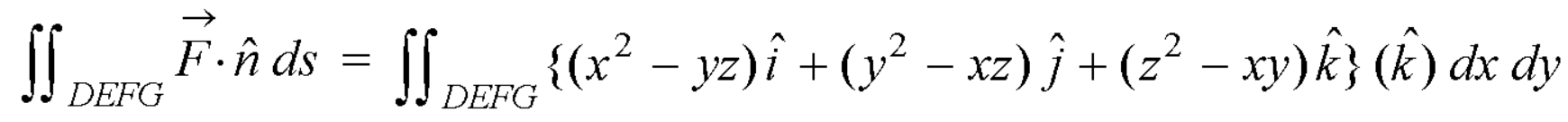
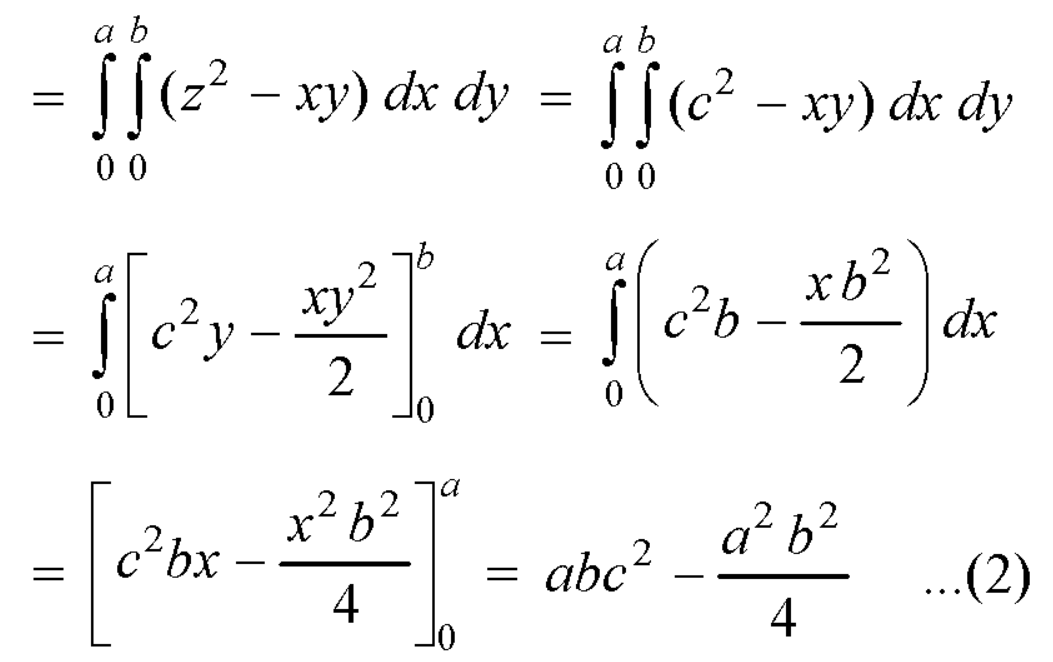
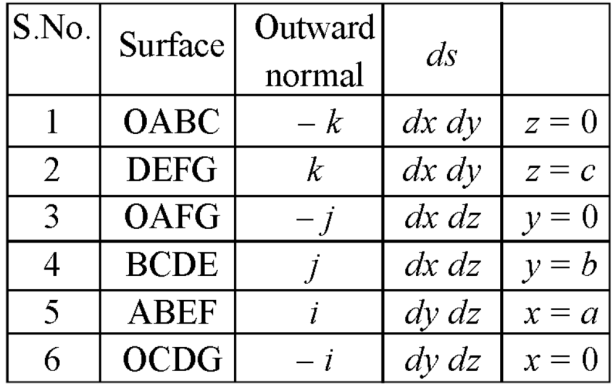 ….. (3)
….. (3)
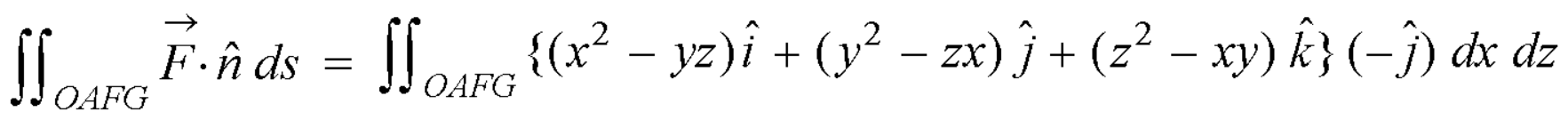
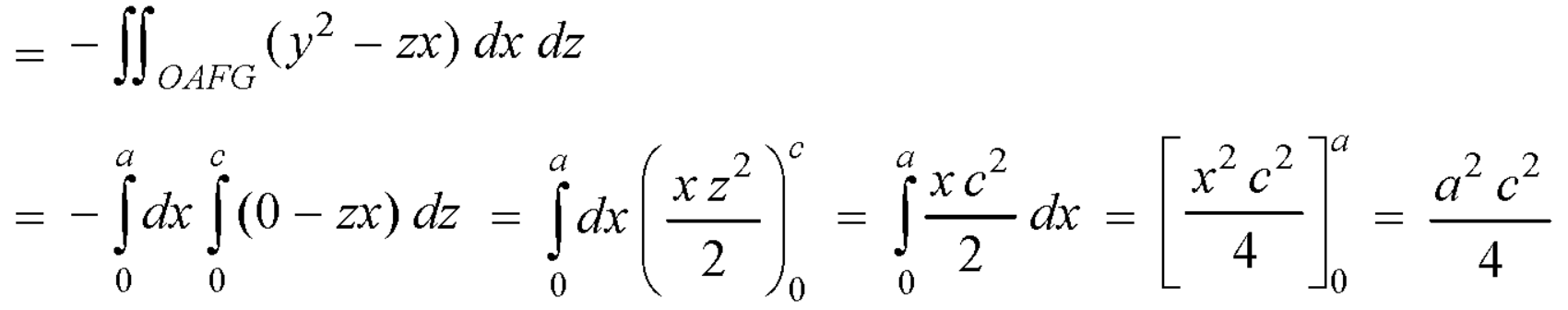
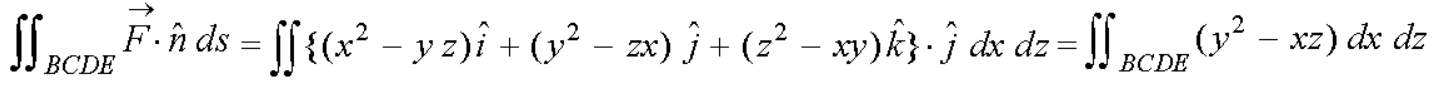
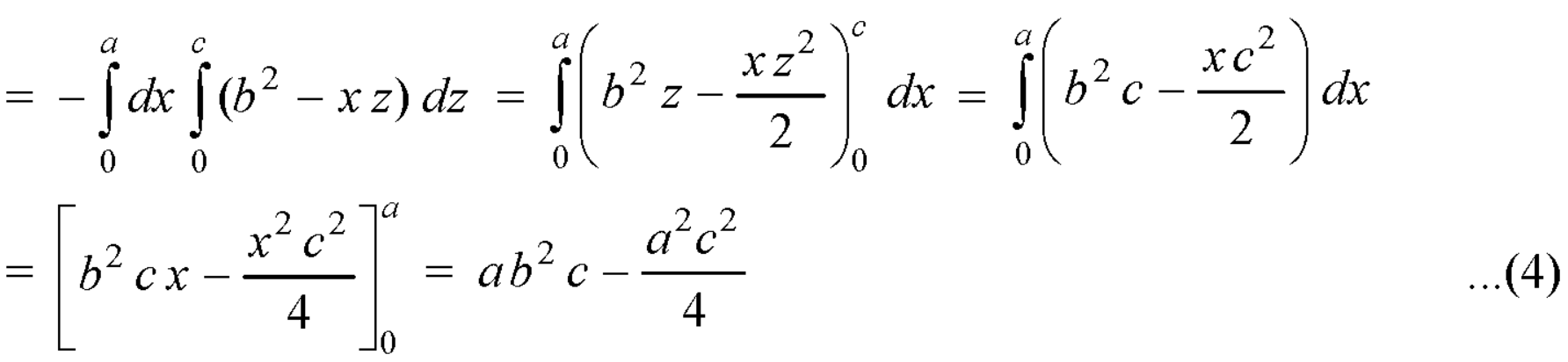
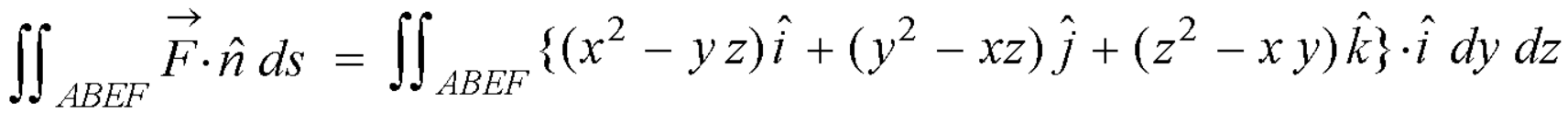
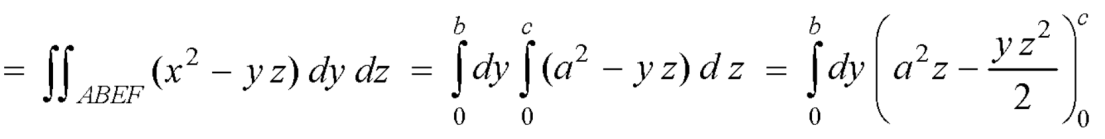
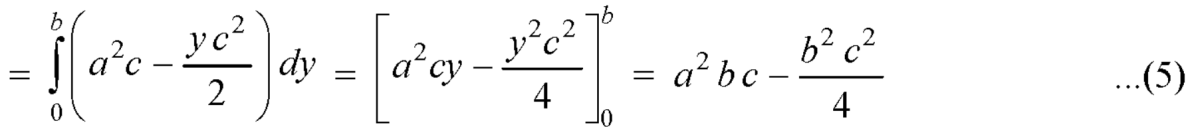
Add 1,2,3,4,5 and 6, we get-
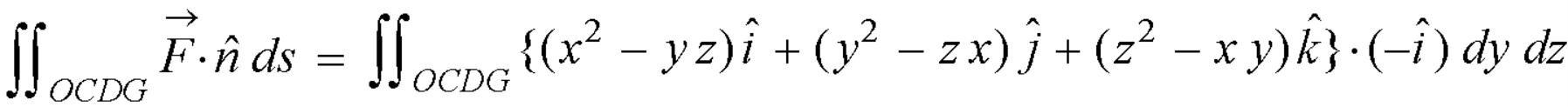
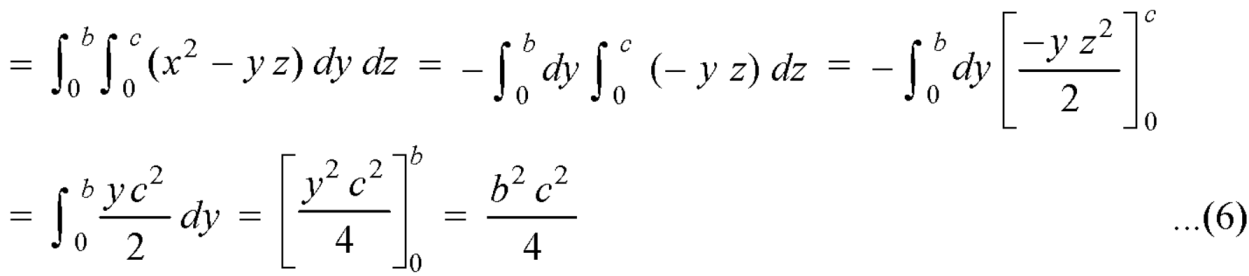
The Gauss divergence theorem is varified.
References:
1. G.B. Thomas and R.L. Finney, “Calculus and Analytic Geometry”, Pearson, 2002.
2. T. Veerarajan, “Engineering Mathematics”, McGraw-Hill, New Delhi, 2008.
3. B. V. Ramana, “Higher Engineering Mathematics”, McGraw Hill, New Delhi, 2010.
4. N.P. Bali and M. Goyal, “A Text Book of Engineering Mathematics”, Laxmi Publications, 2010.
5. B.S. Grewal, “Higher Engineering Mathematics”, Khanna Publishers, 2000.