Unit - 7
Partial differential equations: First Order
A partial differential equation (PDE) is an equation involving one or more partial derivatives of an (unknown) function, call it u, that depends on two or more variables, often time t and one or several variables in space. The order of the highest derivative is called the order of the PDE. Just as was the case for ODEs, second-order PDEs will be the most important ones in applications.
Just as for ordinary differential equations (ODEs) we say that a PDE is linear if it is of the first degree in the unknown function u and its partial derivatives. Otherwise, we call it nonlinear.
The standard methods of solving the differential equations of the following
Types:
(i) Equations solvable by separation of the variables.
(ii) Homogeneous equations.
(iii) Linear equations of the first order.
(iv) Exact differential equations.
The differential equation of first order and first degree is namely:
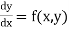
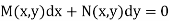
Example 1. Solve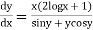 
Solution. We have,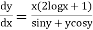 
Separating the variables, we get
(sin y + y cos y) dy = {x (2 log x +1} dx
Integrating both the sides we get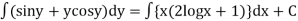 
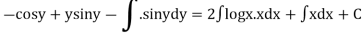
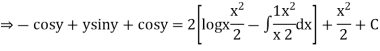
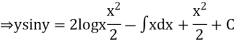
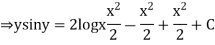
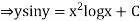
Example 2. Solve the differential equation
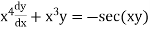
Solution.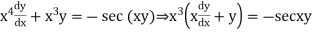 
Put,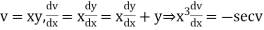 
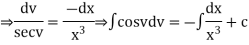
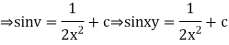
Solutions of first order linear PDEs
Linear Equations of the First Order
A linear partial differential equation of the first order, commonly known as Lagrange’s Linear equation is of the for4m
Pp + Qq = R (1)
Where, P, Q and R are functions of x, y, z. This equation is called a quast linear equation. When P, Q and R are independent of z it is known as linear equation.
Such asn equation is obtained by eliminating an arbitrary function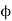 
from 
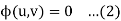
where u,v are are some functions of x, y, z.
Differentiating (2) partially with respect to x and y
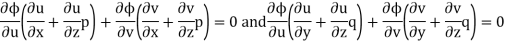
Eliminating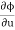 
and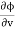 
, we get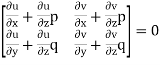 
which simplifies to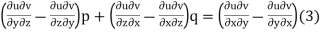 
This is of the same form as (1)
Now suppose u = a and v=b, where a, b are constants, so that
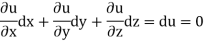
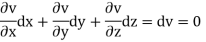
By cross multiplication we have,
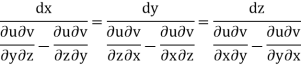
The solution of these equations are u = a and v = b
Therefore,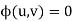 
is the required solution of (1).
Thus, to solve the equation Pp + Qq =R.
(i) Form the subsidiary equations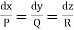 
(ii) Solve these simultaneous equations
(iii) Write the complete solution as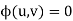 
or u=f(v)
Example. Solve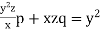 
Solution. Rewriting the given equation as
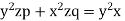
The subsidiary equations are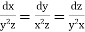 
The first two fractions give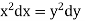 
Integrating we get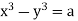 
n (i)
Again, the first and third fraction give xdx = zdz
Integrating, we get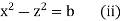 
Hence from (i) and (ii), the complete solution is
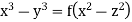
Example. Solve 
Solution. Here the subsidiary equations are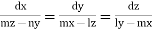 
Using multipliers x, y and z we get each fraction =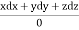 
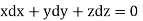which on integration gives 
Again, using multipliers l, m and n we get each fraction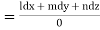 
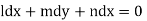which on integration gives lx +my +nz = b (ii)
Hence from (i) and (ii) the required solution is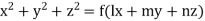 
Example. Solve 
Solution. Here the subsidiary equations are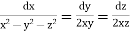 
From the last two fractions, we have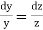 
Which on integration gives log y = log z + log a or y/z=a (i)
Using multipliers x, y and z we have
Each fraction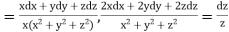 
which on integration gives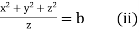 
Hence from (i) and (ii) the required solution is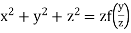 
Lagrange’s linear equation in an equation of the type-
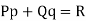
Here P, Q and R are the functions of x, y, z and p =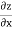 
and q =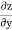 
Working steps to solve-
Step-1: Write down the auxiliary equation-
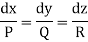
Step-2: Solve the auxiliary equations-
Suppose the two solutions are- u = 
and v =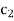 
Step-3: Then f (u, v) = 0 or u = ∅(v) is the required solution of
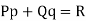
Method of multipliers-
Let the auxiliary equation be
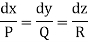
L, m, n may be the constants of x, y, z then we have-
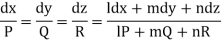
L, m, n are selected in a such a way that-
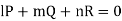
Thus
On solving this differential equation, if the solution is- u =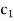 
Similarly, choose another set of multipliers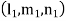 
and if the second solution is v =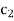 
So that the required solution is f (u, v) = 0.
Example: Solve-
Sol.
We have-
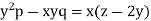
Then the auxiliary equations are-
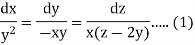
Consider first two equations only-
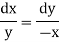
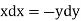
On integrating
…….. (2)
Now consider last two equations-
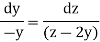
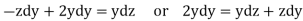
On integrating we get-
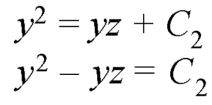…………… (3)
From equation (2) and (3)-
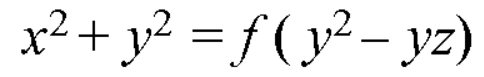
Example: Find the general solution of-
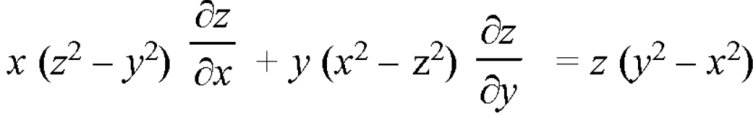
Sol. The auxiliary simultaneous equations are-
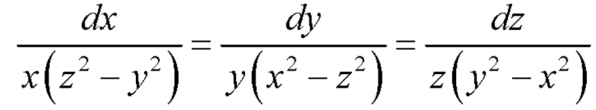……….. (1)
Using multipliers x, y, z we get-
Each term of (1) is equals to-
Xdx + ydy + zdz=0
On integrating-
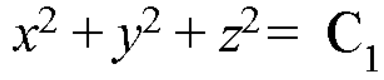………… (2)
Again equation (1) can be written as-
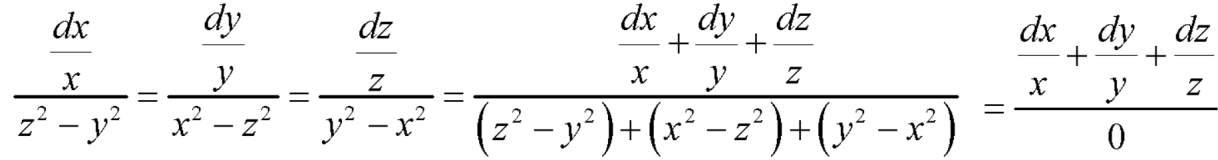
Or
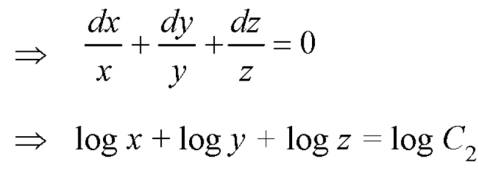
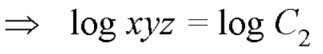
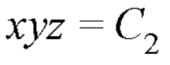………….. (3)
From (2) and (3), the general solution is-
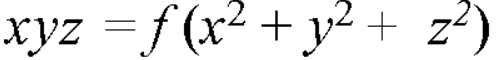
Non-linear partial differential equations-
Type-1: Equation of the type f (p, q) = 0
Method-
Let the required solution is-
Z = ax + by + c ……... (1)
So that-
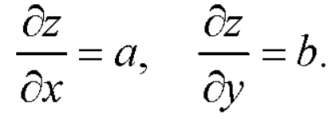
On putting these values in f (p, q) = 0
We get-
f (a, b) = 0
So, from this, find the value of b in terms of a and put the value of b in (1). It will be the required solution.
Type-2: Equation of the type-
Z = px + qy + f (p, q)
Its solution will be-
Z = ax + by + f (a, b)
Type-3: Equation of the type f (z, p, q) = 0
Type-4: Equation of the type-
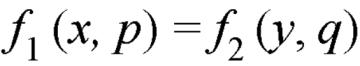
Method-
Let-
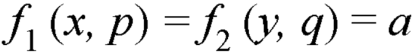
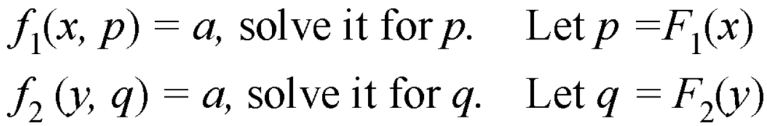
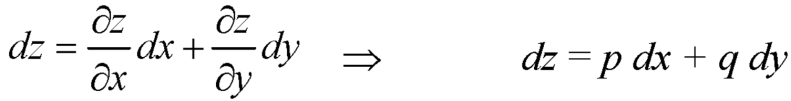
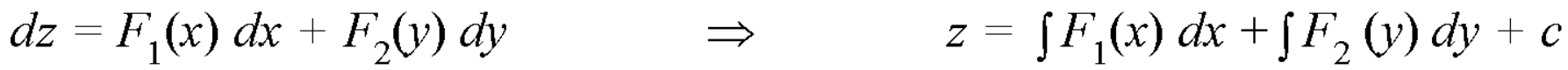
Example: Solve-
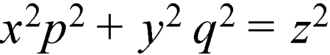
Sol.
This equation can be transformed as-
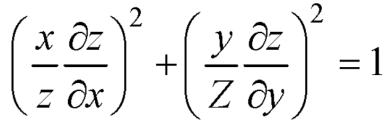
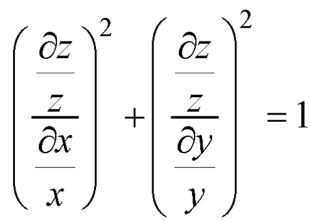………. (1)
Let
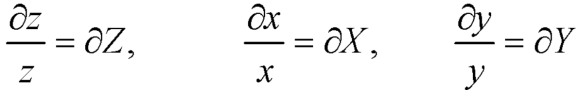
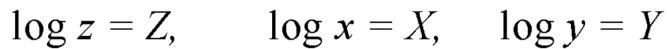
Equation (1) can be written as-
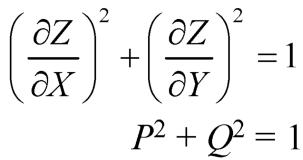………… (2)
Let the required solution be-
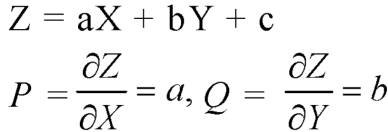
From (2) we have-
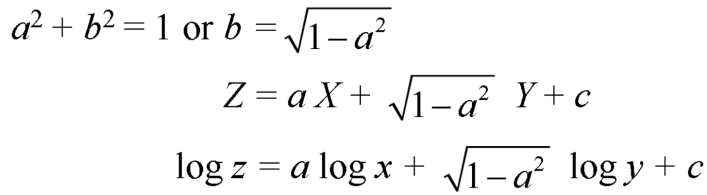
Example: Solve-
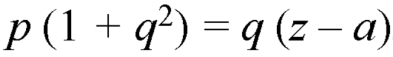
Sol.
Let u = x + by
So that-
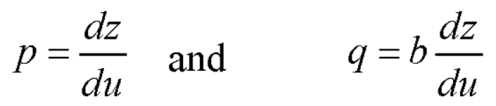
Put these values of p and q in the given equation, we get-
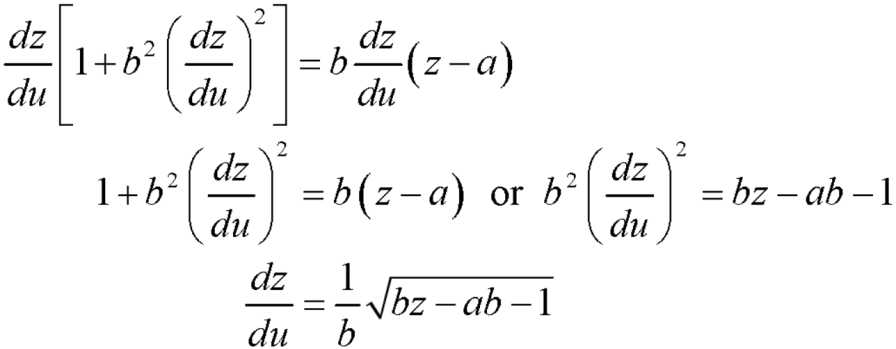
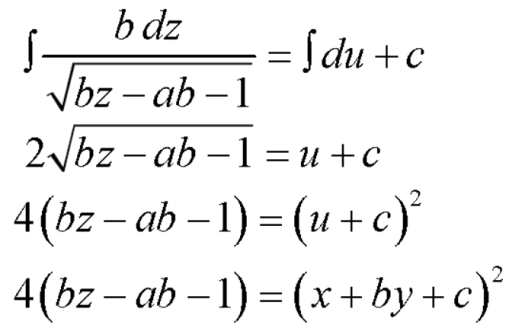
Example: Solve-
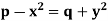
Sol.
Let-
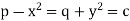
That means-
Put these values of p and q in
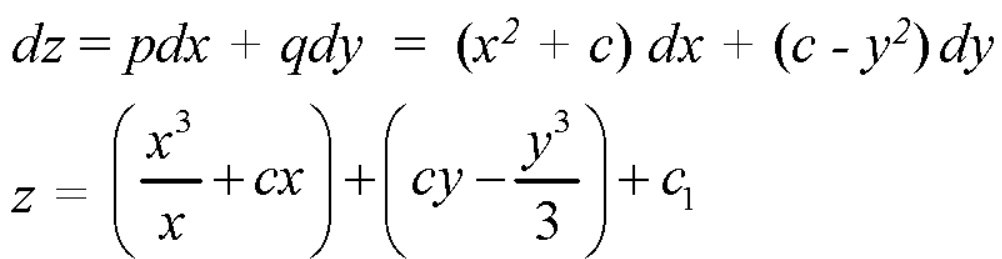
References:
1. G.B. Thomas and R.L. Finney, “Calculus and Analytic Geometry”, Pearson, 2002.
2. T. Veerarajan, “Engineering Mathematics”, McGraw-Hill, New Delhi, 2008.
3. B. V. Ramana, “Higher Engineering Mathematics”, McGraw Hill, New Delhi, 2010.
4. N.P. Bali and M. Goyal, “A Text Book of Engineering Mathematics”, Laxmi Publications, 2010.
5. B.S. Grewal, “Higher Engineering Mathematics”, Khanna Publishers, 2000.
