Module 01

Atomic and molecular structure

1.1             in a box solutions and their applications for conjugated molecules and nano particles:

The Schrodinger equation is a linear partial differential equation that describes the wave function or state function of a quantum-mechanical system. Schrodinger wave equation is a mathematical expression describing the energy and position of the electron in space and time, taking into account the matter wave nature of the electron inside an atom.

It is based on three considerations. They are;

• Classical plane wave equation,
• Broglie’s Hypothesis of matter-wave, and
• Conservation of Energy.

Classical Plane Wave Equation

A wave is a disturbance of a physical quantity undergoing simple harmonic motion or oscillations about its place. The disturbance gets passed on to its neighbors in a sinusoidal form.

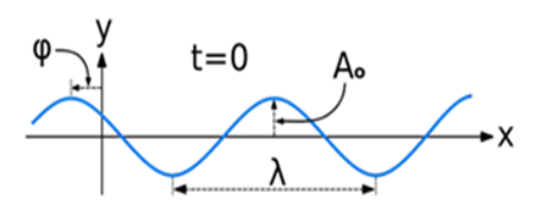
The equation for the wave is a second-order partial differential equation of a scalar variable in terms of one or more space variable and time variable. The one-dimensional wave equation is-

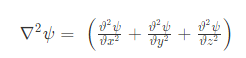
The amplitude (y) for example of a plane progressive sinusoidal wave is given by:

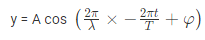
Where, A is the maximum amplitude, T is the period and φ is the phase difference of the wave if any and t is the time in seconds. For a standing wave-

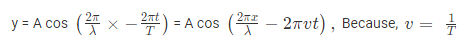
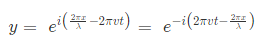
Importance of Schrodinger wave equation:

1. The electronic structure of atoms and molecules can be well explained using the Schrodinger’s equation
2. The solution of Schrodinger’s equation results in quantized properties of systems. E.g.: energy quantization. The quantization is a consequence of the periodic boundary conditions involved.
3. Solving the Schrodinger’s equation for a solid is basically a many-body problem and it involves the solution of complex differential equations. The problem can be solved by expressing the differential Schrodinger’s equation in momentum space/reciprocal space, where the Schrodinger’s equation can be expressed as a set of linear algebraic equations rather than complex differential equations. Those linear equations can be solved using available computational numerical techniques accurately to predict the ground state properties of solids
4. The solution of the Schrodinger’s equation in the reciprocal space gives the electronic band structure of solids.
5. Most of the semiconductor properties such as the origin of energy band gap, the behavior of dopants and their energy levels present in relation to the observed band structure etc., can be well studied by solving the Schrodinger’s equation.
6. Today it is possible to obtain a suitable dopant for a given semiconductor just by solving the corresponding Schrodinger’s equation, without conducting any experiments
7. The materials in practice are impure, they contain various impurities as well as crystalline defects. The ground state properties of these defects can be well studied by solving the Schrodinger’s equation

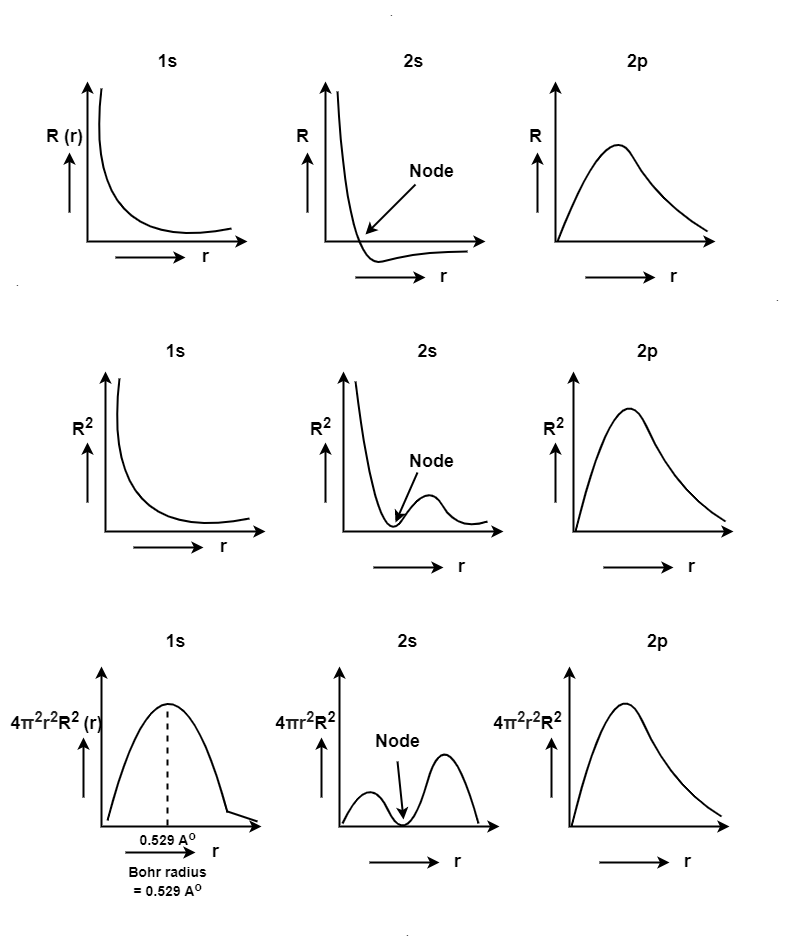
1.2             Form of hydrogen atom wave function and the plot of these functions to explore their spatial variations:

Electrons race around a central nucleus at such high speeds that they act like waves of energy. As electrons push each other around, the electron orbital clouds form different shapes. Scientists propose that atoms can have up to seven principle energy levels of electrons around the nucleus. This is the electron configuration for the element sulfur, which has 16 electrons.

We and everything around us are made of tiny groups of particles, called atoms. While atoms were once thought to be the smallest unit of matter, they are actually made up of much tinier, high-energy, negatively-charged particles circling at a crazy pace around a nucleus of positive and neutral particles. Since the outer-shell (valence) electrons control the chemical properties of a material, it would be useful to understand how they behave.

One popular theory is the wave-mechanical model, which proposes that electrons are almost as much like a wave of energy as they are like particles. They're moving so fast that they're not really in any one place at any given time, and they keep changing their path in response to the fields around them. In this lesson, we will discuss how scientists use the wave-mechanical model to describe the construction of an atom. The wave-mechanical model was proposed in the 1920s when scientists Erwin Schrödinger and Louis Victor de Broglie determined that the previous model (the Bohr model) was not useful for determining electron locations. The wave-mechanical theory proposes that each electron circling an atom's nucleus occupies a specific orbital and spins a certain direction, but the orbital is like a cloud or wave of energy, not the ring you might imagine thinking about the earth's orbit around the sun.

Developments Leading to Quantum Mechanical Model of Atom Dual Nature of Electron: Matter Waves In 1923 a young French physicist, Louis de Broglie, argued that if light can show a wave as well as a particle nature, why should particles of matter not possess wave like characteristics? He proposed that matter particles should indeed have a wave nature and said that a particle of mass m moving with a velocity v has an associated wavelength, some times called de Broglie wavelength given by the formula;

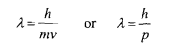
The de Broglie wavelength of a body is inversely proportional to its momentum mv. Since the magnitude of h is very small, the wavelength of the objects of our everyday world would be too small to be observed.

1.3             Molecular orbital of diatomic molecule and plots of the multicenter orbital&Equation for atomic and molecular orbital:

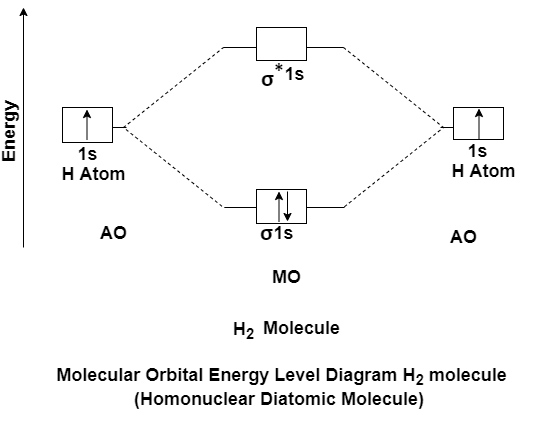
The molecular orbital theory of diatomic molecule can be considered as by taking the H2 molecule in consideration. The molecular orbital are made up of symmetric (both the hydrogen atom are stretched toward the same side) or asymmetric (both the hydrogen atom are stretched toward the opposite side) combination of the 2 atoms to their 1s atomic orbital. According to Molecular Orbital Theory the 1s orbital combine together to form the 2 new molecular orbital of the same Hydrogen molecule with one of it having a lower energy state than the 1s orbital. This MO is denoted by (Sigma1s and Sigma*1s). The molecular orbital with higher energy are called as bonding molecular orbital while the other orbital is anti-bonding molecular orbital.

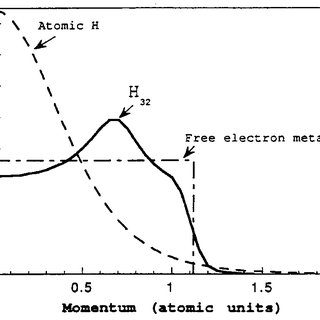
MO Energy level Diagram for Homonuclear Diatomic molecules:

H2 molecule

H2molecule consists of two H atoms and their two electrons. Two 1s orbitals give two MOs- one bonding that is σ and another one is antibonding that is σ*. The bonding orbital is in lower in energy state, the two electron occupies the bonding MO. The    MO electron configuration of H2 molecule is written as (σ1s) 2.

Energy evolved =

[No. Of electron in BMO (-) +  No. of electron in Anti Bonding Molecular Orbital  * (+)]

=2*(- ) + 0*(+ )

= -2 

This evolved energy is called as stabilization energy.

The molecule is stable so it is diamagnetic.

MO Energy level Diagram for Heteronuclear Diatomic molecules:

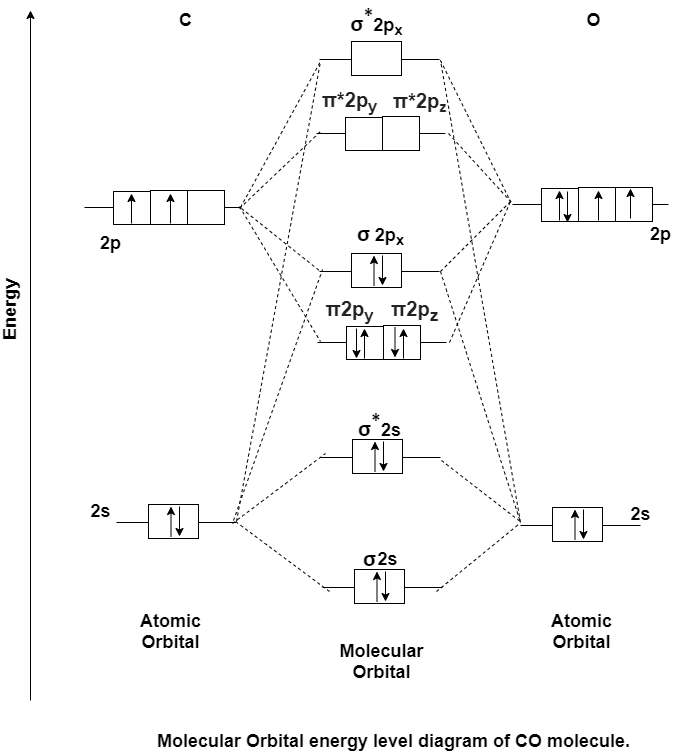
Carbon Monoxide Molecule (CO)

CO is the hetronuclear diatomic molecule. This molecule is formed by the combination of carbon and oxygen atom. Electronic configuration of carbon atom is 1s2, 2s2, 2p2 and that of oxygen is 1s2, 2s2, 2p4

Stabilisation Energy

[No. Of electron in BMO * (-) + No. Of electrons in anti bonding molecular orbital * (+)]

=(8) * (-) + (2) * (+)

= -6 

1.4             Energy level diagram of diatomic:

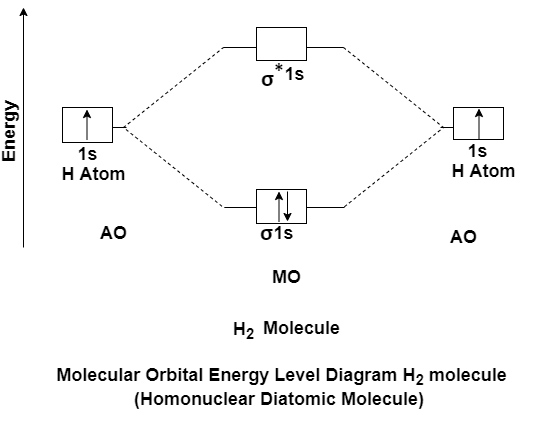
H2 molecule

H2molecule consists of two H atoms and their two electrons. Two 1s orbitals give two MOs- one bonding that is σ and another one is antibonding that is σ*. The bonding orbital is in lower in energy state, the two electron occupies the bonding MO. The    MO electron configuration of H2 molecule is written as (σ1s) 2.

Energy evolved =

[No. Of electron in BMO (-) +  No. of electron in Anti Bonding Molecular Orbital  * (+)]

=2*(- ) + 0*(+ )

= -2 

This evolved energy is called as stabilization energy.

The molecule is stable so it is diamagnetic.

MO Energy level Diagram for Heteronuclear Diatomic molecules:

Carbon Monoxide Molecule (CO)

CO is the hetronuclear diatomic molecule. This molecule is formed by the combination of carbon and oxygen atom. Electronic configuration of carbon atom is 1s2, 2s2, 2p2 and that of oxygen is 1s2, 2s2, 2p4

1.5             Pi-molecular orbital of butadiene and benzene and aromaticity:

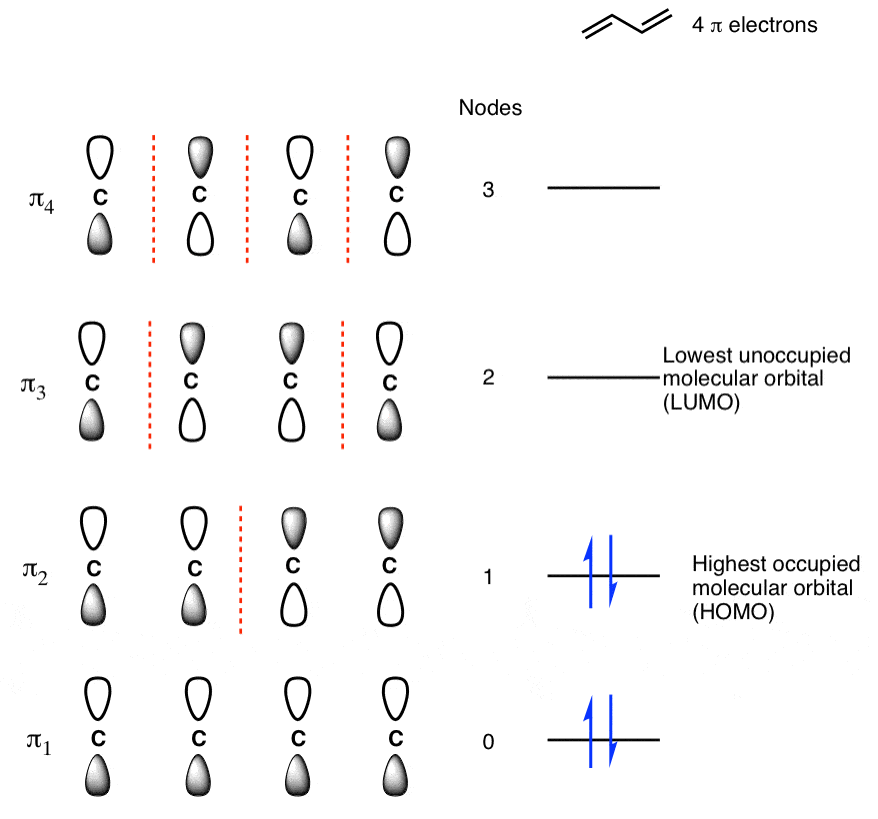
The Butadiene Pi System has zero nodes at its lowest energy molecular orbital.

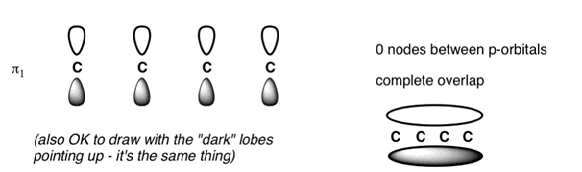
The Butadiene Pi System has three nodes at its lowest energy molecular orbital. The drawn n-p orbital are at alternate phase to each other. This create the a pi system with three nodes.

The Butadiene second lowest energy molecular orbital has one node.

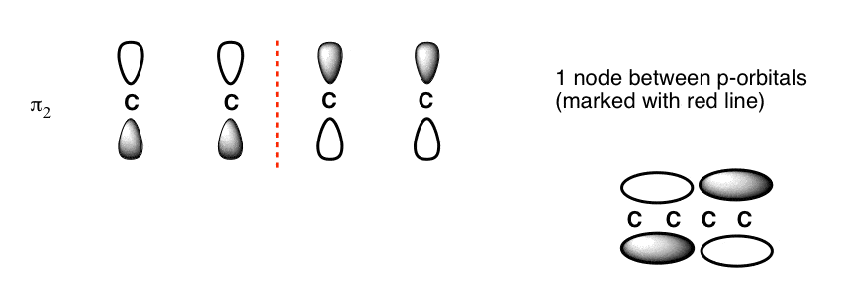
The third lowest energy molecular orbital has two nodes.

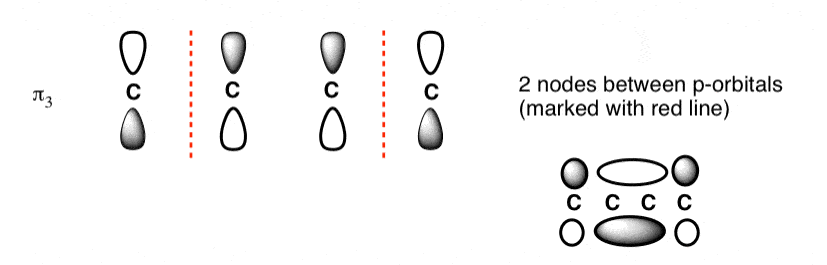
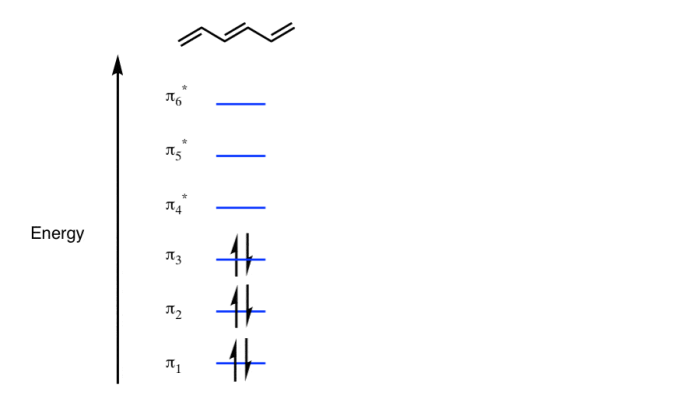
The Full Molecular Orbital Diagram For The Butadienyl System.

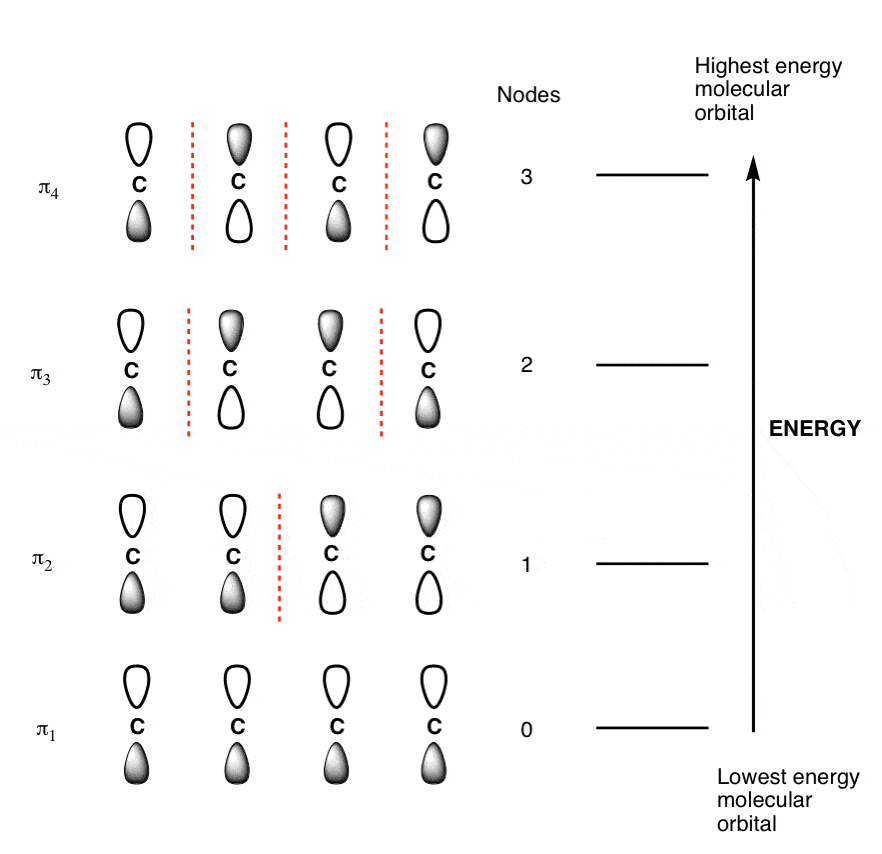
Populating The Molecular Orbitals Of Butadiene With Electrons

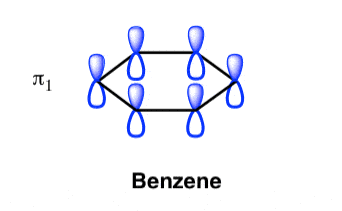
π molecular orbital of benzene:

The Pi molecular orbital diagram for Benzene

In the above increasing energy level the bottom three orbital are all bonding orbital while the top 3 orbital are anti bonding orbital.

The Benzene System has zero nodes at its lowest energy molecular orbital.

• All p orbital are aligned with phases pointing in the same direction.
• Nodes are absent between orbital.
• In this orbital, electrons are delocalized over the length of molecule, resulting in greatest lowering of energy.

Benzene Has Nodal Planes. The Maximum Energy Level Has 3 Nodal Planes

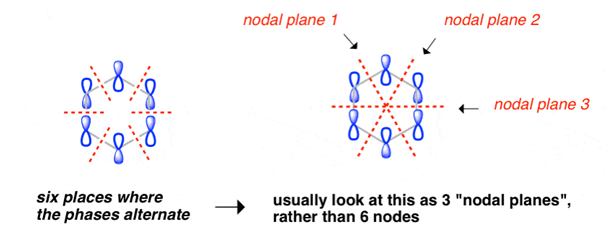
This orbital has zero overlap between adjacent p orbitals and therefore electrons in this orbital have the minimum possible delocalization. They are therefore the highest energy.

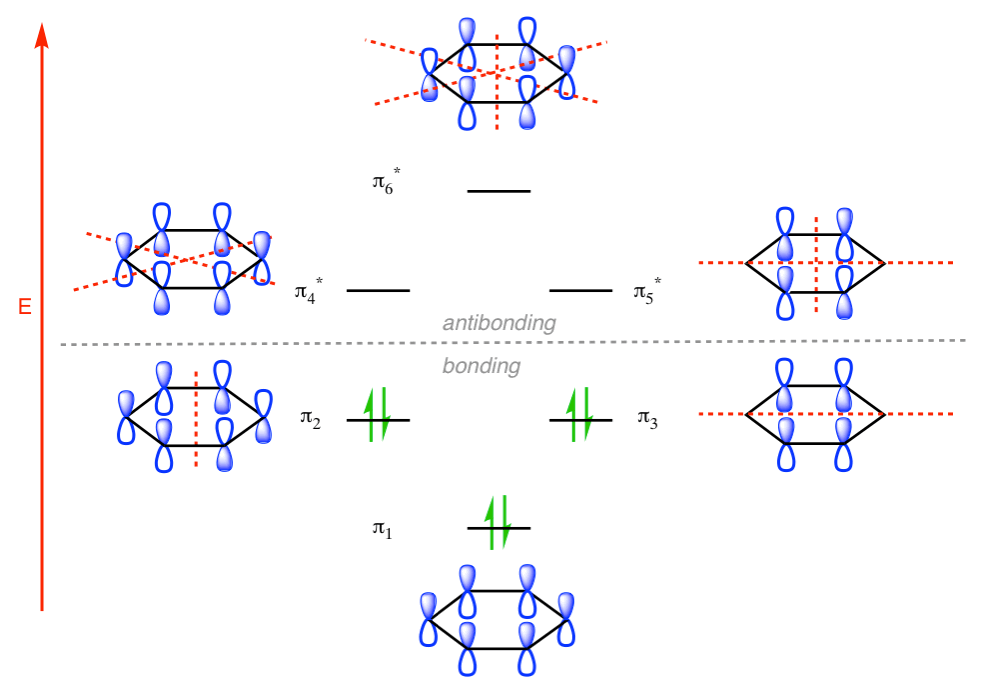
The pi molecular orbital of benzene

1.6 Crystal field theory and the energy level diagram for transition metal ions and their magnetic properties:

On attraction of positively charged metal cation and the negative charge on the non-bonding electrons of the ligand the interaction between a transition metal and ligands arises.

As a ligand approaches the metal ion, the electrons from the ligand will be closer to some of the d-orbitals and farther away from others, causing a loss of degeneracy. The electrons in the d-orbitals and those in the ligand repel each other due to repulsion between like charges. Thus the d-electrons closer to the ligands will have a higher energy than those further away which results in the d-orbitals splitting in energy.

The splitting can be affected by following factors:-

• Metal ion nature

• Metals oxidation state.

• Ligand arrangement around the metal ion

• Metal coordination number

Spectrochemical Series: The ability of ligands to cause a large splitting of the energy between the orbital is essentially independent of the metal ion and the spectrochemical series is a list of ligands ranked in order of their ability to cause large orbital separations.

I- < Br- < SCN- ~Cl- < F- < OH- ~ ONO- < C2O42- < H2O< NCS- < EDTA4-<NH3 ~ pyr ~ en<bipy<phen< CN- ~ CO

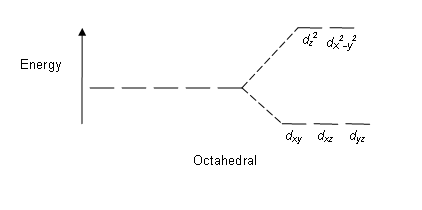
Octahedral: The crystal field stabilization energy (CFSE) is the stability that results from placing a transition metal ion in the crystal field generated by a set of ligands. It arises due to the fact that when the d orbitals are split in a ligand field, some of them become lower in energy than before. For example, in the case of an octahedron, the t2g set becomes lower in energy. As a result, if there are any electrons occupying these orbitals, the metal ion is more stable in the ligand field by the amount known as the CFSE. Conversely, the eg orbitals are higher in energy. So, putting electrons in them reduces the amount of CFSE.

Square Planar: Crystal field stabilization is applicable to the transition-metal complexes of all geometries. The reason that many d8 complexes are square-planar is the very large amount of crystal field stabilization that this geometry produces with this number of electrons.

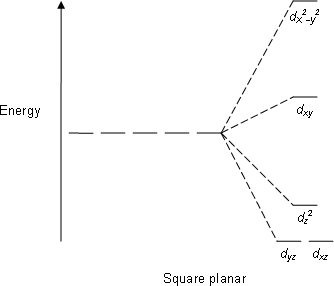
E.g; XeF4, PtCl2−

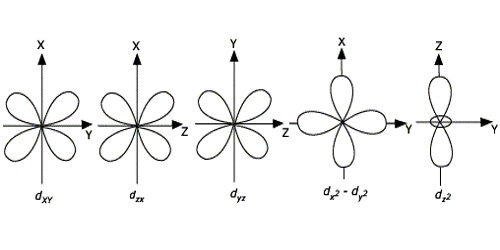
Tetrahedral:

dxy    dxz    dyz
The electron density (i.e., the lobes of the orbitals) lies nearest to the point charges.
dx2-y2    dz2
The electron density lies further away from the point charges.

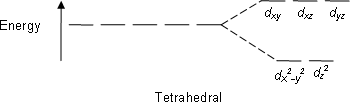
E.g; CH4

Energy level diagram of transition metal ion & magnetic property:

d-Orbital Splitting

CFT focuses on the interaction of the five (n −1)d orbital’s with ligands arranged in a regular array around a transition-metal ion. Other common structures, such as square planar complexes, can be treated as a distortion of the octahedral model. According to CFT, an octahedral metal complex forms because of the electrostatic interaction of a positively charged metal ion with six negatively charged ligands or with the negative ends of dipoles associated with the six ligands. In addition, the ligands interact with one other electrostatically. According to VSEPR theory the lowest-energy arrangement of six identical negative charges is an octahedron, which minimizes repulsive interactions between the ligands.

The energies of the d-orbital of a transition-metal ion are affected by an octahedral arrangement of six negative charges. The five d-orbital are initially degenerate. On the distribution of six negative charges uniformly over the surface of a sphere, the d-orbital remain degenerate, but their energy will be higher due to repulsive electrostatic interactions between the spherical shell of negative charge and electrons in the d-orbital. Placing the six negative charges at the vertices of an octahedron does not change the average energy of the d-orbital, but it does remove their degeneracy: the five d-orbital split into two groups whose energies depend on their orientations. The dz2 and dx2−y2 orbital point directly at the six negative charges located on the x, y, and z axes. Consequently, the energy of an electron in these two orbital will be greater than it will be for a spherical distribution of negative charge because of increased electrostatic repulsions. In contrast, the other three d orbital (dxy, dxz, and dyz, collectively called the t2g orbitals) are all oriented at a 45° angle to the coordinate axes, so they point between the six negative charges. The energy of an electron in any of these three orbital is lower than the energy for a spherical distribution of negative charge.

The Nature of the Ligands

The Δo observed for a series of complexes of the same metal ion depends strongly on the nature of the ligands. For a series of chemically similar ligands, the magnitude of Δo decreases as the size of the donor atom increases. E.g.: Δo values for halide complexes generally decrease in the order F− > Cl− > Br− > I−. In addition, a small neutral ligand with a highly localized lone pair, such as NH3, results in significantly larger Δo values that might be expected. Because of the lone pair points directly at the metal ion, the electron density along the M–L axis is greater than for a spherical anion such as F−. The experimentally observed order of the crystal field splitting energies produced by different ligands is called the spectro-chemical series, shown here in order of decreasing Δo:

CO≈CN−> NO−2>en>NH3>SCN−>H2O>oxalate2−>OH−>F>acetate−>Cl−>Br−>I−

Magnetic Property of transition elements:

A magnetic field is generated due to the orbital motion and spin of the electron. The spinning of an electron in an orbit is very much similar to flow of electric current in a closed circuit. Therefore, an unpaired electron is regarded as a micro magnet which has a definite magnetic moment. A substance which contains an unpaired electron when placed in a magnetic field interacts with the applied field. Consequently, an attractive force is exerted and the paramagnetic property is shown. The number of unpaired electrons determines the magnitude of magnetic moment. Higher the number of unpaired electrons more is the magnetic moment and greater will be the paramagnetic behavior of the substance.

In the case of paired electrons, the electrons in each pair will have opposite spin. The magnetic field created by the electrons of same pair is equal and opposite in nature. Hence, the magnetic field which is created by one electron is cancelled by the other. So the net effect of the magnetic moment is zero. These kinds of substances show diamagnetic property and are repelled by the applied magnetic field.

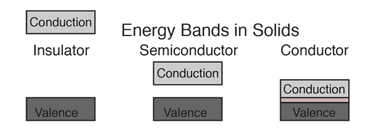
1.7             Band structure of solids and the role of doping on band structures:

This is extracted from the X-ray diffractions that solid is an ordered structure therefore the atoms of solids interact strongly and setup an internal electric field that is periodic in nature. This periodic electric field affects the motion of free electrons. The application of quantum mechanics to the motion of electrons in solids shows that the allowed value of electron energy is distributed in bands. The most fundamental unit of matter which is capable of independent existence is called atoms. The atoms consist of center body which is called as the nucleus while it is surrounded by the electrons. The electrons present at the outermost orbits are called valence electrons. Each atom has different orbits and hence all of it is having the different energy levels. This energy level consists of several quantum states. The atoms are grouped together to form the solids which consist of millions of electron belonging to each orbit of atoms. Each of them possessing the different energy. As long as the atoms are separated their interaction are negligible.  As atoms comes together to create a closed pact periodic structure they interact strongly due to their proximity to each other. This tends to leave behind the state of energy level is same for all an isolated atoms there arise closely state separate levels which falls into groups. The transformation of single energy level into 2 or more separate energy level is energy level splitting. When 2 atoms come close then 1 energy level split in 2 energy level and so on. In general an interacting atoms cause a particular energy level split into n level. The group of discrete and closely space energy levels resulting from splitting is called as the energy bands.

The arrangement of atoms is very close to each other such that the energy levels of the outermost orbital electrons are affected while the energy level of the innermost electrons is not affected by the neighboring atoms. The different energy bands in solid are

Valence band

Conduction band

Forbidden band

Valence band: The energy band that consists of valence electrons energy levels, is known as the valence band.

Conduction band: The energy band that consists of free electrons energy levels, is known as the conduction band.

Forbidden band: The energy gap between the valence band and the conduction band is known as the forbidden band which is also known as the forbidden gap. The electrical conductivity of a solid is determined from the forbidden gap and also the classification of the materials as conductors, semiconductors, and insulators.