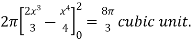UNIT– 1
Calculus
Definition- The locus of centres of curvature of a given curve is called the evolute of that curve.
The locus of the center of curvature C of a variable point P on a curve is called the evolute of the curve and the curve itself is called involute of the evolute.
Evolute is nothing but an equation of the curve.
Method to find the evolute-
1. If a curve equation is given and we need to prove left hand side is equal to right hand side (L.H.S = R.H.S), then
First of all find the curvature C(X,Y), where
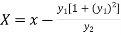
And

Then take left hand side and put X in place of x and Y in place of y.
2. When the curve is given and we need to find the evolute, then-
Find the curvature first and then re-write as x in terms of X and y in terms of Y and then put in the given curve
Properties of envelope and evolute-
1. The normal at any point of a curve is a tangent to its evolute touching at the corresponding centre of curvature.
2. The evolute is one only but there can be infinite involutes.
3. The difference between the radii of curvature at two points of a curve is equal to the length of the arc of the evolute between the two corresponding points.
Example: Prove that the evolute of parabola 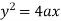 is given by
is given by 
Sol. It is given that-
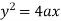
If (X,Y) are the coordinates of the centre of curvature at any point P(x , y) on the curve y = f(x), then X and Y are given as-
 …………….. (1)
…………….. (1)
Now consider the equation of parabola (given)

On differentiating w.r.t x-
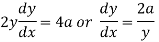
Again differentiating w.r.t.x
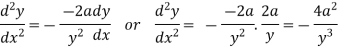
Put these derivatives in (1), we get-
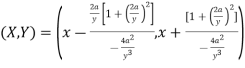
Now consider X,
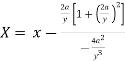
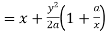
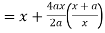
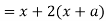
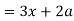
Here we get-
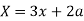
Now consider,
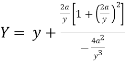
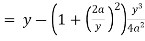


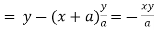
Here we get-

Now
Taking L.H.S of 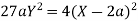
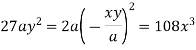
Taking R.H.S of 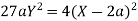
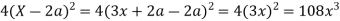
Hence proved.
Example: Find the evolute of the ellipse 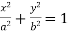 .
.
Sol. It is given that-
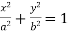
The parametric equations are 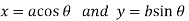
Now,
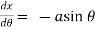 and
and 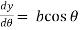
So that-
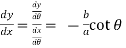
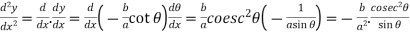
Which gives,
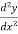
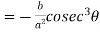
Co-ordinates of centre of curvature are (X , Y),
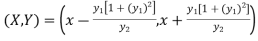 …………….. (1)
…………….. (1)
Consider X,
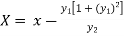
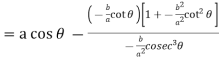
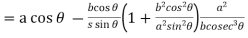
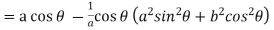
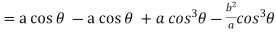
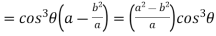
We get-
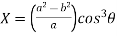
Now consider Y,
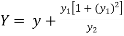

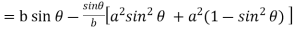
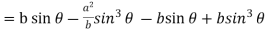

So that we get-
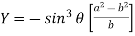
Eliminating 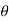 from X and Y, we get,
from X and Y, we get,
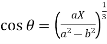 and
and 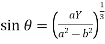
We know that 
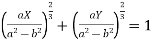
Which gives on solving,
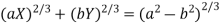
Which is the required evolute.
When we apply limits in indefinite integrals are called definite integrals.
If an expression is written as 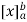 , here ‘b’ is called upper limit and ‘a’ is called lower limit.
, here ‘b’ is called upper limit and ‘a’ is called lower limit.
If f is an increasing or decreasing function on interval [a , b], then
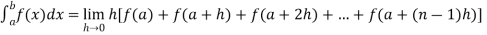
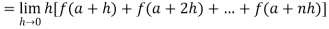
Where 
Properties-
1. The definite integral applies only if a<b, but it would be appropriate to include the case a = b and a>b as well, in that case-
If a = b, then
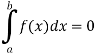
And if a>b, then
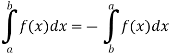
2. Integral of a constant function-
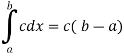
3. Constant multiple property-
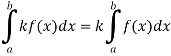
4. Interval union property-
If a < c < b, then
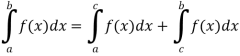
5. Inequality-
If c and d are constants such that 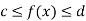 for all x in [a , b], then
for all x in [a , b], then
c(b – a)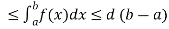
Note- if a function f:[a , b]→R is continuous, then the function ‘f’ is always Integrable.
Example-1: Evaluate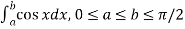 .
.
Sol. Here we notice that f:x→cos x is a decreasing function on [a , b],
Therefore by the definition of the definite integrals-
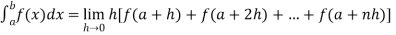
Then
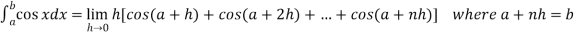
Now,
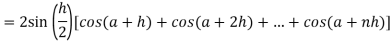
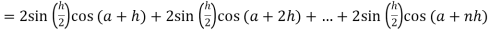
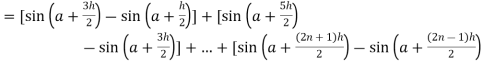
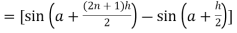
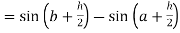
Here 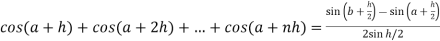
Thus
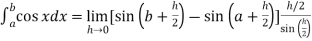

Example-2: Evaluate 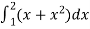
Sol. Here 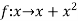 is an increasing function on [1 , 2]
is an increasing function on [1 , 2]
So that,
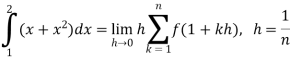
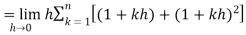

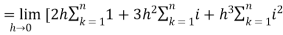 …. (1)
…. (1)
We know that-
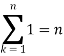

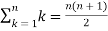
And


Then equation (1) becomes-
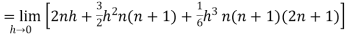
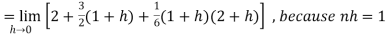
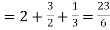
Note- we can find the definite integral directly as-
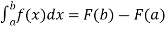
Example-3: Evaluate- 
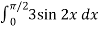

Sol. 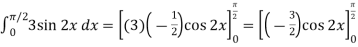
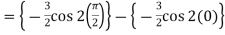
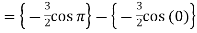
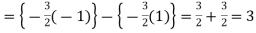
Improper integrals
(1) Let f is function defined on [a , ∞) and it is integrable on [a , t] for all t >a, then
If 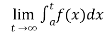 exists, then we define the improper integral of f over [a , ∞) as follows-
exists, then we define the improper integral of f over [a , ∞) as follows-
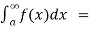
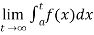
(2) Let f is function defined on (-∞,b] and it is integrable on [t , b] for all t >b, then
If  exists , then we define the improper integral of f over (-∞ , b] as follows-
exists , then we define the improper integral of f over (-∞ , b] as follows-
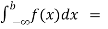
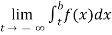
(3) ) Let f is function defined on (-∞, ∞] and it is integrable on [a , b] for every closed and bounded interval [a , b] which is the subset of R., then
If 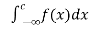 and
and 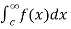 exist for some c belongs to R , then we define the improper integral of f over (-∞ , ∞ ) as follows-
exist for some c belongs to R , then we define the improper integral of f over (-∞ , ∞ ) as follows-
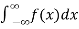 =
= 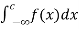 +
+ 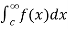
(4) Let f is function defined on (a , ∞) and 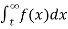 exists for all t>a , then
exists for all t>a , then
If 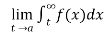 exists , then we define the improper integral of f over (a , ∞) as follows-
exists , then we define the improper integral of f over (a , ∞) as follows-

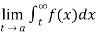
(5) ) Let f is function defined on (-∞ , b) and 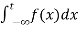 exists for all t<b , then
exists for all t<b , then
If 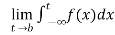 exists , then we define the improper integral of f over (-∞ , b) as follows-
exists , then we define the improper integral of f over (-∞ , b) as follows-
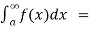
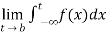
Improper integrals over finite intervals-
(1) Let f is function defined on (a, b] and 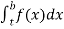 exists for all t ∈(a,b) , then
exists for all t ∈(a,b) , then
If 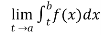 exists , then we define the improper integral of f over (a , b] as follows-
exists , then we define the improper integral of f over (a , b] as follows-
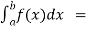
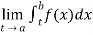
(2) Let f is function defined on [a, b) and 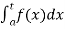 exists for all t ∈(a,b) , then
exists for all t ∈(a,b) , then
If 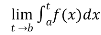 exists , then we define the improper integral of f over [a , b) as follows-
exists , then we define the improper integral of f over [a , b) as follows-
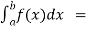
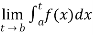
(3) Let f is function defined on [a, c) and (c , b] . if 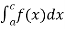 and
and 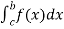 exist
exist
then we define the improper integral of f over [a , b] as follows-
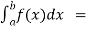
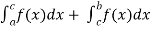
The beta and gamma functions are defined as-
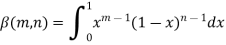
And
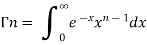
These integrals are also known as first and second Eulerian integrals.
Note- Beta function is symmetrical with respect to m and n.
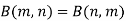
Some important results-
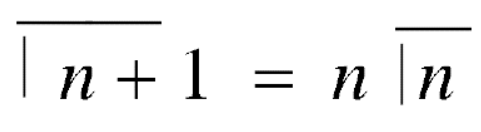
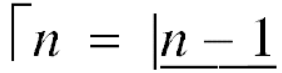
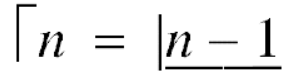
Ex.1: Evaluate 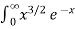 dx
dx
Solution
 dx =
dx = 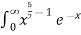 dx
dx
= γ(5/2)
= γ (3/2+ 1)
= 3/2 γ(3/2 )
= 3/2. ½ γ (½ )
= 3/2. ½ π
= ¾ π
Ex. 2: Find γ(-½)
Solution: (-½) + 1 = ½
γ(-1/2) = γ(-½ + 1) / (-½)
= - 2 γ(1/2 )
= - 2 π
Ex. 3. Show that 

Solution:
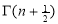 =
= 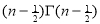
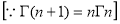
= 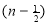
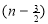

= 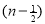
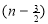
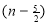
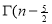 ) .......................
) .......................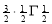
= 
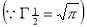
= 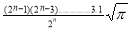


Ex. 4: Evaluate 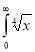
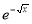 dx.
dx.
Solution: Let 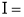
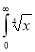
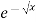 dx
dx
X | 0 |  |
t | 0 |  |
Put  or
or 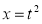 ;dx =2t dt .
;dx =2t dt .
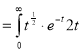 dt
dt
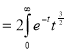 dt
dt
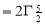
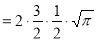
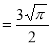
Ex. 5: Evaluate 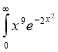 dx.
dx.
Solution: Let 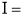
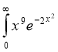 dx.
dx.
x | 0 | 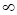 |
t | 0 | 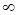 |
Put 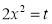 or
or 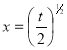 ; 4x dx = dt
; 4x dx = dt
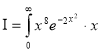 dx
dx

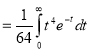
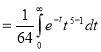
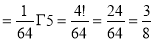
Evaluation of beta function 𝛃 (m, n)-
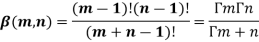
Here we have-
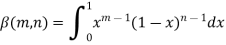
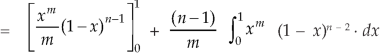
Or
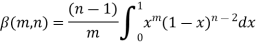
Again integrate by parts, we get-
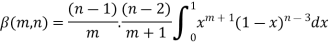
Repeating the process above, integrating by parts we get-
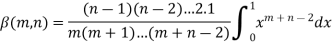
Or
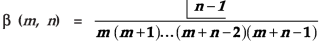
Evaluation of gamma function-
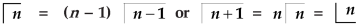
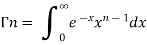
Integrating by parts, we take 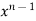 as first function-
as first function-
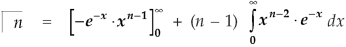
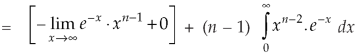
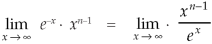
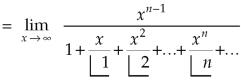
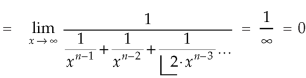
We get-
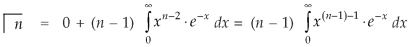
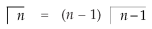
Replace n by n+1,

Relation between beta and gamma function-
We know that-
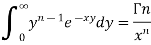
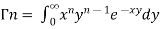 ………… (1)
………… (1)
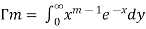 …………………..(2)
…………………..(2)
Multiply equation (1) by 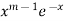 , we get-
, we get-
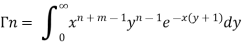
Integrate both sides with respect to x within limits x = 0 to x = 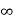 , we get-
, we get-
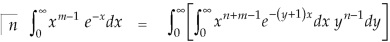
But
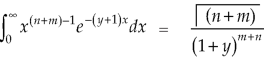
By putting λ = 1 + y and n = m + n
We get by using this result in (2)-
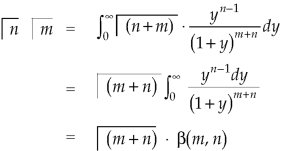
So that-
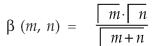
Definition : Beta function
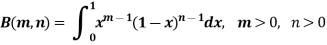
Properties of Beta function :
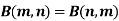
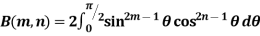
3. 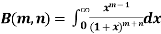
4. 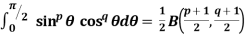
Example(1): Evaluate I = 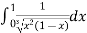
Solution:
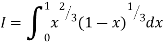
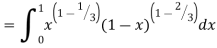
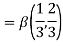
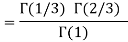
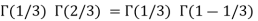
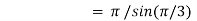
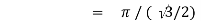
= 2 π/3
Example (2): Evaluate: I = 02 x2 / (2 – x ) . dx
Solution:
Letting x = 2y, we get
I = (8/2) 01 y 2 (1 – y ) -1/2dy
= (8/2). B (3, 1/2 )
= 642 /15
BETA FUNCTION MORE PROBLEMS
Relation between Beta and Gamma functions :
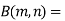 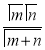 | ||||||
Example(1): Evaluate: I = 0a x4 (a2 – x2 ) . dx Solution: Letting x2 = a2 y , we get I = (a6 / 2) 01 y 3/2 (1 – y )1/2dy = (a6 / 2) . B(5/2 , 3/2 ) = a6 /3 2 Example(2): Evaluate: I = 02 x (8 – x3 ) . dx Solution: Let x3 = 8y I = (8/3) 01 y-1/3 (1 – y ) 1/3 . dy
= (8/3) B(2/3 , 4/3 ) = 16 π / ( 9 3 ) Example(3): Prove that 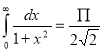 Solution : Let 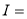 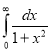 Put 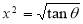 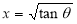 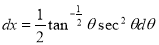 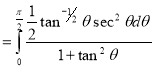 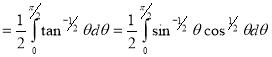 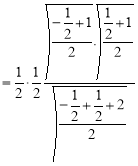  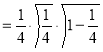 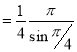 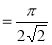  Example(4): Evaluate 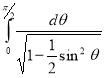 Solution :Let 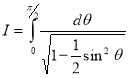 Put 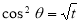   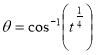 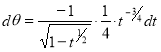 When 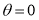 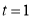 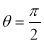 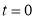
Also 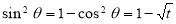 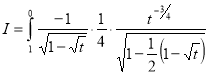 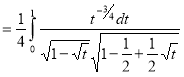 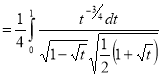  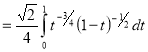 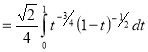 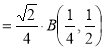 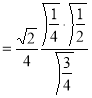 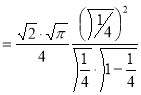 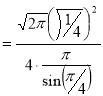 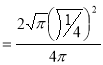 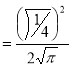
Example(5): Show that 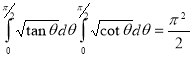 Solution : 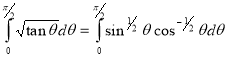 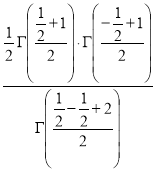 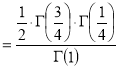 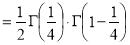 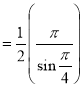  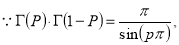 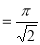 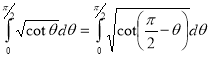 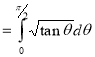 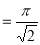  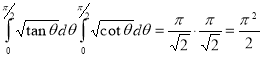 |
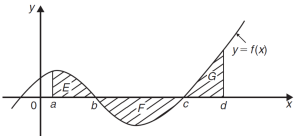
Area under and between the curves-
Total shaded area will be as follows of the given figure( by using definite integrals)-
Total shaded area = 
Example-1: Determine the area enclosed by the curves-
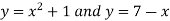
Sol. We know that the curves are equal at the points of interaction, thus equating the values of y of each curve-
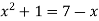
Which gives-
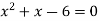
By factorization,
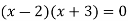
Which means,
x = 2 and x = -3
By determining the intersection points the range the values of x has been found-
x | -3 | -2 | -1 | 0 | 1 | 2 |
 | 10 | 5 | 2 | 1 | 2 | 5 |
And
x | -3 | 0 | 2 |
y = 7 - x | 10 | 7 | 5 |
We get the following figure by using above two tables-
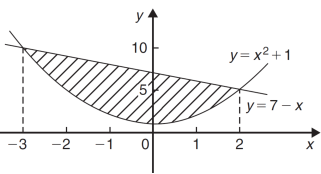
Area of shaded region = 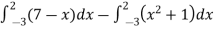
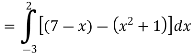
= 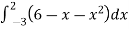
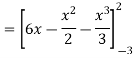
= ( 12 – 2 – 8/3 ) – (-18 – 9/2 + 9)
= 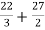
= 125/6 square unit
Example-2: Determine the area bounded by three straight lines y = 4 – x, y = 3x and 3y = x
Sol. We get the following figure by using the equations of three straight lines-
y = 4 – x, y = 3x and 3y = x
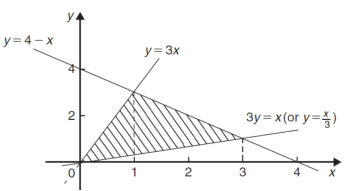
Area of shaded region-
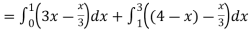
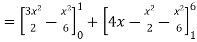
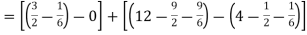
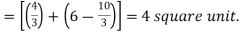
Example-3: Find the area enclosed by the two functions-
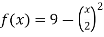 and g(x) = 6 – x
and g(x) = 6 – x
Sol. We get the following figure by using these two equations 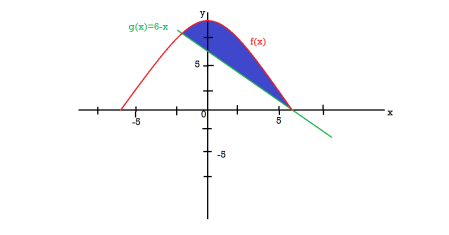
To find the intersection points of two functions f(x) and g(x)-
f(x) = g(x)
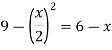
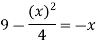
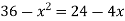
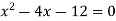
On factorizing, we get-
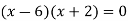
x = 6, -2
Now
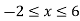
Then, area under the curve-
A = 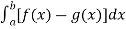
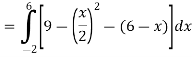
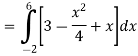
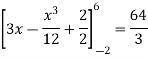
Therefore the area under the curve is 64/3 square unit.
Areas and volumes of revolutions
The volume of revolution (V) is obtained by rotating area A through one revolution about the x-axis is given by-
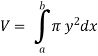
Suppose the curve x = f(y) is rotated  about y-axis between the limits y = c and y = d, then the volume generated V, is given by-
about y-axis between the limits y = c and y = d, then the volume generated V, is given by-
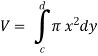
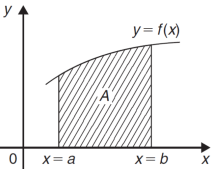
Example-1: Find the area enclosed by the curves 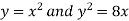 and if the area is rotated
and if the area is rotated  about the x-axis then determine the volume of the solid of revolution.
about the x-axis then determine the volume of the solid of revolution.
Sol. We know that, at the point of intersection the coordinates of the curve are equal. So that first we will find the point of intersection-
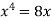
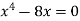
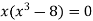
We get,
x = 0 and x = 2
The curve of the given equations will look like as follows-
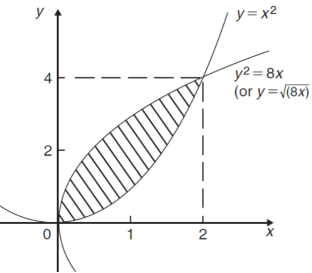
Then,
The area of the shaded region will be-
A = 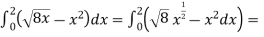
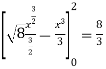
So that the area will be 8/3 square unit.
The volume will be
= (volume produced by revolving 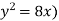 – (volume produced by revolving
– (volume produced by revolving 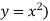
= 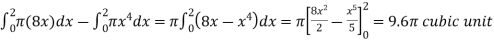
Method of cylindrical shells-
Let f(x) be a continuous and positive function. Define R as the region bounded above by the graph f(x), below by the x-axis, on the left by the line x = a and on the right x = b, then the volume of the solid of revolution formed by revolving R around the y-axis is given by
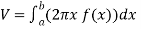
Example-2: Find the volume of the solid of revolution formed by revolving R around y-axis of the function f(x) = 1/x over the interval [1 , 3].
Sol. The graph of the function f(x) = 1/x will look like-
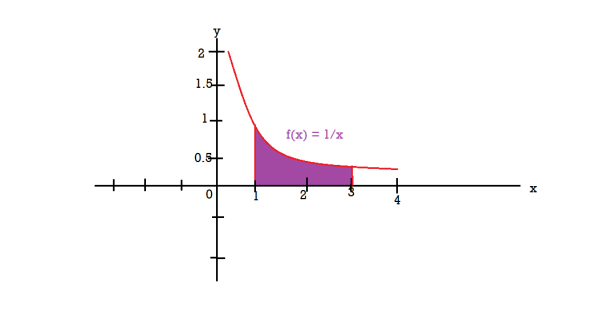
The volume of the solid of revolution generated by revolving R(violet region) about the y-axis over the interval [1 , 3]
Then the volume of the solid will be-
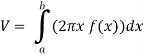
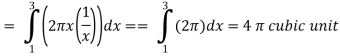
Example-3: Find the volume of the solid of revolution formed by revolving R around y-axis of the function f(x) = 2x - x² over the interval [0 , 2].
Sol. The graph of the function f(x) = 2x - x² will be-
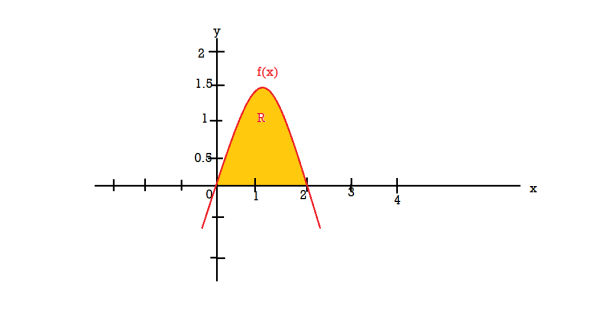
The volume of the solid is given by-
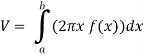
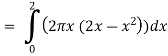
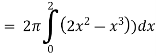
=