MODULE 7
SOLUTION OF WAVE EQUATION
Define the potential energy:
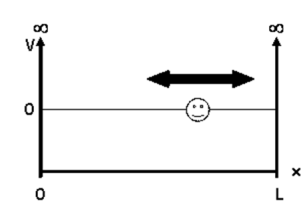 A particle in a 1D infinite potential well of dimension LL.
A particle in a 1D infinite potential well of dimension LL.
The potential energy is 0 in the box (V=0 for 0<x<L) and drives to eternity at the walls of the box (V=∞ for x<0 or x>L).
We accept the walls have infinite potential energy to confirm that the particle has zero chance of existence at the walls or outside the box.
Undertaking significantly simplifies our later mathematical designs as we employ these boundary circumstances when resolving the Schrödinger Equation.
Solution of the Schrödinger Equation:
The time-independent Schrödinger equation for a particle of mass (m) moving in one direction with energy (E) is



- ℏ is the reduced Planck Constant ,here ℏ=h/2π
- m is the mass of the particle
- ψ(x) is the stationary time-independent wavefunction
- V(x) is the potential energy as a function of position
- E is the energy, a real number
Take a modest, attractive delta function potential and aspect for the bound states.
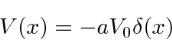
These will have energy less than zero so the answers are
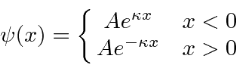
Wherever
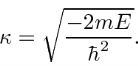
There are only double regions, above and below the delta function.
We don't necessity to worry about the one point at x=0 -the two solutions will bout there.
We have previously made the wave function nonstop at x=0 by using the similarcoefficient A for the answer in both sections.

We now need to see the boundary state on the first derivative at x=0. Recall that the delta function causes a recognized gap in the first derivative.
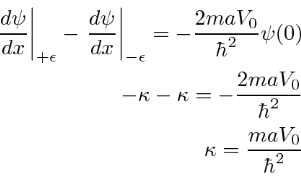
Placing in the formula for k in relations of the energy.
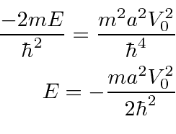
The linear harmonic oscillator defines pulsations in molecules and their equivalents in solids, the phonons.Though the linear harmonic oscillator can signify justly non-elementary substances like a solid and a molecule, it delivers a gap into the most basic structure in the physical domain.
The Schrodinger Equation for a one –dimentional harmonic oscillator is
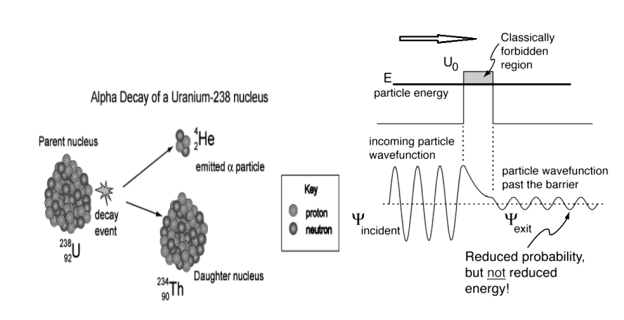
7.4 Scattering from A Potential Barrier and Tunnelling; Related Examples Like Alpha- Decay: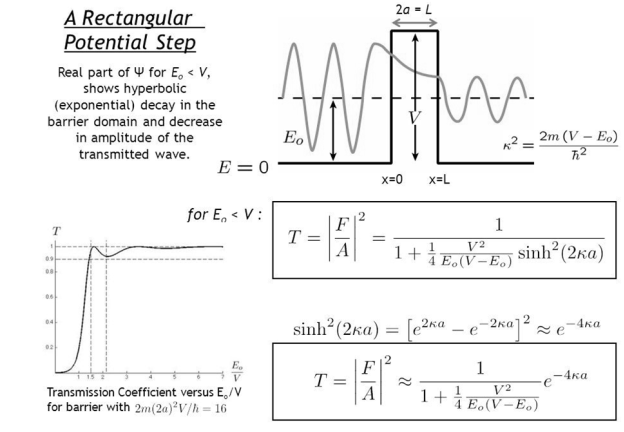
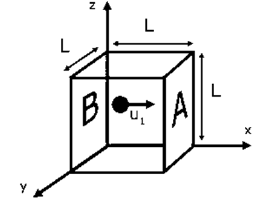
Simplification of the consequences for a two-dimensional square box to a three-dimensional cubic box is direct.
Meanwhile we living in a three-dimensional world, this simplification is ansignificant one, and we want to be able to consider about energy levels and wave functions in three dimensions.
The potential energy 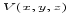 for the cubic box is well-defined to be 0 if
for the cubic box is well-defined to be 0 if ![$x\in[0,L]$](https://glossaread-contain.s3.ap-south-1.amazonaws.com/epub/1643209523_0476847.png) ,
, ![$y\in[0,L]$](https://glossaread-contain.s3.ap-south-1.amazonaws.com/epub/1643209523_0939684.png) and
and ![$z\in[0,L]$](https://glossaread-contain.s3.ap-south-1.amazonaws.com/epub/1643209523_193772.png) and infinite else.
and infinite else.
This resources that the wave function 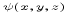 needcontent six boundary circumstances
needcontent six boundary circumstances 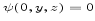 ,
, 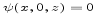 ,
, 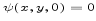 ,
, 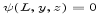 ,
, 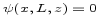 and
and 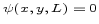 .
.
We first note that the classical energy is the sum of three terms
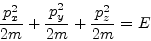
Where 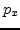 ,
, 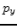 and
and 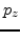 are the three mechanisms of the particle's momentum vector
are the three mechanisms of the particle's momentum vector 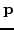 . Thus, we can write the energy as
. Thus, we can write the energy as
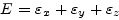
Equivalent to the kinetic energy in the 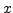 ,
, 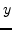 and
and 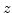 directions. Since the energy is a simple sum of energies for the
directions. Since the energy is a simple sum of energies for the 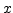 ,
, 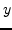 and
and 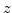 directions, the wave function will be a invention of wave function forms for the one-dimensional box, and in order to fulfill the first three of the boundary conditions,
directions, the wave function will be a invention of wave function forms for the one-dimensional box, and in order to fulfill the first three of the boundary conditions,
we can take the 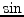 functions:
functions:
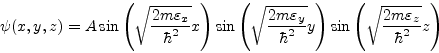
As in the two-dimensional case, applying second three boundary conditions yields the allowed values of 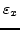 ,
, 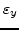 and
and 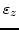 , which now need three integers
, which now need three integers 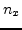 ,
, 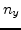 and
and 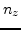 :
:
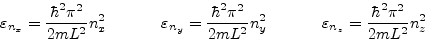
So that the allowableprinciples of the total energy are
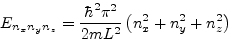
And the wave functions develop
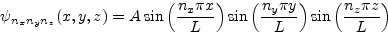
The constant 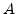 is resolute from the standardizationstate
is resolute from the standardizationstate
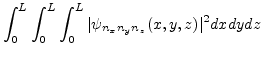
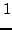
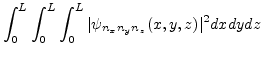
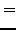
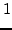
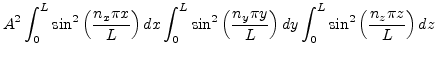
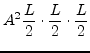


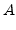
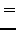
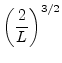
Thus, the wave meanings are
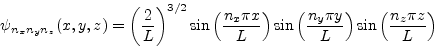
As using the two-dimensional box, the three integers 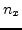 ,
, 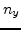 and
and 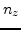 are limited to the usual numbers 1,2, 3, Thus, the lowest energy or ground-state energy is
are limited to the usual numbers 1,2, 3, Thus, the lowest energy or ground-state energy is
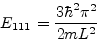
Imagining the wave functions is delicatesince of their high dimensionality. The most common method of imagining functions of three variables is the use of an isosurface.
An isosurface of a function 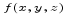 is the whole set of points
is the whole set of points 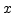 ,
, 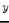 , and
, and 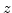 for which
for which 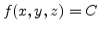 , where
, where 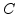 is a selected constant. Henceforth the name isosurface - the value of the function
is a selected constant. Henceforth the name isosurface - the value of the function 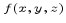 is the same at all points on the surface.
is the same at all points on the surface.
For wave functions, where the sign can be positive or negative, it is valuable to base the value of 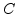 not on the wave function significance but rather on the probability density
not on the wave function significance but rather on the probability density 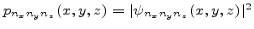 .
.
The numberunderdemonstrations two isosurfaces of the wave function 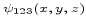 .
.
The first happens at a probability density value of 0.64 and the other happens at 0.04. These values then suggest that the wave function can have a fixed positive or negative value beside the surface.
In one case, 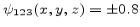 , while in the other,
, while in the other, 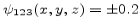 .
.
Consider a particle of mass 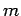 and energy
and energy 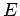 relating with the simple square potential well
relating with the simple square potential well
![\begin{displaymath}
V(x) = \left\{\begin{array}{lcl}
-V_0&\mbox{\hspace{1cm}}&\m...
... x\leq a/2$}\ [0.5ex]
0&&\mbox{otherwise}
\end{array}\right.,
\end{displaymath}](https://glossaread-contain.s3.ap-south-1.amazonaws.com/epub/1643209527_0684721.png)
Where 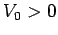 .
.
Now, if E>0 then the particle is unbounded. Therefore, when the particle meets the well it is either reflected or transmitted. As is simply confirmed, the reflection and transmission probabilities are assumed and given by
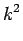
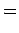
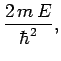
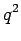
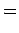
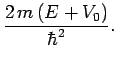
Assume that E<0.
In this example, the particle is bounded (i.e., 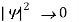 as ).
as ). 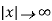
Now, it is simply got that independent solutions of Schrödinger's equation in the symmetric V(-x)= V(x) potential must be either completely symmetric
[i.e., (-x)=(x)], or completely anti-symmetric [i.e., (-x)=-(x)].
Also, the answers must fulfil the boundary condition

Let us, first of all, search for a completely symmetric solution. In the section to the left of the well x<-a/2 the solution of Schrödinger's equation which fulfills the boundary condition 0 and x - 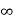 is
is
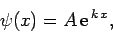
Where
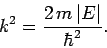
By symmetry, the explanation in the region to the right of the well (i.e., x>a/2) is
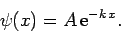
The solution inside the well (i.e., 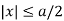 ) which fulfills the symmetry limit
) which fulfills the symmetry limit
(-x)=(x) is
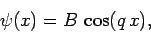
Where
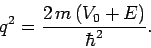
Now, we have supposed that .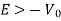 The constraint that (x) and its first derivative be constant at the edges of the well (i.e., at
The constraint that (x) and its first derivative be constant at the edges of the well (i.e., at 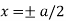 ) yields
) yields

Let Y=qa/2
It show that
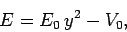
where
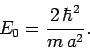
Furthermore,
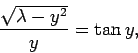
With
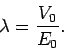
Now, y need lie in the range 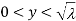 : i.e.,
: i.e., 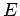 must lie in the range
must lie in the range .
.
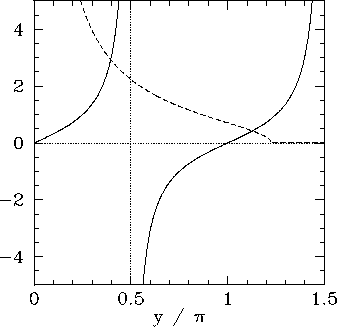
Figure: The curves tany (solid) and 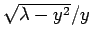 (dashed), calculated for
(dashed), calculated for 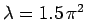 . The latter curve takes the value
. The latter curve takes the value  when
when 
Now, the solutions correspond to the connection of the curve 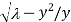 with the curve tan y. Figure shows these two curves designed for a particular value of
with the curve tan y. Figure shows these two curves designed for a particular value of 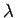 . In this case, the curves meet twice, representing the presence of two completely symmetric bound states in the well. Also, it is obvious, from the figure, that as
. In this case, the curves meet twice, representing the presence of two completely symmetric bound states in the well. Also, it is obvious, from the figure, that as 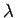 rises (i.e., as the well becomes deeper) there are more and more bound states. However, it is also obvious that there is continually at least one completely symmetric bound state, no matter how small
rises (i.e., as the well becomes deeper) there are more and more bound states. However, it is also obvious that there is continually at least one completely symmetric bound state, no matter how small 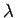 becomes (i.e., no matter how shallow the well becomes). In the limit
becomes (i.e., no matter how shallow the well becomes). In the limit 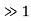 (i.e., the limit in which the well becomes very deep), the solutions to Eq. (384) asymptote to the roots of tan y=
(i.e., the limit in which the well becomes very deep), the solutions to Eq. (384) asymptote to the roots of tan y=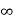 .
.
This provides y=(2j-1)/2, where j is a positive integer, or
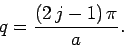
These explanations are equal to the odd- infinite square well explanations stated by
infinite square well explanations stated by
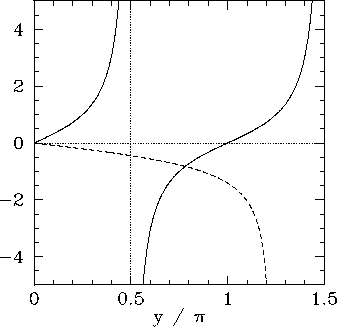
Figure: The curves tan y (solid) and 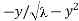 (dashed), calculated for
(dashed), calculated for 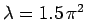 .
.
For the case of a completely anti-symmetric bound state, like examination to the above yields
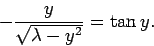
The solutions of this equation correspond to the intersection of the curve tan y with the curve 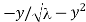 . Figure shows these two curves plotted for the same value of
. Figure shows these two curves plotted for the same value of 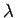 as that used in Fig. In this case, the curves interconnect once, representing the being of a single completely anti-symmetric bound state in the well. It is, over, evident from the figure that as
as that used in Fig. In this case, the curves interconnect once, representing the being of a single completely anti-symmetric bound state in the well. It is, over, evident from the figure that as  increases (i.e., as the well becomes deeper) there are more and more bound states. However, it is also evident that when
increases (i.e., as the well becomes deeper) there are more and more bound states. However, it is also evident that when 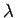 becomes sufficiently small [i.e.,
becomes sufficiently small [i.e., 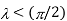 ] then there is no completely anti-symmetric bound state. In other words, a very shallow potential well continuously keeps a completely symmetric bound state, but does not usually keep a completely anti-symmetric bound state.
] then there is no completely anti-symmetric bound state. In other words, a very shallow potential well continuously keeps a completely symmetric bound state, but does not usually keep a completely anti-symmetric bound state.
In the boundary 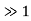 (i.e., the limit in which the well becomes very deep), the solutions asymptote to the roots of tan y=0. This gives y=j, where j is a positive integer, or
(i.e., the limit in which the well becomes very deep), the solutions asymptote to the roots of tan y=0. This gives y=j, where j is a positive integer, or
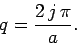
The linear harmonic oscillator defines pulsations in molecules and their equivalents in solids, the phonons.Though the linear harmonic oscillator can signify justly non-elementary substances like a solid and a molecule, it delivers a gap into the most basic structure in the physical domain.
The Schrodinger Equation for a one –dimentional harmonic oscillator is

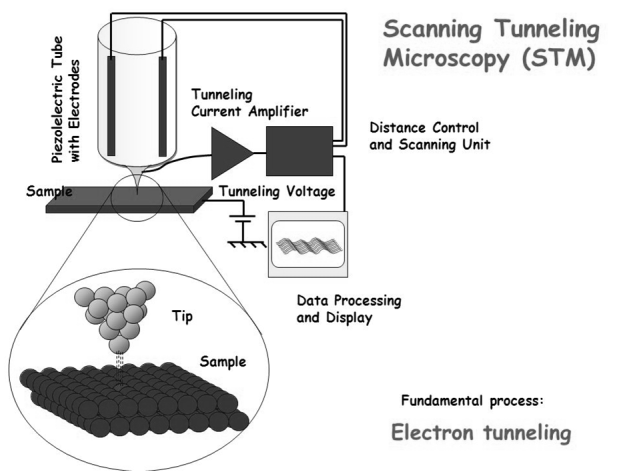
Tunneling is a quantum mechanical effect. A tunnel current occurs when electrons move through a barrier that they should not be able to cross. In classic terms, if you don't have enough energy to move "over" a barrier, you won't. However, in the world of quantum mechanics, electrons have wave properties. These waves do not end abruptly on a wall or barrier, but decrease rapidly. If the barrier is thin enough, the probability function can be extended to the next region via the barrier! Due to the low probability that an electron is on the other side of the barrier, given enough electrons, some will move and appear on the other side. When an electron moves through the barrier in this way, it is called a tunnel.
Quantum mechanics tells us that electrons have wave and particle properties. The construction of the tunnel is an undulating effect.

Schematic of electron wavefunction.
The image above shows that when an electron (the wave) hits a barrier, the wave does not stop abruptly, but gradually decreases exponentially. For a thick barrier, the wave does not pass.
The image below shows the scenario if the barrier is rather thin (about a nanometer). Part of the wave passes through, and therefore some electrons can appear on the other side of the barrier.
Due to the sharp decrease in the probability function across the barrier, the number of electrons that will actually form a tunnel depends heavily on the thickness of the barrier. The current through the barrier falls exponentially with the thickness of the barrier.
To extend this description to STM: The starting point of the electron is the tip or the sample, depending on the configuration of the instrument. The barrier is the gap (air, vacuum, liquid) and the second region is the other side, i.e. the tip or the sample, depending on the experimental configuration. By monitoring the current through the gap, we have excellent control over the distance of the tip-sample.
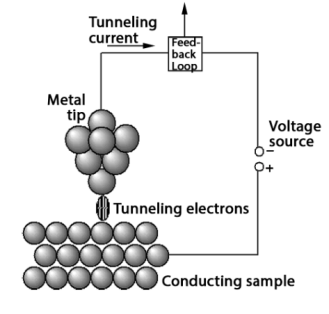
Feedback loop and electron tunneling for scanning tunneling microscopy (STM).
Reference:
1. G. MAIN, “VIBRATIONS AND WAVES IN PHYSICS”, CAMBRIDGE UNIVERSITY PRESS,1993.
2. H. J. PAIN, “THE PHYSICS OF VIBRATIONS AND WAVES”, WILEY, 2006.
3. E. HECHT, “OPTICS”, PEARSON EDUCATION, 2008.
4. A. GHATAK, “OPTICS”, MCGRAW HILL EDUCATION, 2012.
5. O. SVELTO, “PRINCIPLES OF LASERS”, SPRINGER SCIENCE & BUSINESS MEDIA, 2010.