MODULE 6
INTRODUCTION TO QUANTUM MECHANICS
Time-independent equation
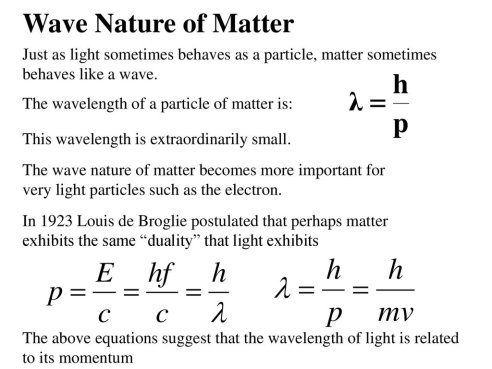
The time-dependent Schrödinger equation described above predicts that wave functions can form standing waves, called stationary states. ... Stationary states can also be described by a simpler form of the Schrödinger equation, the time-independent Schrödinger equation (TISE).
Time Dependent Schrodinger Equation
The time dependent Schrodinger equation for one spatial dimension is of the form
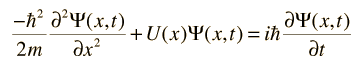
For a free particle where U(x) =0 the wavefunction solution can be put in the form of a plane wave
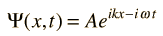
For other problems, the potential U(x) serves to set boundary conditions on the spatial part of the wavefunction and it is helpful to separate the equation into the time-independent Schrodinger equation and the relationship for time evolution of the wavefunction
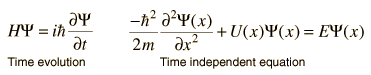
It was Max Born, in 1926, who linked the function of the quantum wave to empirical meaning. He found that squaring the wave function module gives the density of the probability of occurrence, like observing an electron. A diffracted electron can be found in any space for which the wave function is not zero. If the meaning of the wave function is limited to what the square of the module refers to, what happens between the diffraction and the subsequent detection of the electron (the intermediate period) is irrelevant. This is vital for any realistic microscopic domain theory.
So what happens with the interim period? Born's discovery is consistent with the electron found somewhere, anywhere or nowhere in the intermediate period. Maybe you can imagine even more possibilities! The important thing is to find out the importance or even the relevance of the difference between the three possibilities. What we do know is that the question has no relevance for the probability that the electron is somewhere, if observed. That is why what is happening in the intermediate period can form the basis for any number of different quantum interpretations, provided that the wave function and its importance are never lost sight of. In this way, each interpretation has the same mathematical result: they are all equally successful, at least it is often said that they are.
The question of the intermediate period becomes somewhat more complicated when you realize that the wave function is relevant for an abstract configuration space, not for a fourth dimensional physical space or space-time. This leaves open the possibility that what is happening in the configuration space and in the physical space is different, because during the intermediate period their mutual relevance is indeterminate. What happens in the configuration space is mathematical and not physical. This question of relevance is the foundation of the perceived incompleteness that influences quantum mechanics.
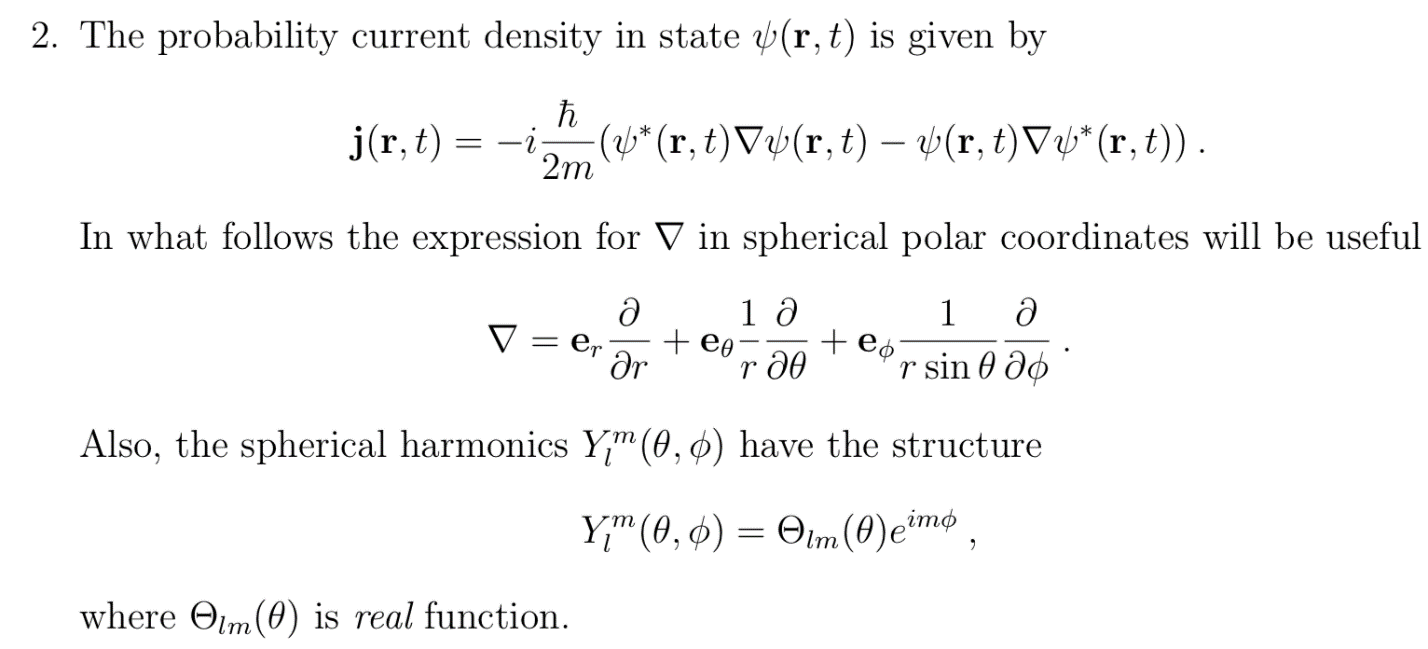
In quantum mechanics, the probability current (sometimes called probability flux) is a mathematical quantity describing the flow of probability in terms of probability per unit time per unit area. ... This is analogous to mass currents in hydrodynamics and electric currents in electromagnetism.
For positive energies, the particle is free to move in either half-space: x < 0 or x > 0. It may be scattered at the delta function potential. For the particle to be reflected. This does not depend on the sign of λ, that is, a barrier has the same probability of reflecting the particle as a well.
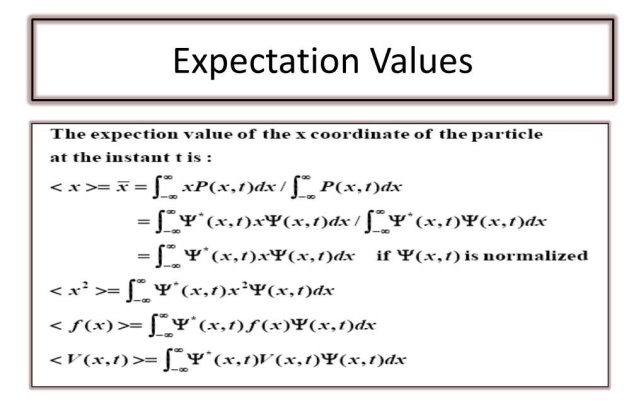
To relate a quantum mechanical calculation to something that can be observed in the laboratory, the "expected value" of the measurable parameter is calculated. For position x, the expected value is defined as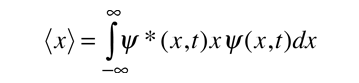
This integral can be read as the regular value of x that we would assume to attain from a large number of dimensions. Alternatively, it could be seen as the average position value for a large number of particles which are described by the same wave function. For example, the expected value of the electron beam in the ground state of the hydrogen atom is the average value that is expected to be obtained when measuring a large number of hydrogen atoms.
While the expected value of a position function looks like an average of the function, the expected value of the moment implies the representation of the moment as a quantum mechanical operator.
Where
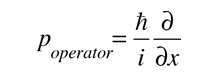
Is the operator for the x section of momentum.
Meanwhile the energy of a free particle is given by
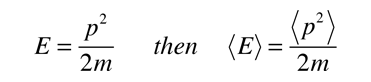
And the expectation value for energy converts
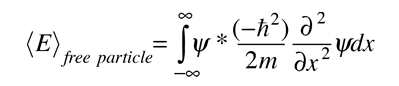
For a particle in one dimension.
In general, the probability value for any visible quantity is establish by putting the quantum mechanical operator for that observable in the integral of the wavefunction over space:
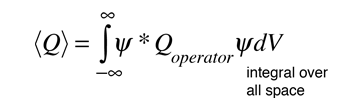
The probability amplitude for a free particle with momentum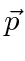 
and
Energy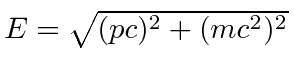 
Is the complex wave function
Note that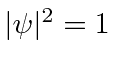 
everywhere so this does not represent a localized particle. In fact we recognize the wave property that, to have exactly one frequency, a wave must be spread out over space.
We can build up localized wave packets that represent single particles by adding up these free particle wave functions (with some coefficients).
(We have moved to one dimension for simplicity.) Similarly we can compute the coefficient for each momentum
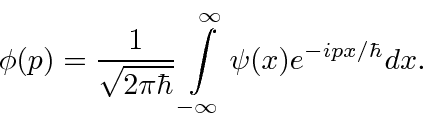
These coefficients,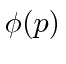 
, are actually the state function of the particle in momentum space. We can describe the state of a particle either in position space with 
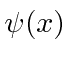or in momentum space with 
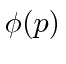. We can use 
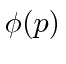to compute the probability distribution function for momentum.
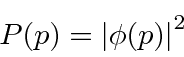
We will show that wave packets like these behave correctly in the classical limit, vindicating the choice we made for
The Heisenberg Uncertainty Principle is a property of waves that we can deduce from our study of localized wave packets.
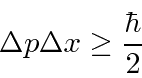
It shows that due to the wave nature of particles, we cannot localize a particle into a small volume without increasing its energy. For example, we can estimate the ground state energy (and the size of) a Hydrogen atom very well from the uncertainty principle.
The next step in building up Quantum Mechanics is to determine how a wave function develops with time - particularly useful if a potential is applied. The differential equation which wave functions must satisfy is called the Schrödinger Equation.
Reference:
1. G. MAIN, “VIBRATIONS AND WAVES IN PHYSICS”, CAMBRIDGE UNIVERSITY PRESS,1993.
2. H. J. PAIN, “THE PHYSICS OF VIBRATIONS AND WAVES”, WILEY, 2006.
3. E. HECHT, “OPTICS”, PEARSON EDUCATION, 2008.
4. A. GHATAK, “OPTICS”, MCGRAW HILL EDUCATION, 2012.
5. O. SVELTO, “PRINCIPLES OF LASERS”, SPRINGER SCIENCE & BUSINESS MEDIA, 2010.
