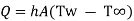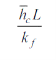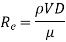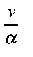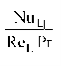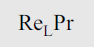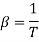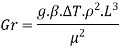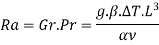2.1 Heat convection and basic equation | unit 2 heat convection

Back to Study material

Unit 2

Heat convection

2.1 Heat convection and basic equation

The process of heat transfer between a solid surface and fluid in motion is called convection.

2.1.1 Types of fluid

Ideal fluid-Fluid which is incompressible and have no viscosity.

Real fluid-Fluid having viscosity.

Newtonian fluid-Real fluid in which shear stress is directly proportional to rate of shear strain.

Non-newtonian fluid- Real fluid in which shear stress is not directly proportional to rate of shear strain.

2.1.2. Laminar and turbulent flow

A flow in which particles move in well defined path or stream line is called laminar flow.

A flow in which fluid move in zigzag motion is called turbulent flow.

Reynold’s number is used to decide whether a flow is laminar or turbulent.

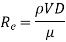
Where 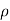=density,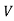=velocity,D=diameter of pipe,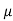=viscosity

Re>2300 flow is turbulent else it is laminar.

2.1.3. Newton’s law of cooling

Rate of convective heat transfer is determined by Newton’s law of cooling.

It states that rate of heat transfer is proportional surface area perpendicular to heat flow direction and temperature difference between wall surface (Tw) and fluid temperature (T∞) in direction perpendicular to flow.

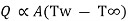
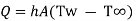...(2.1)

Where, Q is rate of heat transfer (W), A is area in m2, h is constant known as coefficient of convective heat transfer or film coefficient with unit W/m2K.

2.2 External and Internal flow convection:

External flow convection-Convection takes place as fluid flows over body with temperature difference.

Internal flow convection- Convection takes place as fluid flows inside body with temperature difference.

2.3 Forced convection and natural convection:

Natural convection- The convection in which fluid moves due to density difference caused by heat transfer between solid surface and fluid it said to be natural or free convection.

Forced convection-If fluid motion is imparted by external means like pump is called forced convection.

2.4 Boundary Layer concept

2.4.1. Velocity boundary layer

When a fluid flows over a flat plate and its velocity is measured at various points normal to the surface in the immediate vicinity of the wall, a velocity profile is obtained.

The velocity begins with the value zero at the wall and increases within a thin layer of thickness d to the value of free-stream velocity u∞. This distance from the wall 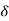is called the boundary layer thickness where there is velocity gradient and above which velocity is uniform and there is no viscous effect.

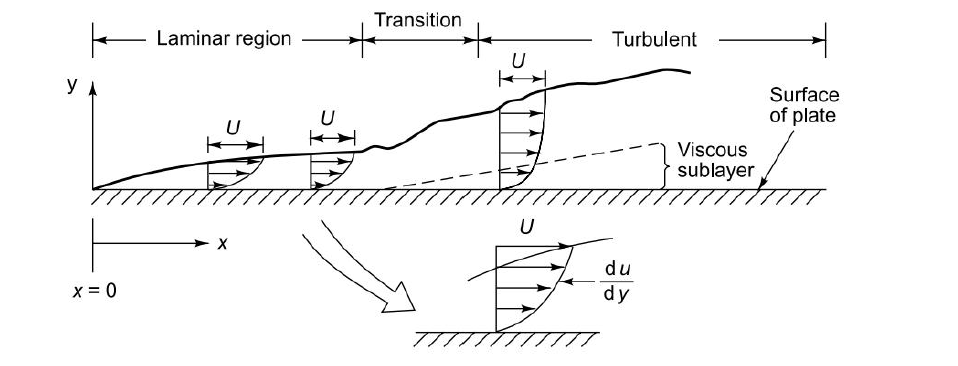
Fig 2.1 Velocity profiles in laminar, transition and turbulent boundary layers in flow over a flat plate

The fluid is moving in the laminar regime, and the boundary layer is thin. This is called laminar boundary layer. As the fluid travels further downstream along the plate, the retardation of fluid flow progresses due to viscous shear, and the boundary layer grows in thickness. As a result the velocity gradient gradually decreases. Meanwhile the boundary shear is reduced as the thick ness increases. When the boundary layer becomes thick enough, the particles begin to move out of the smooth layers, the laminar motion becomes unstable, and finally the flow becomes turbulent.

Thickness of velocity boundary layer is defined as distance from surface at which local velocity is 99% of free stream velocity.

2.4.1 Thermal boundary layer

Analogous to the concept of velocity boundary layer, one can visualize the development of a thermal boundary layer with temperature varying from Tw to 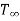in the boundary layer thickness 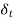. Let us consider that a fluid at a uniform temperature 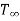flows along a flat plate maintained at a constant temperature Tw. If we define the dimensionless temperature 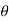(x, y) as

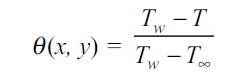
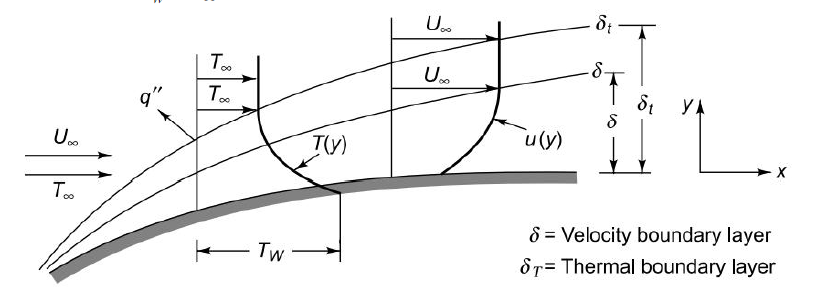
Fig 2.2 Growth of velocity and thermal boundary layers in flow over a flat surface

where T (x, y) is the local temperature in the fluid, then at y = 0, 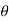= 0 and at y = 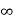, 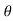= 1. Therefore, at each location x along the plate one finds a location y = 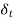(x) in the fluid where q equals 0.99. The locus of such points where 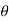= 0.99 is called the thermal boundary layer 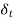(x).

The relative thickness of the thermal boundary layer 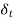(x) and the velocity boundary layer 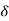(x) depend on the Prandtl number of the fluid.

2.4.2 Velocity boundary layer in circular pipes

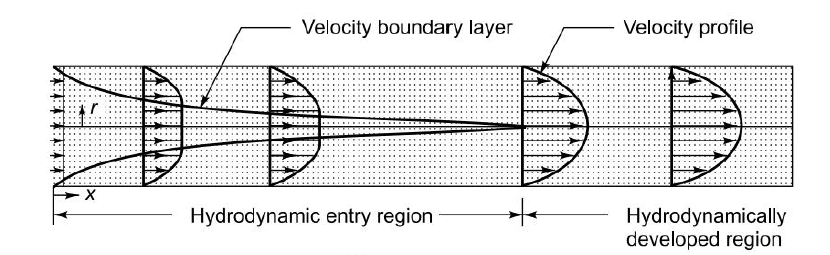
Fig 2.3 Velocity profile in circular pipe

As fluid moves along the pipe, the fluid layer in contact with surface tends to become stationary due to friction between surface of pipe and fluid.

Due to viscous force, stationary layer retards the velocity of second layer toward the center. Second layer retards third and so on.

Thus velocity of fluid keeps on increasing from V=0 to V=Vmax.

The velocity profile becomes parabolic and fully developed and does not change there after till time fluid remains laminar.

The axial distance covered by fluid from entrance upto the point of fully developed velocity profile is called entry length denoted by Le. This region is called entrance zone. Le for laminar flow is given as

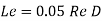
Where Re= Reynold’s number, D= diameter

For turbulent flow

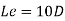
2.4.3 Thermal boundary layer in circular pipes

Consider that a fluid at a uniform temperature enters a circular tube with its wall at a different temperature.

The fluid particles in the layer in contact with the surface of the tube will assume the tube surface or wall temperature Tw. This will initiate convection heat transfer in the tube, and the development of the thermal boundary layer along the tube.

The thickness of this boundary layer also increases in the flow direction until the boundary layer reaches the tube centre and thus fills the entire tube. The region of flow over which the thermal boundary layer develops and reaches the tube centre is called the thermal entry region, and the length of this region is called the thermal entry length (Le )t. Value of (Le )t for laminar flow is given as

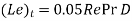
Where Re= Reynold’s number, D= diameter, Pr= Prandtl number

For turbulent flow

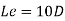
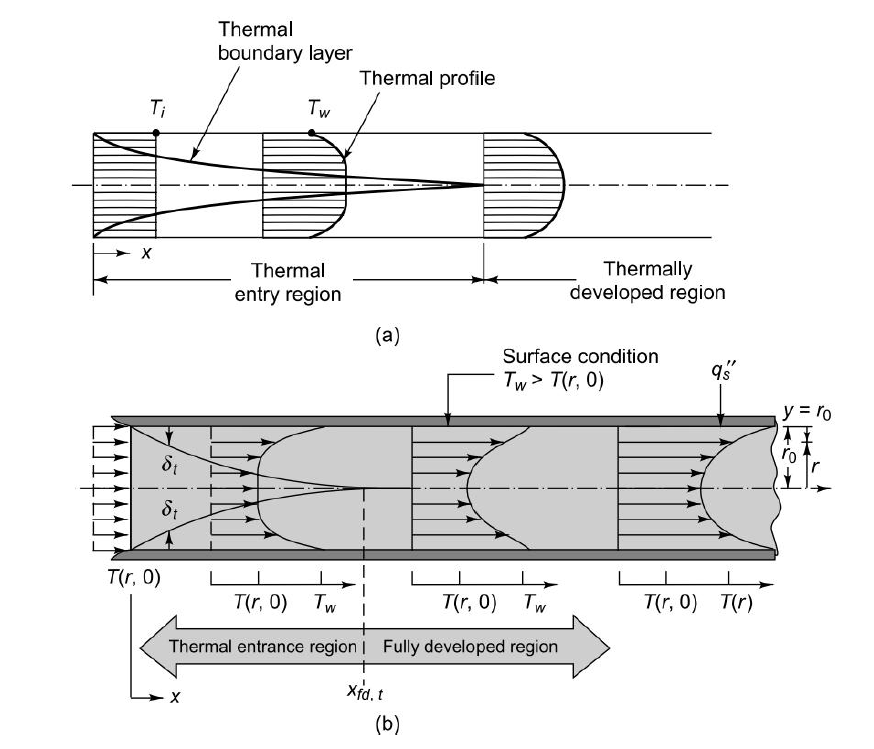
Fig 2.4 Development of thermal boundary layer in a tube (a) when T > Tw and (b) when Tw > T

2.5 Natural convective heat transfer coefficient

There are various methods available for finding out heat transfer coefficient as there are many terms involved as compared to heat conduction. These methods are:

Dimensional analysis:

This method is simple and used widely. Biggest limitation of this method is that it is incomplete and highly dependent on experimental data. Understanding the concept is difficult by the use of dimensional method. It relates dimensionless number with experimental data with the help of correlations.

Exact mathematical method:

This method depends on fluid motion and transfer energy in form of simultaneous equations. This method helps in better understanding of physics behind the concept mathematically. Exact solutions are, however, important because the assumptions made can be specified precisely and their validity can be verified experimentally. High speed computers have made it easy to use this method nowadays.

Approximate analysis of boundary layer:

It avoids use of complex mathematical equations and provides simple solution to equations used to describe thermal and velocity boundary layer. The problem is then analysed on a macroscopic basis by applying the equation of motion and the energy equation to the fluid in the boundary layer. This method is relatively simple and yields solutions within accuracy.

Analogy between heat and momentum transfer:

This method is useful for analyzing turbulent heat convection. One such model explains a mixing motion in a direction perpendicular to the mean flow accounting for the transfer of momentum as well as energy. Experimental results are agree with analytical predictions based on the model.

Numerical method:

These methods are used to solve equations of motion and energy. These methods can be easily programmed in computer. Numerical methods can handle very complex equations.

2.6 Dimensionless parameters and correlations for forced convection

The convection in which fluid moves due to motion imparted by external means like pump is called forced convection.

Convection heat transfer coefficient depends on large number of variables like density, dimension of pipe, viscosity etc. various methods are can be opted to find heat transfer. One of which is dimensionless analysis which deals with usage of characteristic dimensionless numbers.

2.6.1. Dimensionless numbers

Nusselt number(Nu):

Dimensionless heat transfer coefficient. It is ratio of convection heat transfer to conduction in a fluid layer of thickness L.

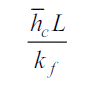
…(2.2)

It measure energy transfer by convection occurring at surface.

If Nu=1, heat transfer is purely by conduction. Larger the value of Nu, larger will be rate of heat transfer by convection.

Reynolds number(Re):

Ratio of inertia to viscous forces.

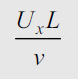
…(2.3)

L=characteristic length which is equal to diameter in pipes. U=velocity and

It characterizes type of flow, whether laminar or turbulent.

Prandtl Number(Pr):

Ratio of molecular momentum diffusivity to thermal diffusivity.

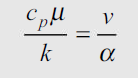
…(2.4)

Cp=specific heat, u=dynamic viscosity, 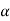=thermal diffusivity.

Higher the Pr higher is Nu and higher is the heat transfer.

Stanton number(St):

It is dimensionless heat transfer coefficient. It is ratio of convective heat flux to heat transfer capacity.

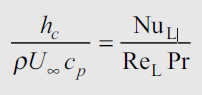
…(2.5)

NuL=Nusselt number, ReL=Reynolds number for liquid, 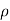=density.

Peclet number(Pe):

It is product of Reynolds and Prandtl numbers.

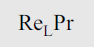
…(2.6)

2.6.2. Correlations for various shapes

Correlation between dimensionless numbers and convective heat transfer coefficient are used to determine heat flow rate.

2.6.2.1. Flow over the plate (External flow)

For laminar flow

Local Nusselt number: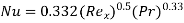

Average Nusselt number: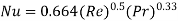

For turbulent flow

Local Nusselt number: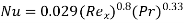

Average Nusselt number: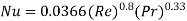

2.6.2.2. Flow through pipes (Internal flow)

For laminar flow

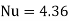(for constant heat flux)

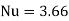(for constant wall temperature)

For turbulent flow

When fluid is heated i.e. :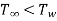

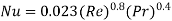
When fluid is cooled i.e.:

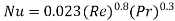
From value of Nusselt number, value of convective heat transfer coefficient can be found out which is then used to find heat transfer rate.

Mean film temperature=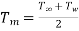

2.6.2.3. Characteristic length

Characteristic length also known as hydraulic diameter is important parameter for analysis of convection. As the geometry of conduit changes in internal flow, this length also changes. It is given as

For circular pipe

For rectangular cross-section

For hollow pipe

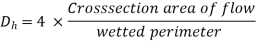
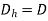
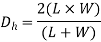
D=Outer diameter, d=inner diameter

2.6.3. Difference between average and local heat transfer coefficient

Local heat transfer coefficient means that it is applicable at given point of solid body, over which fluid flow is taking place. Similarly local heat transfer coefficient applies to that particular point only.

In practice, heat transfer rate would be required to found out over the entire surface hence, average heat transfer coefficient is used.

Average heat transfer coefficient is found out by integrating local heat transfer coefficient over length of entire surface.

2.7 Dimensionless parameters and correlations for natural(free) convection

The convection in which fluid moves due to density difference caused by heat transfer between solid surface and fluid it said to be natural or free convection.

2.7.1. Mechanism of natural convection

When the air comes in contact with hot surface, its molecule in immediate vicinity of surface receives heat from hot surface. This causes temperature of molecules and its volume to increase.

The density of air decrease due to increase in temperature of air adjacent to surface. Thus hot air rises under the action of buoyancy forces.

The place of hot air is taken by heavier molecules which will also rise in similar manner on receiving energy from hot surface.

This way natural motion in molecules is set and hence heat transfer is called natural convection.

2. 7.2. Coefficient of volumetric expansion

The coefficient of volumetric expansion is defined as density of fluid variation per degree change in temperature at constant pressure. It is denoted by 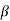and equal to

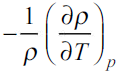
…(2.7)

Negative sign since density decrease with increase in temperature. For ideal gas

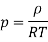
Therefore,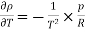

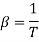
Higher value of 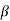higher will be density gradient hence higher heat transfer rate.

2.7.3. Dimensionless numbers

Grashoff number(Gr):

It represents the product of buoyant and inertia forces to the square of viscous force.

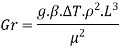
The role of Grashoff number in natural convection is similar to role of Reynolds number in forced convection. It provides criteria to decide whether flow is laminar or turbulent.

Rayleigh number(Ra):

It is defined as product of Grashoff number and Prandtl number.

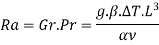
2.7.4. Correlations for various shapes

Vertical plates and cylinders

Nu=0.59(Gr.Pr)0.25 when 104<Gr.Pr<109

Nu=0.13(Gr.Pr)0.33 when 109<Gr.Pr<1012

Horizontal plates

Nu=0.54(Gr.Pr)0.25 when 105<Gr.Pr<2 x 107

Nu=0.15(Gr.Pr)0.33 when 2 x 107<Gr.Pr<3 x 1010

2.8 Comparison between Forced and natural convection

Sr. No.	Forced Convection	Natural convection
1	If movement of molecules occurs due to external force or by using external means like pumps is called forced convection.	If movement of molecules is due to density difference then such type of convection is natural convection.
2	Heat transfer rate is more.	Heat transfer rate is less.
3	External power is required for heat transfer.	No external power is required.
4	Dimensionless numbers used are Reynolds number, Nusselt number, Stanton number, Pecelt number.	Dimensionless numbers used are Grashoff number and Prandtl number.
5	Applications are in heat exchanger.	Application is cooling of tea in atmosphere.

2.9 Approximate solution of laminar boundary layer equation over flat surface

Another method of analysis of convection is approximate solution of momentum and energy equation. To determine the forced convection heat transfer coefficient hc and the friction coefficient Cf for incompressible steady laminar flow over a flat surface, we must satisfy the continuity, momentum and energy equations simultaneously.

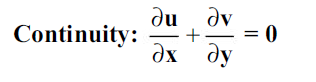
…(2.7)

…(2.8)

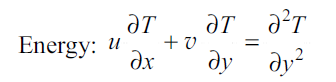
..(2.9)

Equation (2.8) must be solved simultaneously with the continuity equation, (Eq.2.7), in order to determine the velocity distribution, boundary layer thickness and skin friction coefficient. Let us first make an order-of-magnitude analysis of the differential equations to obtain the functional form of the solution. Within the boundary layer we may say that the velocity u is of the order of free-stream velocity 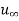. Similarly, the y dimension is of the order of the boundary layer thickness 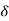. Thus

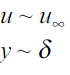
And continuity equation can be approximated as

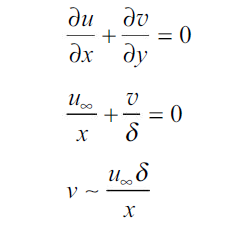
Then, by using this order of magnitude for 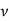, the analysis of momentum equation would yield

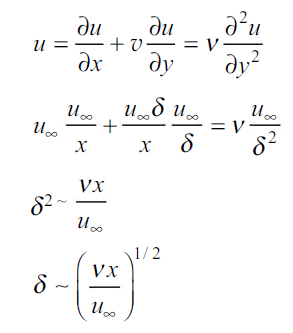
…(2.10)

Dividing by x to express the result in dimensionless form gives

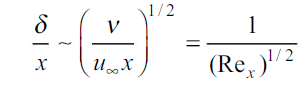
…(2.11)

This is the functional relationship of 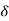with the local Reynolds number. Since the velocity profiles have similar shapes at various distances from the leading edge of the flat plate, the important variable is y/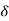, and we assume that the velocity may be expressed as a function of this variable.

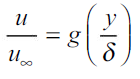
Introducing the order-of-magnitude estimate for 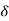from 2.10

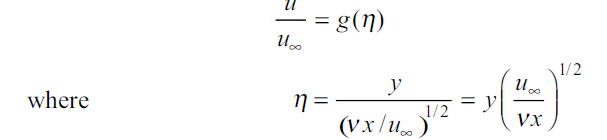
…(2.12)

Where

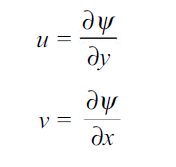
Here, 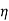is called the similarity variable and 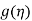is the function for which we seek a solution. In accordance with the continuity equation, a stream function 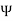may be defined so that

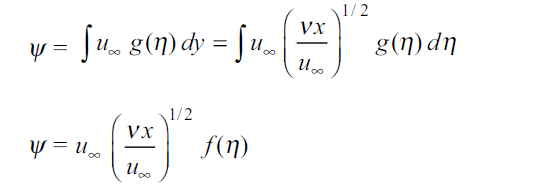
…(2.13)

From 2.13 and 2.12

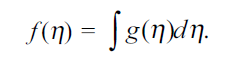
…(2.14)

Where

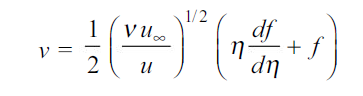
From 2.13 and 2.14

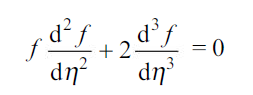
…(2.15)

Expressing 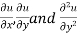in terms of 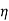and inserting these relations in 2.8

…(2.16)

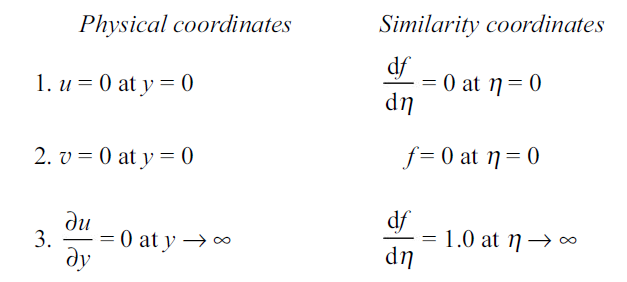
It may be solved numerically for the function f() subject to the three boundary conditions:

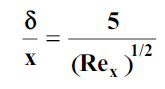
the solution to Eq. (2.16) was first obtained by Blasius

Figure 2.5 shows the Blasius velocity profiles in the laminar boundary layer on a flat plate in dimensionless form together with the experimental data. The ordinate is the ratio of local and free-stream velocities, and the abscissa is a dimensionless distance parameter (y/x) (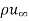x/ m)1/2. The velocity u reaches 99% of the free-stream velocity 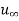at (y/x) (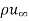x/m)1/2 = 5.0. If we define the hydrodynamic boundary layer thickness d as that distance from the surface at which the local velocity u reaches 99% of the free-stream velocity 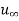, then

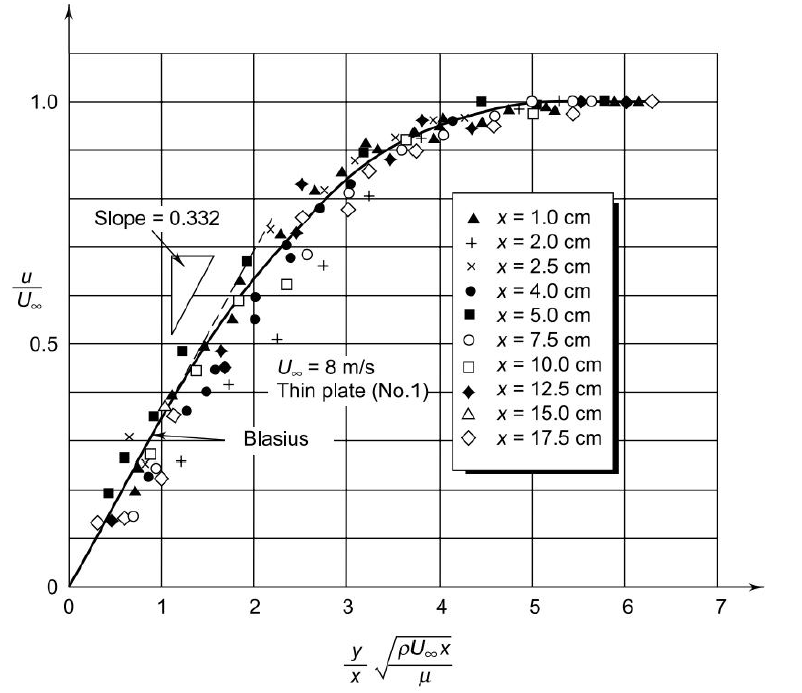
…(2.17)

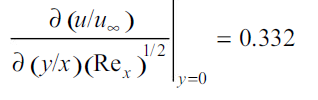
Fig 2.5 Velocity profile in a laminar boundary layer according to Blasius, with the experimental data

The shear stress at the wall can be obtained from the velocity gradient at y = 0 in Fig. 2.5. We find that

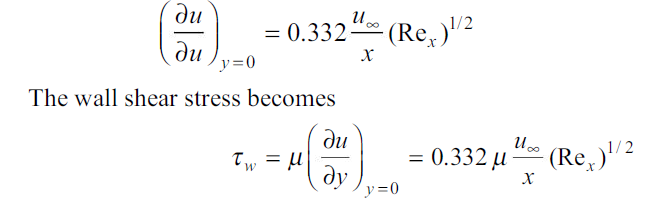
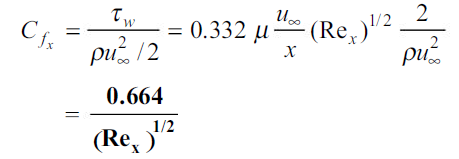
…(2.18)

It may be noted that the wall shear stress at the leading edge (x = 0) is very large, and it decreases with increasing distance (x) from the leading edge. Dividing both sides of Eq. (2.18) by the dynamic pressure of the free-stream, 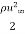, we obtain

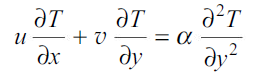
…(2.19)

where Cfx is the dimensionless local drag or friction coefficient.

Convective heat transfer coefficient:

The velocities u and v in the energy conservation equation

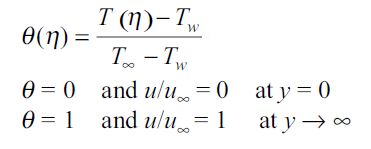
have the same values at any point (x, y) as in the momentum equation, Eq. (2.8). For the case of the flat plate, Pohlhausen used the velocities calculated previously by Blasius to obtain the solution for the heat transfer problem. If the momentum and energy equations are compared, we find them to be similar if 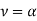and the surface temperature Tw is constant. A solution for u (x, y) is also a solution for T (x, y) which can easily be checked if the symbol T is replaced by the symbol u while the boundary conditions for both are identical. If we define a dimensionless temperature

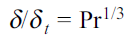
where Tw is the wall or surface temperature and is the free–stream temperature. The condition 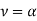corresponds to a Prandtl number (=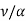) of unity. For Pr = 1, the velocity distribution is identical to the temperature distribution. The transfer of momentum is analogous to the transfer of heat when Pr = 1. For gases Prandtl number ranges from 0.6 to 1.0. The analogy is, therefore, satisfactory for gases. Liquids, however, have Prandtl numbers considerably different from unity, and the preceding analysis is not applicable directly to liquids.

We define a thermal boundary layer thickness d t as the distance from the wall at which the temperature difference between the wall and the fluid 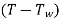reaches 99% of the free-stream value 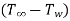. From the temperature profi les, we observe that for Pr < 1, 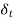> 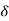, and for fluids having Pr > 1, 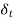< 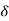. According to Pohlhausen,

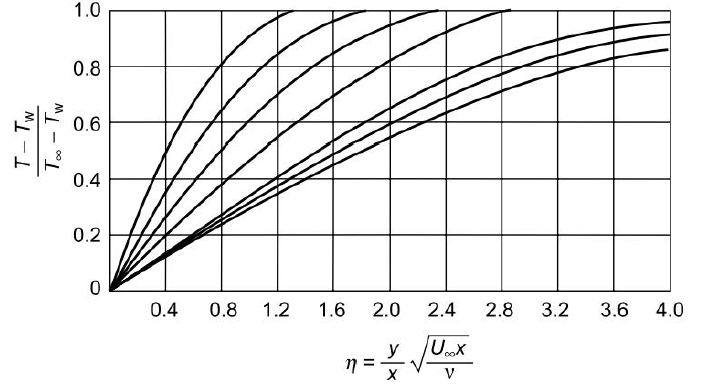
Fig2.6 Temperature distributions in a fluid flowing over a heated plate for various Prandtl numbers

The curves of Fig. 2.6 are replotted in Fig. 2.7 using the correction factor Pr1/3 in the abscissa, which is now (y/x) (Rex)1/2 Pr1/3. The dimensionless temperature gradient at the surface ( y = 0) is

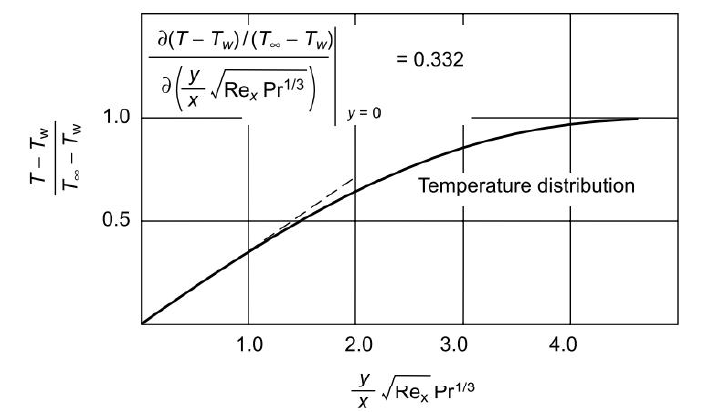
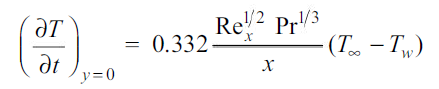
Fig 2.7 Temperature distribution for laminar flow over a heated plate at uniform temperature

Therefore, at any specified value of x,

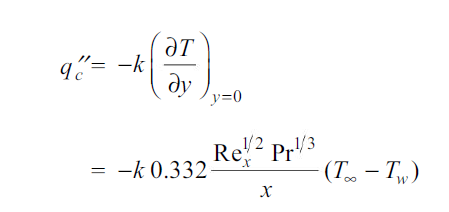
The local rate of convection heat transfer per unit area becomes

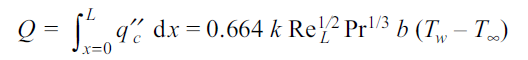
…(2.20)

The total rate of heat transfer from a plate of width b and length L is

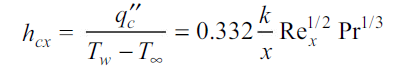
The local heat transfer coefficient obtained from Eq. (2.20),

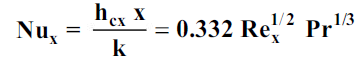
The local Nusselt number is

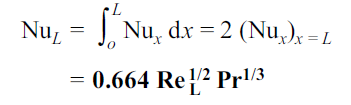
The average Nusselt number over the entire plate of length L is

2.10 Estimating heat transfer rates in laminar and turbulent flow situations using appropriate correlations for forced and free convection

Laminar flow over flat plate:

Average friction coefficient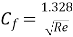

Local friction coefficient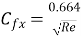

Thickness of boundary layer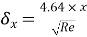

Thickness of thermal boundary layer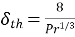

Turbulent flow over plate:

Average friction coefficient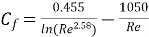

Local friction coefficient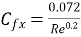

Thickness of boundary layer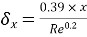

2.11 Important Formulae

Heat transfer convection transfer
Nusselt number
Reynolds number
Prandtl number
Stanton number
Peclet number
Coefficient of volumetric expansion
Grashoff number
Rayleigh number

Sign Up

Index

Notes

Highlighted

Underlined

Browse by Topics

Notes

Highlighted

Underlined