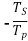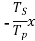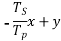Unit 4

Gear

4.1 Gear

Gear can be defined as the toothed member that is used to transmit the power from one shaft to another shaft. Gear transmits the motion by direct contact. Among the two meshing gears smaller one is known as the pinion while larger one is known as wheel or gear. Gears are broadly classified on the basis of layout of connecting shaft.

Table.1. Classification of Gear

Gear Terminology

Gear terminology is the various terms to study the gear which is being discussed below and figure 1 show the various terms used in gear terminology.

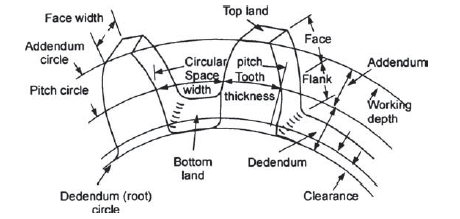
Figure.1. Gear Terminology

Pitch Circle: It is an imaginary circle which transmits the same motion by pure rolling as the transmitted by the gears in mesh.

Pitch Circle Diameter: Diameter of pitch circle is known as the pitch circle diameter.

Circular pitch: Circular pitch is the distance of a point on one tooth to the corresponding point on the adjacent tooth along the pitch circle. It is denoted by the p.

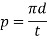
t = number of teeth.

d = pitch circle diameter.

Diametral Pitch: Diametral pitch of a gear is the number of teeth per unit length of pitch circle diameter in inches. It is denoted by P.

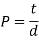
Module: it is the ratio of pitch circle diameter in mm to the number of teeth on the gear. it is denoted by m.

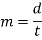
Gear Ratio: Gear ratio is the ratio of number of teeth on driven gear to the number of teeth on driving gear. it is denoted by G.

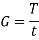
T= number of teeth on driven gear.

t = number of teeth on driving gear.

Velocity ratio: It is the ratio of angular velocity of driven gear to the angular velocity of driving gear. It is denoted by VR.

Let N be the rpm,  be the angular velocity and T be the number of teeth on gear. while subscript 1 and 2 are used for the driving and driven gears respectively.

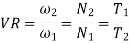
Addendum circle: It is the circle passing through the tip of the gear tooth.

Addendum: It is the height of the gear tooth in radial direction above the pitch circle. Standard value of addendum is one module.

Dedendum Circle: The circle passing through the root of circle is known as the dedendum circle.

Dedendum: It is the depth of gear tooth along the radial direction below the pitch circle. Its standard value is 1.157 modules.

Clearance: It is the difference between the addendum and dedendum of the gear. Standard value of clearance is 0.157m.

Full depth of teeth: It is the total radial depth of the gear tooth. It is calculated by adding the addendum and dedendum of the gear tooth.

Working depth of teeth: It the maximum depth up to which tooth of one gear penetrate in the mating gear. It is calculated by adding the addendum of the mating gears.

Space width: It is the width of space between the two adjacent teeth of a gear along the pitch circle.

Tooth thickness: It is the thickness of the gear tooth measured along the pitch circle.

Backlash: Backlash is defined as the difference between the tooth thickness and the space width.

Face width: It is the width of the gear tooth measured along the axis of gear.

Top land: Top surface of the gear tooth.

Bottom land: The bottom surface of the tooth between the adjacent fillets.

Face: It the surface of tooth between the pitch circle and top land.

Flank: It the surface of tooth between the pitch circle and the bottom land which also includes the fillet.

Line of action: It is the line joining the pitch point to the point of contact of two mating gear along which the driving gear exerts force on the driven gear. It is also known as the pressure line.

Pressure angle: It is the angle between the common tangent to the pitch circle and the line of action. It is also known as angle of obliquity and denoted by .

Path of contact: It is the locus of point of contact of two mating gears from beginning of contact up to disengagement of the gears. It constitutes of following two paths.

Path of approach: It is the locus of point of contact from starting of engagement to pitch point.

Path of recess: It is the locus of point of contact from pitch point to the end of engagement.

Arch of contact: It is the locus of point of contact along pitch circle form beginning of engagement to end of engagement. Just like path of contact it can also be divided in two parts.

Arc of Approach: It is the part of arc of contact from beginning of engagement to pitch point.

Arc of Recess: It is the part of arc of contact from pitch point to end of engagement.

Angle of action: It is the angle turned by the pair of teeth of gears from beginning of engagement to the end of engagement.

Contact ratio: It is the ration of arc of contact to the circular pitch.

Involute Gear Profile

Involute profile is obtained by the locus of a point on a straight line when it rolls about a circumference of a circle without slipping. The circle about which the straight-line rolls is known as the base circle. Figure 2 shows the generation of involute profile in which the line BD is rolling about the base circle with center O and the path ABC shows the involute profile traced by the line.                           

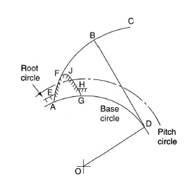
figure 2 Involute profile

Cycloidal Profile

Cycloidal profile is obtained by the locus of a point on the circumference of a circle when it rolls on a straight line without slipping. Gear tooth are made by two kind of cycloidal profiles. Face of gear tooth have epicycloid profile while flank have hypocycloid profile. Figure 3 shows the cycloidal profile of gear teeth.

Epicycloid profile is obtained by the locus of a point on the circumference of a circle when it rolls on the circumference of another circle without slipping, while hypocycloid profile is obtained by the locus of a point the circumference of a circle when it rolls inside the circumference of another circle.                             

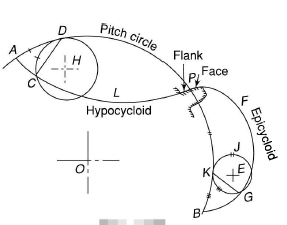
Figure 3 Cycloidal Profile of gear teeth

Gear Parameters

Gear parameters listed below.

Path of contact

In order to calculate the path of contact of gear consider the figure 4 which shows the two gears in contact. In which smaller gear is termed as the pinion while lager gear is known as the wheel.

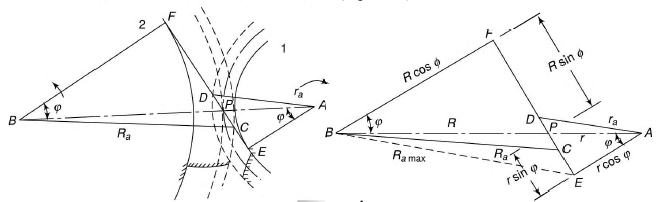
Figure 4 Two gears in contact

Path of contact is given by the line CD shown in the figure 4. In the given figure:

R = radius of pitch circle for the wheel

r = radius of pitch circle for pinion

Ra = radius of addendum circle for wheel

ra = radius of addendum circle for pinion

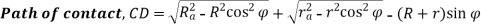
Arc of contact

Arc of contact is the path on the pitch circle of pinion and the wheel from start of their engagement to the end of the disengagement. The arc of contact is calculated by the given formula.

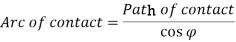
Contact ratio

It is also known as the number of pairs of teeth in contact during meshing of a pair of gears. During the meshing of teeth all the teeth lying in between the arc of contact are the number of pairs of teeth in contact. We can define the contact ratio as the ratio of arc of contact to the circular pitch.

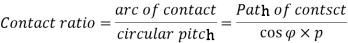
Fundamental Law of Gearing

This law states the necessary condition for the constant angular velocity for two mating gears i.e. the common normal at the point of contact of the gears in mesh must pass through the pitch point, and the ration in which the pitch point divides the common normal is the inverse ratio of the angular velocity of the mating gears.

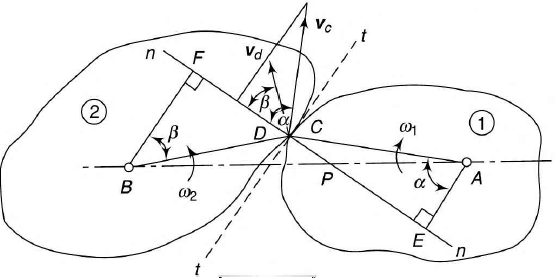
Figure.5. Gear teeth profile in mesh

Consider the figure.5 in which point C and D are the contact point on gear 1 and 2 respectively, line n-n is the common normal at the point of contact and point P is the pitch point. So, the necessary condition for the constant angular velocity is:

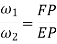
Where, 1= angular velocity of gear 1.

2= angular velocity of gear 2.

Conjugate Action

If the meshing gears are shaped in such a way that they produce a constant angular velocity ratio, such surfaces are termed as conjugate surfaces. This act of producing constant angular velocity ratio by two meshing gear is known as conjugate action.

Interference/Undercutting

Locking of meshed gear teeth due non-conjugate or non-involute profile is termed as the interference. Non-conjugate gear teeth profile causes improper sliding of gear teeth which results in rough action and binding. Rough action and binding cause different sliding velocity of mating gears and results in locking.

Minimum number of teeth to avoid interference

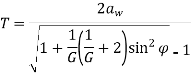
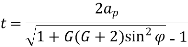
Undercutting

In case of involute gear profile addendum of mating gears removes some part in the dedendum if there is interference. This removed portion in the dedendum is known as the undercut and the process of removal of the material from at the root of gears is known as undercutting. Figure 6 shows the undercutting in the gears.

4.2 Gear Train

Gear train is the drive which uses the combination of gears to transmit the motion from one shaft to another shaft. These drives are used where high-speed reduction is required at limited space. Different kinds of gear trains used are:

Simple gear train

A simple gear train is the combination of gears in which only one gear is fixed on one shaft. in these gear trains two mating pairs rotate in opposite direction. If there are more than two gears in the gear train then even number gears rotate in one direction and odd number gears rotate in same direction but opposite to even number gears. Figure 7 shows the arrangement of gears in simple gear train.

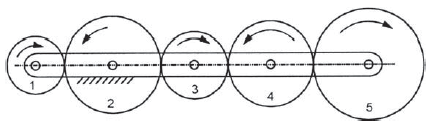
Figure.7. Arrangement of gears in simple gear train

In the analysis of a gear train we try to find out the train value which is defined as the ratio of speed of speed of driven gear to the speed of driving gear. The train value is used to calculate the angular velocity transmitted by the gear train.

For simple gear train shown in figure 7, N represents the angular velocity of each gear followed by its number in subscript, similarly T represents the number of teeth on each gear.

In case of gears in mesh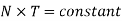 

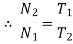
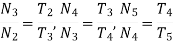
By multiplying all the above equations

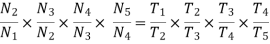
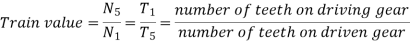
Compound gear train

These are the gear trains in which one shaft contains more than one gears. Consider the gear train shown in figure 8 which shows the arrangement of gears in a compound gear train. In the given compound gear train gear 1 is driving gear, gear 2 and 3 are mounted on same shaft.

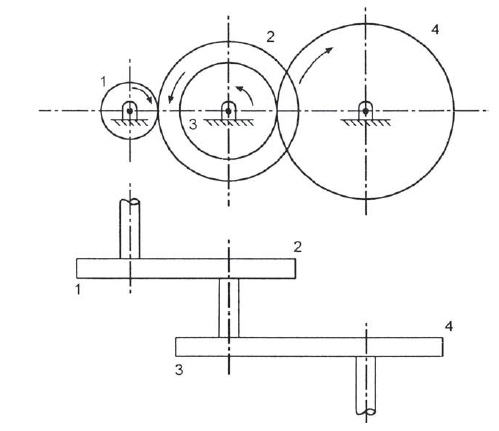
Figure.8. Compound gear train

For the given gear train

Multiplying all the above equations

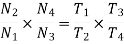
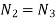
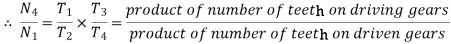
Reverted gear train

These are the gear train are special types of compound gear trains, in which axis of first and last gears are same. These kind of gear trains are used in clocks. Figure 9 shows the reverted gear train in which the axis of 1st and 4th gear are coinciding while gear 2 and 3 comprises a compound gear.

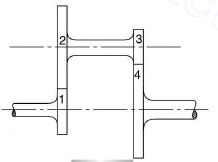
Figure.9. Reverted gear train

For the gear train shown in figure 9

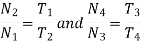
Multiplying all the above equations

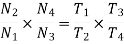
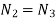
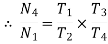
And

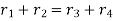
Epicyclic gear train

Apart from rotation of gears if any gear axis is also rotating with respect t some other axis the gear train is termed as epicyclic gear train.To rotate the axis of gear a very important link is used which is known as arm or carrier which is not gear.

The epicyclic great train shown in figure 10 has gears S and P and the arm a. The gear S and P are in mesh are rotating about their own axis with the help on arm a, axis of both the gear are rotating with respect to axis of each other. The degree of freedom for the epicyclic gear train is 2.

Consider the number of teeth on S and P gears are Ts and Tp respectively. In order to analyze the epicyclic gear train shown in figure 10 let us assume clockwise rotation is taken as positive and counter clockwise rotation as negative and now follow the given steps and prepare the table 1 shown below.

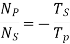
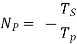
3.     Now multiply the rpm of each component by x.

4.     At last add the rpm of arm which is assumed to be y.

5.     Then put the rpm of each component equal to the condition given in question and solve the problem accordingly.

Table.1. Analysis table for epicyclic gear train

Now substitute the rpms of given component according the condition given in question and solve.

Reference Books

1. Thomas Bevan, Theory of Machines, 3rd edition, CBS Publishers & Distributors, 2005.

2. CleghornW.L. , Mechanisms of Machines, Oxford University Press, 2005.

3. Robert L. Norton, Kinematics and Dynamics of Machinery, Tata McGraw Hill, 2009.

4. Ghosh A. and Mallick A.K., Theory of Mechanisms and Machines, Affiliated East West Pvt. Ltd, New Delhi, 1988.