Unit-2
Design of Shaft
Shaft is a rotating machine element which is used to transmit power from one place to another. In order to transfer the power from one shaft to another, the various member such as pulleys, gears etc.
It is the machine members, mostly cylindrical in cross-section, which support the revolving parts of a machine, such as pulleys, gear, flywheels etc
The following stresses are induced in the shafts:
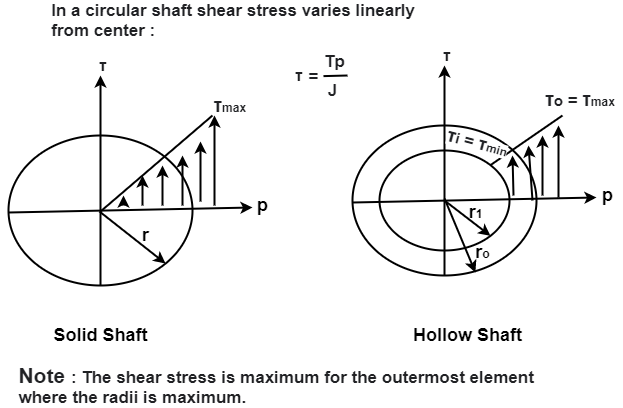
Figure 1: stresses generated in shaft
Key takeaways
Torsional load, bending load and torsional-bending load are the kinds of stresses that is exerted on the shaft.
The static load safety factor is calculated by dividing the basic static load rating (C0) by the static equivalent load of the most heavily loaded bearing.
When the shaft is subjected to a twisting moment (or torque) only, then the diameter of the shaft only may be obtained by using the torsion equation. We know that T/J=Ϡ/r……………………………..(i)
Where, T= twisting moment acting on the shaft
J=Polar moment of inertia
Ϡ= Torsional shear stress
R= distance from neutral axis to the outermost fibre
=d/2, where d is the diameter of shaft
When the shaft is subjected to combination of torque and bending moment, principal stresses are calculated and then different theories of failure are used. Bending stress and torsional shear stress can be calculated using the above relations.
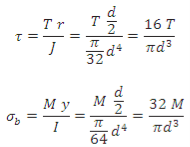
Maximum Shear Stress Theory
Maximum shear stress is given by,
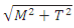is called equivalent torque, Te, such that
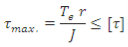
Maximum Principal Stress Theory
Maximum principal stress is given by,
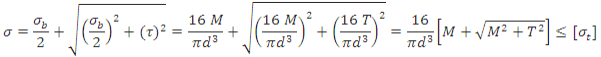
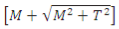is called equivalent bending moment, Me, such that
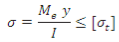
Key takeaways
Maximum shear stress theory and maximum principle stress theory is the major stress which acts in combination while designing of a shaft.
When shaft rotates, bending stress changes from tensile to compressive and then compressive to tensile, ie, completely reversing state of stress. Fa will give rise to normal axial stress in the shaft.
The driving forces and the driven resistances result in bending of the shaft and, for helical gearing, an axial loading is also produced. These loadings generate both normal and shear stresses in the shaft. The torsion loading produces a maximum shear stress at the shaft surface calculated from. fs=T rJ.
A shaft is a part of a machine or system of machines, and is used to transmit power by virtue of its torsional strength, or resistance to twisting. Shafts are almost always made of metal and are usually circular in cross-section, being sometimes made hollow.
Shaft design based on the deflection:
Maximum bending stress developed in a shaft is given by,
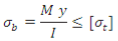
where M = Bending Moment acting upon the shaft,
I = Moment of inertia of cross-sectional area of the shaft about the axis of rotation
= 
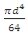for solid shafts with diameter d
= 
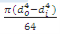for hollow shafts with do and di as outer and inner diameter.
y = Distance from neutral axis to the outer most fibre = d / 2 (or do/2)
So dimensions of the shaft subjected to bending moment can be determined from above relation for a known value of allowable tensile stress.
Design of shaft on the strength basis:
Transmission shafts are subjected to axial tensile force, bending moment or torsional moment or their combination.
Most of the transmission shafts are combined bending and torsional moment. The design of transmission shafts means finding diameter or inner or outer diameter in case of hollow shaft from the strength and rigidity consideration. Design of shaft subjected to pure axial load.
Let P be the axial load
d = required diameter of the shaft
σt=permissible tensile load
σt= P/A=(P)/(πd2/4)
or, σt=(4P)/(πd2)
or, d=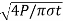
for hollow shaft
σt=(P)/(π(do2- di2/4)
Shaft subjected to pure bending moment
σb=Mby/I
σb=32Mb/πd3
For hollow shaft
I=(π(do4- di4)/64
Shaft subjected to pure torsional moment, the torsional shear stress is given by
Ϡ=Tr/J
For solid shaft
It is, 16T/πd3
In case of hollow shaft
J=(π(do4- di4)/32
The critical speed is the theoretical angular velocity which excites the natural frequency of a rotating object, such as a shaft. As the speed of rotation approaches the objects natural frequency, the object begins to resonate which dramatically increases systemic vibration.
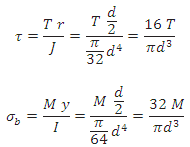
Figure 2: Stationary and rotating shafts
For a rotating shaft if the centripetal force is equal to the elastic restoring force, the deflection increases greatly and the shaft is said to "whirl”
Below and above this speed this effect is not pronounced
This critical (whirling speed) is dependent on: –
The factor which affects the critical speed of a shaft is diameter of the disc, span of the shaft, and eccentricity.
For linear motion:
Power = F.v (force x velocity)
For rotational motion
Power P = Torque x angular velocity
= T (in-lb).w (rad/sec) in-lb/sec
= T.(2 p n/60) in-lb/sec [n=rpm]
= T.(2 p n/(60*12*550)) HP [HP=550 ft-lb/sec]
= T.n/63,025 HP
or, T= 63,025HP/n (in-lb), where n = rpm
Similarly, T= 9,550,000kW/n (N-mm), where n = rpm
Sliding contact bearing: In this bearing, the sliding action takes place along the surface of contact between the moving element and fixed element. The sliding contact bearing is known as plain bearing.
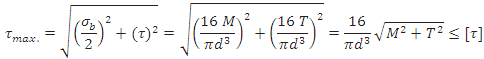
Figure 3: Sliding contact bearing
Rolling contact bearing: In this type of bearing, steel balls or rollers are interposed between the working surfaces. These bearings are also known as anti-friction bearings due to less friction imposed on moving element.
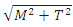
Figure 4: Roller contact bearing
In rolling contact bearings, the contact between the bearing surfaces is rolling instead of sliding as in sliding contact bearings. Sliding contact bearing starts from rest with practically metal-to-metal contact and has a high coefficient of friction.
Desirable properties of material for designing a bearing:
Bearing design selection parameters:
b. Unit bearing pressure:
2.8 Start up load:
c. Radial clearance (c):
d. Minimum oil film thickness (h0 ) :
e. Maximum oil film temperature:
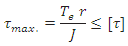
Reference:
