Module 4
Analysis of Gyroscopic Motion
A gyroscope is a device used for measuring or maintaining orientation, based on the principle of conservation of angular momentum. It is a spinning wheel or disc in which the axis of rotation is free to assume any rotation by it.
Any spinning object exhibits gyroscopic properties. A gyroscope is a spinning wheel (or rotor) mounted in a special frame (gimbal) so that its axis is unrestrained in one or more planes. Its properties are useful in indicating changes to direction and attitude.
There are two fundamental properties of gyroscopic action
Rigidity in space: Rigidity in space refers to the principle that a gyroscope remains in a fixed position in the plane in which it is spinning.
Precession: Precession is the tilting or turning of a gyro in response to a deflective force. The reaction to this force does not occur at the point at which it was applied; rather, it occurs at a point that is 90° later in the direction of rotation. This principle allows the gyro to determine a rate of turn by sensing the amount of pressure created by a change in direction.
Whenever a body is rotating in a plane (plane YZ) about an axis (axis OX) and a couple is applied on the rotating body across the axis of rotation or spin in another perpendicular plane (plane XY), the rotating or spinning body starts processing in a third mutually perpendicular plane (plane XZ).
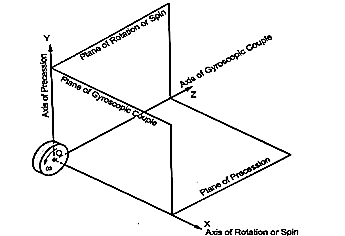
Figure1: Principle of gyroscope
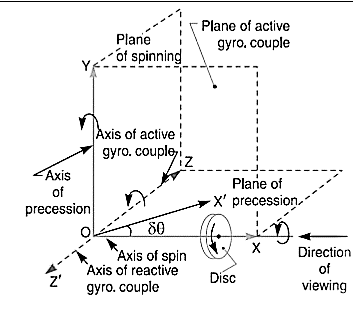
Figure 2: Gyroscopic Couple
Consider a disc spinning with an angular velocity 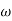 rad/s about the axis of spin OX, inanticlockwise direction when seen from the front, as shown in Fig.2. Since the plane in which the disc is rotating is parallel to the plane YOZ, therefore it is called plane of spinning. The plane XOZ is a horizontal plane and the axis of spin rotates in a plane parallel to the horizontal plane about an axis OY. In other words, the axis of spin is said to be rotating or processing about an axis OY. In other words, the axis of spin is said to be rotating or processing about an axis OY (which is perpendicular to both the axes OX and OZ) at an angular velocity
rad/s about the axis of spin OX, inanticlockwise direction when seen from the front, as shown in Fig.2. Since the plane in which the disc is rotating is parallel to the plane YOZ, therefore it is called plane of spinning. The plane XOZ is a horizontal plane and the axis of spin rotates in a plane parallel to the horizontal plane about an axis OY. In other words, the axis of spin is said to be rotating or processing about an axis OY. In other words, the axis of spin is said to be rotating or processing about an axis OY (which is perpendicular to both the axes OX and OZ) at an angular velocity 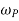 rap/s. This horizontal plane XOZ is calledplane of precession and OY is the axis of precession.
rap/s. This horizontal plane XOZ is calledplane of precession and OY is the axis of precession.
Let, I = Mass moment of inertia of the disc about OX, and
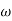 = Angular velocity of the disc.
= Angular velocity of the disc.
Angular momentum of the disc= I.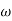
Let the axis OX is turned in the plane XOZ through a small angle 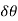 radians to the position OX
radians to the position OX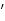 , in time
, in time 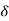 t seconds. Assuming the angular velocity
t seconds. Assuming the angular velocity 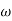 to be constant, theangular momentum will now be represented by vector ox
to be constant, theangular momentum will now be represented by vector ox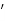 .
.
Change in angular momentum = 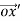 - ox =
- ox =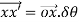 ...(in the direction of
...(in the direction of 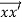 )
)
= I.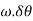
and rate of change of angular momentum = I.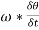
Since the rate of change of angular momentum will result by the application of a couple to the disc, therefore the couple applied to the disc causing precession,
C = Lt δt->0 I.w x δ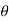 /δt = I.w x d
/δt = I.w x d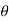 /dt = I.w.wp since d
/dt = I.w.wp since d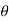 /dt = wp
/dt = wp
Where 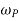 = Angular velocity of precession of theaxis of spin or the speed of rotation of the axis ofspin about the axis of precession OY.
= Angular velocity of precession of theaxis of spin or the speed of rotation of the axis ofspin about the axis of precession OY.
In S.I. units, the units of ‘C’ is N-m when I is in kg-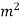 .
.
Key takeaway:
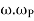
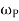
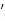
Gyroscopic effects on the movements of aeroplanes, ships, Two wheel and four wheeled vehicles
Let engine or propeller rotates in the clockwise direction when seen from the rear or tail end and the aeroplane takes a turn to the left.
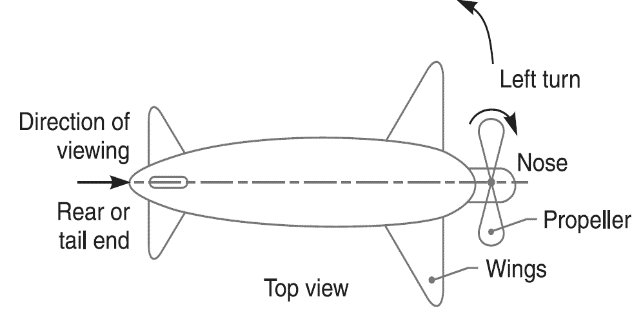
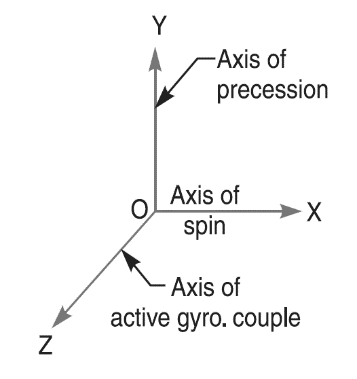
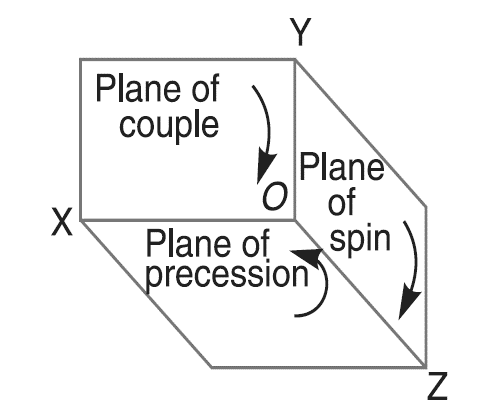
Figure 3: Airplane taking a left turn
Let 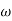 = Angular velocity of the engine in rad/s,
= Angular velocity of the engine in rad/s,
m = Mass of the engine and the propeller in kg,
k = Its radius of gyration in meters,
I = Mass moment of inertia of the engine and the propeller in kg-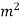
= m.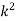 ,
,
v = Linear velocity of the aeroplane in m/s,
R = Radius of curvature in meters, and
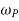 = Angular velocity of precession=
= Angular velocity of precession= rad/s
rad/s
i.e., Gyroscopic couple acting on the aeroplane,
C = I.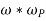
Before taking the left turn, the angular momentum vector is represented by ox. When it takes left turn, the active gyroscopic couple will change the direction of the angular momentum vector from ox to ox as shown in Fig.(a). The vector xx
as shown in Fig.(a). The vector xx , in the limit, represents the change of angular momentum or the active gyroscopic couple and is perpendicular to ox. Thus, the plane of active gyroscopic couple XOY will be perpendicular to xx
, in the limit, represents the change of angular momentum or the active gyroscopic couple and is perpendicular to ox. Thus, the plane of active gyroscopic couple XOY will be perpendicular to xx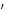 , i.e., vertical in this case, as shown in Fig.(b). By applying right hand screw rule to vector xx
, i.e., vertical in this case, as shown in Fig.(b). By applying right hand screw rule to vector xx , we find that the direction of active gyroscopiccouple is clockwise as shown in the front view of Fig.(a). In other words, for left hand turning, the active gyroscopic couple on the aeroplane in the axis OZ will be clockwise as shown in Fig.(b). The reactive gyroscopic couple (equal in magnitude of active gyroscopic couple) will act in the opposite direction (i.e. in the anticlockwise direction) and the effect of this couple is, therefore, to raise the nose and dip the tail of the aeroplane.
, we find that the direction of active gyroscopiccouple is clockwise as shown in the front view of Fig.(a). In other words, for left hand turning, the active gyroscopic couple on the aeroplane in the axis OZ will be clockwise as shown in Fig.(b). The reactive gyroscopic couple (equal in magnitude of active gyroscopic couple) will act in the opposite direction (i.e. in the anticlockwise direction) and the effect of this couple is, therefore, to raise the nose and dip the tail of the aeroplane.
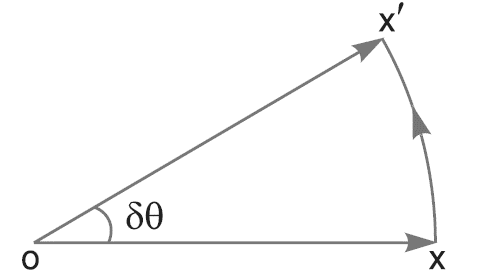
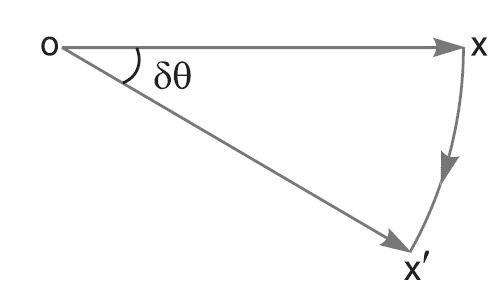
(a) Airplane taking left turn (b) Airplane taking right turn
Figure 4: Effect of gyroscopic couple on an aeroplane
Key takeaway:
1. When the aeroplane takes a right turn under similar conditions as discussed above, the effect of the reactive gyroscopic couple will be to dip the nose and raise the tail of the aeroplane.
2. When the engine or propeller rotates in anticlockwise direction when viewed from the rear or tailend and the aeroplane takes a left turn, then the effect of reactive gyroscopic couple will be to dip the nose andraise the tail of the aeroplane.
3. When the aeroplane takes a right turn under similar conditions as mentioned in point 2 above, theeffect of reactive gyroscopic couple will be to raise the nose and dip the tail of the aeroplane.
4. When the engine or propeller rotates in clockwise direction when viewed from the front and theaeroplane takes a left turn, then the effect of reactive gyroscopic couple will be to raise the tail and dip the noseof the aeroplane.
5. When the aeroplane takes a right turn under similar conditions as mentioned in point 4-above, theeffect of reactive gyroscopic couple will be to raise the nose and dip the tail of the aeroplane.
Steering:
Steering is the turning of a complete ship in a curve towards left or right, while it moves forward.
Consider the ship taking a left turn, and rotor rotates in the clockwise direction when viewed from the stern.
When the rotor of the ship rotates in the clockwise direction when viewed from the stern, it will have its angular momentum vector in the direction ox as shown in Fig.(a). As the ship steers to the left, the active gyroscopic couple will change the angular momentum vector from ox to ox . Thevector xx
. Thevector xx now represents the active gyroscopic couple and is perpendicular to ox. Thus, the plane ofactive gyroscopic couple is perpendicular to xx
now represents the active gyroscopic couple and is perpendicular to ox. Thus, the plane ofactive gyroscopic couple is perpendicular to xx and its direction in the axis OZ for left hand turn is
and its direction in the axis OZ for left hand turn is
Clockwise as shown. The reactive gyroscopic couple of the same magnitude will act in the opposite direction (i.e., in anticlockwise direction). The effect of this reactive gyroscopic couple is toraise the bow and lowers the stern.
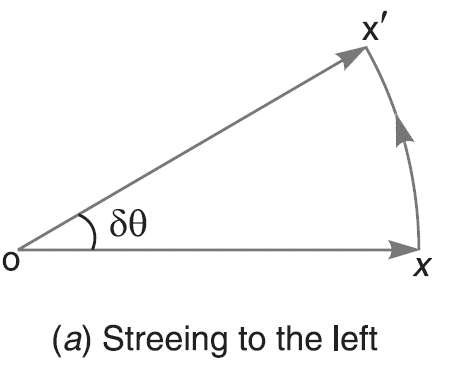
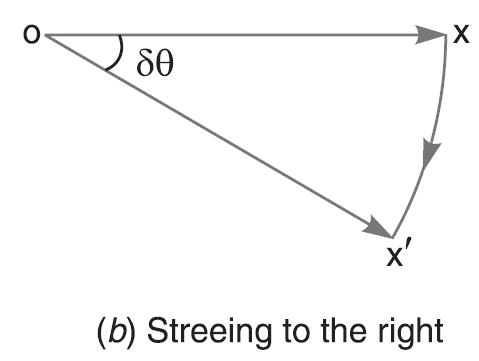
Figure 5: Effect of gyroscopic couple on a naval ship during steering.
KEWAYPOINTS:
Notes: 1. When the ship steers to the right under similar conditions as discussed above, the effect of the reactive gyroscopic couple, as shown in Fig.(b), will be to raise the stern and lower the bow.
2. When the rotor rates in the anticlockwise direction, when viewed from the stern and the ship is steering to the left, then the effect of reactive gyroscopic couple will beto lower the bow and raise the stern.
3. When the ship is steering to the right under similar conditions as discussed in point 2 above, then the effect of reactive gyroscopic couple will be to raise the bow and lower the stern.
4. When the rotor rotates in the clockwise direction when viewed from the bow or fore end and the ship is steering to the left, then the effect of reactive gyroscopic couple will be to raise the stern and lower the bow.
5. When the ship is steering to the right under similar conditions as discussed in point 4 above, then the effect of reactive gyroscopic couple will be to raise the bow and lower the stern.
6. The effect of the reactive gyroscopic couple on a boat propelled by a turbine taking left or right turn is similar
as discussed above.
Pitching:
Pitching is the movement of a complete ship up and down in a vertical plane about transverse axis, as shown in Fig. 14.10 (a). In this case, the transverse axis is the axis of precession. The pitching of the ship is assumed to take place with simple harmonic motion i.e. the motion of the axis of spin about transverse axis is simple harmonic.

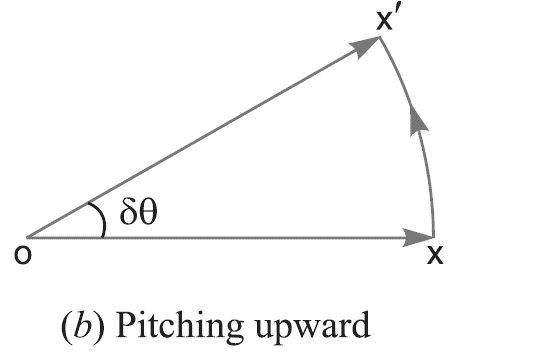
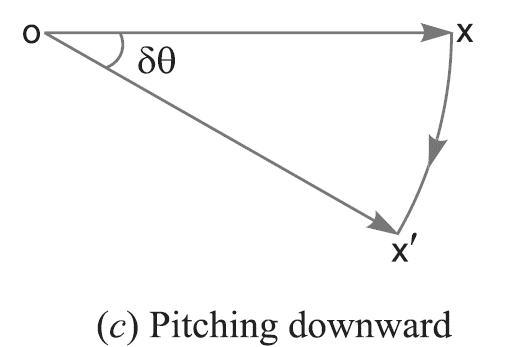
Figure 6: Effect of gyroscopic couple on a naval ship during pitching
Angular displacement of the axis of spin from mean position after time t seconds,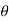 =
= 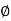 sin
sin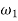 . t
. t
where 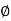 = Amplitude of swing i.e., maximum angle turned from the mean
= Amplitude of swing i.e., maximum angle turned from the mean
position in radians, and
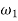 = Angular velocity of S.H.M.
= Angular velocity of S.H.M.
= 2π/ Time period of S.H.M in seconds = 2π/tp rad/s
Angular velocity of precession,
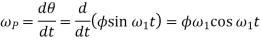
The angular velocity of precession will be maximum, if cos  .t = 1.
.t = 1.
Maximum angular velocity of precession,
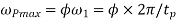
Substituting 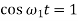
Let I = Moment of inertia of the rotor in kg-m2, and
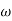 = Angular velocity of the rotor in rad/s.
= Angular velocity of the rotor in rad/s.
Maximum gyroscopic couple = 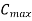 = I.
= I. 
When the pitching is upward, the effect of the reactive gyroscopic couple, as shown in Fig.(b), will try to move the ship toward star-board. On the other hand, if the pitching is downward, the effect of the reactive gyroscopic couple, as shown in Fig.(c), is to turn the ship towards port side.
1. The effect of the gyroscopic couple is always given on specific position of the axis of spin i.e. whether it is pitching downwards or upwards.
2. The pitching of a ship produces forces on the bearings which act horizontally and perpendicular to the motion of the ship.
3. The maximum gyroscopic couple tends to shear the holding-down bolts.
4. The angular acceleration during pitching,
Differentiating  with respect to t
with respect to t
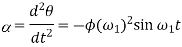
The angular acceleration is maximum, if sin 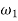 t = 1.
t = 1.
Maximum angular acceleration during pitching,
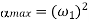
Rolling:
We know that, for the effect of gyroscopic couple to occur, the axis of precession should always be perpendicular to the axis of spin. If, however, the axis of precession becomes parallel to the axis of spin, there will be no effect of the gyroscopic couple acting on the body of the ship.
In case of rolling of a ship, the axis of precession (i.e., longitudinal axis) is always parallel to the axis of spin for all positions. Hence, there is no effect of the gyroscopic couple acting on the body of a ship.
Consider a two-wheel vehicle taking a right turn as shown in Fig.(a).
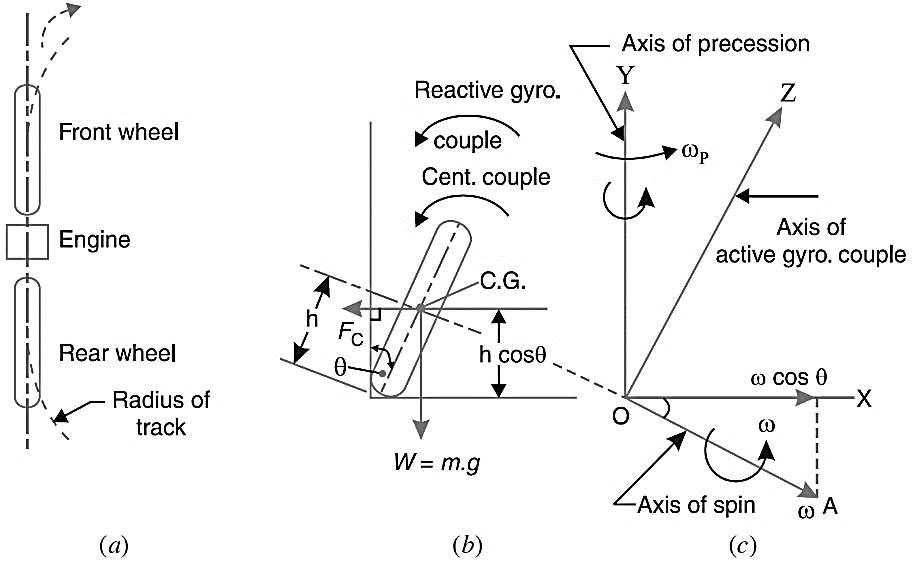
Figure 7: Stability of a two-wheel vehicle taking a turn
Let m = Mass of the vehicle and its rider in kg,
W = Weight of the vehicle and its rider in newtons = m.g,
h = Height of the centre of gravity of the vehicle and rider,
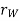 = Radius of the wheels,
= Radius of the wheels,
R = Radius of track or curvature,
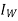 = Mass moment of inertia of each wheel,
= Mass moment of inertia of each wheel,
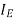 = Mass moment of inertia of the rotating parts of the engine,
= Mass moment of inertia of the rotating parts of the engine,
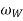 = Angular velocity of the wheels,
= Angular velocity of the wheels,
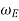 = Angular velocity of the engine,
= Angular velocity of the engine,
G = Gear ratio = 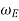 /
/ 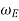 ,
,
v = Linear velocity of the vehicle = 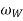 ×
× 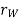 ,
,
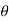 = Angle of heel. It is inclination of the vehicle to the vertical for equilibrium.
= Angle of heel. It is inclination of the vehicle to the vertical for equilibrium.
v = 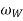 ×
× 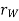 or
or 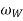 = v /
= v / 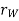
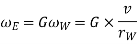
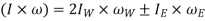

Gyroscopic couple,
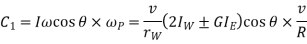
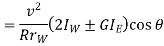
KEYWAY POINTS:
(a) When the engine is rotating in the same direction as that of wheels, then the positive sign is used in the above expression and if the engine rotates in opposite direction, then negative sign is used.
(b) The gyroscopic couple will act over the vehicle outwards i.e., in the anticlockwise direction when seen from the front of the vehicle. The tendency of this couple is to overturn the vehicle in outward direction.
Consider the four wheels A, B, C and D of an automobile locomotive taking a turn towards left as shown in Fig. The wheels A and C are inner wheels, whereas B and D are outer wheels. The center of gravity (C.G.) of the vehicle lies vertically above the road surface.
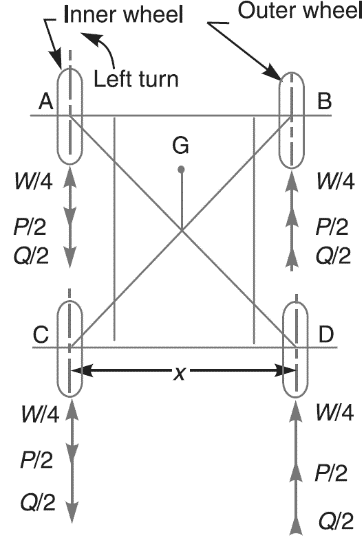
Figure 8: Four-wheel drive moving in a curved path
Let m = Mass of the vehicle in kg,
W = Weight of the vehicle in nektons = m.g,
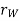 = Radius of the wheels in metres,
= Radius of the wheels in metres,
R = Radius of curvature in meters (R >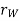 ),
),
h = Distance of center of gravity, vertically above the road surface in meters,
x = Width of track in meters,
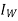 = Mass moment of inertia of one of thewheels in kg-
= Mass moment of inertia of one of thewheels in kg-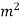 ,
,
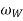 = Angular velocity of the wheels or velocityof spin in rad/s,
= Angular velocity of the wheels or velocityof spin in rad/s,
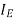 = Mass moment of inertia of the rotatingparts of the engine in kg-
= Mass moment of inertia of the rotatingparts of the engine in kg-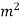 ,
,
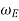 = Angular velocity of the rotating parts ofthe engine in rad/s,
= Angular velocity of the rotating parts ofthe engine in rad/s,
Road reaction over each wheel= W/4 = m.g /4 newtons
Since the vehicle takes a turn towards left due to the precession and other rotating parts, therefore a gyroscopic couple will act.
Velocity of precession,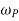 =
= 
Gyroscopic couple due to 4 wheels,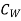 = 4
= 4 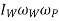
And gyroscopic couple due to the rotating parts of the engine,
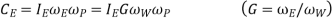
Net gyroscopic couple,
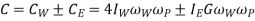
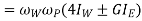
The positive sign is used when the wheels and rotating parts of the engine rotate in the same direction. If the rotating parts of the engine revolve in opposite direction, then negative sign is used.
Due to the gyroscopic couple, vertical reaction on the road surface will be produced. The reaction will be vertically upwards on the outer wheels and vertically downwards on the inner wheels.
Let the magnitude of this reaction at the two outer or inner wheels be P newtons. Then P × x = C or P = C/x
Vertical reaction at each of the outer or inner wheels,
P /2 = C/ 2x
Key takeaway:
sign is used, i.e., net gyroscopic couple,
C = 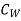 –
– 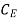
2. When 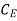 >
>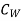 , then C will be –ve. Thus, the reaction will be vertically downwards on the outer wheels and vertically upwards on the inner wheels.
, then C will be –ve. Thus, the reaction will be vertically downwards on the outer wheels and vertically upwards on the inner wheels.
A Gyroscopic stabilizer is a control system that reduces the tilting movement of a ship or an aircraft. It senses orientation using a small gyroscope, and counteracts rotation by adjusting control surfaces or by applying force to a large gyroscope.
Such devices are used for maintaining the equilibrium of something such as a ship, aircraft, or platform.
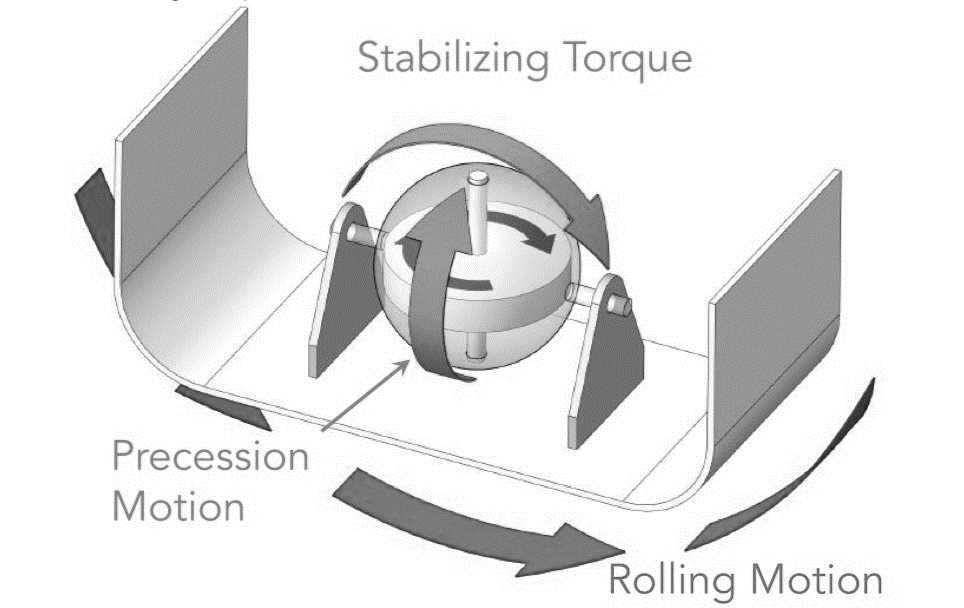
Figure 9: Gyrostabilizers
References: