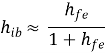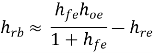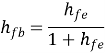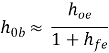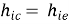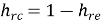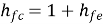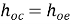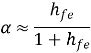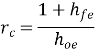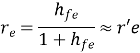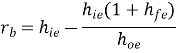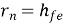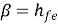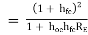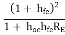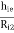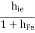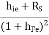Unit - 2

Small Signal Analysis of BJTs and FETs

2.1 Small Signal Analysis of BJTs: Small-Signal Equivalent-Circuit Models

The hybrid two port model is shown below.

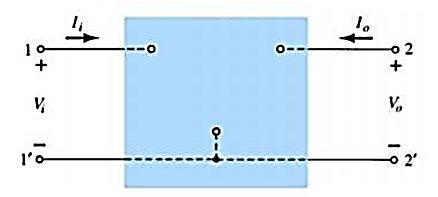
Fig 1 hybrid model

Vi = h11Ii + h12Vo

Io = h21Ii + h22Vo

h11 = hi = Vi/Io for Vo =0 [Input resistance]

h12 = hr = Vi/Vo for Ii =0 [Reverse voltage gain]

h21 = hf = Io/Ii for Vo =0 [forward current gain]

h22 = ho = Io/Vo for Ii =0 [output admittance]

The hybrid equivalent model is shown below

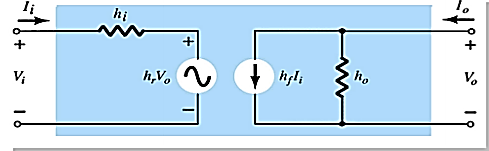
Fig 2 Equivalent hybrid model

The transistor model has three terminals with two ports.

hi = input resistance

ho= output conductance

hr= Vi/Vo = reverse transfer voltage

hf= Io/Ii = forward transfer current ratio

The simplified model is shown below

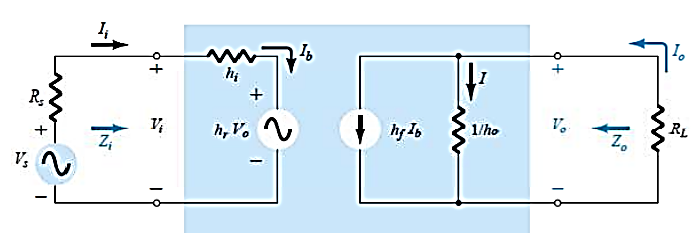
Fig 3 Simplified Model

Finding current gain Ai

Applying KCL at the output of above circuit

Io =I + hf Ib = Vo ho + hf Ib

Vo = -Io RL

Io = -Io RL ho + hf Ib

Io (1 + RL ho) = hf Ib

Ai = Io/ Ib = hf/ (1 + RL ho)

Finding Voltage Gain AV

Applying KVL at input of the above h-model

Vi = hi Ib + hr Vo

But Ib = (1 + ho RL)/hf

Io = - Vo/RL

Substituting in above equation and solving for Vo/Vi­­ we get

Av = - hf RL/hi + (hi ho- hf hr) RL

Finding Input Impedance Zi

Vi = hi Ib + hr Vo

Io = - Vo/RL

Ai = Io/ Ib

Vi = hi Ib - hrRLAiIb

Zi = Vi/Ib = hi - hrRLAi

Finding Output impedance Zo

It is ratio of output voltage to output current with Vs =0

Io = Voho + hfIb

Ii = -hrVo/Rs + hi

Zo = Vo/Io = 1/ [ho – (hfhr/hi+ Rs)]

Key takeaway

2.2 Small Signal Analysis of CE, CC, CB amplifiers

Small Signal Analysis of CE Amplifiers

In most practical cases it is appropriate to obtain approximate values of AV, Ai etc rather than calculating exact values. How the circuit can be modified without greatly reducing the accuracy. Figure shows the CE amplifier equivalent circuit in terms of h-parameters Since 1 / hoe in parallel with RL is approximately equal to RL if 1 / hoe >> RL then hoe may be neglected. Under these conditions.

Ic = hfe IB.

hre vc = hre Ic RL = hre hfe Ib RL

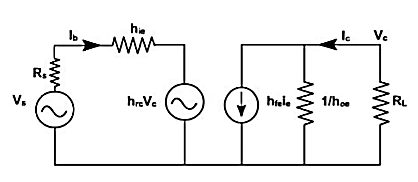
Fig 4 CE Amplifiers

Since hfe hre » 0.01, this voltage may be neglected in comparison with hic Ib drop across hie provided RL is not very large. If load resistance RL is small than hoe and hre can be neglected.

Ai = Io/ Ib = hfe/ (1 + RL hoe)

Ri= hie

Av= AIRL/Ri= -hfe RL/hie

Output impedance seems to be infinite. When Vs = 0, and an external voltage is applied at the output we fined Ib = 0, IC = 0. True value depends upon RS and lies between 40 K and 80K. On the same lines, the calculations for CC and CB can be done.

CE amplifier with an emitter resistor:

The voltage gain of a CE stage depends upon hfe. This transistor parameter depends upon temperature, aging and the operating point. Moreover, hfe may vary widely from device to device, even for same type of transistor. To stabilize voltage gain AV of each stage, it should be independent of hfe. A simple and effective way is to connect an emitter resistor Re as shown in figure. The resistor provides negative feedback and provide stabilization.

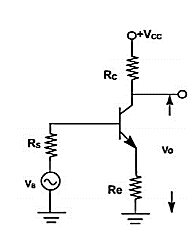
Fig 5 CE amplifier with an emitter resistor

Current gain Ai = = =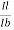

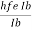
Input Resistance is given by

Ri =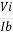

    =

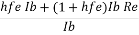
    = hfe = (1+ hfe) Re

The input resistance is increased by (1+hfe) Re

Ai = =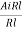

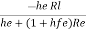
Ai = =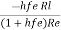

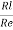
CE amplifiers are very popular to amplify the small signal ac. After a transistor has been biased with a Q point near the middle of a dc load line, ac source can be coupled to the base. This produces fluctuations in the base current and hence in the collector current of the same shape and frequency. The output will be enlarged sine wave of same frequency. The amplifier is called linear if it does not change the wave shape of the signal.

 As long as the input signal is small, the transistor will use only a small part of the load line and the operation will be linear. On the other hand, if the input signal is too large. The fluctuations along the load line will drive the transistor into either saturation or cut off. This clips the peaks of the input and the amplifier is no longer linear.

The CE amplifier configuration is shown

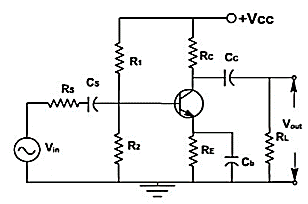
Fig 6 CE Amplifier Circuit

The coupling capacitor (CC) passes an ac signal from one point to another. At the same time, it does not allow the dc to pass through it. Hence it is also called blocking capacitor.

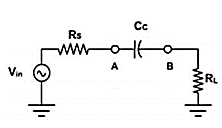
Fig 7 Coupling Capacitor

From Figure above the ac voltage at point A is transmitted to point B. For this series reactance XC should be very small compared to series resistance RS. The circuit to the left of A may be a source and a series resistor or may be the Thevenin equivalent of a complex circuit. Similarly, RL may be the load resistance or equivalent resistance of a complex network. The current in the loop is given by

i =

  =

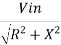
As frequency increases, XC decreases, and current increases until it reaches to its maximum value vin / R. Therefore, the capacitor couples the signal properly from A to B when XC<< R. The size of the coupling capacitor depends upon the lowest frequency to be coupled. Normally, for lowest frequency XC 0.1R is taken as design rule. The coupling capacitor acts like a switch, which is open to dc and shorted for ac. The bypass capacitor Cb is similar to a coupling capacitor, except that it couples an ungrounded point to a grounded point. The Cb capacitor looks like a short to an ac signal and therefore emitter is said ac grounded. A bypass capacitor does not disturb the dc voltage at emitter because it looks open to dc current. As a design rule XCb =0.1RE at

 Analysis of CE amplifier: In a transistor amplifier, the dc source sets up quiescent current and voltages. The ac source then produces fluctuations in these current and voltages. The simplest way to analyse this circuit is to split the analysis in two parts: dc analysis and ac analysis. One can use superposition theorem for analysis.

Characteristics of Common Emitter Amplifier

(i)                The current gain Ai is high for RL < 10 kΩ.

(ii)              The voltage gain is high for normal values of load resistance RL.

(iii)           The input resistance Ri is medium.

(iv)            The output resistance Ro is moderately high.

Applications: CE amplifier is widely used for amplification

Common Base Amplifier

The common base amplifier circuit is shown in Figure below. The VEE source forward biases the emitter diode and VCC source reverse biased collector diode. The ac source vin is connected to emitter through a coupling capacitor so that it blocks dc.

Fig 8 CB Amplifier

This ac voltage produces small fluctuation in currents and voltages. The load resistance RL is also connected to collector through coupling capacitor so the fluctuation in collector base voltage will be observed across RL. The dc equivalent circuit is obtained by reducing all ac sources to zero and opening all capacitors. The dc collector current is same as IE and VCB is given by VCB = VCC - IC RC. Figure above.

These current and voltage fix the Q point. The ac equivalent circuit is obtained by reducing all dc sources to zero and shorting all coupling capacitors. r'e represents the ac resistance of the diode as shown in Figure below.

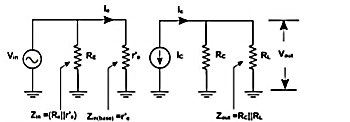
Fig 9 Equivalent CB Amplifier

The above figure shows the diode curve relating IE and VBE. In the absence of ac signal, the transistor operates at Q point (point of intersection of load line and input characteristic). When the ac signal is applied, the emitter current and voltage also change. If the signal is small, the operating point swings sinusoidally about Q point (A to B).

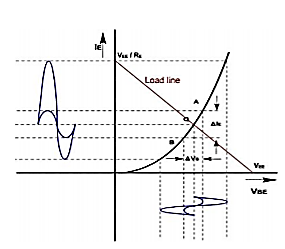
Fig 10 Q-Point

If the ac signal is small, the points A and B are close to Q, and arc A B can be approximated by a straight line and diode appears to be a resistance given by

 r’e =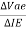

        = =

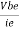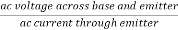
If the input signal is small, input voltage and current will be sinusoidal but if the input voltage is large then current will no longer be sinusoidal because of the non-linearity of diode curve. The emitter current is elongated on the positive half cycle and compressed on negative half cycle. Therefore, the output will also be distorted. r'e is the ratio of ΔVBE and Δ IE and its value depends upon the location of Q. Higher up the Q point small will be the value of r' e because the same change in VBE produces large change in IE. The slope of the curve at Q determines the value of r'e. From calculation it can be proved that

 r'e = 25mV / IE

Characteristics of Common Base Amplifier

(i)                Current gain is less than unity and its magnitude decreases, with the increase of load resistance RL

(ii)              Voltage gain AV is high for normal values of RL

(iii)           The input resistance Ri is the lowest of all the three configurations, and

(iv)            The output resistance Ro is the highest of all the three configurations.

Applications The CB amplifier is not commonly used for amplification purpose. It is used for

(i)                Matching a very low impedance source

(ii)              As a non-inverting amplifier to voltage gain exceeding unity.

(iii)           For driving a high impedance load. (iv)As a constant current source.

Common Collector Amplifier

(i)                For low RL (< 10 kΩ), the current gain Ai is high and almost equal to that of a CE amplifier.

(ii)              The voltage gain AV is less than unity.

(iii)           The input resistance is the highest of all the three configurations.

(iv)            The output resistance is the lowest of all the three configurations.

Applications The CC amplifier is widely used as a buffer stage between a high impedance source and a low impedance load.

Key takeaway

2.3 Effects of RS and RL on CE amplifier operation

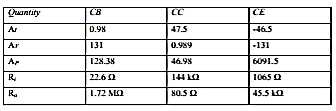
Voltage Amplification Factor (Avs) taking into account the resistance (Rs) of the source

This overall voltage gain Avs is given by

Avs = V2 / VS = V2 V1 / V1VS = Av V1/ VS

From the equivalent input circuit using Thevenin’s equivalent for the source shown in Figure

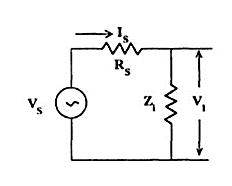
Fig11 Thevenin’s Equivalent

 V1 = VS Zi / (Zi + RS)

V1 / VS = Zi / (Zi + RS)

Then, Avs = Av Zi / (Zi + RS)

Substituting Av = Ai ZL / Zi

Avs = Ai ZL / (Zi + RS)

Avs = Ai ZL RS / (Zi + RS) RS

Avs = Ais ZL / RS

Avs = (AI RL/Zi) * (Zi/Rs + ZL) = (AI RL/Rs + Zi)

Effect due to RL

If Rs= 0

Then,

Avs = (AI RL/Zi)

Current Amplification (Ais) taking into account the source Resistance (RS)

The modified input circuit using Norton’s equivalent circuit for the calculation of Ais is shown in Figure.

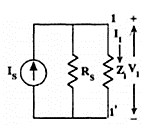
Fig 12 Norton Equivalent

Overall Current Gain,

Ais = -I2 / IS = - I2I1 /I1

IS = Ai I1/IS

From Figure

I1= IS RS / (RS + Zi)

I1 / IS = RS / (RS + Zi)

and hence, Ais = Ai RS / (RS + Zi)

Key takeaway

The current gain becomes

Ais = Ai RS / (RS + Zi)

Effect due to RL

If Rs= 0

Then,

Avs = (AI RL/Zi)

2.4 Emitter Follower

This is also called as common collector amplifier. It is basically used to provide current gain and for impedance matching. The circuit is shown below where the input is applied through base and output is obtained from emitter. The voltage gain is always<1 and output voltage are in phase with input voltage. The output follows the input hence it is called as emitter follower.

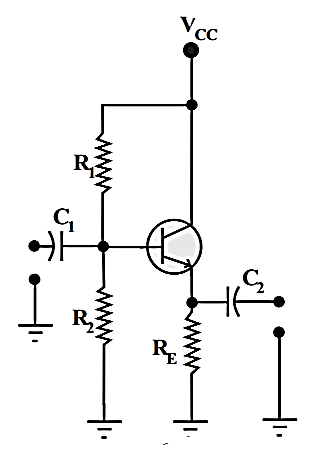
Fig 13 Emitter Follower

The base voltage can be obtained by applying voltage division rule on R2 and R1

VB = 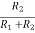  VCC

The emitter voltage is VE = VB – 0.7

The emitter current is IE =

VCEQ = VCC-VE

For A.C analysis the circuit can be drawn as shown below

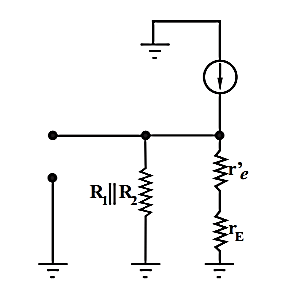
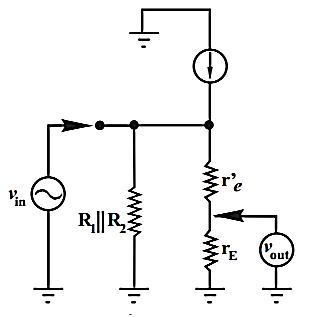
Fig 14 A.C equivalent circuit

The total equivalent ac emitter resistance is rE which is

rE = RE||RL

Practically re’ << rE. The voltage gain then becomes

AV = 1

This relationship is due to the current divisions that occur in both the input and output circuits. we have used in place of hfe. We are assuming that is approximately equal to hfe. The subscript “c” merely indicates that the parameter applies to the emitter follower (common collector) rather than the common emitter amplifier. The exact equation for hfc is

hfc = hfe +1

Since is normally much greater than 1, we normally assume that they are approximately equal.

where:

hfe= transistor current gain

re’= the ac emitter resistance

rE = RL||RE

The output impedance is the impedance that the circuit presents to its load. When a load is connected to the circuit, the output impedance of the circuit acts as the source impedance for that load.

Zout = RE|| [re’ + (Rin’/hfe)]

Key takeaway

The voltage gain is always<1 and output voltage are in phase with input voltage. The output follows the input hence it is called as emitter follower.

2.5 Cascade amplifier

A cascade amplifier is a two-port network designed with amplifiers which are connected in series when every amplifier transmits its o/p to the second amplifiers input in a chain. The circuit diagram for two stage amplifiers is shown below.

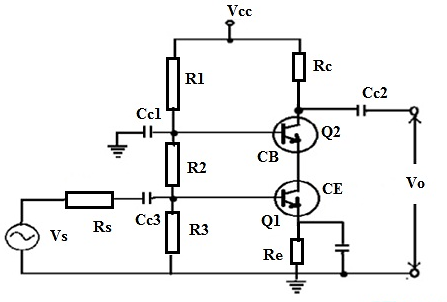
Fig 15 Cascade Amplifier

In order to achieve proper amplification cascade amplifiers are used. The overall gain is Av= Av1 x Av2

The CB configuration provides high frequency operation. The CB and CE configurations are used. The current gain and input resistance are equivalent to related values of CE amplifier. The output can be equivalent to CB configuration. They are used in tuned RF amplifiers. They can also be used as wideband amplifiers.

2.6 Darlington Connection and Current Mirror Circuits

Darlington Connection

The Darlington pair is a combination of two common collector cascade configuration. In Darlington pair the collectors of the two transistors are connected together, emitter of Q1 is connected to the base of Q2 and emitter of Q2 acts as the emitter of Darlington connection.

The important feature of the Darlington connection is that the composite will act as a single unit, the current gain of which is equal to the product of the individual current gains β1 and β2.

In general Darlington connection with more than 2 transistors is not used because

1)     With increase in transistors, leakage current increases.

2)     The voltage gain of CC is approximately 1 but that of the Darlington is much less than 1. With increase in transistor, voltage gain will decrease further.

Advantages:

1)     Very high current gain.

2)     Very low voltage gain.

3)     Very high input impendence.

4)     Very low output impendence.

Disadvantage – more than 2 transistors cannot be used.

Analysis of Darlington emitter follower –

The circuit diagram of the Darlington emitter follower is shown above.

Assumptions –

1)     Both Q1 and Q2 are identical.

2)     hoe 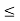0.1 i.e., approximate analysis.

3)     hfe RE >> hie

1.Current gain of the Darlington emitter follower (AI):

a)     Current gain of Q2:

AI2 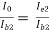= (1 + hfe)

b)    Current gain of Q1:

the input resistance of stage 2 acts as the load for Q1

Ri2 = hie + (1 + hfe) RL2

= hie + AI2RE {where RL2 = RE}

As Ri2 is large, the condition hoe RL’ < 0.1 is not satisfied by first stage

Where RL’ = RL2 = RE

Hence using exact analysis for Q1

AI1 =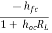

Substitute 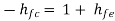and 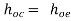, we get

AI1 = 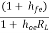                 -------------(1)

But 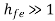hence, RL1 = Ri2 = hie + hfeRE

Substituting the expression for RL1 in equation (1)

AI1 =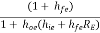

hoehie is very small so neglecting it,

AI1 =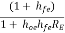

Overall current gain:  AI =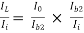

i.e., AI = AI1 AI2

=

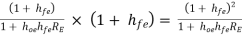
AI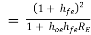              -------------(2)

The above equation shows that overall current gain of the Darlington connection is much higher than that of the single emitter follower (common collector).

2.Overall input resistance (Ri):

The overall input resistance will be same as the input resistance of first stage Q1

Ri = Ri1

Ri1 = hie + AI1RL1     where RL1 = Ri2

Substituting the value of AI1 and Ri2, we get

Ri1 = hie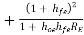RE                       --------------(3)

2nd term in equation (3) is much larger than first term, so neglecting hie

Input resistance, Ri1 =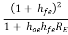RE   

If hoehfeRE << 1, then

Ri1 =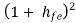RE        ----------------(4)

For a single CC amplifier Ri = hi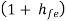e + RE

Equation (4) shows that due to Darlington connection, there is tremendous increase in input resistance.

3.Voltage Gain of Darlington connection (AV):

Overall voltage gain AV =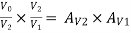

AV2 is calculated using approximate analysis.

AV1 is obtained by exact analysis.

a)     Voltage gain AV2 =

AI2 = 1 + hfe

Ri2 = hie + (1 + hfe) RL2

RL2 = RE

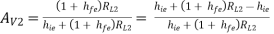
{Adding and subtracting hie in numerator}

AV2 = 1 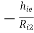            ----------(5)

b)    Voltage gain AV1: AV1 = AI1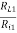

But for single emitter follower,

AI1 = (1 + hFe),

Ri1 = hie + AI1RL1

AV1 = 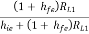   where RL1 = Ri2

Adding and subtracting hie in numerator of right-hand side, we get

AV1 =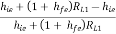

AV1 = 1 -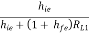

AV1 = 1 - 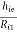              -----------(6)

Overall voltage gain = AV = AV1 × AV2

i.e., AV = AV2 =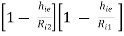 

AV =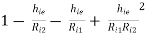 

It is evident that Ri1 >> Ri2 hence 3rd and 4th term in above equation can be neglected

AV = 1 - 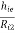                             -------------(7)

Comparing (5) and (7)

AV AV2

The overall voltage gain is approximately equal to the voltage gain of second stage.

4.Overall output resistance RO:

The overall output resistance of the emitter follower Darlington circuit is same as the output resistance of second stage.

YO = hoc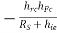

Hence YO2 = hOC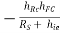

Hence YO2 = hOC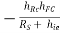

RS for 2nd stage is R01 i.e., output resistance of 1st stage.

But hrc = 1, hOC = hOe, hFC = - (1 + hFe) and hiC = hie

YO2 = hoe +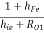

Neglect hOe because we are performing approximate analysis for the second stage we get,

YO2 =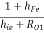

Hence, RO2 =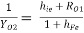

But, RO1 =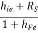

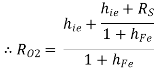
RO = RO2 = +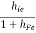

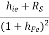
Neglect the 2nd term due to 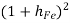in denominator

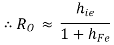
The above equation tells us that the RO of Darlington emitter follower is much less than that of a single emitter follower.

Key takeaway

For emitter follower

The value of Z is typically higher than the common emitter amplifier. The voltage gain is always less than 1 The heavy swamping of the circuit virtually eliminates the effect of r’ on the voltage gain.

Que. For T1 hie1 = 6 KΩ     hFe1 = 80       hre1 = hoe1 = 0

For T2 hie2 = 1 KΩ     hFe2 = 100     hre2 = hoe2 = 0

For the Darlington pair emitter follower, shown in figure, determine:

a)     Input impendence R1                      

b)    Voltage gain VO / VS

c)     Current gain IO / IS

d)    Output impendence

Sol – Given: hie1 = 6 KΩ     hFe1 = 80       hre1 = hoe1 = 0

hie2 = 1 KΩ     hFe2 = 100     hre2 = hoe2 = 0

Amplifier configuration:

Q1 → CC           Q2 → CC

Therefore, approximate analysis is used, for both stages

For second stage:

Current gain (Ai2)

AI2 = 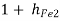= 1 + 100 = 101

Input resistance (Ri2)

RL1 = Ri2 = hie2 + (1 + hFe2) RL = 1 × 103 + (1 + 100) × 103 = 102 KΩ

Voltage gain (AV2):

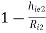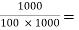AV2 = =1 - 0.99

For first stage:

Current gain (Ai1):

Ai1 = 1 + hFe1 = 81

Input resistance (Ri1):

Ri1 = hie1 + (1 + hFe1) RL

= 6 × 103 + (1 + 80) × 102 × 103 = 8.268 MΩ

Voltage gain (AV1):

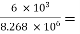AV1 = = 1 - 0.999

RO1 = 86.42 Ω

RO2 = 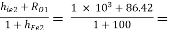 10.76 Ω

Overall voltage gain (AV):

AV = AV1 × AV2 = 0.999 × 0.99 = 0.989

AVS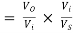

Figure shows equivalent circuit of the input side

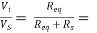0.998

AVS = 0.989 × 0.998 = 0.987

Overall voltage gain:

AI = Ai = Ai1 × Ai2 = 81 × 101 = 8181                      Ans.

Input impendence:

Ri = 0.89 MΩ                                                            Ans.

Overall voltage gain:

AV = 0.989                                                                 Ans.

Voltage gain include source:

AVS = 0.987                                                               Ans.

Output Impendence:

RO = 10.76 Ω                                                             Ans.

Que. For the bootstrap circuit shown, calculate AI, Ri and AV. the transistor parameters are hie = 2 KΩ, hFe = 100, 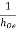= 40 K and hre = 2.5 × 10-4.

Sol – Given: hie = 2 KΩ, hFe = 100, 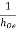= 40 K, hre = 2.5 × 10-4.

Amplifier configuration: common emitter with Re

AC analysis: For a.c. analysis of amplifier we consider the d.c. supply voltage as ground and capacitor as short circuit and apply Miller’s theorem across feedback resistor (10K) then modified circuit is given as:

The circuit down in fig. (a) represents a CE amplifier. But to analyse the bootstrapped element we need to consider the CC voltage gain first.

AV = common collector voltage gain

RL’ = 2K || 4K || 4K || 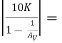1 K || 490 K = 1 KΩ

Assume AV 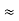0.98

Checking approximation:

hoeRL’ 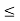0.1

0.025 < 0.1

Hence, valid approximation

Approximate analysis:

AI = 1 + hFe = 101

Ri = hie + (1 + hFe) RL’ = 2 + 101 = 103 KΩ             Ans.

AV = AI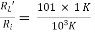= 0.98

From fig. (a): R1 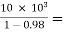 500 KΩ

Now, fig. (a) can be analysed by CE and the modified circuit is given as,

Checking approximation:

hoe (Re + RL) 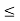0.1

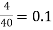
Hence, valid approximation

Approximate analysis:

AI’= -hFe = -100

Ri’= hie + (1 + hFe) Re’ = 2 + 101 × 1 = 103 KΩ

AV’ =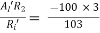

AV = AV’ = -2.9126

From fig. (b): Ri = Ri’ || 500K = 100 || 500 = 85.406 KΩ

AI =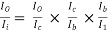

    = (-1) × 100 ×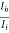

From fig. (c): 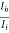- 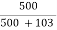= 0.8291

Hence, AI = - 100 × 0.82918 = -82.918

Que. The bootstrapped Darlington pair uses identical transistors with the following h-parameters.

hie = 1 KΩ, hre = 2.5 × 10-4, hoe = 2.5 × 10-4 A/V, hFe = 100

Find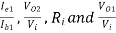

Sol - Given: hie = 1 KΩ, hre = 2.5 × 10-4, hoe = 2.5 × 10-4 A/V, hFe = 100

Q1 – CC, Q2 - CC

For a.c. analysis of amplifier we consider the d.c. supply voltage as ground and capacitor as short circuit.

Apply Miller’s theorem across R3 = (100K)

Voltage Gain:

AV = 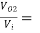AV1 × AV2

Let us assume AV = 0.98

Where, 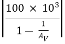= 4900 KΩ

RL’ = 0.1K || 82K || 10K || 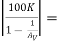0.1 K || 82 K || 10 K || 4900 K = 0.0988 KΩ

Analysis for Q2 [CC]:

Checking approximation:

hoeRL’ = 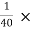0.0988 = 2.47 × 10-3 < 0.1

Approximate analysis:

AI2 = 1 + hFe = 101

Ri2 = hie + (1 + hFe) RL’ = 1 + 101 × 0.0988 = 10.9788 KΩ          

AV2 = AI2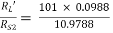= 0.908915

Analysis for Q1 [CC]:

RL = Ri2

hoeRL1 = 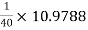= 0.27447 > 0.1

Exact analysis:

AI2 = 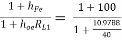= 79.2486

Ri1 = hie + AI1hrcRL1 = 1 + 79.2486 × 10.9788

= 871.054 KΩ   (hrc 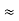1)

AV1 =

= 0.99885

AV 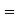AV1 × AV2 = 0.99885 × 0.908915

= 0.9079                                      (Ans)

Ri = R || Ri1 = 1085.78 || 871.054                {Fig. (a)}

= 483.32 KΩ

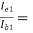AI1 = 79.2486

AI =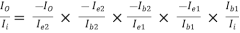

=

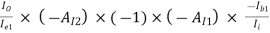
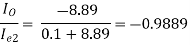
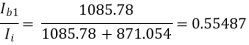
AI = (-0.9889) × (-101) × (-1) × (-79.2486) × 0.55487

= 4391.94                                    (Ans)

Equation for VO/Vi:

Analysis of Q2 [CC]:

hoe (Re+RL)

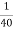× [0.0988 + 1] = 0.02747 < 0.1

Approximate analysis

AI2 = - hFe = -100

Ri2 = hie + (1 + hFe) Re

= 1 +(1 + 100)0.0988 = 10.9788 KΩ

AV2 = 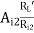= 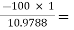-9.1084

Analysis of Q1 [CC]:

RL1 = Ri2 = 10.9788 KΩ

hoeRL1 = 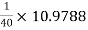 = 0.27447 > 0.1

Exact analysis:

AI1 = 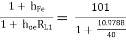= 79.2486

RL1 = hie + AI1RL1 = 1 + 79.2486 × 10.9788

= 871.054 KΩ

AV1 = =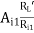

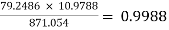
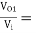AV = AV1 × AV2 = -9.0975

Hence, -AI1 = 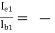79.248

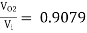
Ri = 483.32 KΩ

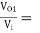-9.0975                                 (Ans)

Que. A bootstrapped Darlington amplifier shown in fig. uses identical transistor with h-parameters, hie = 1.5 KΩ, hoe = 50 μA/V. If the circuit is to have Ri of 268 MΩ. Find the value of hFe for the transistor used.

Sol – Given: hie = 1.5 KΩ, hoe = 50 μA/V, Ri = 2.68 MΩ, R1 = 1 MΩ, R2 = 1 MΩ, Re2 = 1 KΩ

RLeft = RC1 || RC2 = ∞ || 1 K = 1 KΩ

Amplifier configuration: bootstrapped Darlington pair amplifier [CC - CC]

Checking for approximation:

hoeRLeft 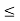0.1

hoeRLeft = 25 × 10-6 × 1 K = 0.025 < 0.1

which is less than 0.1

Approximate analysis:

Calculation for hFe

Ai2 = 1 + hFe

Ri2 = hie2 + Ai2RLeft = 1.5 K + (1 + hFe)1 K

Ai1= 1 + hFe

Ri1 = hie1 + Ai1RL1

Where RL1 = Ri2

Ri1 = 1.5K + (1 + hFe) [1.5K + (1 + hFe)1K]

2.68 MΩ = 1.5K + 1.5K + (1 + hFe)1K + 1.5Khfe + (1+hFe) 1KhFe

= 3K + 1K + 1KhFe + 1.5KhFe + 1KhFe + 1KhFe2

= 4K + 3.5KhFe + 1KhFe2

= (4 + 3.5hFe + hFe2) × 103

hFe2 + 3.5hFe + 4 = 2680

hFe2 + 3.5hFe - 2676 = 0

The quadratic equation can be solved as,

hFe =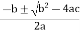

Substituting values we get,

hFe

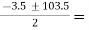= 50 or -53.5

hFe is always positive.

hFe = 50                                                          Ans.

Overall voltage gain:

(AV): AV = AV1 × AV2 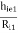= (1 - 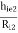)( 1 - )

= (1 - )( 1 - )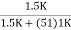

= 0.999 × 0.971 = 0.97

Overall current gain –

Ai = Ai1 × Ai2

= (1 + hFe) (1 + hFe)

= (51)(51)

= 26.01

Current Mirror Circuits

Here at the Q2 side, more current mirrors need to be used to provide current KID2=KID1. Also, it can be seen that all internal nodes have low impedance except the output node. By using proper current mirrors with high output impedance, good gain can be achieved. The overall transfer function of this Op-Amp closely approximate dominant-pole operation.

Av = = =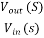

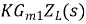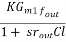
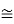
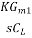
Unity-gain frequency = =

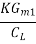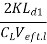
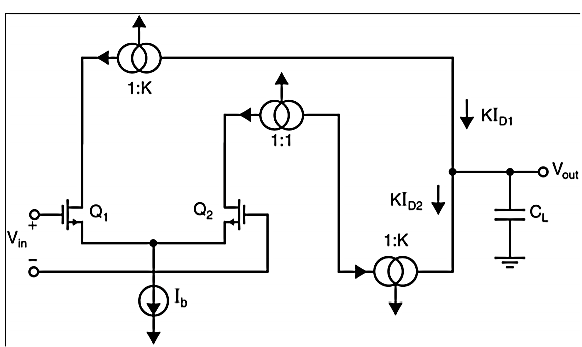
Fig 16 Current Mirror Circuit

If power dissipation is specified, then total current is given by,

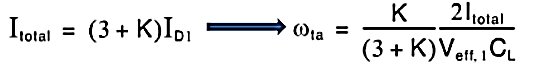
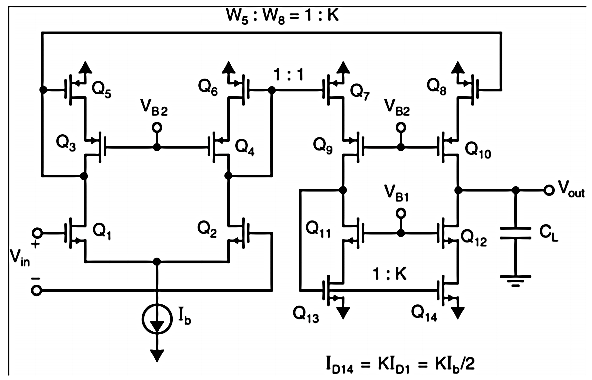
Fig 16 Circuit for Current Mirror

It can be seen that larger K increases the unity-gain frequency assuming the load capacitor dominates the time constants. Larger K also increases the gain. A typical upper limit for K is 5. A detailed analysis reveals important nodes for determining the non-dominant poles, at the drain of Q1 first and drain of Q2 and Q9 secondly.

Larger K increases the capacitances at these nodes while also increases the resistance (assuming a fixed Itotal), which reduce the non-dominant poles. In this case, then CL has to be increased to maintain a large phase margin.

So, K should not be too large, i.e., K<=2 usually. During slew rate, all of the bias current Ib of the first stage is diverted through Q1/2 and amplified by the current mirror gain to the output. The total current to charge/discharge the load is KIb.

So, the slew rate is Due primarily to the larger unity-gain frequency and slew rate, the current-mirror OpAmp may be preferred over the folded-cascade OpAmp.

 However, one has to be careful that the current-mirror OpAmp has larger input noise as well, as its input stage is biased at a lower portion of the total bias current and therefore a relatively smaller gm given the same power consumption.

Key takeaway

2.7 Small Signal Analysis of FETs: Small-Signal Equivalent-Circuit Model

Field Effect Transistor (FET) amplifiers provide an excellent voltage gain and high input impedance. Because of high input impedance and other characteristics of JFETs they are preferred over BJTs for certain types of applications.

There are 3 basic FET circuit configurations

i)                   Common Source

ii)                 Common Drain

iii)              Common Gain

Similar to BJT CE, CC and CB circuits, only difference is in BJT large output collector current is controlled by small input base current whereas FET controls output current by means of small input voltage. In both the cases output current is controlled variable. FET amplifier circuits use voltage-controlled nature of the JFET. In Pinch off region, ID depends only on VGS.

The linear small signal equivalent circuit for the FET can be obtained in a manner similar to that used to derive the corresponding model for a transistor. We can express the drain current iD as a function f of the gate voltage and drain voltage Vds.

Id = f(Vgm, Vrd) (1)

The transconductance gm and drain resistance rd: - If both gate voltage and drain voltage are varied, the change in the drain current is approximated by using Taylor’s series considering only the first two terms in the expansion

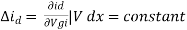
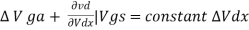
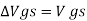
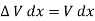
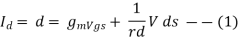
Where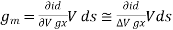

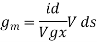
Is the mutual conductance or trans-conductance. It is also called as gfs or yfs common source forward conductance. The second parameter rd is the drain resistance or output resistance is defined as

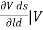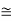
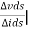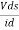rd = gs Vgs = |vgs

rd  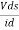= |vgs

The reciprocal of the rd is the drain conductance gd. It is also designated by Yos and Gos and called the common source output conductance. So, the small signal equivalent circuit for FET can be drawn in two different ways.

 1.small signal current –source model

2.small signal voltage-source model.

A small signal current –source model for FET in common source configuration can be drawn satisfying Equation (1). This low frequency model for FET has a Norton’s output circuit with a dependent current generator whose magnitude is proportional to the gate-to –source voltage.

The proportionality factor is the transconductance ‘gm’. The output resistance is ‘rd’. The input resistance between the gate and source is infinite, since it is assumed that the reverse biased gate draws no current. For the same reason the resistance between gate and drain is assumed to be infinite. The below section covers the detailed analysis.

2.8 Small Signal Analysis of CS, CD, CG Amplifiers.

Common Source (CS) Amplifier

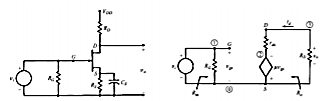
Fig 17 CS Amplifier and Small Signal equivalent Circuit

A simple Common Source amplifier and associated small signal equivalent circuit using voltage-source model of FET is shown in Figure above.

Voltage Gain

Source resistance (RS) is used to set the Q-Point but is bypassed by CS for mid-frequency operation. From the small signal equivalent circuit, the output voltage

VO = -RDµ Vgs (RD + rd)

Where Vgs = Vi,

the input voltage,

Hence, the voltage gain,

AV = VO / Vi = -RDµ (RD + rd)

Input Impedance

Zi = RG

For voltage divider bias as in CE Amplifiers of BJT

RG = R1 ║ R2

Output Impedance

Output impedance is the impedance measured at the output terminals with the input voltage VI = 0. When the input voltage Vi = 0, Vgs = 0

and hence µ Vgs = 0

The equivalent circuit for calculating output impedance is given in Figure below.

 Output impedance

Zo = rd ║ RD

 Normally rd will be far greater than RD. Hence Zo ≈ RD

Common Drain Amplifier

A simple common drain amplifier is shown in Figure above and associated small signal equivalent circuit using the voltage source model of FET. Since, voltage Vgd is more easily determined than Vgs, the voltage source in the output circuit is expressed in terms of Vgs and Thevenin’s theorem.

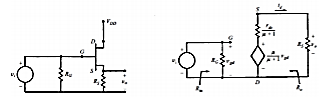
Fig 18 CD Amplifier and Small Signal equivalent circuit

Voltage Gain

The output voltage, VO = RS µVgd / (µ + 1) RS + rd

Where Vgd = Vi the input voltage.

Hence, the voltage gain,

Av = VO / Vi = RS µ / (µ + 1) RS + rd

Input Impedance

From Figure above,

Input Impedance

Zi = RG

Output Impedance

From above figure,

Output impedance measured at the output terminals with input voltage Vi = 0 can be calculated from the following equivalent circuit.

As Vi = 0: Vgd = 0: µ vgd / (µ + 1) = 0

Output Impedance ZO = rd / (µ + 1) ║RS

When µ » 1

ZO = ( rd / µ) ║RS = (1/gm) ║RS

Common Gate Amplifier

In common source amplifier and source follower circuits, the input signal is applied to the gate of a MOSFET. It is also possible to apply the input signal to the source terminal by keeping common gate terminal. This type of amplifier is called as common gate amplifier.

Figure below shows the CG amplifier in which the input signal is sensed at the source terminal and the output is produced at the drain terminal. The gate terminal is connected to VB i.e., dc potential which will maintain the proper operating conditions.

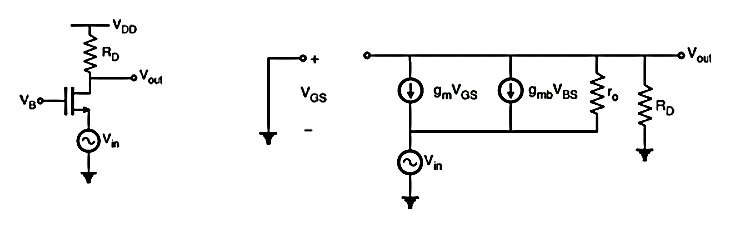
Fig 19 CG Amplifier and Small Signal equivalent circuit

By analysing the small signal equivalent circuit, the voltage gain of CG amplifier is given by,

Av = = gm RD

The important point is the gain is positive, further the input impedance is given by which shows that the input impedance of common gate amplifier is relatively low. Furthermore, the input impedance of gate stage is relatively low only if the load resistance connected to the drain is small.

Key takeaway

2.9 Effects of RSIG and RL on CS Amplifier

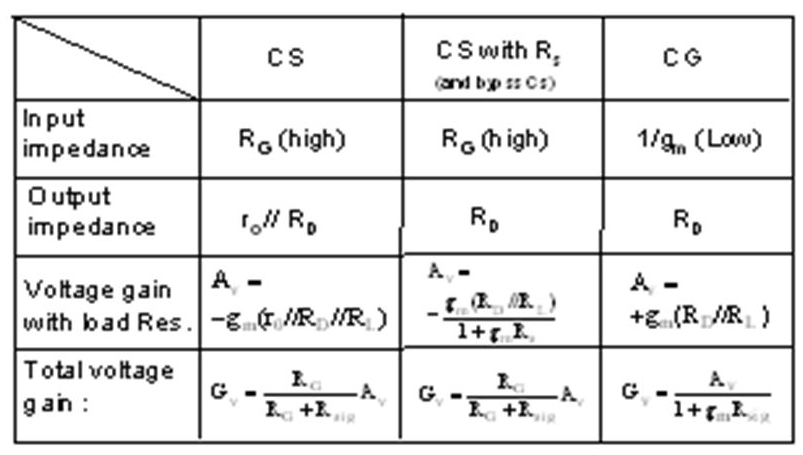
1. The input resistance Rin is infinite.

2. The open-circuit voltage gain, Avo, is reduced by a factor of 1 + gmRs

3. For the same nonlinear distortion, the input signal can be increased by a factor of 1 + gmRs compared to without Rs.

4. As shall be shown later, the high-frequency response of this design is improved.

In general, the addition of the source resistance Rs gives rise to a “negative” feedback factor 1 + gmRs that reduces voltage gain, but improves linearity, and high-frequency response. Because of the negative-feedback action of Rs, it is also called the source-degenerate resistance.

2.10 Source Follower and Cascaded System

This is similar to the emitter follower for the BJT, which is used as a voltage buffer. It is a unit-gain amplifier with a very large input impedance but a smaller output impedance. Therefore, it is good for matching a high-impedance circuit to a low-impedance circuit or to a circuit that needs a larger supply of current.

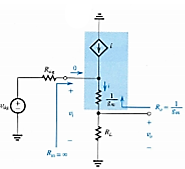
Fig 20 CD Source Follower and it’s equivalent circuit

Characteristics of a Source Follower

The small-signal circuit and a T-model equivalent circuit diagram for a source follower. The input source is represented by a Thevenin equivalent voltage vsig and resistor Rsig. A load resistor is connected to the output between the source and ground.

Since the gate current is zero for this circuit,

 Rin = ∞ 

Using the voltage divider formula, it is seen that voltage gain proper or terminal voltage gain is

 Av = vo/vi = RL/ (RL + 1/gm)

For the open-circuit voltage gain, RL = ∞ and Avo = 1

The output resistance is obtained by replacing the proper part of the amplifier with a Thevenin equivalence. To this end, with the use of the test-current method, one sets the value of vi = 0, and thus Ro = 1/gm

Because of the infinite input impedance Rin, then vi = vsig, and the overall voltage gain Gv (also called the total voltage gain) is the same as the voltage gain proper Av (also called terminal voltage gain) Gv = Av = RL/ (RL + 1/gm)

Since 1/gm is typically small, with large RL, the gain is less than unity, but is close to unity. Hence, this is a source follower, because the source voltage follows the input voltage, but yet, it can provide a larger current to the output than the input current.

Cascade Amplifier

Cascade of Self Biased FET

Zi = RG1

Zo =RD2 || rd

Z01 = RD1 || rd || RG2 = RD

AV = AV1 * AV2

= -gm (RD1 || rd) * -gm (RD2|| rd)

=gm2 (RD1 || rd) * (RD2|| rd)

References:

1. Microelectronics Circuits, Adel Sedra and Kenneth C Smith, Oxford University Press, New Delhi, 5th Edition, International Student Edition,2009. (Selected portion of Chapter 2,4, 5, 6, 8, 13, and 14)

2. Electronic Devices and Circuits theory, R.L. Boylestad and L. Nashelsky, Pearson Education, New Delhi, 9th/10th Edition,2013. (Selected portions of Chapter 4, 5, 6, 7, 8, 9, 10, 11, 12, and 14)

3. Milliman’s Electronics Devices and Circuits, J. Milliman, C. Halkias, S. Jit., Tata McGraw Hill