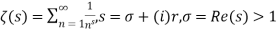Unit-3 SEQUENCES AND SERIES
3.1 Convergence of sequences and series.
Sequences:
Suppose that 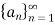 is a sequence. We say that
is a sequence. We say that 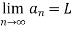 if for every
if for every 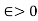 there is an N>0 so that whenever n>N,
there is an N>0 so that whenever n>N,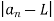 <
<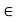 . If
. If  we say that the sequence converges, otherwise it diverges.
we say that the sequence converges, otherwise it diverges.
Example1: Determine whether 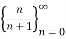 converges or diverges .If it converges, compute the limit.
converges or diverges .If it converges, compute the limit.
Solution: we consider,
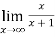
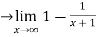 = 1-0 = 1
= 1-0 = 1
Thus the sequence converges to 1.
Example 2: Determine whether 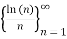 converges or diverges.If it converges, compute the limit.
converges or diverges.If it converges, compute the limit.
Solution:
Now we consider,
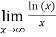

Therefore by using L’Hospital’s rule. The sequence converges to 0.
Example 3: Suppose that 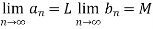 and k is some constant
and k is some constant
Solution: 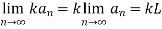
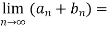
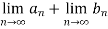 = L+M
= L+M
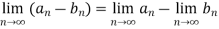 = L – M
= L – M
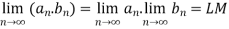
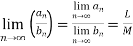 ,if M not equal to zero.
,if M not equal to zero.
Series: A series 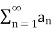 is said to Converge to the sum s if the sequence of partial
is said to Converge to the sum s if the sequence of partial
Sums (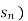
 = 1 converges to s, i.e.,
= 1 converges to s, i.e., 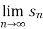 =s.
=s.
*If the sum of the series is finite then the series is convergent.
Ie., 1 -  .........
.........
Example 1: Determine whether the following series converges or diverges
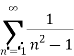
Solution: Consider the given series ie., 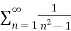
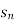 =
= 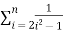 =
= -
-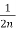 -
-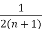
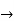
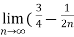 -
-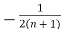 ) =
) = 
Since ,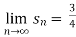
 therefore the given series is convergent.
therefore the given series is convergent.
Example 2: Determine whether the following series is divergent or convergent
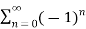
Solution: Given, 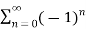
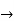 s0 =1
s0 =1
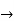 s1 = 1-1=0
s1 = 1-1=0
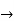 s2 = 1-1+1=1
s2 = 1-1+1=1
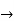 s3 = 1-1+1-1=0
s3 = 1-1+1-1=0
Hence the series diverges since 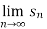 doesn’t exist
doesn’t exist
P-series test:
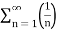 p =
p =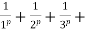 .........
.........
Converges , if p>1
Diverges , if p<1
Example 1:
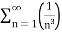 =
= 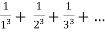
Here p = 3 so p>1 ,thus the given series converges.
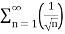 =
= 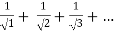
Here p= ie., p<1,thus the given series diverges
ie., p<1,thus the given series diverges
Example 2:
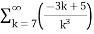
= 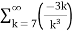 +
+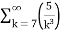
= -3.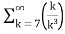 +5.
+5.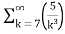
=-3. +5.
+5.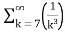
Therefore., here p=2 ,3 ie., p>1
Hence the given series converges
Taylor series:
The taylor’s series can be represented as the following
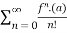 (x-a)n
(x-a)n
Example 1:
Find the taylor series for the following:
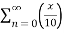
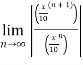 =
= 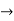
 <1
<1
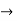 (x/10)<1 and (x/10) > -1
(x/10)<1 and (x/10) > -1
Therefore radius of convergence is (-10,10)
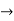 ROC =10
ROC =10
Example 2:
f(n)5 = 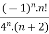
Here the ROC is 4
Exponential Series:
Example 1: 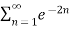 test for convergence
test for convergence
Solution: given f(x) = 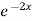
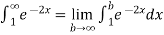
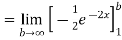
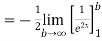
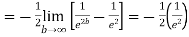
=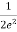
Thus, 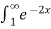 converges so by integral test
converges so by integral test 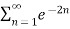 also converges.
also converges.
Example2: Solve for convergence.
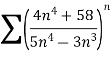
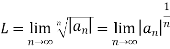
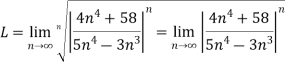
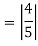
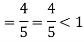
Convergence for Logarithmic:
Example 1: f’(x) = ln(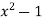 ) ,
) , 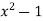 >0 x
>0 x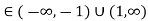
Solution:
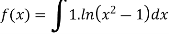
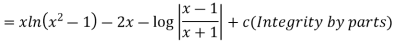

Example2: Solve for convergence of the following
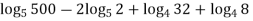
Solution: 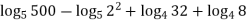
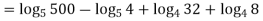
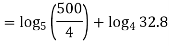
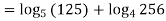

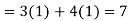
Convergence for trigonometric functions:
Example:1: 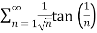 solve for convergence
solve for convergence
Given 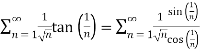
Note: 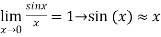
For small x values ,thus for large n’s we have,
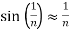
Thus,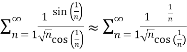
For large n’s 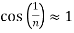
Thus, 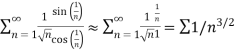
Which clearly converges
Example2: Solve for convergence sin2x
Solution: we have sin2x =2sinx cosx…..(1)
Now, we find the convergence for the given trigonometric function.
Let 2x=u , then x =u/2 now we substitute these values in equation (1)
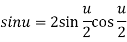
If 0<u<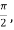 wwe can rewrite this as
wwe can rewrite this as
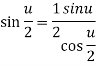
But u< , then
, then 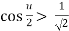 , and therefore
, and therefore
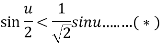
Let u=1. Since sin 1<1 , we find by using (*)
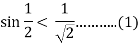
Let u = ½ . By using (*) again , and (1) we find that
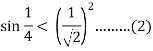
Let u = 1/4 . By using(*) and (2) , we find that
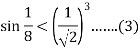
Continue .in general we have
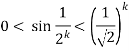
Thus
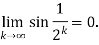
For 0 <x< , the since finction is an increasing function . It follows that
, the since finction is an increasing function . It follows that

Example 1:
Using complex form, find the Fourier series of the function
f(x) = sinnx = 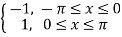
Solution:
We calculate the coefficients 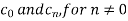
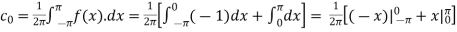
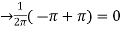
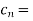
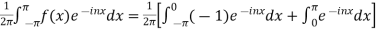
 =
= 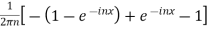
=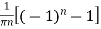
Hence the Fourier series of the function in complex form is
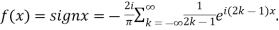
We can transform the series and write it in the real form by renaming as
n=2k-1,n=


= 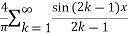
Example 2:
Using complex form find the Fourier series of the function f(x) = x2, defined on te interval [-1,1]
Solution:
Here the half-period is L=1.Therefore, the co-efficient c0 is,
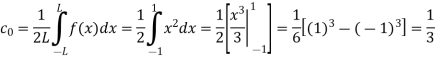
For n
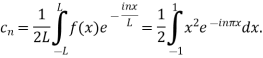
Integrating by parts twice, we obtain
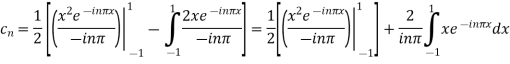
= 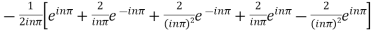
= 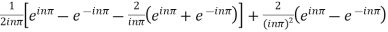
= 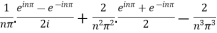 .
.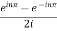
= 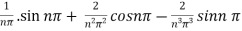 .
.
Statement: If a function has a Fourier Series given by
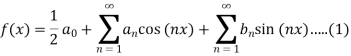
Then Bessel’s inequality becomes an equality known as Parseval’s theorem .From (1)
Proof: We have 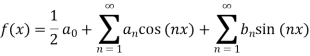
By squaring on both sides the above equation we get the following
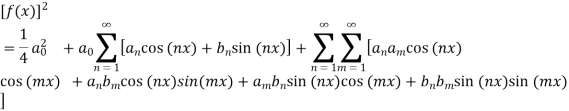
Now by Integrating the above equation we get the following

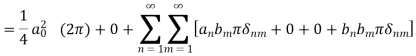
So ,
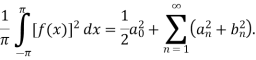
For a generalized Fourier Series of a complete orthogonal system 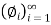 , an analogous relationship holds.
, an analogous relationship holds.
For a complex Fourier series,
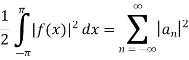
Example : consider  ,
, 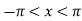
Solution: The Fourier expansion is,
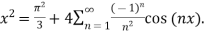
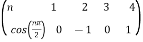
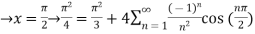
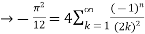
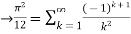
By Parseval’s formulae
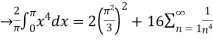
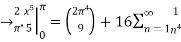
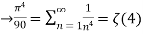
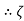 is Reiman Zeta function defined by:
is Reiman Zeta function defined by: