UNIT 1

D.C. Networks

1.1 Introduction

The three essential elements in circuit analysis:

• Resistance R

• Capacitance C

• Inductance L

Their V and I relationships are summarized below

Resistance is a static element in the sense v(t) versus i(t) relationship is instantaneous. For example, v(t) at time t = 2 seconds simply depends only on i(t) at t = 2 seconds and nothing else. This implies that the resistance does not know what happened in the past, in other words it does not store any energy unlike other elements C and L.

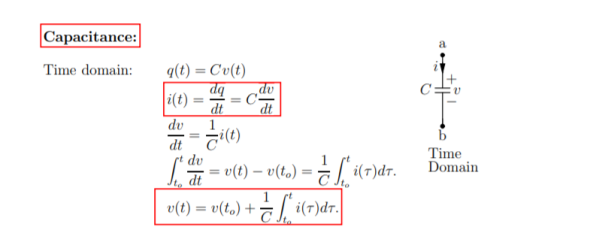
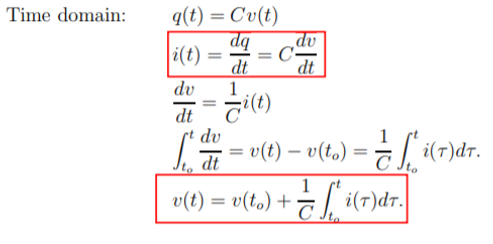
Capacitance: Time domain:

q(t) = Cv(t)

i(t) = dq /dt = C dv/ dt

Dv/dt = 1/C i(t)

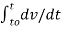= v(t) – v(to) = 1/C   d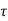

For a capacitance the v(t) versus i(t) relationship and vice versa at any time t depends on the past as they involve differentials and integrals. This implies that the capacitance is a dynamic element.

Inductance:

Time domain v(t) = L di/dt

Di/dt = 1/L v(t)

        = i(t) – i(to) = 1/L     d τ

i(t) = i(to) + 1/L 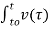  dτ

1.2   Ohm’s law, Kirchhoff’s laws

The algebraic sum of currents meeting at a junction or node in an electric circuit is zero ② or the summation of all incoming current is always equal to summation of all outgoing current in an electrical network.

Explanation

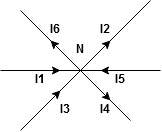
Assuming the incoming current to be positive and outgoing current negative we have

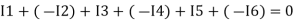
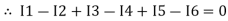
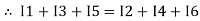
Ie incoming current = ∑ outgoing current thus, the above Law can also be stated as the sum of current flowing towards any junction in an electric circuit is equal to the sum of currents flowing away from that junction

Kirchhoff’s Voltage Law (KVL)

Statement: the algebraic summation of all Voltage in any closed circuit or mesh of loop zero.

Ie; ∑ Voltage in closed loop = 0 the summation of the Voltage rise (voltage sources) is equal to summation of the voltage drops around a closed loop in 0 circuit for explanation from here

Determination of sigh and direction of currents (Don’t write in exams just for understanding)

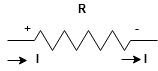
Current entering a resistor is +ve and leaving should be –ve

Now

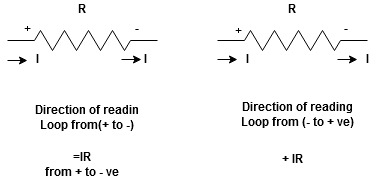
Potential Rise    Potential Drop

We are reading from +V to –V  we are reading from –V to +V

potential drops     potential rise

-V       +V

Given Circuit

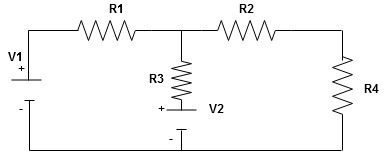
First identify no of loops and assign direction of current flowing in loop

Note: no of loops in circuit = No, of unknown currents = no, of equations in the circuit

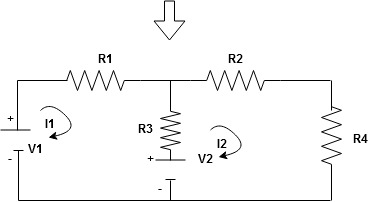
Note: keep loop direction and current direction same ie either clockwise or anticlockwise for all loops I1 I2

Now according to direction of direction assign signs (+ve to –ve) to the resistors

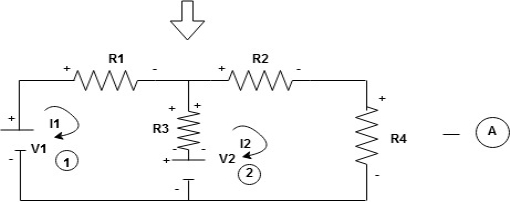
Note: voltage sources (V) polarities do not change is constant.

Note: for common resistor between 2 loops appearing in the circuit like R3 give signs according to separate loops as shown

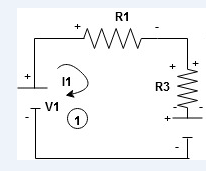
    When considering only loop no 1 (+ R3 - )

-         B

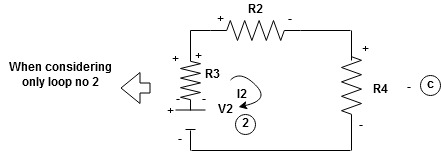
Now consider diagram A and write equations

Two loops two unknown currents two equation

Apply KVL for loop ① [B. Diagram]

(+ To drop -) = - sign and (- to rise +) = + sign

for drop = -sign

for rise = + sign

-

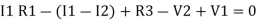
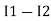-() R2 is considered because in R3,2 currents are flowing 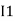and 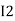  and we have taken (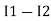) because we are considering loop no 1 and current flowing is 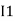in loop no 1

)

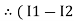Similarly for loop no. 2 currents flowing is 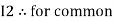resistor R3 it should be 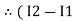)R3

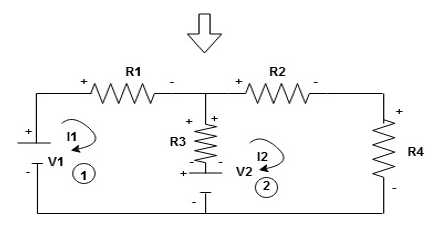
Consider loop no. 1 apply KVL

- …….①

-

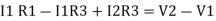
Consider loop no. 2 apply KVL

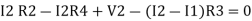-…….②

-

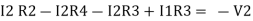
After solving equation ① and ② we will get branch current 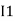and

1.3   Mesh and Nodal analysis

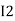
NODE ANALYSIS:

For these we assume every node as a voltage point and write the current equation for every element. For current source, current entering is negative.

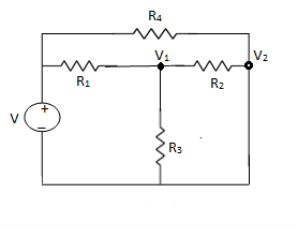
For node V1

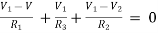
For node V2

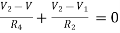
For V

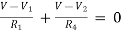
Example: Using nodal analysis find voltage across 5resistor.

Solution:

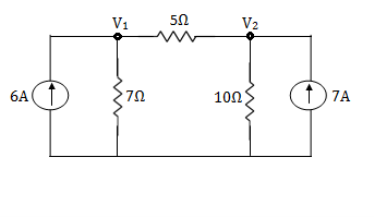
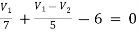
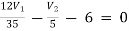
For V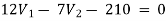1

      1

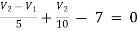
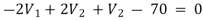
For V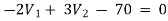2

     2

Solving 1 and 2:

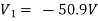
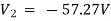
For 5 voltage =

= -50.9 + 57.27

= 6.37V

Mesh current:

• Equation No 1 :    10 =  50I1 + 40I2
• Equation No 2 :    20 =  40I1 + 60I2

Mesh Current Analysis:

An easier method of solving the above circuit is by using Mesh Current Analysis or Loop Analysis which is also sometimes called Maxwell´s Circulating Currents method.

Instead of labelling the branch currents we need to label each “closed loop” with a circulating current.

Any required branch current may be found from the appropriate loop or mesh currents as before using Kirchhoff´s method.

For example: :    i1 = I1 , i2 = -I2  and  I3 = I1 – I2

Therefore,

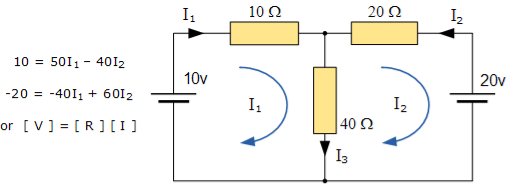
These equations can be solved quite quickly by using a single mesh impedance matrix Z. Each element ON the principal diagonal will be “positive” and is the total impedance of each mesh. Where as, each element OFF the principal diagonal will either be “zero” or “negative” and represents the circuit element connecting all the appropriate meshes.

[ V] = [I] x [R]  or [R] x [I] = [V]

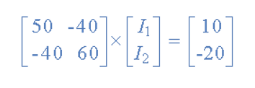
I = V/R = R-1 x V

Inverse of R = [ 60      40

40      50 ]

|R| = (60 x 50 ) – (40 x 40 ) = 1400

R – 1 = 1/1400 [ 60       40

40       50]

Having found the inverse of R, as V/R is the same as V x R-1, we can now use it to find the two circulating currents.

[I] = [ R -1] x [V]

[ I1]= 1/1400  [ 60       40             [10]

[I2 ] =                 40        50 ]    x    [ -20]

I1 = (60 x 10 ) + (40 x -20 ) /1400 = -200/1400 = -0.143 A

I2 = (40 x 10 ) + (50 x -20) /1400 = -600/1400 = -0.429A

Where:

• [ V ]   gives the total battery voltage for loop 1 and then loop 2
• [ I ]     states the names of the loop currents which we are trying to find
• [ R ]   is the resistance matrix
• [ R-1 ]   is the inverse of the [ R ] matrix

And this gives I1 as -0.143 Amps and I2 as -0.429 Amps

As :    I3 = I1 – I2

The combined current of I3 is therefore given as :   -0.143 – (-0.429) = 0.286 Amps

1.4   Superposition theorem, (only independent sources)

Statement:

The response in any element of the linear bilateral network containing more than one source is the sum of the responses produced by the individual source acting alone. The responses mean the voltage across the element or the current in the element the voltage sources should be replaced by a short circuit and the current source must be replaced by an open circuit.Steps to apply superposition theorem :

1.

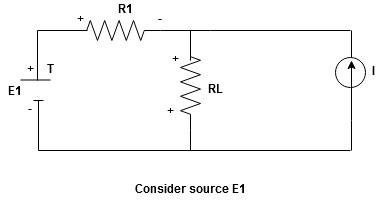
Current sources should be open

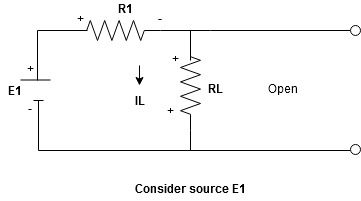
Find the current (IL1)(+) through or the voltage across the required element due to the source under consideration using a suitable n/w technique.

1. Consider the current source (I) Voltage source should be a short circuit 

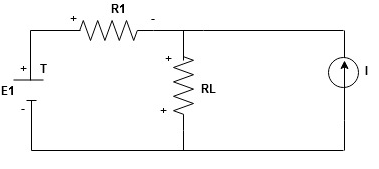
Find the current (ILII)(+) by a suitable network simplification technique

2.     Add the individual current IL and ILII by the individual sources acting alone to find the total current flowing in load resistance (RL)

IeL =L + L 

1.5   Definitions of MMF, Magnetic field strength, Reluctance, Leakage flux and fringing

1. Magnetic motive force MMF: It is the force that is required for production of flux

MMF = No. Of turns x current

= N x I

= Ampere Turns

=AT

2.    Magnetic field strength / intensity / magnetising force:

It is the force experienced by a unit north poles placed at that point in the magnetic field Denoted by H

Measured in Newtown / w­b or AT/n

H =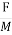  N/wb

H =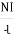

3.     Reluctance:

It is defined as the opposition to the flow of flux in a material

Reluctance is denoted by S and measured in AT/wb or Siemens

S = ɺ/ μ₀μᵣₐ         - AT/wb

It can also be defined as the ratio of (MMF) to the magnetic flux (Ø)

S = 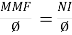     - AT/wb

4.    Leakage coefficient:

The leakage coefficient or leakage factor indicates the percentage or part of total flux lost due to leakage. It is defined as the ratio of total flux (Ø7) to useful / air gap flux Øu

Leakage factor (h) =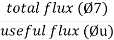

It is also called as Hopkinson’s coefficient value is from 1.1 to 1.25

5.    Magnetic fringing :

The tendency of the magnetic flux to spread out at the edges of the air gap is called magnetic fringing

6.    Effect of fringing:

1. Increases cross sectional area of airgap
2. the flux density (B) is reduce

1.6   Core losses

Core loss is broadly classified into:

Hysteresis loss

Eddy current loss

Hysteresis Loss:

The loss occurs due to reversal of armature core is said to be hysteresis loss. Each part of the rotor core passes under the North Pole N and South Pole S alternately and attains S and N polarity, respectively. When the core passes under one pair of poles, the core is said to complete one cycle of frequency reversal. Hysteresis loss ∝  B maxn f.

Eddy current Loss:

The armature core cuts the magnetic flux during its rotation and emf is induced in the surface of the core, according to the laws of electromagnetic induction. This emf is very small, but it sets up a large current in the surface of the core. This lager current is called the eddy current and the loss is known as the eddy current loss. Eddy current loss ∝ Bmax2 f2.

Core losses are constant for shunt and compound generators, as their field currents are approximately constant. Core loss is about 20 % to 30 % of full-load losses.

1.7   Comparison of the Electric and Magnetic Circuits

Electric       magnetic

1. Current is actual flow of electrons  1. Flux does not flow in the mag circuit
2. Energy is required to produce the  2. Energy is required to produce

Current and maintain it   flux but not for its maintenance

3.        Current does not pass through air  3. Flux can pass through air

4.        Resistance is independent of the  4. Reluctance of current changes with of the current                                                              flux

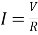5.             5.

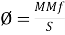
6.        Current is reduced to zero after  6. Residual flux persists after removal of removal of emf                            mmf

7.              7.

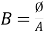
Current density           Flux density

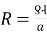8.                 8.

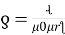
9.        Conductance =         9. Permeance =

10.   Electric field Intensity 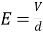     10. Magnetic filed Intensity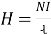

11.   emf drop = I x R       11. Mmf drop = Ø x S

1.8 Problems on Series Magnetic Circuits

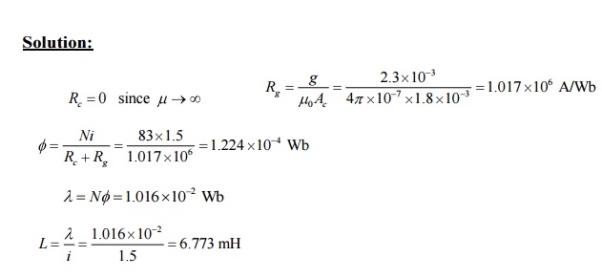
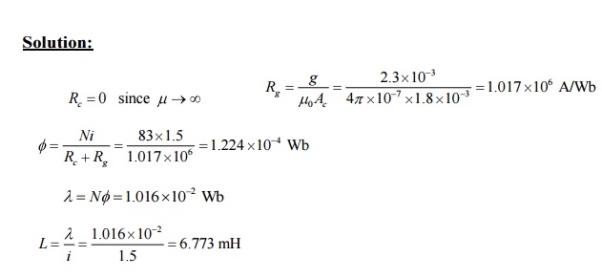
Eg .No.1

A magnetic circuit with a single air gap is shown in below figure. The core dimensions are:

Cross-sectional area Ac = 1.8 × 10-3 m2

Mean core length lc = 0.6 m

Gap length g = 2.3 x 10-3 m

N = 83 turns

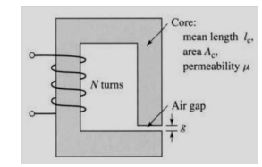
Assume that the core is of infinite permeability ( m -> ¥) and neglect the effects of fringing fields at the air gap and leakage flux. (a) Calculate the reluctance of the core

Re and that of the gap Rg. For a current of i = 1.5 A, calculate (b) the total flux ϕ, (c) the flux linkages λ of the coil, and (d) the coil inductance L.

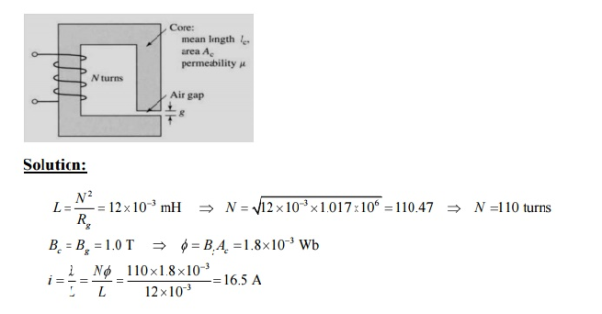
Eg .No.2

Consider the magnetic circuit of with the dimensions of Problem 1.1. Assuming density of 1.0 T.infinite core permeability, calculate (a) the number of turns required to achieve an inductance of 12 mH and (b) the inductor current which will result in a core flux