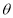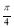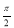Module 4
Multivariable Calculus-I
Double Integral
Consider a function f (x, y) defined in the finite region R of the X-Y plane. Divide R into n elementary areas A1, A2,…,An. Let (xr, yr) be any point within the rth elementary are Ar
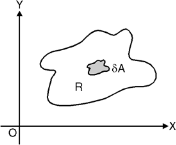
Fig. 6.1
f (x, y) dA = f (xr, yr) A
Evaluation of Double Integral when limits of Integration are given(Cartesian Form).
Ex. 1: Evaluate 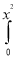 ey/x dy dx.
ey/x dy dx.
Soln. :
Given : I = 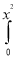 ey/x dy dx
ey/x dy dx
Here limits of inner integral are functions of y therefore integrate w.r.t y,
I = 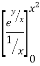
 dx
dx
= 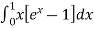
= 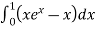
I = 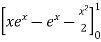
= =
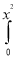 ey/x dy dx =
ey/x dy dx =
Ex. 2 : Evaluate x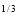 y
y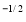 (1 – x –y)
(1 – x –y)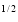 dx dy.
dx dy.
Soln. :
Given : I = x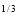 y
y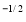 (1 – x –y)
(1 – x –y) dx dy.
dx dy.
Here the limits of inner integration are functions of y therefore first integrate w.r.t y.
I = x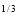 dx
dx 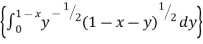
Put 1 – x = a (constant for inner integral)
I = x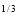 dx
dx 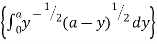
put y = at dy = a dt
y | 0 | a |
t | 0 | 1 |
I = x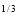 dx
dx 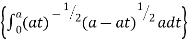
I = x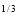 dx
dx 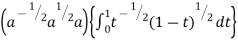
I = x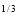 a dx
a dx
I = x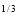 (1 – x) dx = (x
(1 – x) dx = (x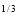 – x4/3) dx
– x4/3) dx
I = 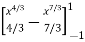
=
I = =
x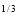 y
y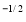 (1 – x –y)
(1 – x –y)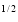 dx dy =
dx dy =
Ex. 3: Evaluate 
Soln. :
Let, I = 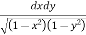
Here limits for both x and y are constants, the integral can be evaluated first w.r.t any of the variables x or y.
I = dy 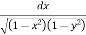
I = 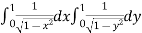
= 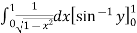
= 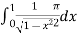
= 
=
= 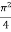
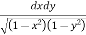 =
=
Ex. 4: Evaluate e–x2 (1 + y2) x dx dy.
Soln. :
Let I = e–x2 (1 + y2) x dy = dy e–x2 (1 + y2) x dy
= dy e– x2 (1 + y2)  dx
dx
= dy [∵ f (x) ef(x) dx = ef(x) ]
= (–1) dy (∵ e– = 0)
= = =
e–x2 (1 + y2) xdx dy =
NOTES:
Type II: Evaluation of Double Integral when region of Integration is provided (Cartesian form)
Ex.1: Evaluate y dx dy over the area bounded by x= 0 y = 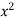 and x + y = 2 in the first quadrant
and x + y = 2 in the first quadrant
Soln. :
The area bounded by y = x2 (parabola) and x + y = 2 is as shown in Fig.6.2
The point of intersection of y = x2 and x + y = 2.
Fig. 6.2
x + x2 = 2 x2 + x – 2 = 0
x = 1, – 2
At x = 1, y = 1 and at x = –2, y = 4
(1, 1) is the point of intersection in the Ist quadrant. Take a vertical strip SR, Along SR x constant and y varies from S to R i.e. y = x2 to y = 2 – x.
Now slide strip SR, keeping IIel to y-axis, therefore y constant and x varies from x = 0 to x = 1.
I = 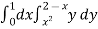
= 
= 
= (4 – 4x + 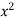 –
– 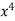 ) dx
) dx
= =
I = 16/15
Ex. 2: Evaluate 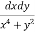 over x 1, y
over x 1, y 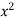
Soln. :
Let I = 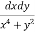 over x 1, y
over x 1, y 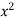
The region bounded by x 1 and y 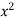
is as shown in Fig. 6.3.
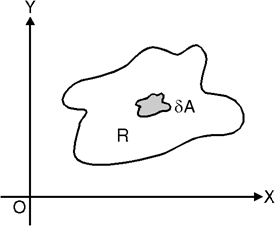
Fig. 6.3
Take a vertical strip along strip x constant and y varies from y = 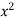
to y = . Now slide strip throughout region keeping parallel to y-axis. Therefore y constant and x varies from x = 1 to x = .
I = 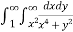
= 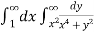
= 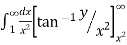 [ ∵
[ ∵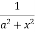 dx = tan–1 (x/a)]
dx = tan–1 (x/a)]
= 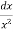 =
=
= – = (0 – 1)
I =
Ex. 3 : Evaluate (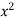 +
+ 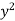 ) dx dy through the area enclosed by the curves y = 4x, x + y = 3 and y =0, y = 2.
) dx dy through the area enclosed by the curves y = 4x, x + y = 3 and y =0, y = 2.
Soln. :
Let I = (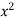 +
+ 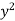 ) dx dy
) dx dy
The area enclosed by the curves y = 4x, x + y =3, y = 0 and y = 2 is as shown in Fig. 6.4.
(find the point of intersection of x + y = 3 and y = 4x)

Fig. 6.4
Take a horizontal strip SR, along SR y constant and x varies from x = to x = 3 – y. Now slide strip keeping IIel to x-axis therefore x constant and y varies from y = 0 to y = 2.
I = dy (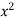 +
+ 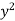 ) dx
) dx
= 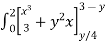
= 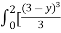 +
+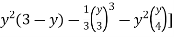 dy
dy
I = 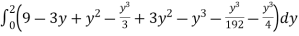
= 
= 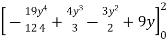
= + – 6 + 18
I =
Triple Integration
Definition: Let f(x,y,z) be a function that is continuous at every point of the finite region (Volume V) of three-dimensional space. Divide the region V into n sub-regions of respective volumes . Let (
. Let (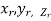 ) be a point in the
) be a point in the 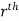 sub-region then the sum:
sub-region then the sum:
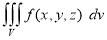 =
= 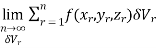
is called triple integration of f(x, y, z) over the region V provided a limit on R.H.S of the above Equation exists.
Spherical Polar Coordinates
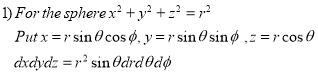
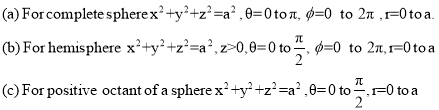
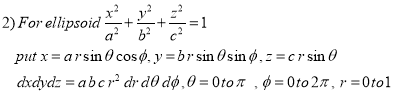
Where the integral is extended to all positive values of the variables subjected to the condition 
Ex.1: Evaluate
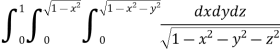
Solution: Let
I = 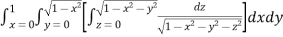
= 
(Assuming m = 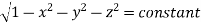 )
)
= 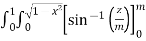 dxdy
dxdy
= 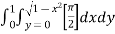
=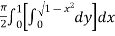
= 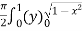 dx
dx
= 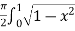 dx
dx
= 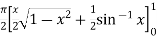
=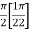
I =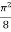
Ex.2: Evaluate 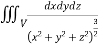 Where V is annulus between the spheres
Where V is annulus between the spheres 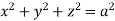
and 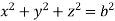 (
(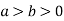 )
)
solution: It is convenient to transform the triple integral into spherical polar co-ordinate by putting
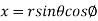 ,
,  ,
, 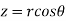
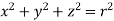 , dxdydz=
, dxdydz=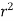 sin
sin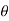 drd
drd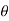 d
d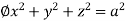 ,
,
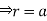 and
and 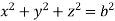
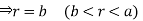
For the positive octant, r varies from r =b to r =a, 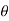 varies from
varies from 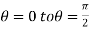
and 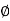 varies from
varies from 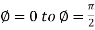
I= 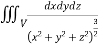
= 8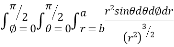
=8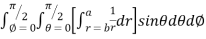
=8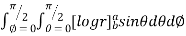
=8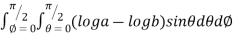
=8 log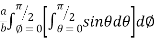
= 8 log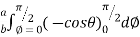
I= 8 log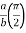 I = 4 log
I = 4 log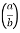
Ex.3: Evaluate 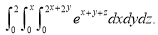
Solution:-
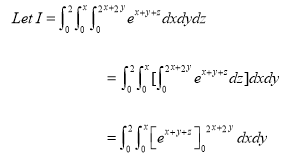
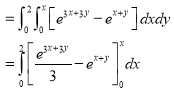
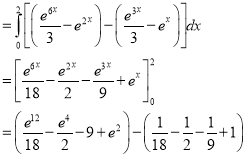
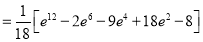
Ex.4: Evaluate 
Solution:- 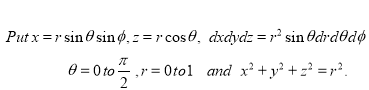

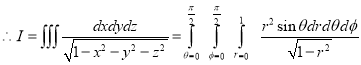
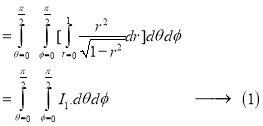
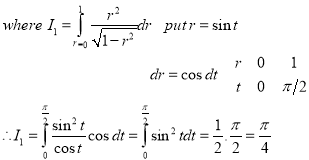
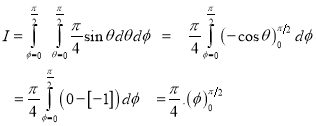
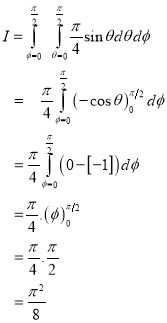
NOTES:
MASS OF A LAMINA:- If the surface density ρ of a plane lamina is a function of the position of a point of the lamina, then the mass of an elementary area dA is ρ dA and the total mass of the lamina is 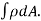
In Cartesian coordinates, if ρ = f(x, y) the mass of lamina, M=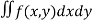
In polar coordinates, if ρ = F(r, Ө) the mass of lamina, M=
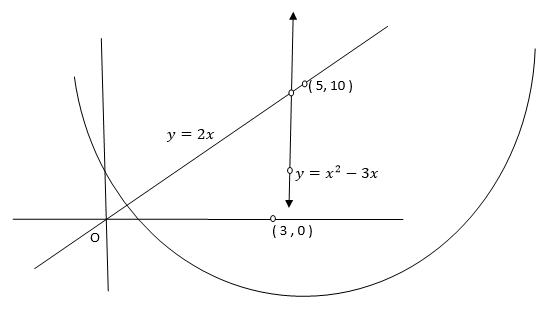
Ex.1: A lamina is bounded by the curves 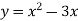 and
and 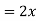 . If the density at any point is
. If the density at any point is 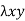 then find the mass of lamina.
then find the mass of lamina.
Solution:

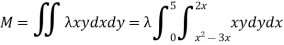
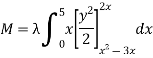
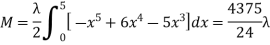
Ex.2: If the density at any point of a non-uniform circular lamina of radius’ a’ varies as its distance from a fixed point on the circumference of the circle then find the mass of lamina.
Solution:
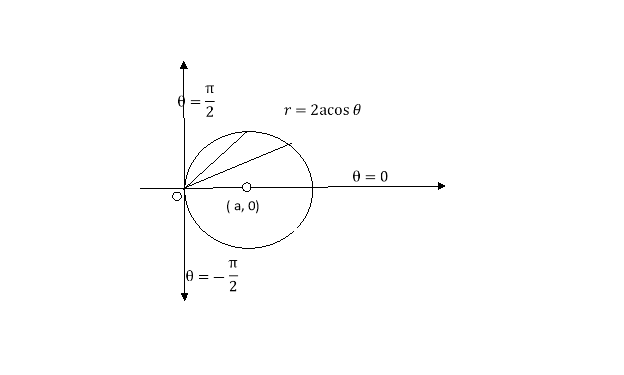
Take the fixed point on the circumference of the circle as origin and diameter through it as the x-axis. The polar equation of circle 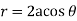
And density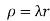 .
.
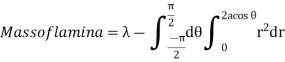
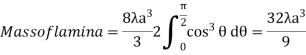
Mean Value:
The mean value of the ordinate y of a function 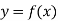 over the range
over the range 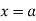 to
to 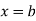 is the limit of the mean value of the equidistant ordinates
is the limit of the mean value of the equidistant ordinates 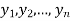 as
as 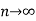
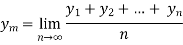
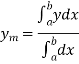
Mean Square values of function 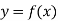 over the range
over the range 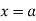 to
to 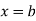 is defined as
is defined as
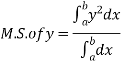
Mean Square values of function 
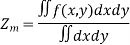
Mean Square values of function 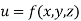
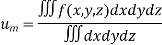
Root Mean Square Value: (R.M.S. value):
If y is a periodic function of x of period p, the root mean square value of y is the square root of the mean value of 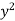 over the range
over the range 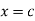 to
to 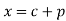 , c is constant.
, c is constant.
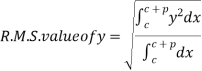
Ex. 1: Find the mean value and R.M.S. value of the ordinate of the cycloid
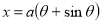 ,
, 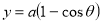 over the range
over the range 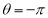 to
to 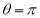 .
.
Sol Let P(x,y) be any point on the cycloid. Its ordinate is y.
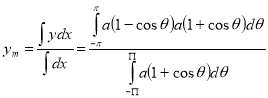
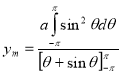
= 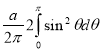
=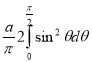
=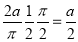
R.M.S.Value= 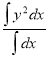
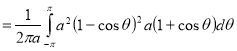
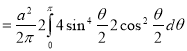
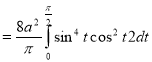
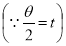

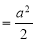

Ex. 2: Find The Mean Value of  Over the positive octant of the
Over the positive octant of the
Ellipsoid 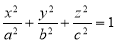
Sol: M.V.=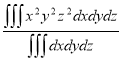
Since 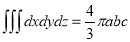
Put 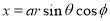
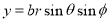
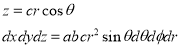
M.V.=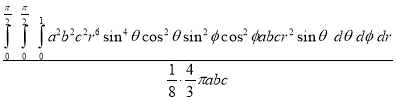

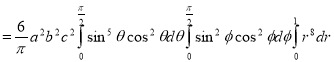
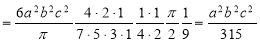
Areas and Volume
The volume of solid is given by
Volume =
In Spherical polar system
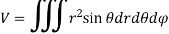
In the cylindrical polar system
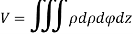
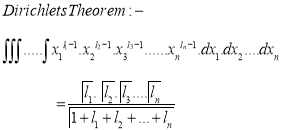
Ex.1: Find Volume of the tetrahedron bounded by the co-ordinates planes and the plane
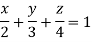
Solution: Volume =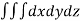 ………. (1)
………. (1)
Put 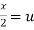 ,
, 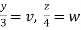
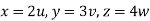
From equation (1) we have
V = 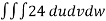
=24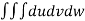
=24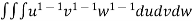 (u+v+w=1) By Dirichlet’s theorem.
(u+v+w=1) By Dirichlet’s theorem.
=24 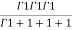
=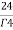 =
=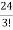 = 4
= 4
Volume =4
Ex.2: Find volume common to the cylinders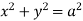 ,
, 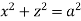 .
.
Solution: For given cylinders,
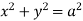 ,
, 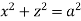 .
.
Z varies from
Z=-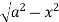 to z =
to z = 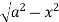
Y varies from
y= -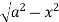 to y =
to y = 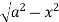
x varies from x= -a to x = a
By symmetry,
Required volume= 8 (volume in the first octant)
=8 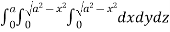
=8
= 8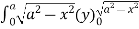 dx
dx
=8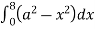
=8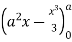
=8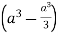
Volume = 16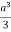
Ex.3: Evaluate
1. 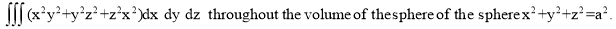 Solution:-
Solution:-
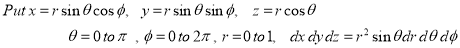
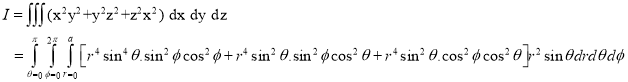

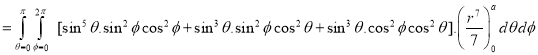
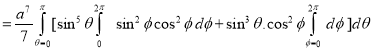
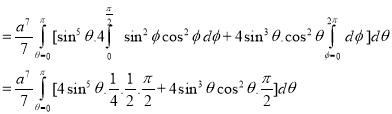
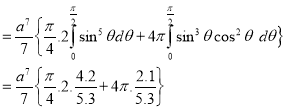
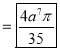
Ex.4: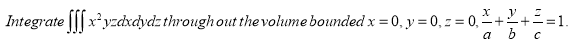
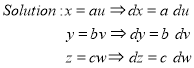
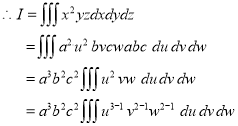

Ex.5:Evaluate 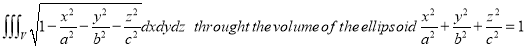
Solution:- Put
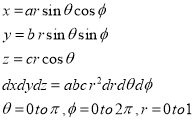
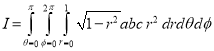
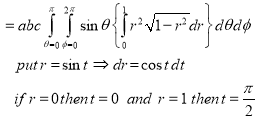
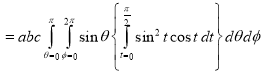
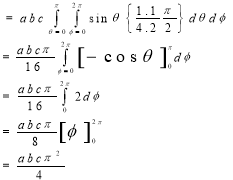
Center of Mass and gravity
DEFINITION:
Center of mass:-The center of mass of a body is the point through which the resultant mass acts.
Center of gravity:-The center of gravity (C.G.) of a body is the point through which the resultant weight acts. Since weight is proportional to mass, the center of a mass is the same point as the center of gravity.
C.G. of an arc of a curve;
The C.G. 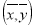 of the arc of a curve is given by
of the arc of a curve is given by
 ;
;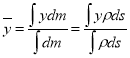
Where 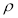 is density.
is density.
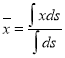 ;
;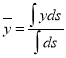 it
it 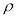 is constant.
is constant.
Remark:
1. If 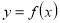 then
then 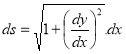
2. If 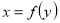 then
then 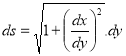
3. If 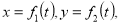 then
then 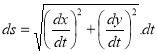
4. If 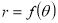 then
then 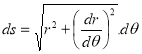
5. If 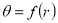 then
then 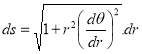
C.G. of a plane area or Lamina:
Let  be the co-ordinates of C.G. of the lamina then;
be the co-ordinates of C.G. of the lamina then;
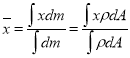 ;
;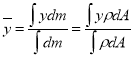
Or in double integral form, Cartesian system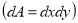
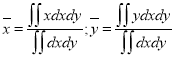
If the curve is given in Polar coordinates,
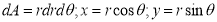 then
then
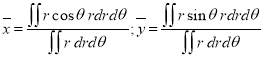
Ex.1: A lamina bounded by the parabolas 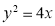 and
and 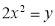 has a variable density
has a variable density 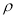
Given by 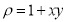 . Prove that
. Prove that 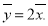
Solution: The points of intersection of two parabolas are (0,0), (1,2), density 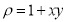 (given)
(given)
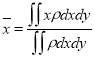 ,
, 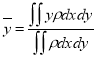
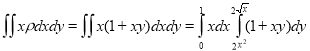
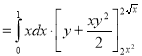
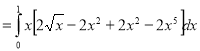
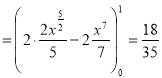 ----------------------------------------(i)
----------------------------------------(i)
Diagram
 dxdy=
dxdy=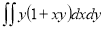
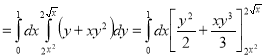

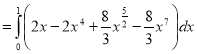
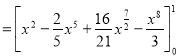
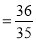 ------------------------------------------(ii)
------------------------------------------(ii)
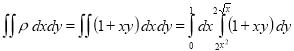
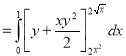
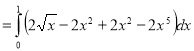

=1 ----------------------------------------------(iii)
From (i),(ii) and (iii), 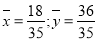

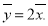
Ex.2: Find the Center of gravity of one of the loops of r =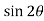
Solution: The curve r=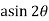 is four-leaved rose lies within the circle r=a,
is four-leaved rose lies within the circle r=a,
Consider a loop lies between 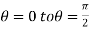 as shown in fig.
as shown in fig.
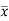 =
=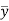
 =
=  …….. (1)
…….. (1)
Where N=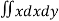
=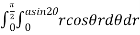
=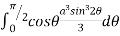
=
=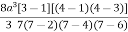 1
1
= 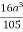 ………..(2)
………..(2)
D=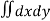
=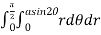
=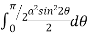
= 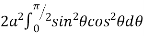
= 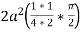
= 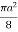 …………………..(3)
…………………..(3)
From Equations (1), (2) and (3)
we have 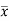 =
=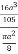 =
=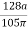 , also
, also 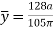
Ex .2: find the centroid of the loop of the curve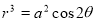 .
.
Sol. C.G lies on x-axis, Therefore,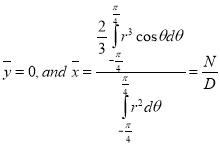
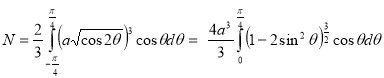

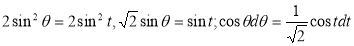
| 0 |
|
t | 0 |
|
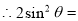
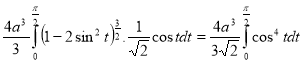
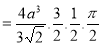

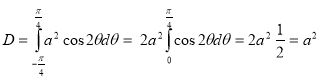
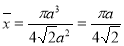
C.G. is 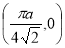
Reference Books-
1.E. Kreyszig, Advanced Engineering Mathematics, John Wiley & Sons, 2005.
2.Peter V. O’Neil, Advanced Engineering Mathematics, Thomson (Cengage) Learning, 2007.
3.Maurice D. Weir, Joel Hass, Frank R. Giordano, Thomas, Calculus, Eleventh Edition, Pearson.
4.D. Poole, Linear Algebra: A Modern Introduction, 2nd Edition, Brooks/Cole, 2005.
5.Veerarajan T., Engineering Mathematics for the first year, Tata McGraw-Hill, New Delhi, 2008.
6.Ray Wylie C and Louis C Barret, Advanced Engineering Mathematics, Tata Mc-Graw-Hill, Sixth Edition.
7.P. Sivaramakrishna Das and C. Vijayakumari, Engineering Mathematics, 1st Edition, Pearson India Education Services Pvt. Ltd
8. Advanced Engineering Mathematics. Chandrika Prasad, Reena Garg, 2018.
9. Engineering Mathematics – I. Reena Garg, 2018.