Unit - 1

Partial differential equation

1.1 Origin of Partial Differential Equations

A differential equation involving partial derivatives with respect to more than one independent variable is called a partial differential equation.

The independent variables will be denoted by x and y and the dependent variable by z. The partial differential coefficients are denoted as-

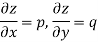
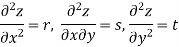
ORDER of a partial differential equation is the same as that of the order of the highest differential coefficient in it.

Classification of the partial differential equation-

Suppose the equation is-

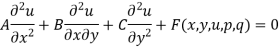
Here A, B, C are the constants of x and y, then the equation-

1. Elliptical- if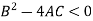

2. Parabolic- if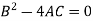

3. Hyperbolic- if if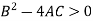

Formation of the partial differential equation

Method of elimination of arbitrary constants

Example: Form a partial differential equation from-

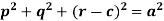
Sol.

Here we have-

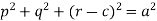
It contains two arbitrary constants a and c

Differentiate the equation with respect to p, we get-

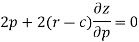
Or

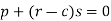
Now differentiate the equation with respect to q, we get-

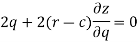
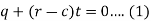
Now eliminate ‘c’,

We get

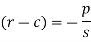
Now put z-c in (1), we get-

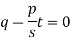
Or

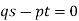
The second method we use is a method of elimination of arbitrary functions.

The solution of partial differential equation by direct partial Integration-

Example: Solve-

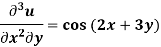
Sol.

Here we have-

Integrate w.r.t. x, we get-

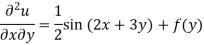
Integrate w.r.t. x, we get-

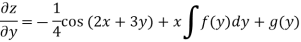
Integrate w.r.t. y, we get-

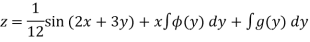
Example: Solve the differential equation-

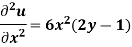
Given the boundary condition that-

At x = 0,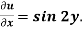

Sol.

Here we have-

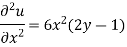
On integrating partially with respect to x, we get-

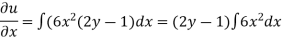
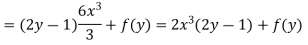
Here f(y) is an arbitrary constant.

Now form the boundary condition-

When x = 0,

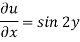
Hence-

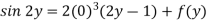
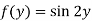
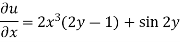
On integrating partially w.r.t.x, we get-

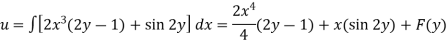
Example: Solve the differential equation-

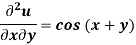
Given that 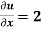when y = 0, and u = when x = 0.

Sol.

We have-

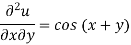
Integrating partially w.r.t. y, we get-

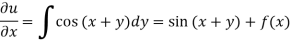
Now from the boundary conditions,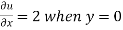

Then-

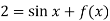
From which,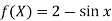
 

It means,

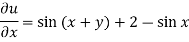
On integrating partially w.r.t. x givens-

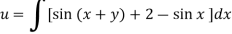
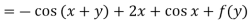
From the boundary conditions, u = 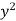when x = 0

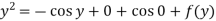
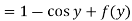
From which-

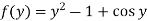
Therefore, the solution of the given equation is-

1.2 Lagrange’s Equations, linear and Non-Linear Partial Equations of the first order

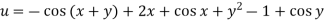
Linear Equations of the First Order

A linear partial differential equation of the first order, commonly known as Lagrange’s Linear equation is of the form

Pp + Qq = R       (1)

Where, P, Q, and R are functions of x, y, z. This equation is called a quast linear equation. When P, Q, and R are independent of z it is known as a linear equation.

Such an equation is obtained by eliminating an arbitrary function 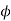from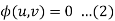

Where u,v are some functions of x, y, z.

Differentiating (2) partially with respect to x and y

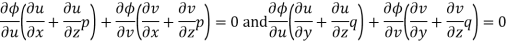
Eliminating 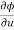and 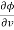, we get

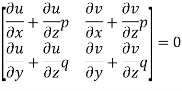
Which simplifies to

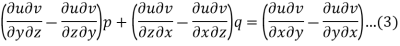
This is of the same form as (1)

Now suppose u = a and v=b, where a, b are constants, so that

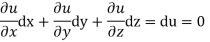
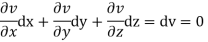
By cross multiplication we have,

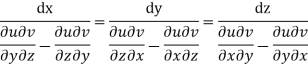
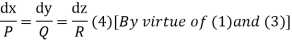
The solution of these equations are u = a and v = b

Therefore, 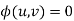is the required solution of (1).

Thus to solve the equation Pp + Qq =R.

(i)form the subsidiary equations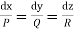

(ii) Solve these simultaneous equations

(iii) write the complete solution as 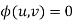or u=f(v)

Method of multipliers-

Let the auxiliary equation be

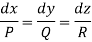
L, m, n may be the constants of x, y, z then we have-

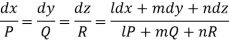
L, m, n are selected in such a way that-

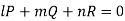
Thus

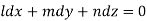
On solving this differential equation, if the solution is- u =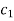

Similarly, choose another set of multipliers 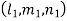and if the second solution is v =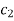

So that the required solution is f(u, v) = 0.

Example. Solve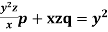  

Solution. Rewriting the given equation as

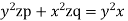
The subsidiary equations are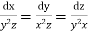

The first two fractions give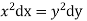

Integrating we get 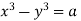n  (i)

Again the first and third fraction give xdx = zdz

Integrating, we get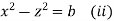

Hence from (i) and (ii), the complete solution is

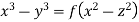
Example. Solve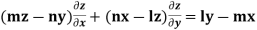

Solution. Here the subsidiary equations are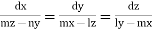

Using multipliers x,y, and z we get each fraction =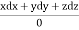

which on integration gives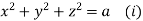

Again using multipliers l, m and n we get each fraction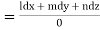

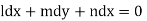which on integration gives lx +my +nz = b   (ii)

Hence from (i) and (ii), the required solution is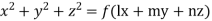

Example. Solve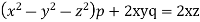

Solution. Here the subsidiary equations are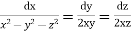

From the last two fractions, we have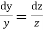

Which on integration gives log y = log z + log a or y/z=a   (i)

Using multipliers x, y and z we have

Each fraction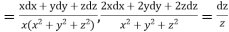

Which on integration gives

Hence from (i) and (ii), the required solution is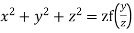

Example: Solve-

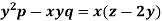
Sol.

We have-

Then the auxiliary equations are-

Consider the first two equations only-

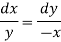
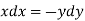
On integrating

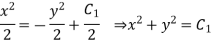
…….. (2)

Now consider the last two equations-

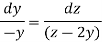
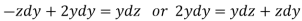
On integrating we get-

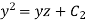
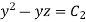…………… (3)

From equation (2) and (3)-

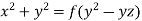
Example: Find the general solution of-

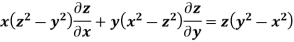
Sol. The auxiliary simultaneous equations are-

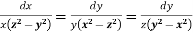……….. (1)

Using multipliers x, y, z we get-

Each term of (1) equals to-

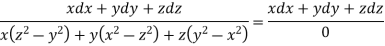
Xdx + ydy + zdz=0

On integrating-

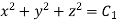………… (2)

Again equation (1) can be written as-

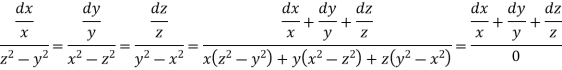
Or

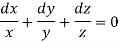
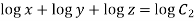
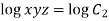
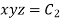………….. (3)

From (2) and (3), the general solution is-

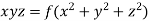
Non-linear partial differential equations-

Type-1: Equation of the type f(p, q) = 0

Method-

Let the required solution is-

Z = ax + by + c …….. (1)

So that-

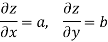
On putting these values in f(p, q) = 0

We get-

f(a, b) = 0

So from this, find the value of b in terms of a and put the value of b in (1). It will be the required solution.

Type-2: Equation of the type-

Z = px + qy + f(p, q)

Its solution will be-

Z = ax + by + f(a, b)

Type-3: Equation of the type f(z, p, q) = 0

Type-4: Equation of the type-

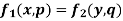
Method-

Let-

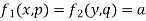
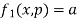, solve it for p. Let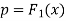

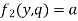, solve it for q. Let q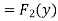

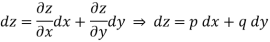
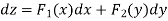
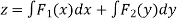
Example: Solve-

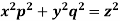
Sol.

This equation can be transformed as-

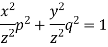
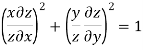
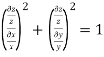……. (1)

Let

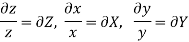
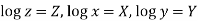
Equation (1) can be written as-

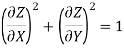
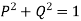………… (2)

Let the required solution be-

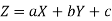
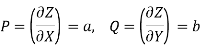
From (2) we have-

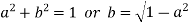
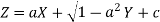
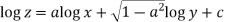
Example: Solve-

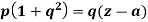
Sol.

Let u = x + by

So that-

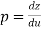and

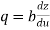
Put these values of p and q in the given equation, we get-

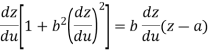
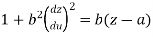or

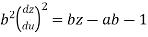
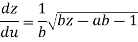
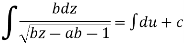
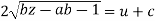
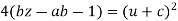
Example: Solve-

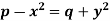
Sol.

Let-

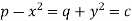
That means-

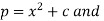
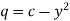
Put these values of p and q in

1.3 Charpit’s method

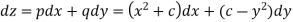
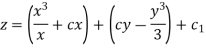
This is the general method for finding the complete integral of a non-linear partial differential equation.

Let us consider the equation-

f(x, y, z , p, q) = 0 ………. (1)

Since z depends on x and y, we have-

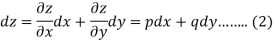
The main thing in Charpit’s method is to find another relationship between the variables x, y, z, and p. q.

And let the relation be-

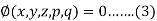
Here on solving equations (1) and (2), we get the values of p and q.

When we substitute these values of p and q in (2), it becomes integrable.

To determine 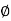, equations (1) and (3) are differentiated with respect to x and y.

We get, when we differentiate with respect to x,

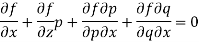
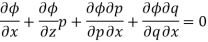
We get, when we differentiate with respect to y,

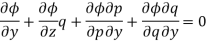
Eliminating 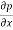between the equations we get from differentiating for x, we get

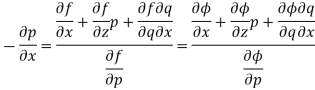
Or

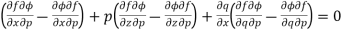…………(4)

Eliminating 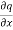between the equations we get from differentiating for y, we get

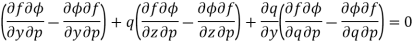
…………(5)

Adding (4) and (5) and keeping in view the relation on, the terms of the last brackets of (4) and (5) cancel. On rearranging, we get-

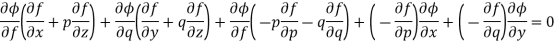
Or

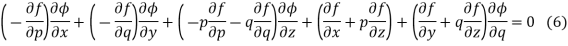
This equation is Lagrange’s linear equation of the first order with x, y, z, p, q as independent variables and equation (4) as the dependent variable.

Its subsidiary equations are-

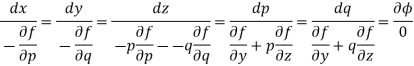
An integral of these equations involving p or q or both can be taken as the relation (3) which along with (1) will give the values of p and q to make (2) integrable.

Example: Solve-

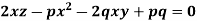
Sol.

Let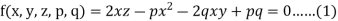
 

Charpit’s subsidiary equations are-

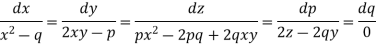
So that- dq = 0 or q = a

On putting q = a in (1) we get-

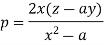
Such that-

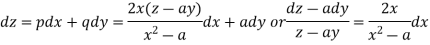
Integrating

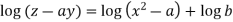
Or

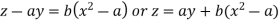
Which is the required solution.

Example: Solve-

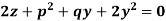
Sol.

Let

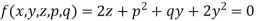
Charpit’s subsidiary equations are-

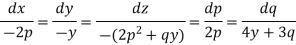
From the first and fourth ratios,

Substituting p = a – x in the given equation, we get-

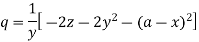
So that-

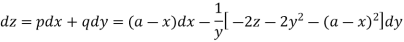
Multiply both sides by ,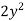

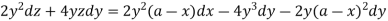
Integrating-

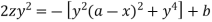
Or

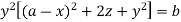
Which is the required solution.

1.4 Cauchy’s method of Characteristics

Consider the following partial differential equation-

Rr + Ss + Tt + f(x, y, z, p, q) = 0. ....... (1)

Here R, S, and T are the functions of x and y only.

Now suppose we need to find the solution of (1) such that on a given space curve C, it takes the prescribed value of z and 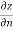where n is a distance measured along the normal to the problem.

Then the Cauchy’s problem can be defined as below-

To find the solution of

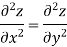
With the following data prescribed on the x-axis-

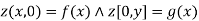
Where the y-axis is normal to the given curve.

Let for equations (1), is given by-

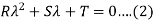
Where 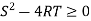has real roots,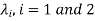

Then the ordinary differential equation is given by-

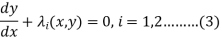
Are called characteristic equations.

The solution of the equation (3) is called characteristic of the differential equation (1).

Now three cases arise,

Case-1- if 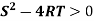[when given partial differential equation is hyperbolic]

In this case, equation (2) has two distinct real roots, say, then we have the following two characteristic equations

On solving these two equations, we get two distinct families of curves.

That means we get two characteristic curves.

Case-2- if 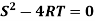[when given partial differential equation is parabolic]

In this case, equation (2) has two real and equal roots. Thus, we get only one characteristic equation given by

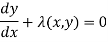
On solving this, we get only one family of characteristics.

Case-3- if 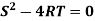[when given partial differential equation is elliptic]

In this case, the equation has complex roots so there are no real roots and hence no real characteristic.

We get two families of complex characteristics

Example: Find the characteristics of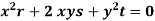

Sol.

Here we have-

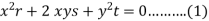
Now comparing (1) with-

Rr + Ss + Tt + f(x, y, z, p, q) = 0

We get-

R =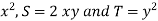

Now we see that-

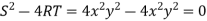
Hence the equation is parabolic.

The 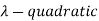is given by-

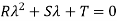
Putting the values of R, S, and T in this, we get-

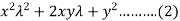
Now simplifying (2), we get-

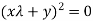
Solving it we get the repeated roots given by

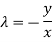
Therefore we get only ne family of the characteristic of (1).

The characteristic equation of (1) is-

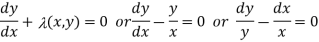
On integrating, we get-

Log y – log x = log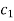

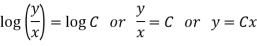
Which is the required family of characteristics and it represents a family of straight lines passing through the origin.

1.5 Solution of Linear Partial Differential Equation of Higher-order with constant coefficients

An equation of the type

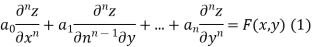
Is called a homogenous linear partial differential equation of nth order with constant coefficients

It is called homogenous because all the terms contain derivatives of the same order.

Putting,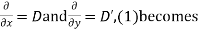

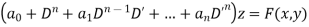
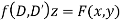
Rules for finding the Complementary function

Consider the equation

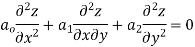
Or

First step: Put D = m and D’ = 1

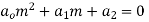
This is the auxiliary equation.

2ndStep: Solve the auxiliary equation.

Case1. If the roots of the auxiliary equations are real and different say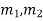

Then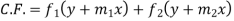

Case2. If the roots are equal say m,

Then,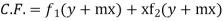

Example. Solve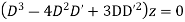

Solution.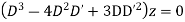

Its auxiliary equation is

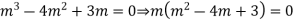
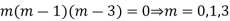
The required solution is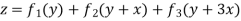

Example. Solve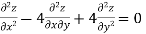

Solve.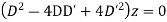

Its auxiliary equation is [D = m, D’ = 1]

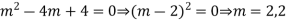
The required solution is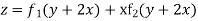

Rules for finding the particular integral

Given partial differential equation is

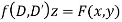
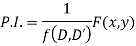
(i)                When F (x, y)=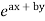

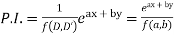  [Put D = a, D’ =b]

(ii)              When F (x, y)=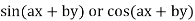

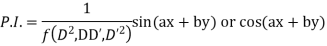
(iii)           When F (x, y)=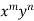

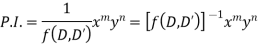
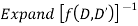in ascending power of D or D’ and operate on 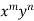term by term.

(iv)            When = Any function F (x, y)

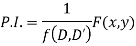
Resolve 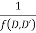into partial fractions

Considering f(D, D’) as a function of D alone

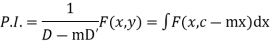
Where c is replaced by y + mx after integration

Case 1. When R.H.S =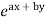

Example 1. Solve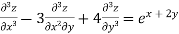

Solution.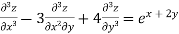

Given equation in symbolic form is

(D3-3D2 D'+4D'3)z=ex+2y

It’s A.E. Is 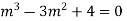where m = -1,2, 2

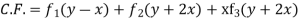
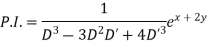
Put, D=1,D’=2

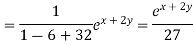
Hence the complete solution is

Case II. When R.H.S =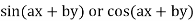

Example 2. Solve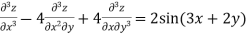

Solution.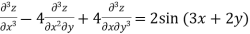

Putting

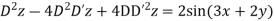
A.E. Is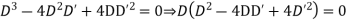

Put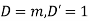

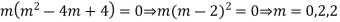
C.F. Is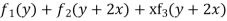

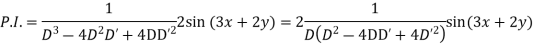
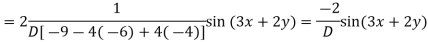
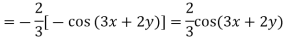
The general solution is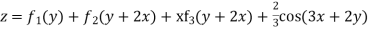

Example 3. Solve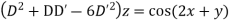

A.E. Is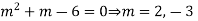

C.F. =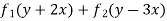

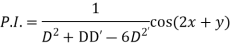
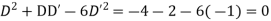
It is a case of failure.

Now,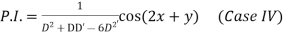

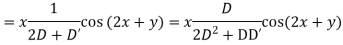
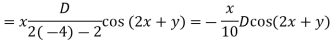
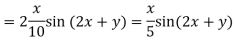
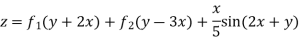
Case III. When R.H.S. =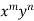

Example 4. Find the general integral of the equation

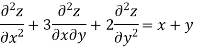
Solution.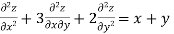

With the given equation can be written in the form

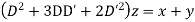
Writing D = m and D’=1the auxiliary equation is

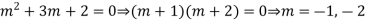
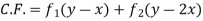
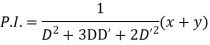
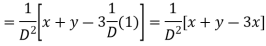
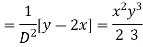
Hence the complete solution is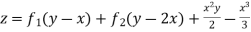

Case IV. When R.H.S. = Any Fraction

Example 5. Solve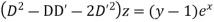

Solution.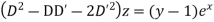

A.E. Is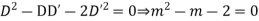

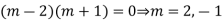
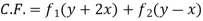
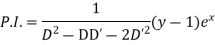
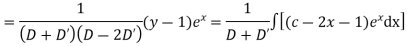
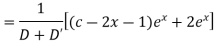
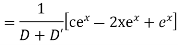
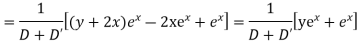
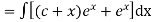
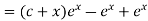
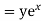
Hence the complete solution is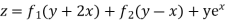

Non-Homogeneous Linear equations

The linear differential equations which are not homogeneous are called non-Homogeneous Linear equations.

For example

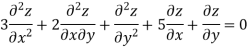
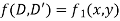
Its solution,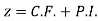

Complementary function: let the non-Homogeneous equation be

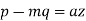
Lagrange’s subsidiary equations are

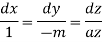
From the first two relations, we have, -mdx = dy

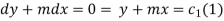
And some first and third relation,

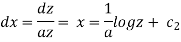
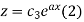
From (1) and (2) we have

Similarly, the solution of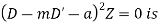

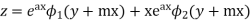
Example. Solve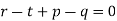

Solution. The equation is written as

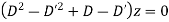
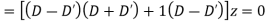
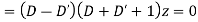
Hence the solution is

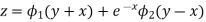
Particular integral

Case 1.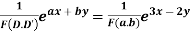

Example.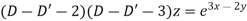

The complementary function is

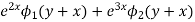
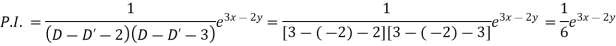
Hence the complete solution is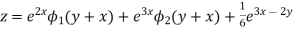

Case 2.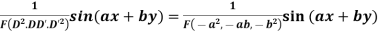

Example. Solve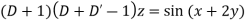

Solution.

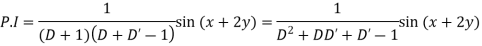
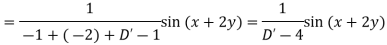
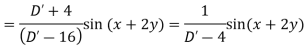
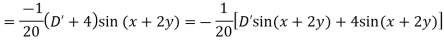
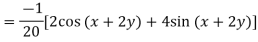
Hence the solution is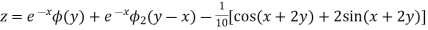

Case 3.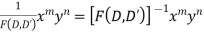

Example. Solve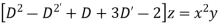

Solution.

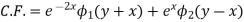
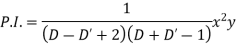
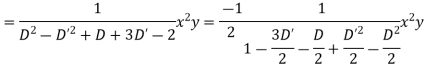
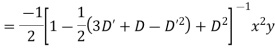
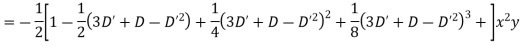
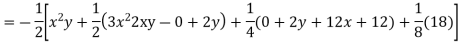
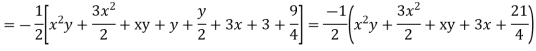
Hence the complete solution is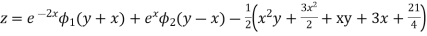

Case 4.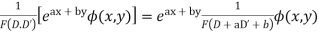

Example. Solve

Solution. A.E. Is

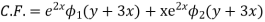
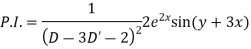
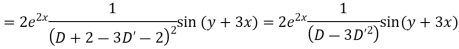
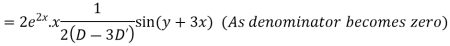
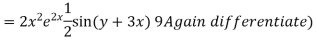
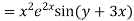
Hence the complete solution is

1.6 Equations reducible to linear partial differential equations with constant coefficients

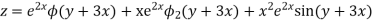
Let us consider the Euler-Cauchy PDE-

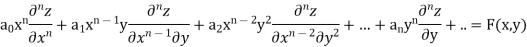
If we write-

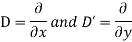
Then it becomes-

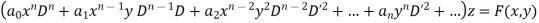
..............(1)

Let introduce the two new variables u and v-

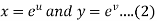
Which gives-

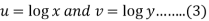
Also let's take-

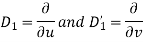
So that, by using (3)-

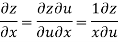
Or

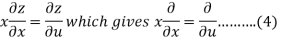
Again, we have-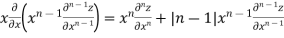

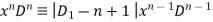...... (5)

Putting n=2,3.... In (5),

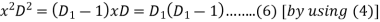
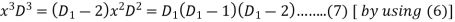
And so on.....

Similarly-

We have-

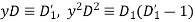
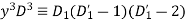  (8)

And so on.......

And similarly, we have-

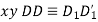     (9)

And

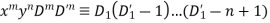........(10)

We use the substitution (2) and the results of 4,5,6,7,8,9,10,

The given equation (1) can be easily solved.

Example: Solve-

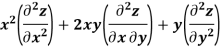
Sol.

The above PDE can be written as-

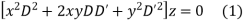
Now put

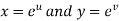
Which gives-

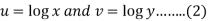
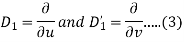
Then the equation (1) can be written as-

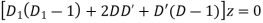
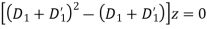
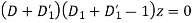
Hence the required solution will be-

References:

1. Erwin Kreyszig, Advanced Engineering Mathematics, 9thEdition, John Wiley & Sons, 2006.

2. N.P. Bali and Manish Goyal, A textbook of Engineering Mathematics, Laxmi Publications.

3. B.S. Grewal, Higher engineering mathematics, Khanna publishers