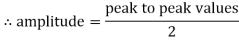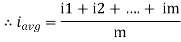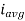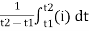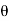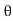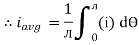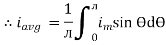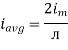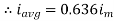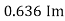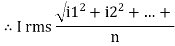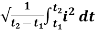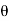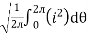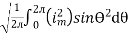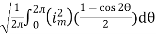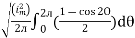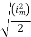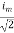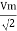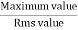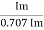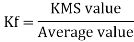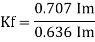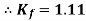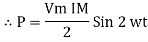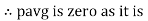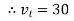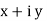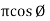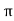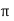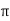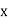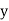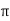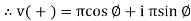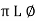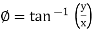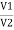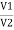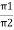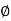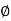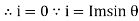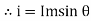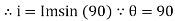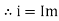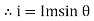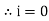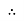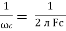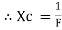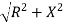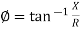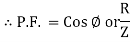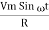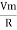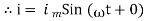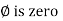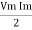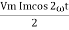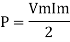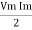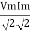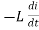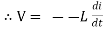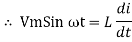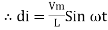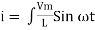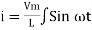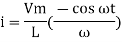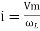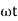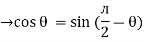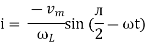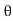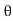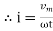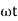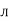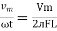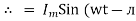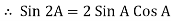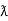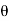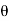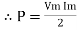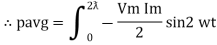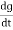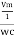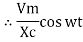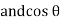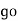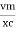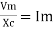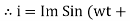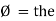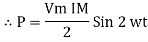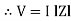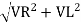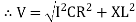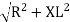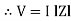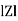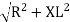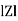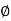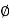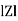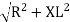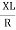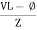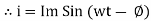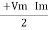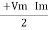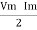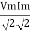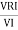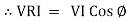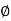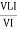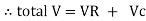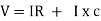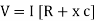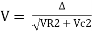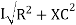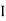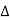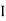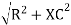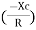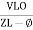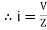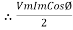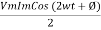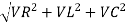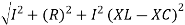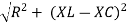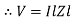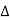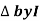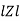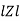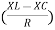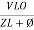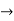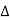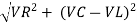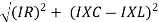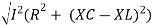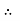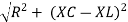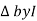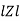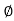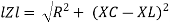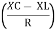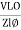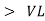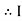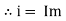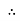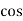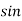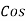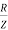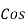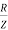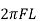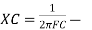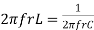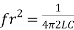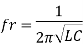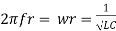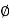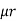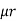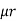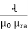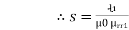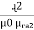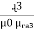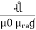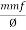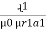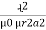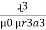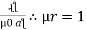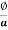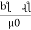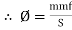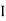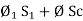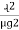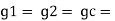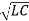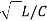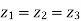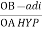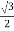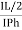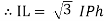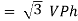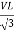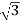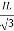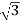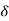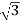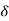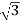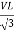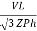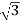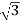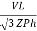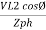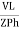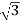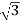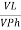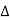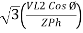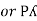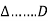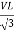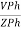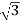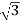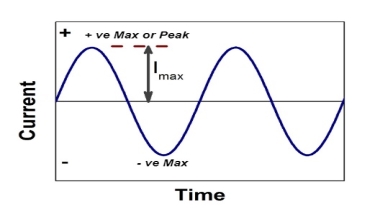
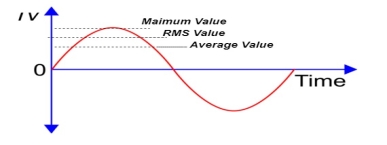
Peat to peak value:

The value of an alternating quantity from its positive peak to negative peak

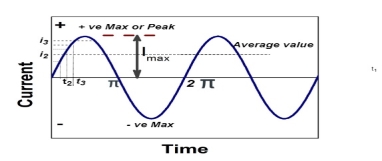
Average Value:

The arithmetic mean of all the value over complete one cycle is called as average value

=

For the derivation we are considering only hall cycle.

 Thus varies from 0 to ᴫ

i = Im   Sin

Solving

We get

Similarly, Vavg=

The average value of sinusoid ally varying alternating current is 0.636 times maximum value of alternating current.

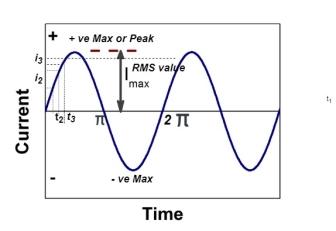
RMS value: Root mean square value

The RMS value of AC current is equal to the steady state DC current that required to produce the same amount of heat produced by ac current provided that resistance and time for which these currents flows are identical.

I rms =  

Direction for RMS value:

Instantaneous current equation is given by

i = Im Sin

but

I rms =  

                                                              =  

                                                              =

                                                              =

                                                              Solving

                                                              =

                                                               =

Similar we can derive

V rms= or 0.707 Vm

the RMS value of sinusoidally alternating current is 0.707 times the maximum value of alternating current.

Peak or krest factor (kp) (for numerical)

It is the ratio of maximum value to rms value of given alternating quantity

Kp =

Kp =

Kp = 1.414

Form factor (Kf): For numerical “It is the ratio of RMS value to average value of given alternating quality”.

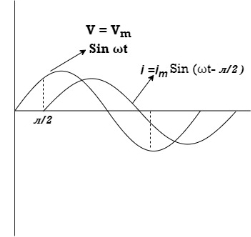
Power   P= Ѵ. i

= [Vm sinwt] [ Im sin (wt + X/2)]

= Vm Im Sin wt Sin (wt + X/2)]

(cos wt)

to charging power waveform [resultant].

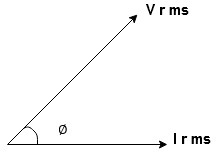
             The phases can be represented in different ways

1. Polar Form
2. Rectangular Form
3. Trigonometric Form (P - R)
4.  Exponential Form (R - P)

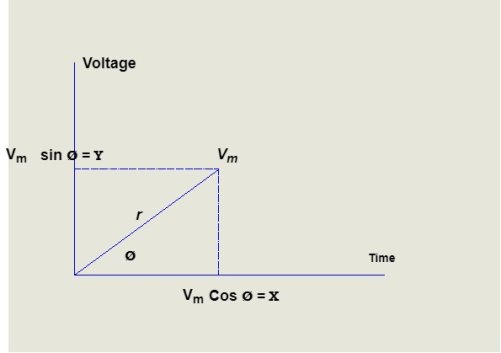
The instantaneous voltage equation

V(t) = V m sin (w t + Ø)

1. Polar Form

              The instantaneous voltage equation is given by  

Vt= vm sin (w t +Ø)

               which can be represented by polar form

vt = L Ø

              where = peak value

              e.g. vt =30 sin (w t + 90)

polar form < 90

              polar form is suitable for multiplication and division of phases.

2.     Rectangular Form:

              The instantaneous voltage equation is given by

               Vt = v m sin (w t +Ø)

               which can be represented by Rectangular Form 

               vt =

               where       X = or Vm cos  Ø

               Y =or Vm sin Ø

               Vt = v m cos Ø + i vm sin Ø

                e.g. 30 sin (w t + 90)

                Rectangular form vt = 30 cos 90 + i 30 sin 90

Rectangular Form is suitable for addition and subtraction of Phases.

3.     Trigonometric Form (Polar to Rectangular)

               If the phases are given in polar form from L Ø then it can be represented in     

               rectangular form by expressing X and Y component in form of  and Ø.

               Polar: Vt = L Ø

               Rectangular: vt= + i   where

𝑥 = cos Ø

𝑦 = sin Ø

4.     Exponential Form (R-P)

             Given equation Rect : v(+) =𝑥 + i𝑦

              Polar:  v(+) =

              Where magnetite 

              And

2 Phase added and substrate using Rect. Form

              Let V1 = 𝑥1 + i𝑦1 

V2 = 𝑥2 + i𝑦2

(V1 + V2) = (𝑥1 + i𝑦1) + (𝑥2 + i𝑦2)

            = (𝑥1 + 𝑥2) + i(𝑦1 + 𝑦2)  

              (V1 – V2) = (𝑥1 – 𝑥2) + i(𝑦1  - 𝑦2) 

For add or subst.  If eqtn. Is given in polar form, we have to connect into Rect. Form and then add/ subtract.               

Two phases divide/ multiply by polar

              Let V1 = π1 L Ø1

              Let V2 = π2 L Ø2

              (V1 V2)  = (π1 L Ø1) (π2 L Ø2)

(V1 X V2) = (π1 x π2) L (Ø1 x Ø2)

           For dividing 

=

=   L 1 - 2

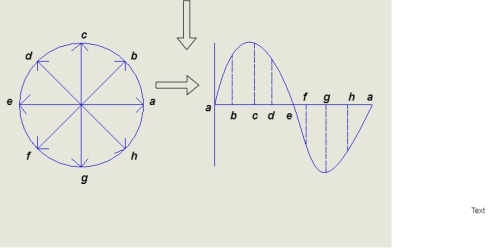
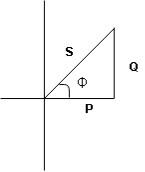
Phases Representation of an Alternating Quantity

1. The sinusoidal varying alternating quantity can be represented graphically by a straight line and arrow in the phase’s representation method.
2. The length of the line represents magnitude and arrow indicates it direction.
3. “The phases are assumed to be rotated in anticlockwise direction with constant Angular speed.”
4. One complete cycle of sine wave is represented by one complete rotation of phases as shown below

           Consider at various position

1. At point a the y axis projection is zero

θ=0   

2.     At point b the y axis projection 0b sin θ 

3.     At point c the y axis projection (0C) represent max value of phase

4.     Point D, y axis projection is (0ɖ)

5.     At point e, y axis projection is zero

Now at point “ȴ” on words the phases change its direction cycle also shifts from the half cycle to –v e   half cycle.

3 Basic element of AC circuit. 

1] Resistance

2] Inductance

3] Capacitance 

Each element produces opposition to the flow of AC supply in forward manner.

Reactance

1. Inductive Reactance (XL)

             It is opposition to the flow of an AC current offered by inductor.

XL = ω L    But     ω = 2 ᴫ F

XL = 2 ᴫ F L

                                  It is measured in ohm

XL∝FInductor blocks AC supply and passes dc supply zero

2.     Capacitive Reactance (Xc)

                It is opposition to the flow of ac current offered by capacitor

Xc =

                                   Measured in ohm

Capacitor offers infinite opposition to dc supply 

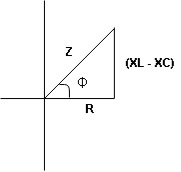
Impedance (Z)

The ac circuit is to always pure R pure L and pure C it well attains the combination of these elements. “The combination of R1 XL and XC is defined and called as impedance represented as 

                                           Z = R +i X

                                          Ø = 0

  only magnitude

R = Resistance, i = denoted complex variable, X =Reactance XL or Xc

Polar Form

Z   = L I

Where =

Measured in ohm

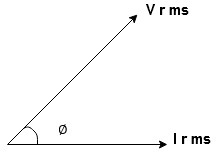
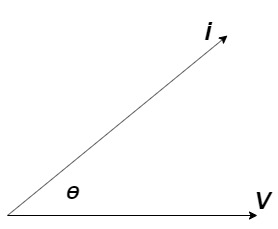
Power factor (P.F.)

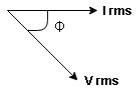
It is the cosine of angle between voltage and current

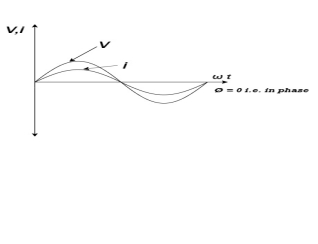
If Ɵis –ve or lagging (I lags V) then lagging P.F.

If Ɵ is +ve or leading (I leads V) then leading P.F.

If Ɵ is 0 or in phase (I and V in phase) then unity P.F.

Ac circuit containing pure resisting

Consider Circuit Consisting pure resistance connected across ac voltage source

V = Vm Sin ωt      ①

According to ohm’s law i = = 

But Im =

②

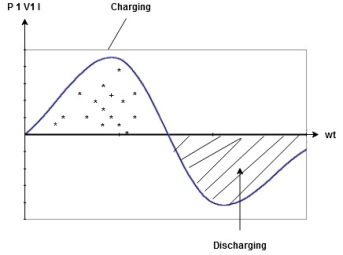
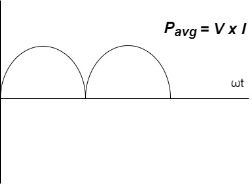
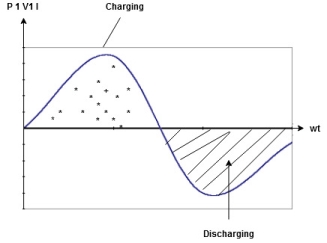
Phasor diagram  From ① and ②phase or represents RMD value.

Power      P = V. i

Equation P = Vm sin ω t       Im sin ω t

P = Vm Im Sin2 ω t

P =   -

Constant        fluctuating power if we integrate it becomes zero

Average power

Pavg =

Pavg =

Pavg = Vrms Irms

Power ware form [Resultant]

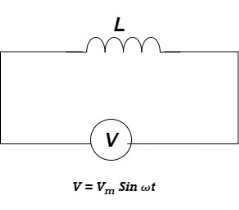
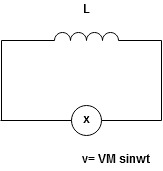
Ac circuit containing pure Inductors

Consider pure Inductor (L) is connected across alternating voltage. Source

V = Vm Sin ωt

When an alternating current flow through inductance it set ups alternating magnetic flux around the inductor.

This changing the flux links the coil and self-induced emf is produced

According to faradays Law of E M I

e =

at all instant applied voltage V is equal and opposite to self-induced emf [ lenz’s law]

V = -e

=

But V = Vm Sin ωt

dt

Taking integrating on both sides

dt

dt

(-cos )

but sin (– ) = sin (+ )

sin ( - /2)

And Im=

/2)

/2

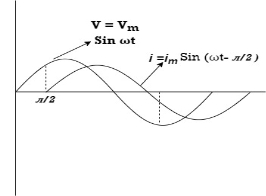
= -ve

= lagging

= I lag v by 900

Waveform:

Phasor:

Power P = Ѵ. I

= Vm sin wt    Im sin (wt /2)

= Vm Im Sin wt Sin (wt – /s)

①

And

Sin (wt - /s) =  - cos wt         ②

Sin (wt – ) = - cos

sin 2 wt    from ① and ②

The average value of sin curve over a complete cycle is always zero

Pavg = 0

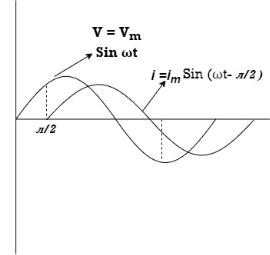
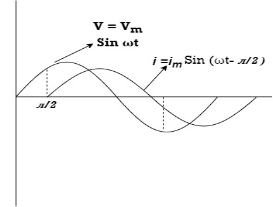
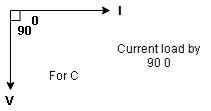
Ac circuit containing pure capacitors:

Consider pure capacitor C is connected across alternating voltage source

Ѵ = Ѵm Sin wt

Current is passing through capacitor the instantaneous charge ɡ produced on the plate of capacitor

ɡ = C  Ѵ

ɡ = c Vm sin wt

the current is rate of flow of charge

i=  (cvm sin wt)

i = c Vm w cos wt

then rearranging the above eqth.

i =     cos wt

= sin (wt + X/2)

i = sin (wt + X/2)

but

X/2)

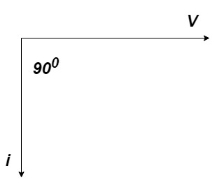
= leading

= I leads V by 900

Waveform :

Phase

Power   P= Ѵ. i

= [Vm sinwt] [ Im sin (wt + X/2)]

= Vm Im Sin wt Sin (wt + X/2)]

(cos wt)

to charging power waveform [resultant].

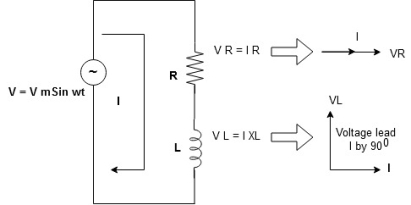
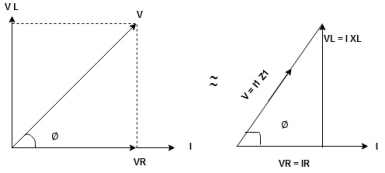
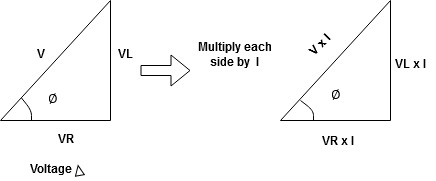
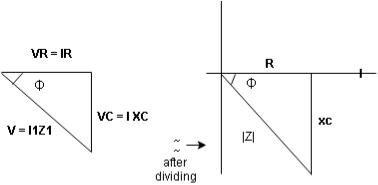
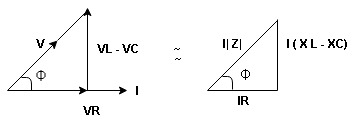
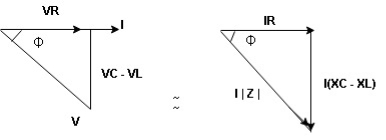
Series R-L Circuit

Consider a series R-L circuit connected across voltage source V= Vm sin wt

As some I is the current flowing through the resistor and inductor due do this current voltage drops arcos R and L      R VR = IR and  L VL = I X L

Total  V = VR + VL

V = IR + I X L  V = I [R + X L]

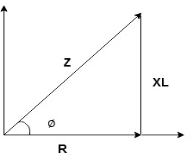
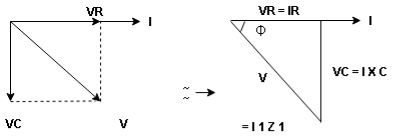
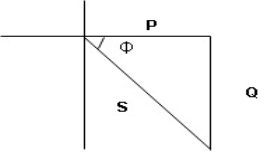
Take current as the reference phasor  : 1) for resistor current is in phase with voltage 2) for Inductor voltage leads current or current lags voltage by 90 0.

For voltage triangle

Ø is power factor angle between current and resultant voltage V and

V =

V =

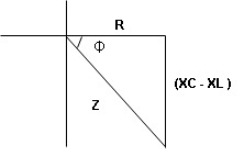
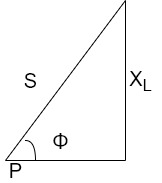
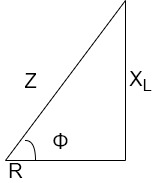
where Z = Impedance of circuit and its value is =

Impedance Triangle

       Divide voltage triangle by I

Rectangular form of Z = R+ixL

and polar from of Z =     L +

(+ j X L  + because it is in first quadrant )

Where     =

+ Tan -1

Current Equation :

From the voltage triangle we can sec. that voltage is leading current by or current is legging resultant voltage by

Or i = =       [ current angles  - Ø )

Resultant Phasor Diagram from Voltage and current eqth.

Wave form

Power equation

P = V .I.

P = Vm Sin wt    Im Sin wt – Ø

P = Vm Im (Sin wt)  Sin (wt – Ø)

P = (Cos Ø) -  Cos (2wt – Ø)

 Since 2 sin A Sin B = Cos (A-B) – Cos (A+B)

P = Cos Ø      -       Cos (2wt – Ø)

①②

Average Power

pang = Cos Ø

Since ② term become zero because Integration of cosine come from 0 to 2ƛ

pang = Vrms Irms cos Ø   watts.

Power Triangle : 

From  

VI  = VRI  + VLI       B

Now cos Ø in A  =

①

Similarly Sin =

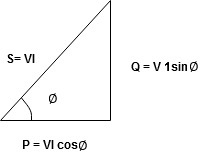
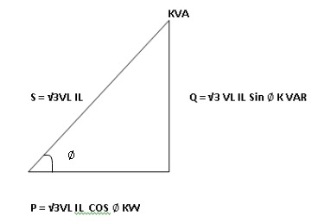
Apparent Power     Average or true          Reactive or useless power

                                   Or real or active

-Unit (VI)                   Unit (Watts)                C/W (VAR) denoted by (Ø)

Denoted by [S]        denoted by [P]

Power for R L ekt.

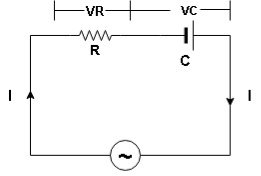
Series R-C circuit

                           V = Vm sin wt

 VR

 I

• Consider a series R – C circuit in which resistor R is connected in series with capacitor C across a ac voltage so use  V = VM Sin wt  (voltage equation).
• Assume Current  I is flowing through

      R and C voltage drops across.

R and C  R VR = IR

And C Vc = Ic

V = lZl

Voltage triangle : take current as the reference phasor 1) for resistor current is in phase with voltage  2) for capacitor current leads voltage or voltage lags behind current by 900

Where Ø is power factor angle between current and voltage (resultant) V

And from voltage

V =

V =

V =

              V = lZl

Where Z = impedance of circuit and its value is lZl =

Impendence triangle :

Divide voltage by as shown

Rectangular from of Z = R - jXc

Polar from of Z = lZl L -  Ø

( - Ø and –jXc because it is in fourth quadrant ) where

lZl =

and Ø = tan -1

Current equation :

from voltage triangle we can see that voltage is lagging current by Ø or current is leading voltage by Ø

i = IM Sin (wt + Ø) since Ø is +ve

Or  i = for RC

  LØ  [ resultant current angle is + Ø]

Resultant phasor diagram from voltage and current equation

Resultant wave form :

Power  Equation :

P = V. I

P = Vm sin wt.   Im  Sin (wt + Ø)

= Vm Im sin wt sin (wt + Ø)

2 Sin A Sin B = Cos (A-B) – Cos (A+B)

  -

Average power

pang =     Cos Ø

since 2 terms integration of cosine wave from 0 to 2ƛ become zero

2 terms become zero

pang  = Vrms  Irms Cos Ø

Power triangle RC Circuit:

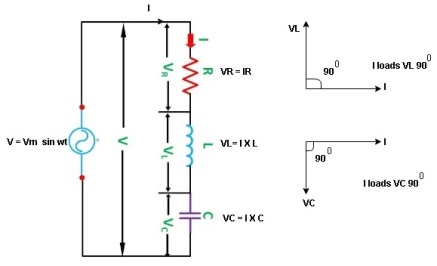
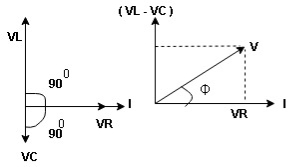
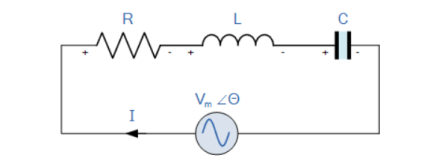
R-L-C series circuit 

Consider ac voltage source V = Vm sin wt connected across combination of R L and C. when I flowing in the circuit voltage drops across each component as shown below.

VR = IR, VL = I L, VC = I C

• According to the values of Inductive and Capacitive Reactance I e XL and XC decides the behaviour of R-L-C series circuit according to following conditions

① XL> XC, ② XC> XL, ③ XL = XC

① XL > XC: Since we have assumed XL> XC

Voltage drop across XL> than XC

VL> VC         A

• Voltage triangle considering condition   A

VL and VC are 180 0 out of phase .

Therefore cancel out each other

Resultant voltage triangle

Now  V = VR + VL + VC c phasor sum and VL and VC are directly in phase opposition and VLVC their resultant is  (VL - VC).

From voltage triangle

V =

V =

V = I

Impendence   : divide voltage

Rectangular form Z = R + j (XL – XC)

Polor form Z = l + Ø       B

Where =

And Ø = tan-1

• Voltage equation : V = Vm Sin wt
• Current equation

i =    from B

i = L-Ø           C

as  VLVC  the circuit is mostly inductive and I lags behind V by angle Ø

Since i = L-Ø

i = Im Sin  (wt – Ø)    from c

• XC XL :Since we have assured XC XL

the voltage drops across XC   than XL

XC XL         (A)

voltage triangle considering condition   (A)

  Resultant Voltage

Now  V = VR + VL + VC   phases sum and VL and VC are directly in phase opposition and VC  VL   their resultant is (VC – VL)

From voltage

V =

V =

V =

V =

Impedance  : Divide voltage

• Rectangular form : Z + R – j (XC – XL) – 4th  qurd

Polar form : Z =    L -

Where

And Ø = tan-1 –

• Voltage equation : V = Vm Sin wt
• Current equation : i =     from B
• i = L+Ø      C

as VC     the circuit is mostly capacitive and leads voltage by angle Ø

since i =   L +  Ø

Sin (wt – Ø)   from C

• Power :

• XL= XC  (resonance condition):

ɡȴ  XL= XC   then VL= VC  and they are 1800 out of phase with each other they will cancel out each other and their resultant will have zero value.

Hence resultant V = VR and it will be in phase with  I as shown in below phasor diagram.

From above resultant phasor diagram

V =VR + IR

Or V = I lZl

Because lZl + R

Thus Impedance Z is purely resistive for XL = XC and circuit current will be in phase with source voltage.

Since  VR=V    Øis zero when  XL = XC power is unity

ie pang = Vrms  I rms  cos Ø = 1   cos o = 1

maximum power will be transferred by condition.  XL = XC

1. Apparent power: (S):- it is defined as product of rms value of voltage (v) and current (I), or it is the total power/maximum power

  S= V × I

Unit - Volte- Ampere (VA)

In kilo – KVA

2.     Real power/ True power/Active power/Useful power: (P) it is defined as the product of rms value of voltage and current and the active component or it is the average or actual power consumed by the resistive path (R) in the given combinational circuit.

It is measured in watts

    P = VI  Φ watts / KW, where Φ is the power factor angle.

3.     Reactive power/Imaginary/useless power [Q]

It is defined as the product of voltage, current and sine B and I

Therefore,

           Q= V.I Φ

Unit –VA R

In kilo- KVAR

As we know power factor is cosine of angle between voltage and current

  i.e.  Φ.F= CosΦ

In other words, also we can derive it from impedance triangle

Now consider Impedance triangle in R.L.ckt

From triangle ,

 Now  Φ – power factor=

 Power factor = Φ or

Resonance with Definition, condition and derivation

Resonance in series RLC circuit

Definition:

It is defined as the phenomenon which takes place in the series or parallel R-L-C circuit which leads to unity power factor

Voltage and current in R-L-C ckt are in phase with each other.

Resonance is used in many communication circuits such as radio receiver.

Resonance in series RLC -> series resonance in parallel->antiresonance/parallel resonance

Condition for resonance

XL=XC

Resonant frequency (fr): For given values R-L-C the inductive reactance XL becomes exactly equal to the capacitive reactance XC only at one particular frequency. This frequency is called as resonant frequency and denoted by ( fr)

Expression for resonant frequency (fr)

We know that

 XL = - inductive reactance

 capacitive reactance

At a particle or frequency f=fr,the inductive and capacitive reactance are exactly equal

Therefore, XL = XC ----at f=fr

i.e.

  Therefore,

and rad/sec

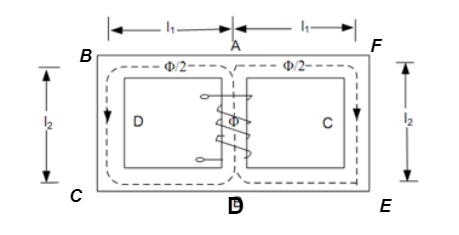
Derivation of series magnetic current with air gap         

In series magnetic circuit flux is same in each part of circuit

Consider a composite magnetic circuit made from different material of lengths ɻ1,ɻ2, and ɻ3 cross sectional area a1, a2 And a3 and relative permeability’s  1 , 2 , 3  resp. with an airgap of length ɻɡ

Total reluctance S = S1 + S2+ S3 + Sg

               We know that S =

+ + +

But S = and mmf = S x Ø

mmf = Ø + + + for air

Now  B = 

mmȴ = + + +

but B=μH

H =   = 

Total mmȴ = H1  ɻ1+ H2  ɻ2 + H3  ɻ3 + Hƪɻƪ

A magnetic circuit which has more than one path for flux called parallel magnetic circuit

Mean length ABCDA = ɻ₁, and ADEFA = ɻ₂

Mean length path for central limb = ɻc

Reluctance ABCDA = S1, ADEFA = S2

And central limb = SC

Now total mmȴ = N

i.e. mmf =

For path ABC AD

N =

Where S1 = , S2 = , and Sc =

Where area of cross selection

for parallel ekt.

Total mmȴ = mmȴ by central limb + mmȴ required by any one of outer limb

       = Ø sc + [Ø1S1  or Ø2 S2]

As mmf across parallel branch is same

  Ø1 S1 = Ø2 S2

Hence while calculating total mmf, the mmf of only one of 2 parallel branches must be considered.

Consider a series RLC circuit

The Inductive reactance XL = 2 π f L = wL

Capacitive reactance: Xc = ½ π f C = 1/wC

When XL > Xc the circuit is inductive

When XC > XL the circuit is Capacitive.

Total circuit reactance = XT = XL -Xc or XC – XL

Total circuit impedance = Z = [R 2 + XT 2]  = R + j X

In a series resonant circuit, the resonant frequency, ƒr point can be calculated as follows.

XL = Xc  --- 2 π f L = 1/ 2 π f C

f 2 = 1/ 2 π L x 2 π C = 1/ 4 π 2 L C

f = [1/ 4 π 2 L C ] ½

fr = 1/ 2 π

In the circuit high quality factor is required to ensure low energy dissipation and low oscillation damping but high-quality factor can only be achieved by bandwidth. Therefore, it is required to maintain balance between these two

Q = fr/BW

Fr = 1/ 2 π

Q = 1/R

BW = R/L

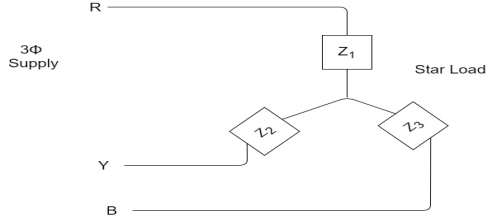
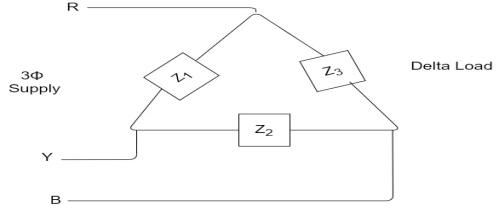
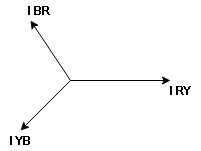
3Φ system in which three voltages are of identical magnitudes and frequency and are displaced by 120° from each other called as symmetrical system.

Phase sequence:

The sequence in which the three phases reach their maximum positive values. Sequence is R-Y-B. Three colours used to denote three faces are red ,yellow and blue.

The direction of rotation of 3Φ machines depends on phase sequence. If a sequence is changed i.e. R-B-Y then the direction of rotation will be reversed.

Types of loads

1. Star connection of load
2. Delta connection of load

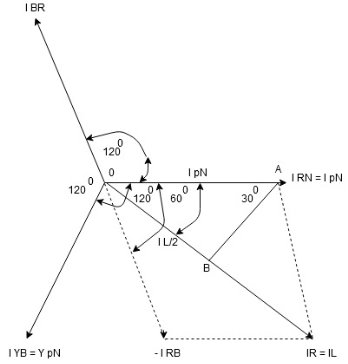
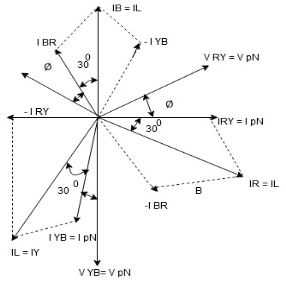
Balanced load:

Balanced load is that in which magnitudes of all impedances connected in the load are are equal and the phase angles of them are also equal.

i.e.

 If.  ≠  ≠ then it is unbalanced load

Phasor Diagram

Consider equation ①

Note: we are getting resultant line current IR      by subtracting 2 phase currents IRY and IBR   take phase currents at reference as shown

Cos 300  =  

=

• Complete phases diagram for delta connected balanced Inductive load.

Phase current IYB  lags behind VYB  which is phase voltage as the load is inductive

Drive

• relation between line value and phase value of voltage and current for a balance () delta connected inductive load

consider a 3 Ø balance delta connected inductive load

• line values

Line voltage = VRY = VYB = VBR = VL

Line current = IR = IY = IB = IL

Phase value

Phase voltage = VRN = VYN = VBN = Vph

Phase current = VRN = VYN = VBN = Vph

• since for a balance delta connected load the voltage measured in line and phase is same because their measuring points are same

for balance delta connected load VL =  Vph

VRV = VYB = VBR = VR = VY = VB = VL = VPh

• since the line current differ from phase current, we can relate the line and phase values of current as follows
• apply KCL at node R

IR + IRY= IRY

IR     =   IRY - IRY … …. ①

  Line    phase

Similarly apply  KCL at node  Y

IY  +  IYB  =  IRY … …. ②

Apply KCL at node  B

IB  +  IBR  =  IYB … ….③

PPh  = VPh IPh  Cos Ø

For 3 Ø  total power is

PT= 3 VPh  IPh  Cos Ø …….①

For star

VL and IL = IPh   (replace in ①)

PT  = 3    IL  Cos Ø

PT  = 3   VL IL  Cos Ø – watts

For delta

VL  = VPh  and IL =      (replace in ①)

PT = 3VL    Cos Ø

PT VL IL  Cos Ø – watts

total average power

P = VL IL  Cos Ø – for ʎ and load

                K (watts)

Total reactive power

Q = VL IL  Sin Ø – for star delta load

                  K (VAR)

Total Apparent power

S = VL IL   – for star delta load

           K (VA)

• Power triangle

• Relation between power

In star and power in delta

Consider a star connected balance load with per phase impedance ZPh

we know that for

VL = VPh    andVL = VPh 

now  IPh  =

VL = =

And VPh  =

IL  =      ……①

Pʎ = VL IL Cos Ø ……②

Replacing ① in ② value of IL

Pʎ = VL IL   Cos Ø

Pʎ =     ….A

• Now for delta

IPh  =

IPh = =

And IL  = IPh

IL  = X    …..①

P = VL IL Cos Ø ……②

Replacing ② in ① value of IL

P = Cos Ø

P =   …..B

                            Pʎ from …A

    …..C

    =   P

We can conclude that power in delta is 3 time power in star from …C

Or

Power in star is time power in delta from ….D

• Step to solve numerical

1. Calculate VPh from the given value of VL by relation

For star VPh =

For delta VPh = VL

2.     Calculate IPh  using formula

IPh =

3.     Calculate IL using relation

IL = IPh  - for star

IL  = IPh  - for delta

4.     Calculate P by formula (active power)

P = VL IL Cos Ø – watts

5.     Calculate Q by formula (reactive power)

Q = VL IL Sin Ø – VAR

6.     Calculate S by formula (Apparent power)

S = VL IL– VA