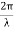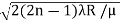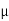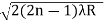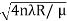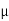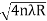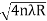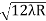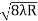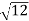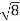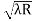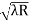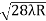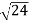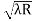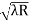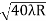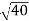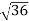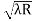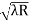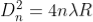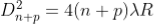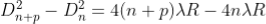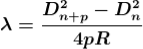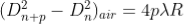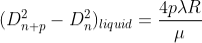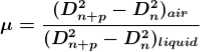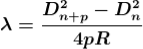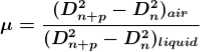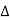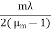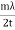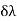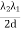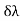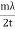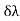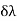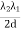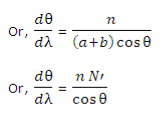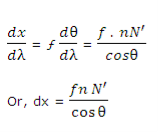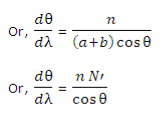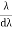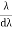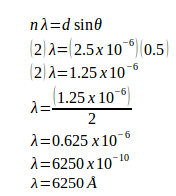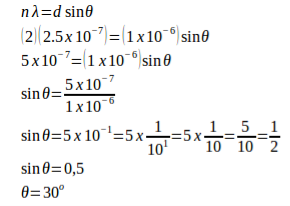UNIT 2
OPTICS
Interference in light waves occurs whenever two or more waves overlap at a given point.
TYPES OF INTERFERENCE
The interference of light waves can be either constructive interference or destructive interference.
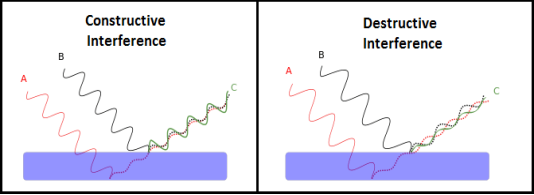
- Constructive Interference: Constructive interference takes place when the crest of one wave falls on the crest of another wave such that the amplitude is maximum. These waves will have the same displacement and are in the same phase.
- Destructive Interference: In destructive interference, the crest of one wave falls on the trough of another wave such that the amplitude is minimum. The displacement and phase of these waves are not the same.
|
Figure 1: Constructive interference & destructive interference
Light waves also interfere with each other. Fundamentally, all interference associated with light waves arises when the electromagnetic fields that constitute the individual waves combine.
If two light bulbs are placed side by side, no interference effects are observed because the light waves from one bulb are emitted independently of those from the other bulb. The emissions from the two light bulbs do not maintain a constant phase relationship with each other over time. Light waves from an ordinary source such as a light bulb undergo random phase changes in time intervals less than a nanosecond.
COHERENT SOURCES
Interference from such waves happen all the time, the randomly phased light waves constantly produce bright and dark fringes at every point. But, we cannot see them since they occur randomly. A point that has a dark fringe at one moment may have a bright fringe at the next moment. This cancels out the effect of the interference effect, and we see only an average brightness value. The interference is not said to be sustained since we cannot observe it.
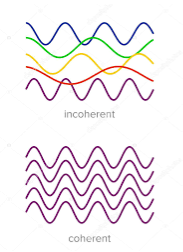
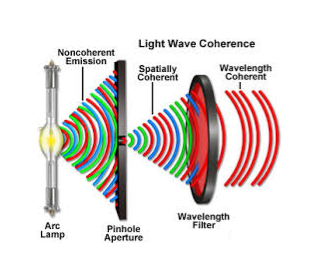
Definition:- A predictable correlation of the amplitude and phase at any one point with another point is called coherence.
|
Figure 2: Incoherence & coherence
Two waves are said to be coherent, the waves must have
In the case of conventional light, the property of coherence exhibits between a source and its virtual source whereas in the case of laser the property coherence exists between any two or more light waves.
There are two types of coherence
i) Temporal coherence
ii) Spatial coherence
TEMPORAL COHERENCE (OR LONGITUDINAL COHERENCE):-
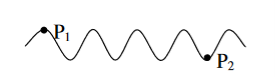
The predictable correlation of amplitude and phase at one point on the wave train w .r. t another point on the same wave train, then the wave is said to be temporal coherence
To understand this, let us consider two points P1 and P2 on the same wave train, which is continuous as shown in the figure.
Figure 3: Wave train
Suppose the phase and amplitude at any one point is known, then we can easily calculate the amplitude and phase for any other point on the same wave train by using the wave equation y= a sin ( Where ‘a’ is the amplitude of the wave and ‘x’ is the displacement of the wave at any instant of time ‘t’. |
SPATIAL COHERENCE (OR TRANSVERSE COHERENCE)
The predictable correlation of amplitude and phase at one point on the wave train w. r .t another point on a second wave, then the waves are said to be spatial coherence (or transverse coherence)
|
Figure 4: Spatial Coherence
CONDITIONS OF INTERFERENCE
- The sources must be coherent, that is, they must maintain a constant phase with respect to each other.
- The two light sources must emit continuous waves of the same wavelength and have the same period.
- The distance between the two sources of light must be small. This gives large fringe width so that the fringes are separately visible.
- The sources should be monochromatic, that is, of a single wavelength.
- The two light sources must emit waves in nearly the same direction.
- The light source must be a point source.
- The distance between the two sources and the screen must be large. This gives again large fringe width so that the fringes are separately visible.
Key Takeaways
- Interference in light waves occurs whenever two or more waves overlap at a given point.
- The interference of light waves can be either constructive interference or destructive interference.
- To obtain sustained interference; the sources must be coherent.
- There are two types of coherence: Temporal coherence & Spatial coherence
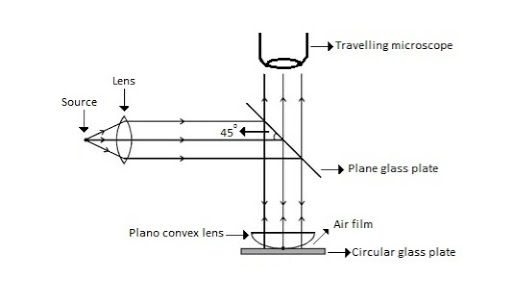
The fringes obtained by interference of light waves by using the following arrangement
When a Plano-convex lens with a large radius of curvature is placed on a plane glass plate such that its curved surface faces the glass plate, a wedge air film (of gradually increasing thickness) is formed between the lens and the glass plate. The thickness of the air film is zero at the point of contact and gradually increases away from the point of contact.
|
Figure 5: Newton Ring Assembly
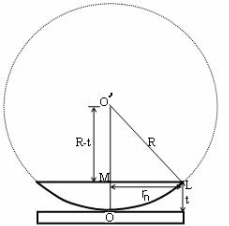
MATHEMATICAL ANALYSIS OF NEWTON’S RING
Figure 6: Mathematical analysis of newton’s ring
(OL)2 =(O’M)2-(ML)2 ……….(1) R2=(R-t)2 +rn2 R2=R2 +t2-2Rt +rn2 Radius is large as compared to the thickness so t2 is neglected as t2<< R2 R2=R2 +-2Rt +rn2 2Rt =rn2 The thickness of the film t =rn2 /2R ……….(2)
THEORY OF FRINGES: ∆ = 2μtcos(r+θ) +λ/2 ……….(3) If the light is incident normally on the lens, r = 0 and near to point of contact θ is small; Therefore near the point of contact, (r+θ) approaches to 0 and cos(r+θ)=cos0=1 Therefore ∆ = 2μt+λ/2 ……….(4)
Also At the point of contact t = 0 therefore the effective path difference ∆ = λ/2 Which is an odd multiple of λ/2 Therefore the Central fringe is dark.
BRIGHT FRINGE: CONDITION OF MAXIMA For the condition of maxima the effective path difference ∆ = ±nλ Using equation (4) ∆ = 2μt+λ/2 we have 2μt+λ/2= ±nλ 2μt = ± (2n-1)λ /2 ……….(5)
DIAMETER OF BRIGHT RINGS we know by equation (2) t =rn2 /2R substitute in equation (5) we have 2μ (rn2 /2R) = ± (2n-1)λ /2 rn2 = ± (2n-1)λR /2μ We know diameter D=2r and for nth fringe Dn=2rn so we have Dn2=± 2(2n-1)λR /μ Dn= The medium enclosed between the lens and glass plate is if air, therefore, D= The diameter of the bright ring is proportional to the square root of odd natural numbers
DARK FRINGE: CONDITION FOR MINIMA For the condition of minima, The effective path difference ∆ =± (2n+1)λ /2 2μt+λ/2 =± (2n+1)λ /2 2μt= ±nλ ……….(7) it is clear that for particular dark or bright fringe t should be constant. Every fringe is the locus of points having equal thickness. Hence the fringes are circular.
DIAMETER OF DARK RINGS we know by equation (2) t =rn2 /2R substitute in equation (7) we have 2μ (rn2 /2R ) = nλ rn2 = nλR/ μ We know diameter D=2r and for nth fringe Dn=2rn so we have Dn2= 4nλR/ μ Dn= The medium enclosed between the lens and glass plate is if air, therefore, Dn= The diameter of the dark ring is proportional to the square root of natural numbers.
SPACING BETWEEN FRINGES For example, the diameter of the dark ring is given by Dn= D3 - D2 = D7 – D6 = D10– D9 = From the above result, we conclude that the fringe width reduces with an increase in n. |
Key Takeaways
- Newton’s Rings consists of concentric bright and dark rings with the point of contact of the lens and the glass plate as centre.
- The diameter of the bright ring is proportional to the square root of odd natural numbers D=
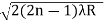 n=0,1,2,3,4
n=0,1,2,3,4 - The diameter of the dark ring is proportional to the square root of natural numbers Dn=
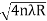 n=0,1,2,3,4
n=0,1,2,3,4 - The Newton’s rings are not equally spaced because the diameter of the ring does not increase in the same proportion as the order of ring and rings get closer and closer as ‘n’ increases.
The diameter of the nth order ring is calculated by subtracting the left and right side positions of the microscope. As we know that the square of the diameter of the nth dark ring is
Therefore the square of the diameter of (n+p)th ring is
Subtracting both the above equation
Therefore 2. Determination of Refractive Index of liquid To determine the refractive index of liquid Newton’s ring experiment is first performed for the air medium and the difference in the square of the diameter of (n+p)th and the nth dark ring is found as discussed above. After this few drops of liquid of μ refractive index is placed on the glass plate. The plano-convex lens is then placed on the glass plate, as a result, a film of liquid is formed between the lens and the plate. The difference in the square of the diameter of (n+p)th and the nth dark ring is again calculated in the same manner for the liquid medium. Dividing equation 2.29 by 2.30, we get
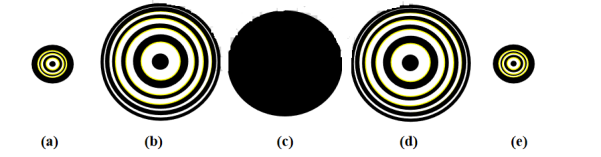
3. Newton’s ring with white light If the monochromatic source is replaced by the white light superposition of rings take place due to different wavelength. Few coloured rings are seen around the dark centre later illumination is seen in the field of view. As shown in the below figure.
Figure 7: Newton’s ring with white light
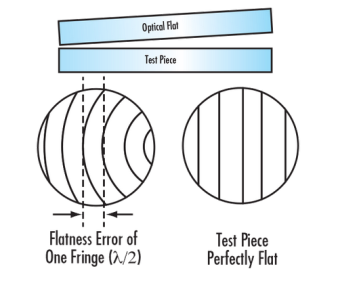
4. Testing Of Optical Flatness When an optical flat's polished surface is placed in contact with a surface to be tested, dark and light bands will be formed when viewed with monochromatic light. These bands are known as interference fringes and their shape gives a visual representation of the flatness of the surface being tested. The surface flatness is indicated by the amount of curve and spacing between the interference fringes. Straight, parallel, and evenly spaced interference fringes indicate that the work surface flatness is equal to or higher than that of the reference surface. An optical flat utilizes the property of interference to exhibit the flatness on the desired surface. When an optical flat, also known as a test plate, and a work surface are placed in contact, an air wedge is formed. Areas between the flat and the work surface that are not in contact form this air wedge. The change in thickness of the air wedge will dictate the shape and orientation of the interference bands. The amount of curvature that is shown by the interference bands can be used to determine the flatness of the surface. If the air wedge is too large, then many closely spaced lines can appear, making it difficult to analyze the pattern formed. Simply applying pressure to the top of the optical flat alleviates the problem. The determination of the flatness of any particular region of a surface is done by making two parallel imaginary lines; one between the ends of anyone fringe, and the other at the top of that same fringe. The number of fringes located between the lines can be used to determine the flatness. Monochromatic light is used to create sharp contrast for viewing and to specify the flatness as a function of a single wavelength.
Figure 8: Testing Of Optical Flatness
Measurement of the surface flatness of polished surfaces can be determined visually by comparing the variations between a work surface and the surface of an optical flat. Optical flats are versatile optical components used in many applications, such as inspection of gauge blocks for wear and accuracy, as well as the testing of various components including windows, prisms, filters, mirrors, etc. They can also be used as extremely flat optical windows for demanding interferometry requirements. Example: In Newton’s rings experiment the diameter of the 15th ring was found to be 0.59 cm and that of the 5th ring is 0.336 cm. If the radius of curvature of the lens is 100 cm, find the wavelength of the light. Solution: The given data are Diameter of Newton’s 15th ring (D15) = 0.59 cm = 0.59×10–2 m Diameter of Newton’s 5th ring (D5) = 0.336 cm = 0.336 × 10–2 m Radius of curvature of lens (R) = 100 cm = 1 m The wavelength of light (λ) =? Here m is difference between rings = 15-5=10 λ = D2n+m - D2n / 4mR λ = (0.59×10–2 )2- (0.336 × 10–2)2 / 4 x 10 x 1 λ = 0.3481 x10-4 – 0.112896 x10-4 / 40 λ =0.00588 x 10-4 m λ =5880 x 10-10 m λ =5880Å |
Key Takeaways
2. Determination of Refractive Index of liquid
s 3. If the monochromatic source is replaced by the white light, dark and bright fringes are not produced. 4. An optical flat utilizes the property of interference to exhibit the flatness on the desired surface. |
INTERFEROMETERS
Interferometry is a word used to study and exploit interference of light in optical devices in which two or more beams originate from the same source and after passing through different paths, they interfere with each other. The interference pattern can be analyzed to get information about the objects or phenomenon being studied. This idea involves the principle of division of amplitude.
An interferometer is designed to make the use of two-beam interference (Michelson Interferometer) and multiple-beam interference (Fabry-Perot Interferometer) of light.
There are two types of interferometers:
(1) Wavefront division interferometers in which the different portions of the same wavefront of a coherent beam of light is used to produce interference (Young's double slit, Lloyd's mirror, Fresnel Biprism, etc.) and
(2) Amplitude division interferometers in which a beam splitter is used to divide the initial coherent beam into two parts.
Basic Principle of Interferometers
When a beam of light is incident on a beam splitter or half-transparent mirror (a glass plate partially coated with sliver acts as a partially reflective surface), the incident light beam splits into two beams; one is directed towards a fixed mirror and the other is transmitted towards another movable mirror. The two beams reflected from these mirrors are subsequently recombined and produce interference pattern due to phase or path difference between them. An optical device that works on the above said principle is called an interferometer.
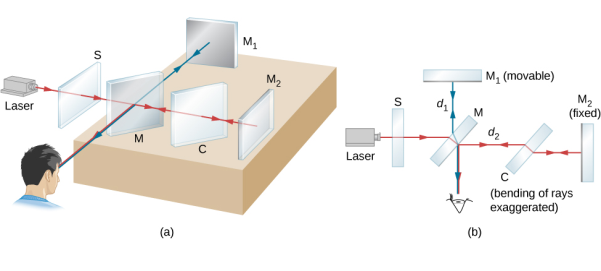
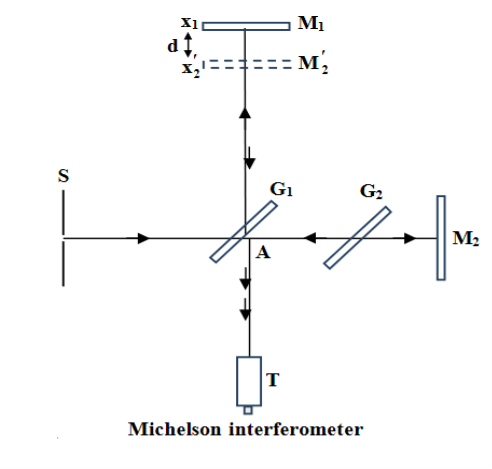
The Michelson Interferometer
|
Figure 9: Michelson Interferometer Experiment
Principle
Construction:
|
Figure 10: Michelson interferometer
Working
Thus, the telescope receives two reflected beams which are originally derived from the same source so that these are mutually coherent. Hence, the two beams M1A T and M2AT will interfere and produces interference fringes in the field of view of the telescope. This process is known as interference by the division of amplitude.
When one looks into the beam splitter from the telescope, there appears both the mirror M1 and a virtual image M2’ of M2 formed in G1 which are separated by a distance d = x1 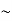 x2. Thus, the two interfering beams reflected by the mirrors M1 and M2 appears as reflected from M1 and M2. Hence, the Michelson interferometer is equivalent to a thin air film enclosed between M1 and M2’, see Figure 10.
x2. Thus, the two interfering beams reflected by the mirrors M1 and M2 appears as reflected from M1 and M2. Hence, the Michelson interferometer is equivalent to a thin air film enclosed between M1 and M2’, see Figure 10.
The path difference between the reflected beams depends on the separation between M1and M2’ and the inclination of M1 and M2’ respectively. Therefore. the interference fringes may be straight, circular, and parabolic is formed.
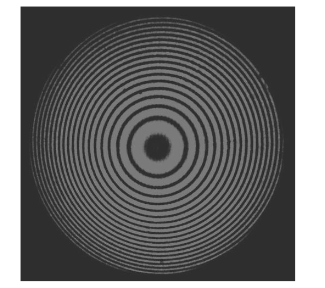
Circular fringes:
|
Figure 11: The circular fringe interference pattern produced by a Michelson interferometer
To view these fringes with monochromatic light, the mirrors must be almost perfectly perpendicular to each other. The conditions for constructive and destructive interference pattern can be understood from Figure 12
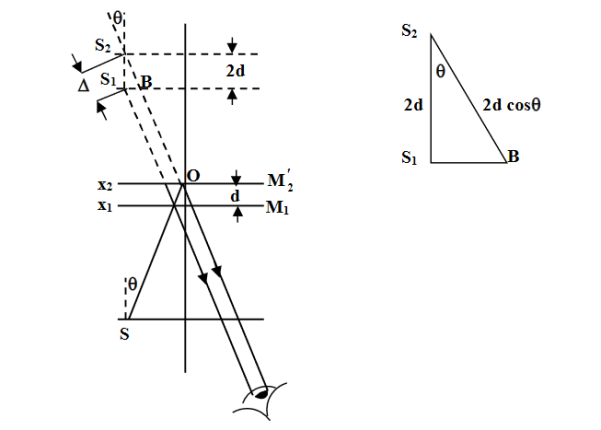
|
Figure 12: Virtual images from the two mirrors created by the light source and the beam splitter in the Michelson interferometer
Let S1 and S2 be the virtual images of a source S, see Figure 12. If the distance between M1 and M2’ is d, then the distance between the virtual images S1 and S2 will be 2d. The geometrical path difference
where, d = x1
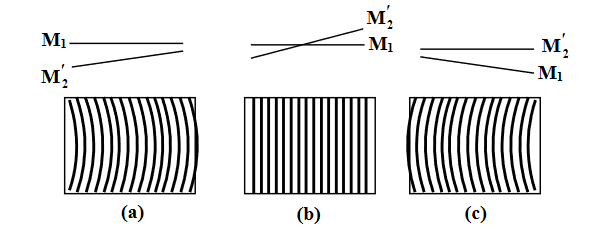
The condition for destructive interference (dark fringes) is: 2dcosθ + λ/2= (2m +1) λ/2 or 2dcosθ = mλ., m = 0, ±1, ±2, ... the order of interference ……... (3) When viewed along the axis θ = 0, then we have: 2d = mλ, m = 0, ±1, ±2, The condition for constructive interference (bright fringes) is: 2dcosθ + λ/2= mλ m = 1, ±1, +2, ... ……... (4) 2dcosθ = (2m +1) λ/2 m = 0, ±1, ±2, ... Expanding the cosine function, it can be shown that the radii of bright fringes are proportional to the square root of integers. The formation of circular fringes for different conditions is shown in Figure 13. • When M1 and M2’are separated by a few centimeters, the fringe system has a general appearance as shown in Figure 13(a). If M1is moved towards M2, the distance d decreases, and from Equation (4), as dcosθ is constant, the order of the fringes m decreases, and the fringe radius decreases. Thus, the rings shrink and vanish at the center. For a ring disappearing each time, 2d decreases by λ or d by λ/2. 2d = m λ since cosθ = 1 • When M1 approaches M2’ r, the rings are more widely spaced as shown in Figure 13 (b). • If the mirror M1 is moved further, the distance between M1 and M2 again increases to give widely spaced rings (Figure 13 (d)), and finally when the distance is large in centimeters, we get rings with smaller radius (Figure 13 (e)).
Figure 13: Shape of circular fringes by varying the movable mirror M1 When the separation between M1 and M2’ decreases, the fringes will move to the left across the field of the telescope, and new fringes crossing the centre of the telescope if d changes by λ/2. If M1 and M2’ are exactly cross with each other, fringes become straight as shown in Figure 14(b), where a wedge-shaped air film is formed. Further movement of M1, as shown in Figure 14(c), the fringes are again curved but in opposite direction. When the distance between M1 and M2’; is too large, the fringes cannot be observed. We conclude that due to the variation of path difference between the reflected light from the mirrors M1 and M2 by changing the distance, the fringe pattern observed contains fringes of equal thickness.
Figure 14: The localized fringe interference pattern–(a) and (c) are depictions of curved fringes, and (b) shows straight, parallel fringes due to wedge-shaped air thin film
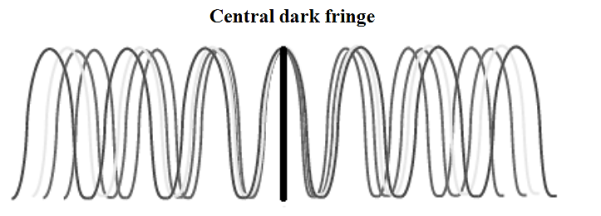
White light source To avoid spurious interference fringes, filters can be used. In the case of monochromatic light, maximum contrast fringes can be obtained with precisely defined focus. Therefore, the white light source will be required in the interferometers. Normally, if white light is used as a source. no fringes will be observed for large path difference. However, for small path differences of the order of few wavelengths, coloured fringes are observed. For observing white light interference, the mirrors are tilted slightly as for localized fringes discussed above and the position of M1 is such that it intersects M2’. At the same time, two important conditions need to be satisfied. • The position of the zero-order interference fringe must be independent of wavelength, i.e., a dark fringe at the centre of the interference pattern. • The spacing of the interference fringes must be independent of wavelength. Overall, the position of interference fringes is independent of order number and wavelength. Generally, in a white light interferometer, only the first condition is satisfied. The white light interference pattern contains a central dark fringe and coloured fringes on either side of the dark fringe. When M1 and M2’coincide, the path difference between the light beams are zero and hence the central fringe is dark in white light fringes. After 8 to 10 fringes from the central dark fringe, so many colours are present at a given point so that it appears white. The white light fringe pattern is shown in Figure 15.
Figure 15: The formation of white light fringes with a dark fringe at the center Applications (i) Determination of wavelength of light: For the determination of the wavelength of light, first, the Michelson interferometer is set for circular fringes with a central bright spot. The mirror M2 is kept fixed and when the mirror M1 is moved, each fringe gets displaced to itself in the field of view of the telescope. If the mirror is moved over a distance d, let m fringes cross the field of view of the telescope, then we have, 2d = mλ ……...... (5) The wavelength of the light source λ =2d/m ……...... (6) By measuring the values of d and m, the wavelength λ can be determined. (ii) Determination of refractive index of the thin film: Consider a thin film of thickness t whose refractive index μm is to be measured will be introduced in one of the paths of interferometer beams, say in the path of beam going towards mirror M2. The thin film causes an additional path difference between the two interfering beams. If m be the number of fringes cross the centre of the field of view, then we have, 2(μm— 1)t = mλ If the reflective index μm and the wavelength λ are known, we can determine the thickness of the thin film t = Or If the thickness of the thin film t and the wavelength λ are known, then the refractive index μm of the thin film can be determined. μm = (iii) Resolution of spectral lines: When a source produces two nearby wavelengths, say λ1 and λ2, then it gives two sets of the interference pattern. When the mirror is advanced, the two sets get in step or out of step alternatively. When a bright fringe of λ1 falls on a bright fringe of λ2, there will be a maximum intensity in the field of view of the telescope. On the other hand, when the bright fringe of λ1 falls on the dark fringe of λ2, there will be a. minimum intensity in the field of view of the telescope. If the distance corresponding to the change from maximum intensity to the minimum intensity is measured as d, then
If λ= (λ1 + λ2)/2, then the spectral resolution is given by the ratio λ / |
Key Takeaways
2dcosθ = mλ., m = 0, ±1, ±2, ... the order of interference 4. The condition for constructive interference (bright fringes) is: 2dcosθ = (2m +1) λ/2 m = 0, ±1, ±2, ... 5. We conclude that due to the variation of path difference between the reflected light from the mirrors M1 and M2 by changing the distance, the fringe pattern observed contains fringes of equal thickness. 6. Determination of wavelength of light by using λ =2d/m 7. Determination of refractive index of the thin film μm = 8. Resolution of spectral lines is given by the ratio λ /
|
Diffraction
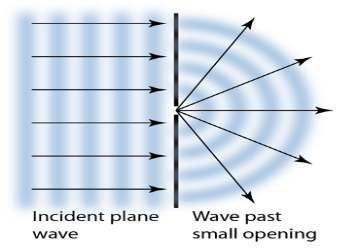
When the light falls on the obstacle whose size is comparable with the wavelength of light then the light bends around the obstacle and enters the geometrical shadow. This bending of light is called diffraction.
Diffraction is a slight bending of light as it passes around the edge of an object. The amount of bending depends on the relative size of the wavelength of light to the size of the opening. If the opening is much larger than the light's wavelength, the bending will be almost unnoticeable. However, if the two are closer in size or equal, the amount of bending is considerable, and easily seen with the naked eye.
|
Figure 16: Diffraction of light
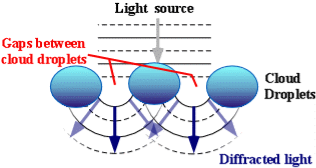
In the atmosphere, diffracted light is bent around atmospheric particles -- most commonly, the atmospheric particles are tiny water droplets found in clouds. Diffracted light can produce fringes of light, dark or colored bands. An optical effect that results from the diffraction of light is the silver lining sometimes found around the edges of clouds or coronas surrounding the sun or moon. The illustration above shows how light (from either the sun or the moon) is bent around small droplets in the cloud.
Optical effects resulting from diffraction are produced through the interference of light waves. To visualize this, imagine light waves like water waves. If water waves were incident upon a float residing on the water surface, the float would bounce up and down in response to the incident waves, producing waves of their own. As these waves spread outward in all directions from the float, they interact with other water waves. If the crests of two waves combine, an amplified wave is produced (constructive interference). However, if a crest of one wave and a trough of another wave combine, they cancel each other out to produce no vertical displacement (destructive interference).
|
Figure 17: Diffraction
This concept also applies to light waves. When sunlight (or moonlight) encounters a cloud droplet, light waves are altered and similarly interact with one another as the water waves described above. If there is constructive interference, (the crests of two light waves combining), the light will appear brighter. If there is destructive interference, (the trough of one light wave meeting the crest of another), the light will either appear darker or disappear entirely.
Types of Diffraction
There are two types of diffractions
- Fresnel Diffraction: Fresnel diffraction is produced when light from a point source meets an obstacle, the waves are spherical and the pattern observed is a fringed image of the object.
- Fraunhofer Diffraction: Fraunhofer diffraction occurs with plane wave-fronts with the object effectively at infinity. The pattern is in a particular direction and is a fringed image of the source.
|
Figure 18: Types of diffractions Fresnel Diffraction & Fraunhofer Diffraction
From the above figure, we observe that the source is located at a finite distance from the slit, and the screen is also at a finite distance from the slit. The source and the screen are not very far from each other. So this is Fresnel diffraction. Here, if suppose the ray of light comes exactly at the edge of the obstacles, the path of the light is changed. So the light bends a little and meets the screen.
A beam of width α travels a distance of α2/λ, called the Fresnel distance before it starts to spread out due to diffraction. But when the source and the screen are far away from each other, and when the source is located at the infinite position, then the ray of light coming from that infinite source are parallel rays of light. So this is Fraunhofer diffraction.
Here we have to make use of the lens. But why do we use the lens? Because in Fraunhofer diffraction, the source is at infinity so the rays of light that pass through the slit are parallel rays of light.
So to make these rays parallel to focus on the screen, we, make use of the converging lens. The zone which we get in front of the slit in the central maxima. On either side of the central maxima, there is a bright zone i.e. 1st maxima.
Comparison
Fraunhofer diffraction | |
1 If the source of light and screen is at a finite distance from the obstacle, then the diffraction is called Fresnel diffraction. | 1 If the source of light and screen is at an infinite distance from the obstacle then the diffraction is called Fraunhofer diffraction. |
2 The corresponding rays are not parallel. | 2 The corresponding rays are not parallel. |
3 The wavefronts falling on the obstacle are not plane. | 3 The wavefronts falling on the obstacle are planes. |
4 No convex lens is needed to converge spherical wavefronts. | 4 Plane diffracting wavefronts are converged by means of a convex lens to produce a diffraction pattern. |
5 Fresnel diffraction patterns on flat surfaces. | 5 Fraunhofer diffraction patterns on spherical surfaces. |
6 Diffraction patterns Change as we propagate them further ‘downstream’ of the source of scattering. | 6 Diffraction patterns Shape and intensity of a Fraunhofer diffraction pattern stay constant. |
7 To obtain Fresnel diffraction, zone plates are used. | 7 To obtain Fraunhofer diffraction, the single-double plane diffraction grafting is used. |
8 Diffraction pattern moves along the corresponding shift in the object. | 8 Diffraction pattern remains in a fixed position. |
DIFFRACTION: HUYGEN’S PRINCIPLE
Huygens' Principle
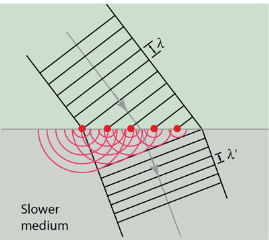
In 1678 Huygens proposed a model where each point on a wavefront may be regarded as a source of waves expanding from that point.
Every point on a wavefront is a source of wavelets that spread out in the forward direction at the same speed as the wave itself. The new wavefront is a line tangent to all of the wavelets.
So Huygens’s Principle states that every point on a wavefront is a source of wavelets, which spread forward at the same speed.
|
Figure 19: Huygens' Principle
The expanding waves may be demonstrated in a ripple tank by sending plane waves toward a barrier with a small opening. If waves approaching a beach strike a barrier with a small opening, the waves may be seen to expand from the opening.
Huygens-Fresnel Theory:
According to Huygens’s wave theory of light, each progressive wave produces secondary waves, the envelope of which forms the secondary wavefront.
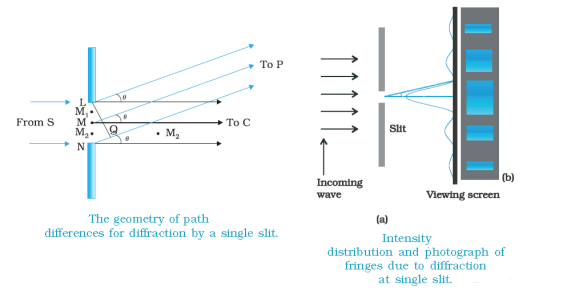
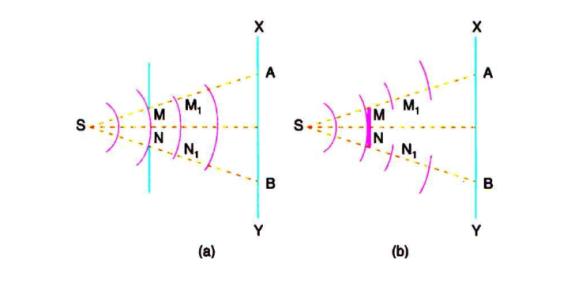
In figure 20 (a). S is a source of monochromatic light and MN is a small aperture. XY is the screen placed in the path of light. AB is the illuminated portion of the screen and above A and below B is the region of the geometrical shadow. Considering MN as the primary wavefront. According to Huygens’s construction, if secondary wavefronts are drawn, one would expect encroachment of light in the geometrical shadow. Thus, the shadows formed by small obstacles are not sharp. This bending of light round the edges of an obstacle or the encroachment of light within the geometrical shadow is known as diffraction. Similarly, if an opaque obstacle MN is placed in the path of light [Figure 20 (b)], there should be illumination in the geometrical shadow region AB also. But the illumination in the geometrical shadow of an obstacle is not commonly observed because the light sources do not point sources and secondly the obstacles used are very large compared to the wavelength of light. If a shadow of an obstacle is cast by an extended source, say a frosted electric bulb, light from every point on the surface of the bulb forms its diffraction pattern (bright and dark diffraction bands) and these overlap such that no single pattern can be identified. The term diffraction is referred to such problems in which one considers the resultant effect produced by a limited portion of a wavefront.
|
Figure 20: Huygens' Principle
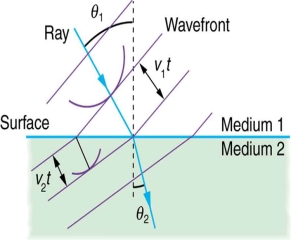
Huygens' principle provides a convenient way to visualize refraction. If points on the wavefront at the boundary of a different medium serve as sources for the propagating light, one can see why the direction of the light propagation changes.
The law of refraction can be explained by applying Huygens’s principle to a wavefront passing from one medium to another (see Figure 21). Each wavelet in the figure was emitted when the wavefront crossed the interface between the media. Since the speed of light is smaller in the second medium, the waves do not travel as far in a given time, and the new wavefront changes direction as shown. This explains why a ray changes direction to become closer to the perpendicular when light slows down.
|
|
(a) | (b) |
Figure 21: Law of refraction by applying Huygens’s principle
If we pass light through smaller openings, often called slits, we can use Huygens’s principle to see that light bends as sound does. The bending of a wave around the edges of an opening or an obstacle is called diffraction.
Diffraction is a wave characteristic and occurs for all types of waves. If diffraction is observed for some phenomenon, it is evidence that the phenomenon is a wave. Thus the horizontal diffraction of the laser beam after it passes through slits is evidence that light is a wave.
Diffraction phenomena are part of our common experience. The luminous border that surrounds the profile of a mountain just before the sun rises behind it, the light streaks that one sees while looking at a strong source of light with half-shut eyes and the colored spectra (arranged in the form of a cross) that one sees while viewing a distant source of light through a fine piece of cloth are all examples of diffraction effects. Augustine Jean Fresnel in 1815, combined in a striking manner Huygens' wavelets with the principle of interference and could satisfactorily explain the bending of light round obstacles and also the rectilinear propagation of light.
Key Takeaways
- When the light falls on the obstacle whose size is comparable with the wavelength of light then the light bends around the obstacle and enters the geometrical shadow. This bending of light is called diffraction.
- There are two types of diffractions Fresnel Diffraction & Fraunhofer Diffraction
- Fresnel diffraction is produced when light from a point source meets an obstacle, the waves are spherical and the pattern observed is a fringed image of the object.
- Fraunhofer diffraction occurs with plane wave-fronts with the object effectively at infinity. The pattern is in a particular direction and is a fringed image of the source.
- Huygens' Principle states that every point on a wavefront is a source of wavelets that spread out in the forward direction at the same speed as the wave itself. The new wavefront is a line tangent to all of the wavelets.
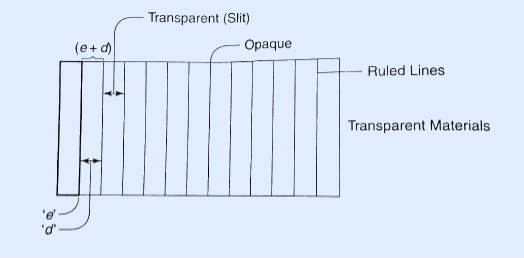
A set of a large number of parallel slits of the same width and separated by opaque spaces is known as a diffraction grating. Diffraction gratings are much more effective than prisms for dispersing light of different wavelengths so they are used almost exclusively in instruments designed to detect and identify characteristic spectral lines. There is nothing mysterious about these devices. Fraunhofer used the first grating consisting of a large number of parallel wires placed side by side very closely at regular separation. Now the gratings are constructed by ruling the equidistance parallel lines on a transparent material such as glass with a fine diamond point. The ruled lines are opaque to light while the space between the two lines is transparent to light and acts as a slit.
Figure 22: Plane Transmission Grating
Let ‘e’ be the width of the line and ‘d’ be the width of the slit. Then (e + d) is known as the grating element. If N is the number of lines per inch on the grating then (𝑒+𝑑)=1𝑖𝑐ℎ=2.54𝑐𝑚 (𝑒+𝑑)=2.54𝑐𝑚/𝑁 Commercial gratings are produced by taking the cost of actual grating on a transparent film like that of cellulose acetate. The solution of cellulose acetate is poured on a ruled surface and allowed to dry to form a thin film, detachable from the surface. This film of the grating is kept between the two glass plates. Example: A parallel beam of monochromatic light of wavelength 500nm is inclined normally on a plane diffraction grating 4000 per centimetre. Calculate the angle of diffraction for first-order principal maximum. Solution: Given: number of lines per cm =4000 Grating element e+b = 1/4000 cm = 2.5 x 10-6m λ=500 nm Order n =1 (e+b) sinθ =nλ sinθ = nλ / (e+b) sinθ = 1 x 500 x 10-9 / 2.5 x 10-6 sinθ =0.2 θ =sin-1 0.2 θ = 11.5o DISPERSIVE POWER OF GRATING Dispersive power of a grating is defined as the ratio of the difference in the angle of diffraction of any two neighbouring spectral lines to the difference in the wavelength between the two spectral lines. Or It can also be defined as the diffraction in the angle of diffraction per unit change in wavelength. The diffraction of the nth order principal maximum for a wavelength λ is given by the equation, a + b is constant and n is constant in a given order.
In equation (2) dθ/dλ is the dispersive power, n is the order of the spectrum, N’ is the number of lines per cm of the grating surface and θ is the angle of diffraction for the nth order principal maximum of wavelength λ. From equation (2), it is clear, that the dispersive power of the grating is directly proportional to the number of lines per cm and inversely proportional to cos θ. Thus, the angular spacing of any two spectral lines is double in the second-order spectrum in comparison to the first order. Secondly, the angular dispersion of the lines is more with a grating having a larger number of lines per cm. thirdly, the angular dispersion is minimum when θ = 0. If the value of θ is not large the value of cosθ can be taken as unity approximately and the influence of the factor cosθ in the equation (2) can be neglected. Neglecting the influence of cosθ, it is clear that the angular dispersion of any two spectral lines (in a particular order) is directly proportional to the difference in wavelength between the two spectral lines. A spectrum of this type is called a normal spectrum. If the linear spacing of two spectral lines of wavelength λ and λ + dλ is dx in the focal plane of the telescope objective or the photographic plate, then, dx = fdθ
|
Key Takeaways
|
It is defined as the capacity of a grating to form separate diffraction maxima of two wavelengths that are very close to each other
It is measured by λ/dλ where dλ is the smallest difference in two wavelengths which are just resolvable by grating and λ is the wavelength of either of them or mean wavelength.
|
Figure 23: Resolving Power of Diffraction Grating
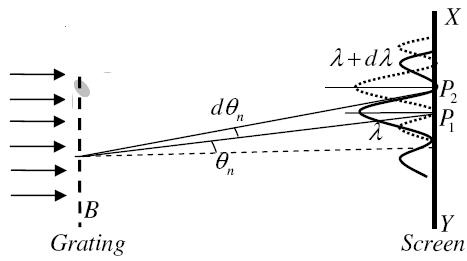
Let AB represent the surface of a plane transmission grating having grating element (e+d) and N total number of slits. Let a beam of light having two wavelengths by λ and dλ is normally incident on the grating. Let P1 is an nth primary maximum of a spectral line of wavelength λ at an angle of diffraction According to the Rayleigh criterion, the two wavelengths will be resolved if the principal maximum λ+dλ of nth order in a direction θ+dθ falls over the first minimum of nth order in the same direction θ+dθ. Let us consider the first minimum of l of nth order in the direction θ+dθ as below. The principal maximum of (e+d)sinθ =nλ ………………(1) The equation of minima is N(e+d)sinθ = mλ ………………(2)
Where m takes all integers except 0, N, 2N, …, nN, because, for these values of m, the condition for maxima is satisfied. Thus first minimum adjacent to the nth principal maximum in the direction θ+dθ can be obtained by substituting the value of ‘m’ as (nN+1). Therefore, the first minimum in the direction of θ+dθ is given by N(e+d)sin(θ+dθ) = (nN+1)λ (e+d)sin(θ+dθ) = (n+ The principal maximum of λ+dλ in direction θ+dθ is given by (e+d)sin(θ+dθ) = n(λ+dλ) ………………(4) Dividing eqn(3) by equation (4), we get (n+ nλ +
Resolving Power = Thus the resolving power is directly proportional to (i) The order of the spectrum ‘n’ (ii) The total number of lines on the grating ‘N Example: A grating has 1100 lines ruled on it. What is the difference between the two wavelengths that just appear resolved in the first-order spectrum in the region of wavelength λ = 660 nm? Solution: N = 1100 nm λ = 660 x 10-9 m n = 1 R = λ /dλ =Nn dλ = λ /Nn dλ = 660 x 10-9 / 1100 x 10-9 x 1 dλ = 0.6 x 10-9 m
NUMERICAL PROBLEM Example: A grating containing 4000 slits per centimetre is illuminated with a monochromatic light and produces the second-order bright line at a 30° angle. What is the wavelength of the light used? (1 Å = 10-10 m) Solution: The distance between slits (d) = 1 / (4000 slits / cm) = 0.00025 cm = 2.5 x 10-4 cm = 2.5 x 10-6 meters Order (n) = 2 Sin 30o = 0.5 1 Å = 10-10 m Wavelength of the light (λ) =?
Example: A monochromatic light with a wavelength of 2.5x10-7 m strikes a grating containing 10,000 slits/cm. Determine the angular positions of the second-order bright line. Solution: Given The distance between slits (d) = 1 / (10,000 slits / cm) = 0.0001 cm = 1 x 10-4 cm = 1 x 10-6 m Order (n) = 2 Wavelength (λ) = 2.5 x 10-7 m Angle (θ)
Example: A monochromatic light with a wavelength of 500 nm (1 nm = 10-9 m) strikes a grating and produces the second-order bright line at a 30° angle. Determine the number of slits per centimetre. Solution: Wavelength (λ) = 500.10-9 m = 5.10-7 m θ = 30o n = 2 Distance between slits: d sin θ = n λ d (sin 30o) = (2)(5.10-7) d (0.5) = 10.10-7 d = (10.10-7) / 0.5 d = 20.10-7 d = 2.10-6 m Number of slits per centimetre: x = 1 / d x = 1 / 2.10-6 m x = 0.5.106 / 1 m x = 0.5.106 / 102 cm x = 0.5.104 cm x = 5.103 /cm x = 5000 /cm
Example: A monochromatic light with a wavelength of 500 nm (1 nano = 10-9) strikes a grating and produces the fourth-order bright line at a 30° angle. Determine the number of slits per centimetre. Solution: Wavelength (λ) = 500.10-9 m = 5.10-7 m θ = 30o n = 4 Distance between slits: d sin θ = n λ d (sin 30o) = (4)(5.10-7) d (0.5) = 20.10-7 d = (20.10-7) / 0.5 d = 40.10-7 d = 4.10-6 m Number of slits per centimetre: x = 1 / 4.10-6 m x = 0.25.106 / m x = 0.25.106 / 102 cm x = 0.25.104 / cm x = 25.102 per cm x = 2500 per cm |
Key Takeaways
- It is defined as the capacity of a grating to form separate diffraction maxima of two wavelengths that are very close to each other
- Resolving Power =
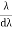 = nN
= nN
The phenomena of interference and diffraction confirm the wave nature of light hence neither of these phenomena can identify the nature of waves that light is. These phenomena do not tell whether the oscillation of light waves is longitudinal or transverse. This is accomplished by the study of polarization.
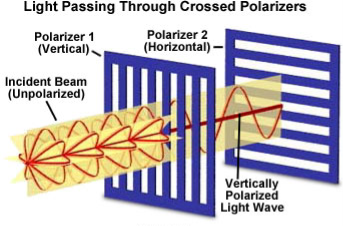
A light wave that is vibrating in more than one plane is referred to as unpolarized light.
Example: Light emitted by the sun, by a lamp in the classroom, or by a candle flame is unpolarized light.
This unpolarized light is created by electric charges that vibrate in a variety of directions, this concept of unpolarized light is difficult to visualize. For simplicity, it is helpful to visualize unpolarized light as a wave that has an average of half its vibrations in a horizontal plane and half of its vibrations in a vertical plane. It is possible to transform unpolarized light into polarized light.
Polarized light waves are light waves in which the vibrations occur in a single plane. The process of transforming unpolarized light into polarized light is known as polarization.
|
Figure 24: Polarization
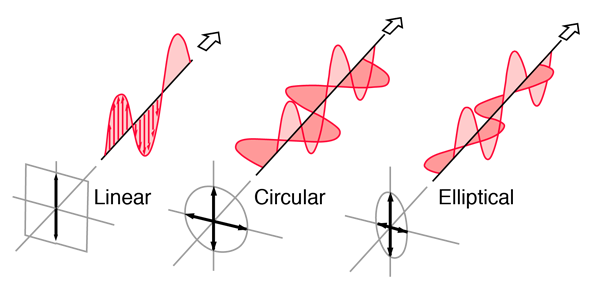
Classification of light
Light in the form of a plane wave in space is said to be linearly polarized. Light is a transverse electromagnetic wave, but natural light is generally unpolarized, all planes of propagation being equally probable. If the light is composed of two plane waves of equal amplitude by differing in phase by 90°, then the light is said to be circularly polarized. If two plane waves of differing amplitude are related in phase by 90°, or if the relative phase is other than 90° then the light is said to be elliptically polarized.
Linearly Polarized: Light in the form of a plane wave in space is said to be linearly polarized. The electric field of light is limited to a single plane along the direction of propagation.
Circularly Polarized If the light is composed of two plane waves of equal amplitude by differing in phase by 90°, then the light is said to be circularly polarized. if the thumb of your right hand were pointing in the direction of propagation of the light, the electric vector would be rotating in the direction of your fingers.
Elliptically Polarized If two plane waves of differing amplitude are related in phase by 90°, or if the relative phase is other than 90° then the light is said to be elliptically polarized. If the thumb of your right hand were pointing in the direction of propagation of the light, the electric vector would be rotating in the direction of your fingers.
|
Figure 25: Showing Linear, Circular and Elliptical Polarization
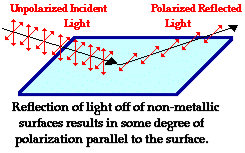
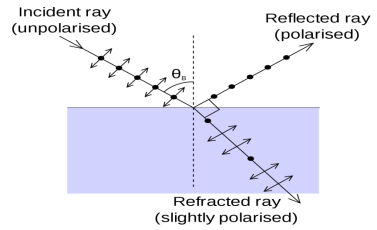
POLARIZATION BY REFLECTION
Production of Polarized Light by Reflection
Brewster’s law can be used to polarise the light by reflection. When unpolarized light is incident on the boundary between two transparent media, the reflected light is polarised with its electric vector perpendicular to the plane of incidence when the refracted and reflected rays make a right angle with each other.
|
Figure 26: Polarization by Reflection
- Tangential component- in the plane of incidence.
- Normal components – in the plane perpendicular to the plane of incidence.
The angle of incidence, in this case, is called Brewster’s angle
|
Figure 27: Polarization by Reflection
If the angle of incidence θi is varied, the reflected coefficient ‘r’ for the em waves corresponding to the tangential component will vary as shown in the figure. As θi increases r will decrease.
At θi = Brewster angle (about 57° for glass) r will be zero and no reflection of the tangential component will take place.
The reflected beam will contain light waves having only the normal component for the electric field as shown in the figure that is the reflected light will be plane-polarized with the oscillations of electric field vector normal to the plane of incidence.
Cause of Polarization by Reflection
The em waves are generated because of the oscillation of electric charge. The electric field vectors of the transmitted ray act on the electrons in the dielectric.
Since the intensity of em wave emitted by the oscillating charge is maximum in a direction normal to the plane of oscillation, and zero along the line of oscillations for the plane-polarized light having electric field vector in the plane of incidence, there will be no emission of em waves in a direction normal to the reflected ray. In the figure, the direction along which no emission should occur is called a zero intensity line. Let the angle between the reflected ray and the zero intensity line be 𝛿 the angle between the reflected and transmitted ray is π/2 + 𝛿 suppose we arrange the angle of incidence such that
n=sinθi / sinθr n=sinθi / sin(π/2 – θi) n=sinθi / cosθi n=tan θi that is possible when θi + θr = π/2 according to Brewster’s law from figure θi + θr+ π/2 + = π As θi + θr = π/2 So π/2+ π/2 + = π = 0 |
The line of zero intensity and the reflected ray will coincide. Thus there will be no reflection of light when the angle of incidence is equal to Brewster’s angle.
Thus the unpolarized light can be polarized by reflection as the reflected beam will contain light waves having electric field vector oscillating along normal to the plane of the incidence only.
Key Takeaways
- Polarized light waves are light waves in which the vibrations occur in a single plane. The process of transforming unpolarized light into polarized light is known as polarization.
- Light in the form of a plane wave in space is said to be linearly polarized.
- Light is a transverse electromagnetic wave, but natural light is generally unpolarized, all planes of propagation being equally probable.
- If the light is composed of two plane waves of equal amplitude by differing in phase by 90°, then the light is said to be circularly polarized.
- If two plane waves of differing amplitude are related in phase by 90°, or if the relative phase is other than 90° then the light is said to be elliptically polarized.
- Brewster’s law can be used to polarise the light by reflection.
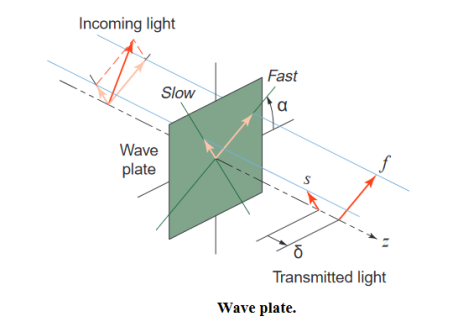
A half-wave plate functions as a polarization rotator for linearly polarized light. It rotates the polarization of a linearly polarized light by twice the angle between its optic axis and the initial direction of polarization, as shown in Figure 28. It introduces a phase difference of radians between the
A typical wave plate is simply a birefringent crystal or a double refracting plastic foil with a carefully chosen thickness. If a beam of parallel light strikes perpendicularly a wave plate the light beam is split into two components due to its double refracting properties. The two components have planes of oscillation perpendicular to each other and slightly different phase velocities.
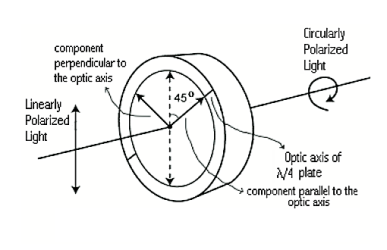
For a quarter-wave plate, the thickness of the foil is chosen in such a manner that the light component whose electric field vector oscillates in parallel to the rotation lever lags by a λ/4 behind other perpendicular oscillating light components. For a half-wave plate, the thickness is chosen so that the created phase difference has the amount of λ/2. In this experiment monochromatic light falls on a quarter-wave and half-wave plate. The polarization of the emergent light is investigated at different angles between the optic axis of the wave plates and the direction of the incident light.
|
Figure 28: wave plate
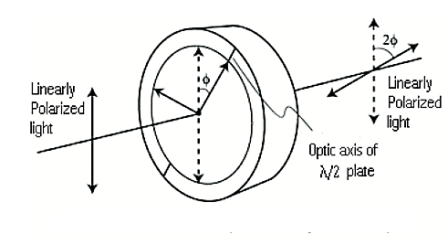
Half-wave plate
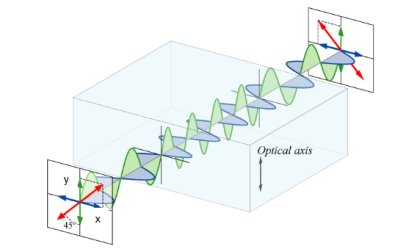
A half-wave plate functions as a polarization rotator for linearly polarized light. It rotates the polarization of a linearly polarized light by twice the angle between its optic axis and the initial direction of polarization, as shown in Figure 29. It introduces a phase difference of radians between the two components of the electric field vectors.
Suppose a plane-polarized wave is normally incident on a wave plate, and the plane of polarization is at an angle ϕ with respect to the fast axis, as shown. After passing through the plate, the original plane wave has been rotated through an angle 2ϕ.
|
Figure 29: Half-wave plate
A half-wave plate is very handy in rotating the plane of polarization from a polarized laser to any other desired plane (especially if the laser is too large to rotate). Most large ion lasers are vertically polarized. To obtain horizontal polarization, simply place a half-wave plate in the beam with its fast (or slow) axis 45° to the vertical. The l/2 plates can also change left circularly polarized light into right circularly polarized light or vice versa. The thickness of the half waveplate is such that the phase difference is 1/2 wavelength (l/2, Zero order) or a certain multiple of 1/2-wavelength [(2n+1)l/2, multiple order].
Quarter Wave Plate
|
Figure 30: Quarter Wave Plate
Key Takeaways
- A wave plate or retarder is an optical device that alters the polarization state of a light beam traveling through it.
- There are many types of retarders. Some common retarders are quarter-wave (λ/4) plates and half-wave(λ/2) plates.
- A half-wave plate functions as a polarization rotator for linearly polarized light.
- A quarter-wave plate is used to convert linear polarization to circular polarization and vice-versa.
Optical Activity
The optical activity was first observed in quartz crystals in 1811 by a French physicist, François Arago. Louis Pasteur was the first to recognize that optical activity arises from the dissymmetric arrangement of atoms in the crystalline structures or in individual molecules of certain compounds.
Optical activity, the ability of a substance to rotate the plane of polarization of a beam of light that is passed through it. (In plane-polarized light, the vibrations of the electric field are confined to a single plane.
The intensity of optical activity is expressed in terms of a quantity, called specific rotation, defined by an equation that relates the angle through which the plane is rotated, the length of the light path through the sample, and the density of the sample (or its concentration if it is present in a solution). Because the specific rotation depends upon the temperature and upon the wavelength of the light, these quantities also must be specified. The rotation is assigned a positive value if it is clockwise with respect to an observer facing the light source, negative if counterclockwise. A substance with a positive specific rotation is described as dextrorotatory and denoted by the prefix d or (+); one with a negative specific rotation is levorotatory, designated by the prefix l or (-).
|
Figure 31: Polarimeter
Measuring Optical Activity
When rotation is quantified using a polarimeter it is known as an observed rotation, because rotation is affected by path length (l, the time the light travels through a sample) and concentration (c, how much of the sample is present that will rotate the light). When these effects are eliminated a standard for comparison of all molecules is obtained, the specific rotation, [a].
[a] = 100a / cl when concentration is expressed as g sample /100ml solution
Enantiomers will rotate the plane of polarisation in exactly equal amounts (same magnitude) but in opposite directions.
As already discussed Dextrorotary designated as d or (+), clockwise rotation (to the right) Levorotary designated as l or (-), anti-clockwise rotation (to the left).
If only one enantiomer is present a sample is considered to be optically pure. When a sample consists of a mixture of enantiomers, the effect of each enantiomer cancels out, molecule for molecule.
For example, a 50:50 mixture of two enantiomers of a racemic mixture will not rotate plane polarised light and is optically inactive. A mixture that contains one enantiomer excess, however, will display a net plane of polarisation in the direction characteristic of the enantiomer that is in excess.
Key Takeaways
- Optical activity is the ability of a chiral molecule to rotate the plane of plane-polarised light, measured using a polarimeter.
- The intensity of optical activity is expressed in terms of a quantity, called specific rotation,
- specific rotation, [a] is given as [a] = 100a / cl when concentration is expressed as g sample /100ml solution
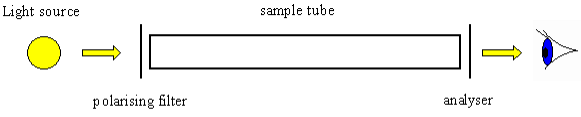
Laurent’s half shade Polarimeter
Polarimeter – it is an optical instrument used to measure the angle of rotation of plane-polarized light when it is passed through an optically active substance.
By measuring the angle, the specific rotation of an optically active substance can be determined.
Two types of polarimeters are generally used in the laboratory nowadays Laurent’s Half Shade Polarimeter and Biquartz Polarimeter. We will discuss Laurent’s Half Shade Polarimeter.
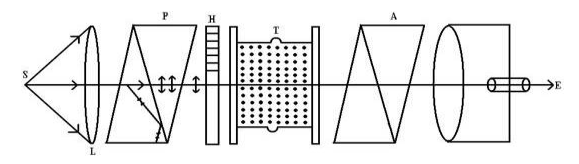
Construction
- S is the source of monochromatic light placed at the focus of convex
- Just after the convex lens, there is a Nicol Prism P is placed. This prism acts as a polariser.
- H is a half shade device that divides the field of polarised light emerging out of the Nicol P into two halves generally of unequal brightness.
- T is a glass tube in which an optically active solution is filled.
- The light after passing through T is allowed to fall on the analyzing Nicol A which can be rotated about the axis of the tube. (Don’t get confused here, we already discussed in the previous section that Nicol prism acts as a polariser as well as an analyzer).
- The rotation of the analyzer can be measured with the help of a scale.
|
Figure 32: Laurent’s half shade polarimeter
Working
To understand the working of this device we have to know the need for a Laurent half shade device. For this, we assume that the half shade device is not present.
In this basic setup (without the half-shade A) you are looking for the maximum and minimum brightness, which then tells you that the analyzer A is precisely aligned with the output rotation.
When the tube is empty placed the analyzer in such a position that the view of the field is completely dark. Note the position of the analyzer with the help of a circular scale.
Now filled the tube with an optically active solution and it is set in its proper position. The optically active solution rotates the plane of polarization of the light emerging out of the polariser P by some angle. So the light is transmitted by analyzer A and the field of view of the telescope becomes bright.
Now the analyzer is rotated by a finite angle so that the field of view of the telescope again becomes dark. This will happen only when the analyzer is rotated by the same angle by which the plane of polarization of light is rotated by the optically active solution.
The position of the analyzer is again noted. The difference between the two readings will give you the angle of rotation of the plane of polarization.
But here the difficulty associated with this procedure that when the analyzer is rotated for the total darkness, then it is attained gradually and hence it is difficult to find the exact position correctly for which complete darkness is obtained.
|
Figure 33: Laboratory Set-Up of Polarimeter
To overcome the above difficulty half shade device is introduced between polariser P and glass tube T.
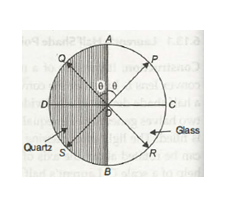
Half Shade Device
- It consists of two semi-circular plates ACB and ADC.
- One-half ACB is made of glass while the other half is made of quartz.
- Both the halves are joined together.
- The quartz is cut parallel to the optic axis.
- The thickness of the quartz is selected in such a way that it introduces a path difference of ‘A/2 between ordinary and extraordinary rays.
- The thickness of the glass is selected in such a way that it absorbs the same amount of light as is absorbed by quartz half.
|
Figure 34: Half Shade Device
- Let us consider that the vibration of polarisation is along with OP. On passing through the glass half the vibrations remain along with OP. But on passing through quartz half of these vibrations will split into o and e-components.
- The e-components are parallel to the optic axis while the o-component is perpendicular to the optic axis.
- The o-component travels faster in quartz and hence an emergence 0-component will be along with OD instead of along OC.
- Thus components OA and OD will combine to form a resultant vibration along OQ which makes the same angle with the optic axis as OP.
Now if the Principal plane of the analyzing Nicol is parallel to OP then the light will pass through a glass half passable. Hence glass half will be brighter than the quartz half or we can say that the glass half will be bright and the quartz half will be dark. Similarly if the principal plane of analyzing. Nicol is parallel to OQ then quartz half will be bright and glass half will be dark.
When the principal plane of the analyzer is along AOB then both halves will be equally bright. On the other hand, if the principal plane of the analyzer is along with DOC. then both the halves will be equally dark.
Thus it is clear that if the analysis Nicol is slightly disturbed from DOC then one half becomes brighter than the other. Hence by using a half shade device, one can measure the angle of rotation more accurately.
Mathematically
The specific rotation is given by S=θ/lC where l is the length of the tube and C is concentration.
Key Takeaways
- Polarimeter – it is an optical instrument used to measure the angle of rotation of plane-polarized light when it is passed through an optically active substance.
- Two types of polarimeters are generally used in the laboratory nowadays Laurent’s Half Shade Polarimeter and Biquartz Polarimeter.
- The specific rotation is given by S=θ/lC where l is the length of the tube and C is concentration.
Optical elements
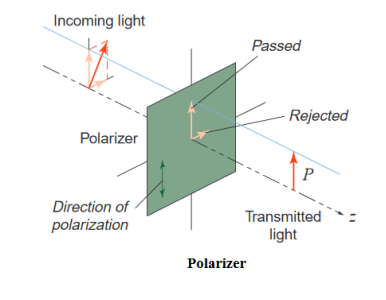
The method of photoelasticity requires the use of two types of optical elements—the polarizer and the wave plate.
Polarizer: A polarizer is an element that converts randomly polarized light into plane-polarized light. It was the introduction of large polarizing sheets by Polaroid Corporation in 1934 that led to the rapid advance of photoelasticity is a stress-analysis tool.
Before that, small naturally occurring crystals were used for this purpose. In the figure, a single light wave with arbitrarily oriented wave amplitude approaches the polarizer from the left. As this wave encounters the polarizer, it is resolved into two vector components—one parallel to the polarizing direction of the
|
Figure 35: Polarizer
Wave plate: A wave plate resolves incident light into two components, but instead of rejecting one of these components, it retards it relative to the other component.
|
Figure 36: Wave plate
Photoelastic materials are birefringent, that is, they act as temporary wave plates, refracting light differently for different light-amplitude orientations, depending upon the state of stress in the material.
When photoelastic materials are subjected to pressure, internal strains develop that can be observed in polarized light; i.e., light vibrating normally in two planes, which has had one plane of vibration removed by passing through a substance called a polarizer. Two polarizers that are crossed ordinarily do not transmit light, but if a stressed material is placed between them and if the principal axis of the stress is not parallel to this plane of polarization, some light will be transmitted in the form of coloured fringes.
Stresses in opaque mechanical structures can be analyzed by making models in plastic and studying the fringe pattern under polarized light, which may be either white (a mixture of all wavelengths) or a single wavelength.
Advantages and Disadvantages
Advantages
- Photoelasticity, as used for two-dimensional plane problems,
- Provides reliable full-field values of the difference between the principal normal stresses in the plane of the model
- Provides uniquely the value of the non-vanishing principal normal stress along the perimeter(s) of the model, where stresses are generally the largest
- Furnishes full-field values of the principal-stress directions (sometimes called stress trajectories
- It is adaptable to both static and dynamic investigations
- Requires only a modest investment in equipment and materials for ordinary work
- It is fairly simple to use
Disadvantages
On the other hand, photo-elasticity
- Requires that a model of the actual part be made (unless photoelastic coatings are used)
- Requires rather tedious calculations to separate the values of principal stresses at a general interior point
- Can require expensive equipment for precise analysis of large components
- It is very tedious and time-consuming for three-dimensional work.
Key Takeaways
- Photoelasticity is the property of some transparent materials, such as glass or plastic, while under stress, to become doubly refracting.
- The method of photoelasticity requires the use of two types of optical elements—the polarizer and the wave plate.
- A polarizer is an element that converts randomly polarized light into plane-polarized light
- A wave plate resolves incident light into two components, but instead of rejecting one of these components, it retards it relative to the other component.
Reference Books
I. Engineering physics- Gaur and Gupta, & S.Chand Publication
2. Engineering physics - Avadhanalu and Kshirsagar, S.Chand Publication
3. Fundamentals of optics-Jenkins and White. McGraw Hill Publication
4. A Text Book of Optics Subrahmanyam, BrijIal, S. Chand Publication
5. Engineering physics- Hitendra K Malik, A.K.Singh, Tata McGraw Hill Education Private Limited, New Delhi
6 Essential University Physics Volume – l and 2 Richard Wolfson, Pearson, Noida 7. Engineering Physics Dattu R Joshi Tata Mc-Graw Hill Education Private Limited