Unit-2

Physics

2.1 Newton’s Rings

Newton’s Rings are the circular interference pattern first discovered by physicist Sir Isaac Newton in 1704. It is cosists of concentric bright and dark rings with the point of contact of lens and the glass plate as centre,

The fringes obtained by interference of light waves by using the following arrangement

When a Plano convex lens with large radius of curvature is placed on a plane glass plate such that its curved surface faces the glass plate, a wedge air film (of gradually increasing thickness) is formed between the lens and the glass plate. The thickness of the air film is zero at the point of contact and gradually increases away from the point of contact.

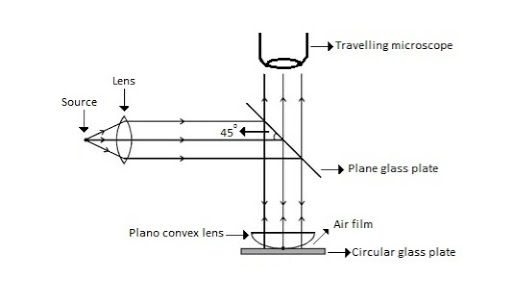
Figure Newton Ring Assembly

If monochromatic (means light with single wavelength) light is allowed to fall normally on the lens from a source 'S', then two reflected rays R1 (reflected from upper surface of the film) and R2 (reflected from lower surface of the air film) interfere to produce circular interference pattern. This interference pattern has concentric alternate bright and dark rings around the point of contact. This pattern is observed through traveling microscope.

Mathematical analysis of Newton’s Ring

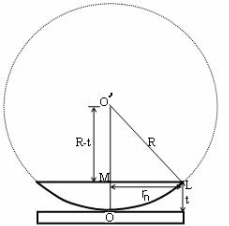
(OL)2 =(O’M)2-(ML)2   ……….(1)

R2=(R-t)2 +rn2

R2=R2 +t2-2Rt +rn2

Radius is large as compared to the thickness

So t2 is neglected as t2<< R2

R2=R2 +-2Rt +rn2

2Rt =rn2

Thickness of the film   t =rn2 /2R  ……….(2)

Theory of Fringes:

The effective path difference between the two reflected rays R1 and R2 for a wedge shaped film from equation

∆ = 2μtcos(r+θ) +λ/2  ……….(3)

If the light is incident normally on the lens,

r = 0 and near to point of contact θ is small;

Therefore near point of contact, (r+θ) approaches to 0 and cos(r+θ)=cos0=1

Therefore

∆ = 2μt+λ/2  ……….(4)

Also At point of contact t = 0 therefore the effective path difference ∆ = λ/2

Which is odd multiple of λ/2 Therefore the Central fringe is dark.

Bright Fringe : Condition of Maxima

For the condition of maxima the effective path difference

∆ = ±nλ

Using equation (4) ∆ = 2μt+λ/2 we have

2μt+λ/2= ±nλ

2μt = ± (2n-1)λ /2   ……….(5)

Diameter of Bright Rings

We know by equation (2)  t =rn2 /2R  substitute in equation (5) we have

2μ (rn2 /2R) = ± (2n-1)λ /2

rn2  = ± (2n-1)λR /2μ

We know diameter D=2r and for nth fringe Dn=2rn

So we have Dn2=± 2(2n-1)λR /μ

Dn=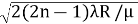

The medium enclosed between the lens and glass plate is if air therefore, 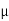=1. The diameter of nth order bright fringe will be

D=  n=0,1,2,3,4……. ……….(6)

The diameter of bright ring is proportional to square root of odd natural numbers

Dark Fringe : Condition for Minima  

For the condition of minima, The effective path difference

∆ =± (2n+1)λ /2

2μt+λ/2 =± (2n+1)λ /2

2μt= ±nλ    ……….(7)

It is clear that for particular dark or bright fringe t should be constant.

Every fringe is the locus of points having equal thickness. Hence the fringes are circular in shape.

Diameter of Dark  Rings

We know by equation (2)  t =rn2 /2R  substitute in equation (7) we have

2μ (rn2 /2R  ) = nλ

rn2  = nλR/ μ

We know diameter D=2r and for nth fringe Dn=2rn

So we have Dn2= 4nλR/ μ

Dn=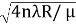

The medium enclosed between the lens and glass plate is if air therefore, =1. The diameter of nth order bright fringe will be

Dn= 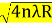 n=0,1,2,3,4…….  ……….(8)

The diameter of dark ring is proportional to square root of natural numbers.

Spacing between Fringes

The Newton’s rings are not equally spaced because the diameter of ring does not increase in the same proportion as the order of ring and rings get closer and closer as ‘n’ increases.

For example the diameter of dark ring is given by Dn= 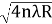   where n=0,1,2,3,4…….             

D3 - D2 =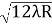 -  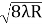  = (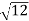 -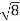) 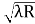  = 0.635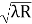  

D7 – D6 =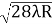 -  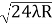  = (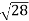 -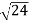) 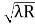  = 0.392  

D10– D9 =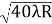 -    = (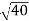 -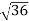) 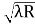  = 0.324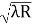  

From above result we conclude that the fringe width reduces with increase in n.

Newton’s Ring with White Light

If the monochromatic source is replaced by the white light, dark and bright fringes are not produced. Because the diameter of the rings depends upon wavelength and it is proportional to the square root of wavelength.

If If the monochromatic source is replaced by the white light superposition of rings take place due to different wavelength. Few coloured rings are seen around dark centre later illumination is seen in the field of view. As shown in below figure.

2.2 Polarization Of Light

The phenomena of interference and diffraction confirm the wave nature of light hence neither of these phenomena is able to indentify the nature of waves that light is. These phenomena do not tell whether the oscillation of light waves are longitudinal or transverse in nature. This is accompalished by the study of polarization.

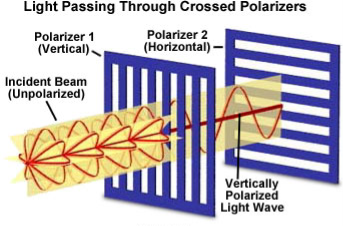
A light wave that is vibrating in more than one plane is referred to as unpolarized light.

Example: Light emitted by the sun, by a lamp in the classroom, or by a candle flame is unpolarized light.

These unpolarized light is created by electric charges that vibrate in a variety of directions, this concept of unpolarized light is difficult to visualize. For simplicity it is helpful to visualize unpolarized light as a wave that has an average of half its vibrations in a horizontal plane and half of its vibrations in a vertical plane. It is possible to transform unpolarized light into polarized light.

Polarized light waves are light waves in which the vibrations occur in a single plane. The process of transforming unpolarized light into polarized light is known as polarization.

Classification of light

We know that Light is a transverse electromagnetic wave, but natural light is generally unpolarized, all planes of propagation being equally probable.

Linearly Polarized: Light in the form of a plane wave in space is said to be linearly polarized. The electric field of light is limited to a single plane along the direction of propagation.

Circularly Polarized If light is composed of two plane waves of equal amplitude by differing in phase by 90°, then the light is said to be circularly polarized. if the thumb of your right hand were pointing in the direction of propagation of the light, the electric vector would be rotating in the direction of your fingers.

Elliptically Polarized If two plane waves of differing amplitude are related in phase by 90°, or if the relative phase is other than 90° then the light is said to be elliptically polarized. If the thumb of your right hand were pointing in the direction of propagation of the light, the electric vector would be rotating in the direction of your fingers.

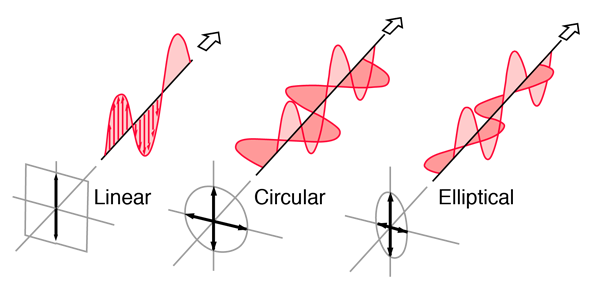
Figure Showimg Linear, Circular and Elliptical Polarization

2.3 Production of Polarized Light by Reflection

The Brewster’s law can be used to polarise the light by reflection. When unpolarised light is incident on the boundary between two transparent media, the reflected light is polarised with its electric vector perpendicular to the plane of incidence when the refracted and reflected rays make a right angle with each other.

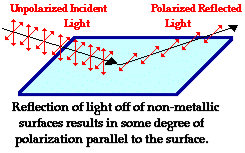
Thus we have seen that when reflected wave is perpendicular to the refracted wave, the reflected wave is a totally polarised wave. The electric field vectors of all unpolarized light may be resolved into two components as follows;

1. Tangential component- in the plane of incidence.
2. Normal components – in the plane perpendicular to plane of incidence.

The angle of incidence in this case is called Brewster’s angle

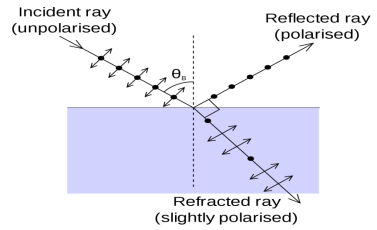
If the angle of incidence θi is varied, the reflected coefficient ‘r’ for the em waves corresponding to the tangential component will vary as shown in figure. As  θi increases r will decrease.

At θi = Brewster angle (about 57° for glass) r will be zero and no reflection of the tangential component will take place.

The reflected beam will contain light waves having only the normal component for the electric field as shown in figure that is the reflected light will be plane polorized with the oscillations  of electric field vector normal to the plane of incidence.

Cause of Polrizaton by Reflection

The em waves are generated because of the oscillation of electric charge. The electric field vectors of the transmitted ray act on the electrons in the dielectric.

Since the intensity of em wave emitted by the oscillating charge is maximum in a direction normal to the plane of oscillation, and zero along the line of oscillations for the plane polarized light having electric field vector in the plane of incidence, there will be no emition of em waves in a direction normal to the reflected ray. In figre the direction along which no emission should accur is called as zero intensity line. Let the angle between the reflected ray and the zero intensity line be 𝛿  the angle between the reflected and tranmitted ray is  π/2 + 𝛿  suppose we arrange the angle of incidence such that

n=sinθi / sinθr

n=sinθi / sin(π/2 – θi)

n=sinθi / cosθi

n=tan θi

That is possible when

θi + θr = π/2  according to Brewster’s law

From figure

θi + θr+ π/2 +   = π

As  θi + θr = π/2  

So  π/2+ π/2 +   = π

= 0

The line of zero intensity and the refected ray will coincide. Thuse there will be no reflection of light when the angle of incidence is equal to Brewster’s angle.

Thus the unpolarized light can be polarized by reflection as the reflected beam will contain light waves having electric field vector oscilating along normal to the plane of the incidence only.

2.4 Production of Polarized Light by Refraction

Polarization can also occur by the refraction of light. Refraction occurs when a beam of light passes from one material into another material. At the surface of the two materials, the path of the beam changes its direction. The refracted beam acquires some degree of polarization.

Birefringence, an optical property in which a single ray of unpolarized light entering an anisotropic medium is split into two rays, each traveling in a different direction. One ray (called the extraordinary ray) is bent, or refracted, at an angle as it travels through the medium; the other ray (called the ordinary ray) passes through the medium unchanged.

The ordinary ray and the extraordinary ray are polarized in planes vibrating at right angles to each other. The refractive index of the ordinary ray is constant in all directions; the refractive index of the extraordinary ray varies according to the direction because it has components that are both parallel and perpendicular to the crystal’s optic axis. An extraordinary ray can move either faster or slower than an ordinary ray.

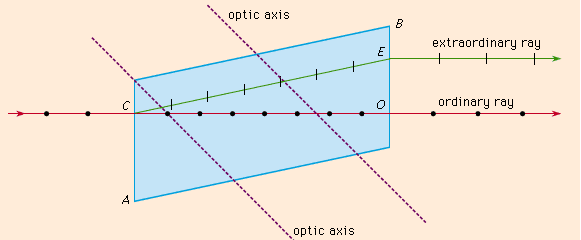
The Figure shows the phenomenon of double refraction through a calcite crystal. An incident ray is seen to split into the ordinary ray CO and the extraordinary ray CE upon entering the crystal face at C. If the incident ray enters the crystal along the direction of its optic axis, however, the light ray will not become divided.

The polarization occurs in a plane perpendicular to the surface. The polarization of refracted light is demonstrated by a unique crystal that serves as a double-refracting crystal.

Iceland Spar (calcite mineral), refracts incident light into two different paths. The light is split into two beams upon entering the crystal.

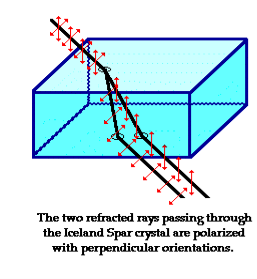
That mean if we see an object through an Iceland spar crystal, two images will observed. These two images are the result of the double refraction of light. Both refracted light beams are polarized - one in a direction parallel to the surface and the other in a direction perpendicular to the surface

Since these two refracted rays are polarized with a perpendicular orientation, a polarizing filter can be used to completely block one of the images. If the polarization axis of the filter is aligned perpendicular to the plane of polarized light, the light is completely blocked by the filter; meanwhile the second image is as bright as can be. And if the filter is then turned 90-degrees in either direction, the second image reappears and the first image disappears.

Negative and Positive Crystals:

Suppose vo and ve be the velocities of the ordinary and extraordinary rays. The crystals in which vo<ve are termed as negative  and in which vo>ve are termed as positive. Calcite is negative crystal and quartz is an example of positive crystal. 

Uniaxial and Biaxial crystals:

Uniaxial crystal: A crystal which has only one optic axis is called uniaxial crystal. The refractive index of the ordinary ray is constant for any direction in the crystal. Examples:- Calcite, Quartz, Ice, Tourmaline etc.

Biaxial crystal: A crystal which has only two optic axis is called biaxial crystal. The refractive index of the extraordinary ray is variable and depends on the direction. Examples:- Mica, Topaz, Borax etc.

All transparent crystals except those of the cubic system, which are normally optically isotropic, exhibit the phenomenon of double refraction: in addition to calcite, some well-known examples are ice, mica, quartz, sugar, and tourmaline. Other materials may become birefringent under special circumstances.

For example, solutions containing long-chain molecules exhibit double refraction when they flow; this phenomenon is called streaming birefringence. Some isotropic materials like glass may even exhibit birefringence when placed in a magnetic or electric field or when subjected to external stress.

2.5 Double Refraction by Nicol Prism 

This was invented by William Nicol in the year 1828. Nicol prism is made from a double refracting calcite crystal.

A Nicol Prism is a type of polarizer, an optical device used to produce a polarized beam of light.

It is made in such a way that it eliminates one of the rays by total internal reflection, i.e. the ordinary ray is eliminated and only the extraordinary ray is transmitted through the prism.

Construction of Nicol prism:

• Length of calcite crystal is three times its breath.
• The corners A′G′ is blunt and A′C G′E is the principal section with ∠A′CG′ = 71°.
• The end faces A′BCD and EFG′H are grounded so that the angle ACG = 68°.
• The crystal is cut along the plane AKGL. The cut surfaces are polished until they are optically flat and cemented together with Canada balsam.

The refractive index of Canada balsam (1.55) is in between the refractive indices of ordinary (1.658) and extraordinary (1.486) rays in calcite crystal.

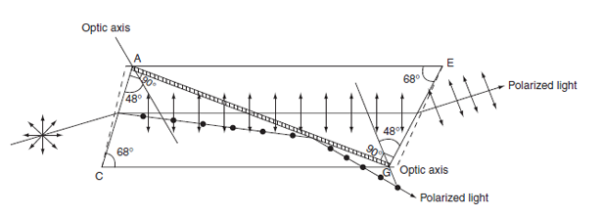Figure Production of plane polarized light using Nicol prism

Working

• The diagonal AG represents the Canada balsam layer.

• A beam of unpolarized light is incident parallel to the lower edge on the face ABCD.
• The ray gets doubly refracted on entering into the crystal.
• From the refractive index values, we know that the Canada balsam acts as a rarer medium for the ordinary ray and it acts as a denser medium for extraordinary ray.
• When the angle of incidence for ordinary ray on the Canada balsam is greater than the critical angle then total internal reflection takes place, while the exaordinary ray gets transmitted through the prism.
• Nicol prism can be used as an analyser.

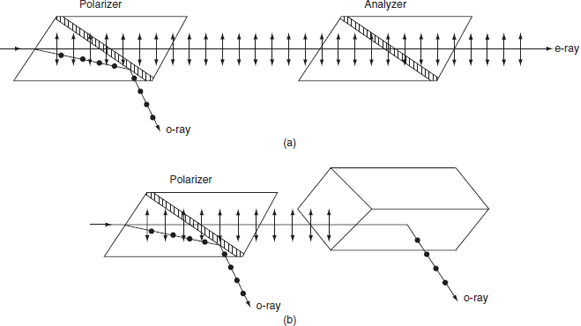
Figure (a) Parallel Nicols; (b) Crossed Nicols

• Two nicol prisms are placed adjacently as shown in figure.
• One prism acts as a polarizer and the other act as an analyzer.
• The exaordinary ray passes through both the prisms.
• If the second prism is slowly rotated, then the intensity of the exaordinary ray decreases.
• When they are crossed, no light come out of the second prism because the e-ray that comes out from first prism will enter into the second prism and act as an ordinary ray. So, this light is reflected in the second prism. The first prism is the polarizer and the second one is the analyser.

2.6 Applications of Polarization

Polarization is widely used in many applications.

• Polaroid glasses are used to reduce the amount of light that is approachable to eye. It is used in sunglasses to reduce the glare.
• 3D movies are possible because of polarisation of light. It is also used in the entertainment industry to produce and show 3-D movies. Three-dimensional movies are actually two movies being shown at the same time through two projectors. The two movies are filmed from two slightly different camera locations. Each individual movie is then projected from different sides of the audience onto a metal screen. The movies are projected through a polarizing filter. This gives the viewer a perception of depth.
• Polarization is used for differentiating between transverse and longitudinal waves.
• Laser is an outcome of polarisation of waves.
• Infrared spectroscopy uses polarization.
• In Chemistry, the chirality of organic compounds is tested using polarization techniques. 
• Polarization Polaroid filters are used in plastic industries for performing stress analysis tests.
• Polarisation is useful in receiving and transmitting wave signals. There has to be an aerial alignment of the polarised waves in the plane in order for them to be able to receive maximum signal.

Our model of the polarization of light provides some substantial support for the wavelike nature of light. It would be extremely difficult to explain polarization phenomenon using a particle view of light. Polarization would only occur with a transverse wave. For this reason, polarization is one more reason why scientists believe that light exhibits wavelike behaviour.

2.7 Laurent’s half shade Polarimeter

Polarimeter – it is an optical instrument used to measure the angle of rotation of plane polarized light when it is passed through an optically active substance.

By measuring the angle, the specific rotation of an optically active substance can be determined.

Two types of polarimeters are generally used in the laboratory now a days Laurent’s Half Shade Polarimeter and Biquartz  Polarimeter.  We will discuss Laurent’s Half Shade Polarimeter.

Construction

• S is source of monochromatic light placed at the focus of convex
• Just after the convex lens there is a Nicol Prism P is placed. This prism acts as a polariser.
• H is a half shade device which divides the field of polarised light emerging out of the Nicol P into two halves generally of unequal brightness.
• T is a glass tube in which optically active solution is filled.
• The light after passing through T is allowed to fall on the analyzing Nicol A which can be rotated about the axis of the tube. (Don’t get confuse here, we already discussed in previous section that Nicol prism acts as a polariser as well as analyser).
• The rotation of analyser can be measured with the help of a scale.

Laurent’s half shade polarimeter is shown in Figure

Working

To understand the working of this device we have to know the need of a Laurent half shade device. For this we assume that half shade device is not present.

In this basic setup (without the half-shade A) you are looking for the maximum and minimum brightness, which then tells you that the analyzer A is precisely aligned with the output rotation.

When tube is empty placed the analyzer in such a position that the view of field is completely  dark. Note the position of the analyzer with the help of circular scale.

Now filled the tube with optically active solution and it is set in its proper position. The optically active solution rotates the plane of polarization of the light emerging out of the polariser P by some angle. So the light is transmitted by analyzer A and the field of view of telescope becomes bright.

Now the analyzer is rotated by a finite angle so that the field of view of telescope again become dark. This will happen only when the analyzer is rotated by the same angle by which plane of polarization of light is rotated by optically active solution.

The position of analyzer is again noted. The difference of the two readings will give you angle of rotation of plane of polarization.

But here the difficulty associated with this procedure that when analyzer is rotated for the total darkness, then it is attained gradually and hence it is difficult to find the exact position correctly for which complete darkness is obtained.

Laboratory Set Up of Poalrimeter

To overcome above difficulty half shade device is introduced between polariser P and glass tube T.

Shade Device

• It consist of two semi-circular plates ACB and ADC.
• One half ACB is made of glass while other half is made of quartz.
• Both the halves are joined together.
• The quartz is cut parallel to the optic axis.
• Thickness of the quartz is selected in such a way that it introduces a path difference of  ‘A/2 between ordinary and extraordinary ray.
• The thickness of the glass is selected in such a way that  it absorbs the same amount of light as is absorbed by quartz half.

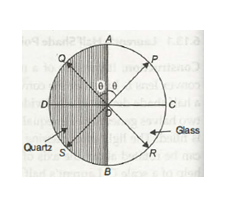
• Let us consider that the vibration of polarisation is along OP. On passing through the  glass half the vibrations remain along OP. But on passing through quartz half these vibrations will split into o  and e-components.
• The e-components are parallel to the optic axis while o-component is perpendicular to optic axis.
• The o-component travels faster in quartz and hence an emergence 0-component will be along OD instead of along OC.
• Thus components OA and OD will combine to  form a resultant vibration along OQ which makes same angle with optic axis as OP.

Now if the Principal plane of the analysing Nicol is parallel to OP then the light will pass through glass half passable. Hence glass half will be brighter than quartz half or we can say that glass half will be bright and the quartz half will be dark. Similarly if principal plane of analysing. Nicol is parallel to OQ then quartz half will be bright and glass half will be dark.

When the principal plane of analyzer is along AOB then both halves will be equally bright. On the other hand if the principal plane of analyzer is along DOC. Then both the halves will be equally dark.

Thus it is clear that if the analysing Nicol is slightly disturbed from DOC then one half becomes brighter than the other. Hence by using half shade device, one can measure angle of rotation more accurately.

Mathematically

The specific rotation is given by S=θ/lC where l is length of tube and C is concentration.
 

2.8 Optical Fibre

A cable which is used to transmit the data through fibres (threads) or plastic (glass) is known as optical fibre cable. This cable includes a pack of glass threads which transmits modulated messages over light waves.

Principle: Optical Fibre works on the principle of Total Internal Reflection.

Total internal reflection:-

When the light ray travels from denser medium to rarer medium the refracted ray bends away from the normal. When the angle of incidence is greater than the critical angle, the refracted ray again reflects into the same medium. This phenomenon is called total internal reflection. The refracted ray bends towards the normal as the ray travels from rarer medium to denser medium. The refracted ray bends away from the normal as it travels from denser medium to rarer medium.

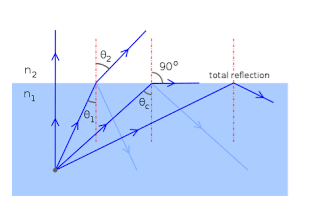
Figure – Total Internal Reflection

Characteristics of Optical Fibre

• It has a large bandwidth.
• The optical frequency of 2 x 1014 Hz can be used and hence the system has higher bandwidth.
• Thus optical fibres have greater information-carrying capacity due to greater bandwidth.
• In optical fibre system transmission losses are as low as 0.1 db/km.
• Optical fibre are of small size and light weight as compared to electrical fibre.
• Optical fibre communication is free from electromagnetic interference.
• Optical fibre do not carry high voltage and current hence they are safer than electrical cable.
• Optical Fibre are flexible and have high tensile strength. Thus can be bent or twisted easily.

Figure: Optical Fibre

Construction of Optical Fibre:

It consists of a very thin fibre of silica or glass or plastic of a high refractive index called the core. The core has a diameter of 10 um to 100 um. The core is enclosed by a cover of glass or plastic called cladding. The refractive index of the cladding is less than that of the core (which is a must condition for the working of the optical fibre). The difference between the two indicates is very small of order 10-3. The core and the cladding are enclosed in an outer protective jacket made of plastic to provide strength to the optical fibre. The refractive index can change from core to cladding abruptly (as in step-index fibre) or gradually (as in graded-index fibre).

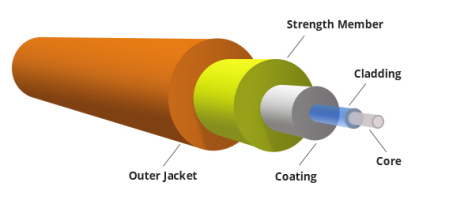
Figure Representation of Optical Fibre

Working of Optical Fibre

When a ray of light is incident on the core of the optical fibre at a small angle, it suffers refraction and strikes the core-cladding interface, As the diameter of the fibre is very small hence the angle of incidence is greater than the critical angle. Therefore, the ray suffers total internal reflection at the core-cladding interface and strikes the opposite interface. At this interface also, the angle of incidence is greater than the critical angle, so it again suffers total internal reflection. Thus, the ray of light reaches the other end of the fibre after suffering repeated total internal reflections along the length of the fibre. At the other end, the ray suffers refraction and emerges out the optical fibre.

We can see that the light travels in the core in a guided manner. Hence the communication through the optical fibre is sometimes referred as an optical waveguide.

Acceptance angle

Definition:- Acceptance angle is defined as the maximum angle of incidence at the interface of air medium and core medium for which the light ray enters into the core and travels along the interface of core and cladding.

Let n0 be the refractive indices of air

n1 be the refractive indices of core

n2 be the refractive indices of cladding

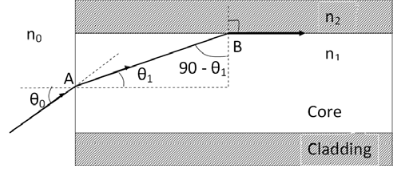
Let a light ray OA is incident on the interface of air medium and core medium with an angle of incidence θ0

The light ray refracts into the core medium with an angle of refraction θ1 and the refracted ray AB is again incidenting on the interface of core and cladding with an angle of incident (90- θ1)

If (90- θ1) is equal to the critical angle of core and cladding media then the ray travels along the interface of core and cladding along the path BC. If the angle of incident at the interface of air and core θ1< θ0 then (90- θ1) will be greater than the critical angle. Therefore,

The total internal reflection takes place.

According to Snell’s law at point A

n0 Sin θ0 = n1 Sin θ1

Sin θ0= (n1 / n0) Sin θ1  ………(1)

According to Snell’s law at point B

n1 Sin(90- θ1) = n2 Sin90  ………(2)

n1 Cosθ1 = n2   as (Sin90=1)

Cosθ1 = n2 /n1

Sinθ1 = (1-Cos2 θ1)1/2

Sinθ1= (1- (n2 /n1)2)1/2

Sinθ1= ( n12- n22 )1/2/ n1  ………(3)

We know   Sin θ0= (n1 / n0) Sin θ1 from equation (1)

Substitute the value of   Sinθ1 from equation (3)

Sinθ0= (n1 / n0) *( n12- n22 )1/2/ n1

On simplification

Sinθ0= ( n12- n22 )1/2/ n0

θ0=Sin-1 ( n12- n22 )1/2/ n0  

Acceptance Angle is θ0=Sin-1 ( n12- n22 )1/2/ n0     ………(4)

Numerical aperture

Definition: -Numerical aperture is defined as the light gathering capacity of an optical fibre and it is directly proportional to the acceptance angle. Numerically it is equal to the sin of the acceptance angle.

NA = Sin(acceptance angle)

NA = Sin {Sin-1 (( n12- n22 )1/2/ n0)}  from equation (4)

NA = (( n12- n22 )1/2/ n0)   ………(5)

If the refractive index of the air medium is unity i.e. n0=1 put in (5)

NA = ( n12- n22 )1/2    ………(6)

Fractional change in refractive index

∆= (n1- n2)/ n1

n1∆ = (n1- n2)     ………(7)

From equation (6), we have

NA = {( n1- n2 )( n1+n2 )}1/2

NA = { n1∆ (n1+n2 )}1/2   as n1∆ = (n1- n2) by Eq(7)

NA = { n1∆ 2n1}1/2    n1 ≈ n2,   so n1+n2 =2n1

NA = n1{2∆}1/2 

This gives the relation between Numerical aperture and Fractional change in refractive index.

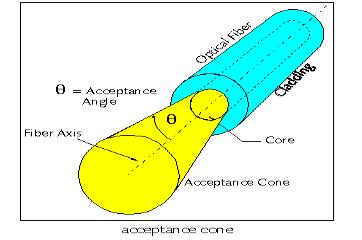
Acceptance Cone

If all possible directions of acceptance angle are considered at the same time. We get a cone corresponding to surface known as Acceptance Cone

This is the maximum angle, represented in three-dimensional view as a cone, at which an optical fibre will accept incident light. Within that cone, as defined by those angles, a light source can inject an optical signal into the fibre core and the signal will remain in the core, reflecting off of the interface between the core and cladding.

2.9 Types of Optical Fibres

The types of optical fibres depend on the refractive index, materials used, and mode of propagation of light.

      The classification based on the refractive index is as follows:

• Step Index Fibres: It consists of a core surrounded by the cladding, which has a single uniform index of refraction.
• Graded Index Fibres: The refractive index of the optical fibre decreases as the radial distance from the fibre axis increases.

      The classification based on the materials used is as follows:

• Plastic Optical Fibres: The polymethylmethacrylate is used as a core material for the transmission of the light.
• Glass Fibres: It consists of extremely fine glass fibres.

      The classification based on the mode of propagation of light is as follows:

• Single-Mode Fibres: These fibres are used for long-distance transmission of signals.
• Multimode Fibres: These fibres are used for short-distance transmission of signals.

The mode of propagation and refractive index of the core is used to form four combination types of optic fibres as follows:

• Step index-single mode fibres
• Graded index-Single mode fibres
• Step index-Multimode fibres
• Graded index-Multimode fibres

2.10 Advantages of fibre optic communication

The optical fibre communication has more advantages than convectional communication.

1. Enormous Bandwidth

2. Low Transmission Loss

3. Electric Isolation

4. Signal Security

5. Small Size and Less Weight

6. Immunity Cross Talk

1. Enormous bandwidth:- The information carrying capacity of a transmission system is directly proportional to the frequency of the transmitted signals. In the coaxial cable transmission the bandwidth range is up to around 500MHz only. Where as in optical fibre communication, the bandwidth range is large as 105 GHZ.

2. Low transmission loss:- The transmission loss is very low in optical fibres (i.e.KmdB/2.0) than compare with the conventional communication system. Hence for long distance communication fibres are preferred.

3. Electric isolation:- Since fibre optic materials are insulators, they do not exhibit earth and interface problems. Hence communicate through fibre even in electrically danger environment.

4. Signal security:- The transmitted signal through the fibre does not radiate, unlike the copper cables, a transmitted signal cannot be drawn from fibre without tampering it. Thus the optical fibre communication provides 100% signal security.

5. Small size and less weight:- The size of the fibre ranges from 10μm to 50μm, which is very small. The space occupied by the fibre cable is negligibly small compared to conventional electrical cables. Optical fibres are light in weight.

6. Immunity cross talk:- Since the optical fibres are dielectric wave guides, they are free from any electromagnetic interference and radio frequency interference. Since optical interference among different fibres is not possible, cross talk is negligible even many fibres are cabled together.

2.11 Disadvantages of Optical Fibre

The disadvantages of optical fibre include the following

• The main disadvantages of these cables are installation is expensive and difficult to fix together.
• The optical fibre cables are very difficult to merge & there will be a loss of the beam within the cable while scattering.
• Fibre optic cables are compact and highly vulnerable while fitting
• These cables are more delicate than copper wires.
• Special devices are needed to check the transmission of fibre cable.

2.12 Application of optical fibre

• Optical fibres are extensively used in communication system.
• Optical fibres are in exchange of information between different computers
• Optical fibres are used for exchange of information in cable televisions, space vehicles, submarines etc.
• Optical fibres are used in industry in security alarm systems, process control and industrial auto machine.
• Optical fibres are used in pressure sensors in biomedical and engine control. 
• Optical fibres are used in medicine, in the fabrication in endoscopy for the visualization of internal parts of the human body.
• Sensing applications of optical fibres are Displacement sensor, Fluid level detector, Liquid level sensor, Temperature/pressure sensor and Chemical sensors 
• Medical applications of optical fibres are Gastroscopy, Orthoscopic, Couldoscope, Peritonescope and Fibrescope.