UNIT 8
Isometric Projections and Isometric View or Drawing
As all the edges of the cube are equally foreshortened, the square faces are rhombuses. The rhombus ABCD (fig.) shows the isometric projection of the top square face of the cube in which BO is the true length of the diagonal.
Construct a square BQDP around BO as a diagonal. Then BP shows the true length of BA.
In triangle ABO, 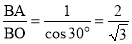
In triangle PBO, 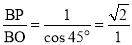
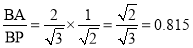
The ratio, 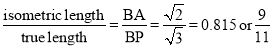 .
.
Thus, the isometric projection is reduced in the ratio  , i.e. the isometric lengths are 0.815 of the true lengths.
, i.e. the isometric lengths are 0.815 of the true lengths.
Therefore, while drawing an isometric projection, it is necessary to convert true lengths into isometric lengths for measuring and marking the sizes. This is conveniently done by constructing and making use of an isometric scale as shown below.
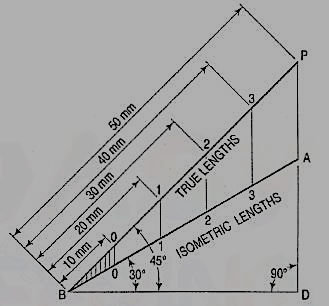
a) Draw a horizontal line BO of any length (fig.). At the end B, draw lines BA and BP, such that L OBA = 30° and L OBP = 45°. Mark divisions of true length on the line BP and from each division-point, draw verticals to BO meeting BA at respective points. The divisions thus obtained on BA give lengths on isometric scale.
(b) The same scale may also be drawn with divisions of natural scale on a horizontal line AB (fig.). At the ends A and B, draw lines AC and BC making 15° and 45° angles with AB respectively, and intersecting each other at C.
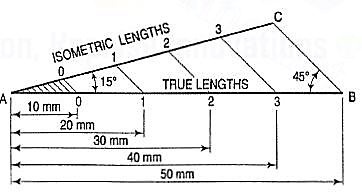
From division-points of true lengths on AB, draw lines parallel to BC and meeting AC at respective points. The divisions along AC give lengths to isometric scale.
The lines BO and AC (fig.) represent equal diagonals of a square face of the cube, but are not equally shortened in isometric projection. BO retains its true length, while AC is considerably shortened. Thus, it is seen that lines which are not parallel to the isometric axes are not reduced according to any fixed ratio. Such lines are called non-isometric lines. The measurements should, therefore, be made on isometric axes and isometric lines only. The non-isometric lines are drawn by locating positions of their ends on isometric planes and then joining them.
7.2 Conversion of orthographic views into isometric projection
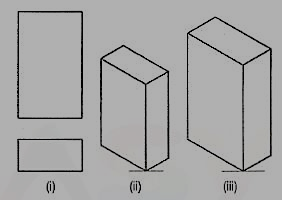
If the foreshortening of the isometric lines in an isometric projection is disregarded and instead, the true lengths are marked, the view obtained [fig.(iii)] will be exactly of the same shape but larger in proportion (about 22.5%) than that obtained using the isometric scale [fig.(ii)]. Due to the ease in construction and the advantage of measuring the dimensions directly from the drawing, it has become a general practice to use the true scale instead of the isometric scale.
To avoid confusion, the view drawn with the true scale is called isometric drawing or isometric view, while that drawn with the use of isometric scale is called isometric projection.
Referring again to fig. 2, the axes BC and CD represent the sides of a right angle in horizontal position. Each of them together with the vertical axis CG, represents the right angle in vertical position. Hence, in isometric view of any rectangular solid resting on a face on the ground, each horizontal face will have its sides parallel to the two sloping axes; each vertical face will have its vertical sides parallel to the vertical axis and the other sides parallel to one of the sloping axes.
In other words, the vertical edges are shown by vertical lines, while the horizontal edges are represented by lines, making 30° angles with the horizontal. These lines are very conveniently drawn with the T-square and a 30°-60° set-square or drafter.
Isometric Graph:
An isometric graph as shown in fig. 6 facilitates the drawing of isometric view of an object. Students are advised to make practice for drawing of isometric view using such graphs.
Isometric drawing of planes or plane figures:
Problem:
1. The front view of a square is given in fig.(i). Draw its isometric view.
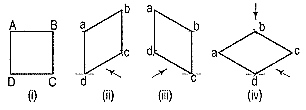
As the top view is a square, the surface of the square is horizontal. In isometric view, all the sides will be drawn inclined at 30° to the horizontal.
(i) From any point d [fig. 7(iv)], draw two lines da and dc inclined at 30° to the horizontal and making 120° angle between themselves.
(ii) Complete the rhombus abed which is the required isometric view.
2. Fig.(i) shows the front view of a circle whose surface is parallel to the V.P. Draw the isometric view of the circle.
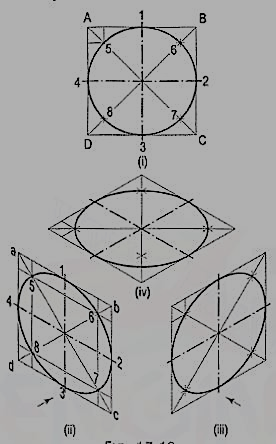
I. Method of points:
(i) Enclose the circle in a square, touching it in points 1, 2, 3 and 4. Draw the diagonals of the square cutting the circle in points 5, 6, 7 and 8.
(ii) Draw the isometric view of the square [fig. (ii) and [fig. (iii)] and on it mark the mid-points 1, 2, 3 and 4 of its sides. Obtain points 5, 6, 7 and 8 on the diagonals.
If the view given in fig. (i) is the top view of a circle whose surface is horizontal, its isometric view will be as shown in fig.(iv).
As the isometric views have been drawn with the true scale, the major axis of the ellipse is longer than the diameter of the circle.
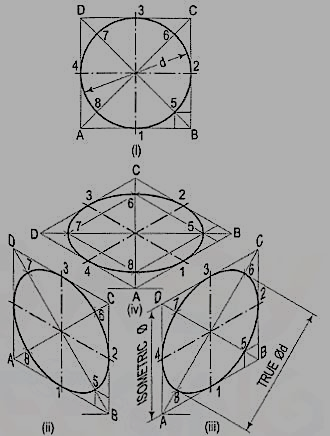
Fig. (ii), fig. (iii) and fig.(iv) show the isometric projection of the circle drawn with isometric scale. Note that when the length of the side of the rhombus is equal to the isometric diameter of the circle, the length of the major axis of the ellipse is equal to the true diameter of the circle.
II. Four-center method:
Draw the isometric view of the square [fig.(i)]. Draw perpendicular bisectors of the sides of the rhombus, intersecting each other on the longer diagonal at points p and q, and which meet at the 120°-angles b and d.
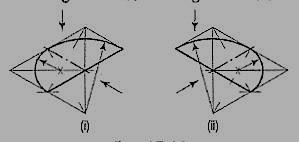
Or, draw lines joining the 120°-angles b and d with the mid-points of the opposite sides and intersecting each other on the longer diagonal at points p and q. Two of these lines will be drawn horizontal, while the other two will make 60°-angles with the horizontal. With centres b and d, draw arcs 3-4 and 1-2 respectively. With centres p and q, draw arcs 1-4 and 2-3 respectively and complete the required ellipse. Fig.(ii) shows the ellipse obtained in the rhombus drawn in the direction of the other sloping axis. Fig.(iii) shows the isometric view of the circle when its surface is horizontal.
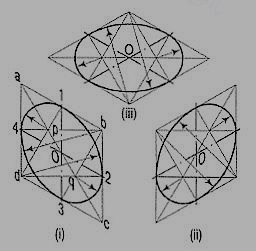
The ellipse obtained by the four-centre method is not a true ellipse and differs considerably in size and shape from the ellipse plotted through points. But owing to the ease in construction and to avoid the labour of drawing freehand neat curves, this method is generally employed.
Problem:
1. Fig.(i) shows the front view of a semi-circle whose surface is para1lel to the V.P. Draw its isometric view.
(i) Enclose the semi-circle in a rectangle. Draw the isometric view of the rectangle [fig.(ii) and [fig. (iii)].
(ii) Using the four-centre method, draw the half-ellipse in it which is the required view. The centre for the longer arc may be obtained as shown or by completing the rhombus.
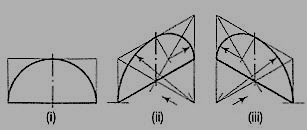
If the view given in fig. (i) is the top view of a horizontal semi-circle, its isometric view would be drawn as shown in fig.(i) and fig.(ii).
2. Fig.(i) shows the front view of a plane parallel to the V.P. Draw its isometric view.
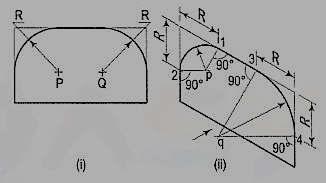
(i) The upper two corners of the plane are rounded with quarter circles. Enclose the plane in a rectangle.
(ii) Draw the isometric view of the rectangle. From the upper two corners of the parallelogram, mark points on the sides at a distance equal to R, the radius of the arcs. At these points erect perpendiculars to the respective sides to intersect each other at points p and q. With p and q as centres, and radii p1 and q3, draw the arcs and complete the required view.
7.3 Isometric view or drawing of simple objects
We have seen that the isometric view of a cube is determined from its orthographic view in a position. The three edges of the solid right-angle of the cube are shown by lines parallel to the three isometric axes. A square prism or a rectangular prism also has solid right-angles. Hence, lines for its edges are also drawn parallel to the three isometric axes.
While drawing the isometric view of any solid, the following important points should be carefully noted:
(i) The isometric view should be drawn according to the given views and in such a way that maximum possible details are visible.
(ii) At every point for the corner of a solid, at least three lines for the edges must converge. Of these, at least two must be for visible edges. Lines for the hidden edges need not be shown, but it is advisable to check up every corner so that no line for a visible edge is left out.
(iii) Two lines (for visible edges) will never cross each other.
Problem:
1. Draw the isometric view of a square prism, side of the base 20 mm long and the axis 40 mm long, when its axis is (i) vertical and (ii) horizontal.
(i) When the axis is vertical, the ends of the prism will be horizontal. Draw the isometric view (the rhombus 1-2-3-4) of the top end [fig. 14 (i)]. Its sides will make 30°-angles with the horizontal. The length of the prism will be drawn in the third direction, i.e. vertical. Hence, from the corners of the rhombus, draw vertical lines 1-5, 2-6 and 3-7 of length equal to the length of the axis. The line 4-8 should not be drawn, as that edge will not be visible. Draw lines 5-6 and 6-7, thus completing the required isometric view. Lines 7-8 and 8-5 also should not be drawn. Beginning may also be made by drawing lines from the point 6 on the horizontal line and then proceeding upwards.
(ii) When the axis is horizontal, the ends will be vertical. The ends can be drawn in two ways as shown in fig.(ii) and fig.(iii). In each case, the length is shown in the direction of the third isometric axis.

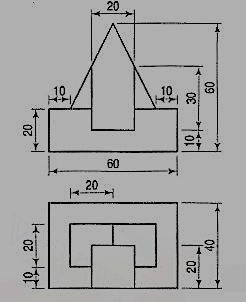
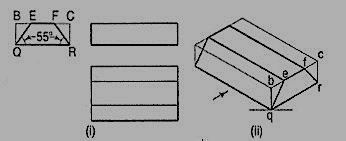
2. Three views of a block are given in fig.Draw its isometric view.
The block is in the form of a rectangular prism. Its shortest edges are vertical. Lines for these edges will be drawn vertical. Lines for all other edges which are horizontal, will be drawn inclined at 30° to the horizontal in direction of the two sloping axes as shown in fig. 15 (ii).
Methods of drawing non-isometric lines.
When an object contains inclined edges which in the isometric view would be shown by non-isometric lines, the view may be drawn by using any one of the following methods:
(i) box method or
(ii) co-ordinate or offset method.
(i) Box method: This method is used when the non-isometric lines or their ends lie in isometric planes. The object is assumed to be enclosed in a rectangular box. Initially, the box is drawn in isometric. The ends of the lines for the inclined edges are then located by measuring on or from the outlines of the box.
Problem:
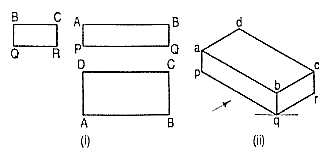
1. Three views of a block are given in fig. (i). Draw its isometric view.
(i) Enclose the block in a rectangular box.
(ii) Draw the isometric view of the box [fig. 16 (ii)].
(iii) Mark points e and f on the line be such that be = BE and fc = FC.
(iv) Complete the required view as shown.
2. Draw the isometric view of the frustum of the hexagonal pyramid shown in fig. (i).
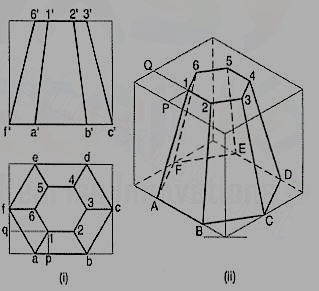
(i) Enclose the front view and the top view in rectangles.
(ii) Draw the isometric view of the rectangular box [fig. 17 (ii)]. Locate the six points of the base of the frustum on the sides of the bottom of the box. The upper six points on the top surface of the box are located by drawing isometric lines, e.g. P1 and Q1 intersecting at a point 1.
(iii) Join the corners and complete the isometric view as shown.
2. Co- ordinate or Offset method:
This method is adopted for objects in which neither non-isometric lines nor their ends lie in isometric planes.
Perpendiculars are dropped from each end of the edge to a horizontal or a vertical reference plane. The points at which the perpendiculars meet the plane, are located by drawing co-ordinates or offsets to the edges of the plane.
Problem:
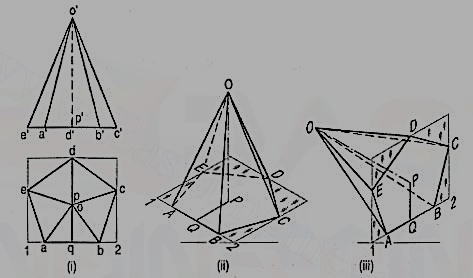
1. Draw the isometric view of the pentagonal pyramid, the projections of which are given in fig (i).
(i) Enclose the base (in the top view) in an oblong.
(ii) Draw an offset oq (i.e. pq) on the line ab.
(iii) Draw the isometric view of the oblong and locate the corners of the base in it [fig. (ii)].
(iv) Mark a point Q on the line AB such that AQ = aq. From Q, draw a line QP equal to qo and parallel to 2C. At P, draw a vertical OP equal to o'p'.
(v) Join O with the corners of the base, thus completing the isometric view of the pyramid.
Fig. (iii) shows the isometric view of the same pyramid with its axis in horizontal position.
Isometric drawing of Cylinders:
Problem:
1. Draw the isometric view of the cylinder shown in fig.(i).
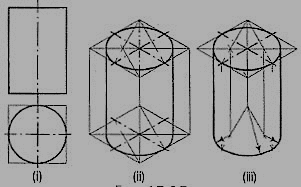
The axis of the cylinder is vertical; hence its ends are horizontal. Enclose the cylinder in a square prism.
Method I:
Draw the isometric view of the prism [fig.(ii)]. In the two rhombuses, draw the ellipses by the four-centre method. Draw two common tangents to the two ellipses. Erase the inner half of the lower ellipse and complete the required view.
Method II:
Draw the rhombus for the upper end of the prism [fig.(iii)] and in it, draw the ellipse by the four-centre method. From the centres for the arcs, draw vertical lines of length equal to the length of the axis, thus determining the centres for the lower ellipse. Draw the arcs for the half ellipse. Draw common tangents, thus completing the required view.
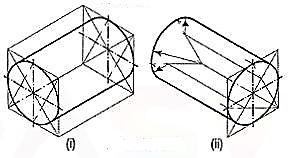
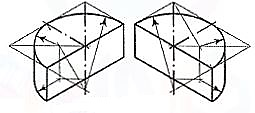
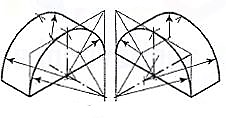
When the axis of the cylinder is horizontal, its isometric view is drawn by method I as shown in fig.(i).
Fig. (ii) shows the view drawn by method II, but the axis is shown sloping in the other direction.
Fig. 21 and fig. 22 respectively show the isometric views (drawn by method II) of a half-cylindrical disc with its axis in vertical and horizontal positions.
Isometric drawing of cones:
Problem:
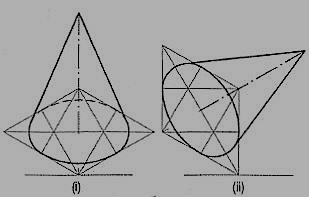
1. Draw the isometric view of a cone, base 40 mm diameter and axis 55 mm long (i) when its axis is vertical and (ii) when its axis is horizontal.
(i) Draw the ellipse for the base [fig. (i)]. Determine the position of the apex by the offset method.
(ii) Draw tangents to the ellipse from the apex. Erase the part of the ellipse between the tangents and complete the view as shown.
(iii) See fig. (ii) which is self-explanatory.
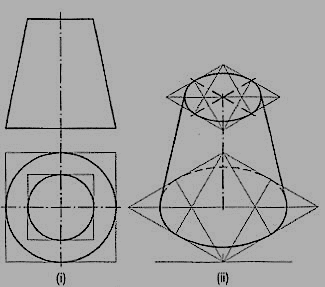
2. Draw the isometric view of the frustum of a cone shown in fig.(i).
(i) Draw the ellipse for the base [fig.(ii)]. Draw the axis.
(ii) Around the top end of the axis, draw the ellipse for the top.
(iii) Draw common tangents, erase the unwanted part of the ellipse and complete the view as shown.
Isometric drawing of Sphere
The orthographic view of a sphere seen from any direction is a circle of diameter equal to the diameter of the sphere. Hence, the isometric projection of a sphere is also a circle of the same diameter as explained below.
The front view and the top view of a sphere resting on the ground are shown in fig. 25 (i). C is its centre, D is the diameter and P is the point of its contact with the ground.

Assume a vertical section through the centre of the sphere. Its shape will be a circle of diameter D. The isometric projection of this circle is shown in fig. 25 (ii) by ellipses 1 and 2, drawn in two different vertical positions around the same centre C. The length of the major axis in each case is equal to D. The distance of the point P from the centre C is equal to the isometric radius of the sphere.
Again, assume a horizontal section through the centre of the sphere. The isometric projection of this circle is shown by the ellipse 3, drawn in a horizontal position around the same centre C. In this case also, the distance of the outermost points on the ellipse from the centre C is equal to O.5D.
Thus, in an isometric projection, the distances of all the points on the surface of a sphere from its centre, are equal to the radius of the sphere.
Hence, the isometric projection of a sphere is a circle whose diameter is equal to the true diameter of the sphere.
Also, the distance of the centre of the sphere from its point of contact with the ground is equal to the isometric radius of the sphere, viz. CP.
It is, therefore, of the utmost importance to note that, isometric scale must invariably be used, while drawing isometric projections of solids in conjunction with spheres or having spherical parts.
Problem:
Draw the isometric projection of a sphere resting centrally on the top of a square prism, the front view of which is shown in fig.(i).

(i) Draw the isometric projection (using isometric scale) of the square prism and locate the centre P of its top surface [fig.(ii)].
(ii) Draw a vertical at P and mark a point C on it, such that PC = the isometric radius of the sphere.
(iii) With C as centre and radius equal to the radius of the sphere, draw a circle which will be the isometric projection of the sphere.
Problems:
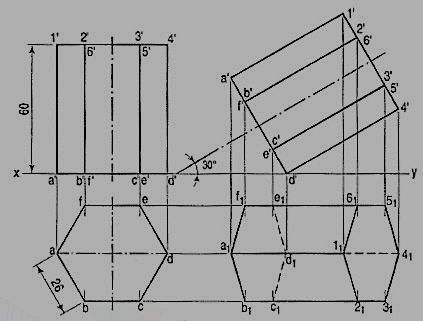
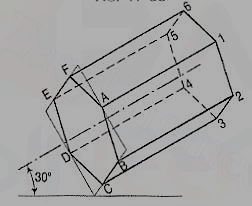
1. A hexagonal prism having the side of base 26 mm and the height of 60 mm is resting on one of the comer of the base and its axis is inclined to 30° to the H.P. Draw its projections and prepare the isometric view of the prism in the above stated condition.
(i) Draw the projections of the prism as shown in figure.
(ii) Construct the isometric view as shown in fig.
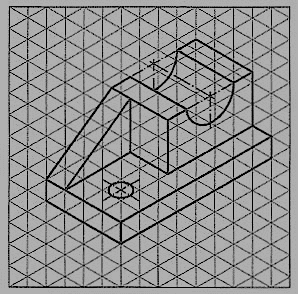
2. The orthographic projections of the object is shown in fig. Draw the isometric view of the object.