UNIT-2
QUANTUM MECHANICS-SCHRODINGER EQUATION AND ITS APPLICATIONS
As we know in the Photoelectric Effect, the Compton Effect, and the pair production effect—radiation exhibits particle-like characteristics in addition to its wave nature. In 1923 de Broglie took things even further by suggesting that this wave–particle duality is not restricted to radiation, but must be universal.
In 1923, the French physicist Louis Victor de Broglie (1892-1987) put forward the bold hypothesis that moving particles of matter should display wave-like properties under suitable conditions.
All material particles should also display dual wave–particle behaviour. That is, the wave–particle duality present in light must also occur in matter.
So, starting from the momentum of a photon p = hν/c = h/λ.
We can generalize this relation to any material particle with nonzero rest mass. Each material particle of momentum 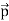 behaves as a group of waves (matter waves) whose wavelength λand wave vector
behaves as a group of waves (matter waves) whose wavelength λand wave vector 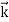 are governed by the speed and mass of the particle. De Broglie proposed that the wave length λ associated with a particle of momentum p is given as where m is the mass of the particle and v its speed.
are governed by the speed and mass of the particle. De Broglie proposed that the wave length λ associated with a particle of momentum p is given as where m is the mass of the particle and v its speed.
λ =  =
= …….(1)
…….(1)  =
=  …….(2)
…….(2)
Where ℏ = h/2π. The expression known as the deBroglie relation connects the momentum of a particle with the wavelength and wave vector of the wave corresponding to this particle. The wavelength λ of the matter wave is called de Broglie wavelength. The dual aspect of matter is evident in the de Broglie relation.
λ is the attribute of a wave while on the right hand side the momentum p is a typical attribute of a particle. Planck’s constant h relates the two attributes. Equation (1) for a material particle is basically a hypothesis whose validity can be tested only by experiment.
However, it is interesting to see that it is satisfied also by a photon. For a photon, as we have seen, p = hν/c.
Therefore
 =
=  = λ
= λ
Example: What is the frequency of a photon with energy of 4.5 eV?
Solution:
E = (4.5 eV) x (1.60 x 10-19 J/eV) = 7.2 x 10-19 J
E = hf
h = 6.63 x 10-34 J x s
f = E / h = (7.2 x 10-19 J) / (6.63 x 10-34 J x s)
f = 1.1 x 1015 Hz
HEISENBERG’S UNCERTAINTY PRINCIPLE
According to classical physics, given the initial conditions and the forces acting on a system, the future behaviour (unique path) of this physical system can be determined exactly. That is, if the initial coordinates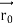 , velocity
, velocity 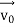 , and all the forces acting on the particle are known, the position
, and all the forces acting on the particle are known, the position  , and velocity
, and velocity 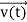 are uniquely determined by means of Newton’s second law. So by Classical physics it can be easily derived.
are uniquely determined by means of Newton’s second law. So by Classical physics it can be easily derived.
Does this hold for the microphysical world?
Since a particle is represented within the context of quantum mechanics by means of a wave function corresponding to the particle’s wave, and since wave functions cannot be localized, then a microscopic particle is somewhat spread over space and, unlike classical particles, cannot be localized in space. In addition, we have seen in the double-slit experiment that it is impossible to determine the slit that the electron went through without disturbing it. The classical concepts of exact position, exact momentum, and unique path of a particle therefore make no sense at the microscopic scale. This is the essence of Heisenberg’s uncertainty principle.
In its original form, Heisenberg’s uncertainty principle states that: If the x-component of the momentum of a particle is measured with an uncertainty ∆px, then its x-position cannot, at the same time, be measured more accurately than ∆x =ℏ/(2∆px). The three-dimensional form of the uncertainty relations for position and momentum can be written as follows:
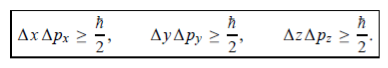
This principle indicates that, although it is possible to measure the momentum or position of a particle accurately, it is not possible to measure these two observables simultaneously to an arbitrary accuracy. That is, we cannot localize a microscopic particle without giving to it a rather large momentum.
We cannot measure the position without disturbing it; there is no way to carry out such a measurement passively as it is bound to change the momentum.
To understand this, consider measuring the position of a macroscopic object (you can consider a car) and the position of a microscopic system (you can consider an electron in an atom). On the one hand, to locate the position of a macroscopic object, you need simply to observe it; the light that strikes it and gets reflected to the detector (your eyes or a measuring device) can in no measurable way affect the motion of the object.
On the other hand, to measure the position of an electron in an atom, you must use radiation of very short wavelength (the size of the atom). The energy of this radiation is high enough to change tremendously the momentum of the electron; the mere observation of the electron affects its motion so much that it can knock it entirely out of its orbit.
It is therefore impossible to determine the position and the momentum simultaneously to arbitrary accuracy. If a particle were localized, its wave function would become zero everywhere else and its wave would then have a very short wavelength. According to de Broglie’s relation p =ℏ /λ,
Time Energy Uncertainty Relation
The momentum of this particle will be rather high. Formally, this means that if a particle is accurately localized (i.e., ∆x  0), there will be total uncertainty about its momentum (i.e., ∆px
0), there will be total uncertainty about its momentum (i.e., ∆px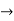 ∞).
∞).
Since all quantum phenomena are described by waves, we have no choice but to accept limits on our ability to measure simultaneously any two complementary variables.
Heisenberg’s uncertainty principle can be generalized to any pair of complementary, or canonically conjugate, dynamical variables: it is impossible to devise an experiment that can measure simultaneously two complementary variables to arbitrary accuracy. If this were ever achieved, the theory of quantum mechanics would collapse.
Energy and time, for instance, form a pair of complementary variables. Their simultaneous measurement must obey the time–energy uncertainty relation:
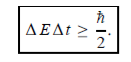
This relation states that if we make two measurements of the energy of a system and if these measurements are separated by a time interval ∆t, the measured energies will differ by an amount ∆E which can in no way be smaller than ℏ /∆t. If the time interval between the two measurements is large, the energy difference will be small. This can be attributed to the fact that, when the first measurement is carried out, the system becomes perturbed and it takes it a long time to return to its initial, unperturbed state. This expression is particularly useful in the study of decay processes, for it specifies the relationship between the mean lifetime and the energy width of the excited states.
In contrast to classical physics, quantum mechanics is a completely indeterministic theory. Asking about the position or momentum of an electron, one cannot get a definite answer; only a probabilistic answer is possible.
According to the uncertainty principle, if the position of a quantum system is well defined its momentum will be totally undefined.
1 Example: The uncertainty in the momentum of a ball travelling at 20m/s is 1×10−6 of its momentum. Calculate the uncertainty in position? Mass of the ball is given as 0.5kg.
Solution:
Given
v = 20m/s,
m = 0.5kg,
h = 6.626 × 10-34 m2 kg / s
Δp =p×1×10−6
As we know that,
P = m×v = 0.5×20 = 10kgm/s
Δp = 10×1×10−6
Δp = 10-5
Heisenberg Uncertainty principle formula is given as,
∆x∆p 
∆x 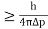
∆x 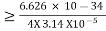
∆x =0.527 x 10-29 m
2 Example: The mass of a ball is 0.15 kg & its uncertainty in position to 10–10m. What is the value of uncertainty in its velocity?
Solution:
Given
m=0.15 kg.
h=6.6×10-34 Joule-Sec.
Δx = 10 –10 m
Δv=?
Δx.Δv ≥h/4πm
Δv≥h/4πmΔx
≥6.6×10-34/4×3.14×0.15×10–10
≥ 3.50×10–24m
APPLICATIONS OF HEISENBERG UNCERTAINTY PRINCIPLE
The Heisenberg uncertainty principle based on quantum physics explains a number of facts which could not be explained by classical physics.
- Non-existence of electrons in the nucleus
One of the applications is to prove that electron cannot exist inside the nucleus.
But to prove it, let us assume that electrons exist in the nucleus.
As the radius of the nucleus in approximately 10-14m. If electron is to exist inside the nucleus, then uncertainty in the position of the electron is given by
According to uncertainty principle
∆x ∆p =h/2π
Thus ∆p=h/2π∆x
Or ∆p=6.62 x10-34/2 x 3.14 x 10-14
Or ∆p=1.05 x 10-20 kg m/ sec
If this is p the uncertainty in the momentum of electron then the momentum of electron should be at least of this order that is p=1.05*10-20 kg m/sec.
An electron having this much high momentum must have a velocity comparable to the velocity of light. Thus, its energy should be calculated by the following relativistic formula
E = 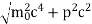
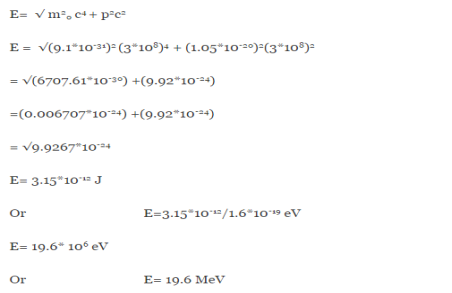
Therefore, if the electron exists in the nucleus, it should have an energy of the order of 19.6 MeV.
However, it is observed that beta-particles (electrons) ejected from the nucleus during b – decay have energies of approximately 3 Me V, which is quite different from the calculated value of 19.6 MeV.
Another reason that electron cannot exist inside the nucleus is that experimental results show that no electron or particle in the atom possess energy greater than 4 MeV.
Therefore, it is confirmed that electrons do not exist inside the nucleus.
2. Calculation of zero point energy
The minimum energy of a system at o k (zero kelvin) is called zero point energy.
Consider a particle which is confined to move under the influence of potential of dimensions a. Since the particle may be present anywhere in these dimensions, so uncertainty in position
∆x = a/2 (half the diameter).
According to uncertainty principle
∆x∆px = ℏ/2
Or
Thus ∆px=ℏ /2∆x
∆px=ℏ /2a/2 = ℏ /a
Uncertainty in momentum of particle along x-axis is
∆px=ℏ /a
Assuming the momentum of particle to be at least equal to uncertainty in it, the lowest possible value of K.E. Of particle is given by
K.E. =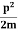 =
= 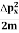 =
=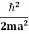
Where m is the mass of the particle.
Energy even at O K is given by the above equation. This minimum energy is called the zero-point energy.
This implies that even at zero kelvin, the particle is never at rest. If it is so, then ∆pX = 0, which is not possible. [It gives ∆x =∞]
3. Existence of proton, neutron and alpha particles within the nucleus.
We know that the rest mass of the protons and neutron is of the order of
1.67x 10-27 kg. Hence, the value of momentum 5.27 x 10-21 kg.m/sec from calculation and also the value of v come out to be 3x 105m/sec.
The corresponding value of kinetic energy of a neutron or a proton is
E=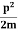 =
= 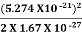 =8.33 x 10-15J =
=8.33 x 10-15J =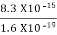 eV
eV
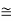 52.05 keV
52.05 keV
Since the rest mass of the a-particle is nearly four times the proton mass, therefore the alpha particle should have a minimum kinetic energy of one fourth of 52.05 keV, or about 13 keV. Since the energy carried by the protons or neutrons emitted by the nuclei are greater than 52 keV and for a-particle more than 13 keV, these particles can exist in the nuclei.
4. Size of Elementary cell in Phase space
We have studied in our previous class that state of a microsystem is defined by six variables – three are due to position and three due to momentum.
Hence, a system of N particles needs 6 N variables
If ∆ x and ∆px be the uncertainly in position and in momentum measurements then
∆ x ∆ px =  ;
;
Similarly, ∆ y ∆ py =  ,
,
And ∆ z ∆ pz =  ,
,
Multiplying these three equations, we get
∆ x ∆ y ∆ z ∆ px ∆ py ∆ pz = ( )3 in the units (J3 S3).
)3 in the units (J3 S3).
The above product is called the volume of elementary cell in phase space.
So, volume of an elementary cell in phase space 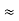 10-101 units, (for quantum statistics) being
10-101 units, (for quantum statistics) being 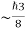 .
.
5. Accurate limit of frequency of radiation emitted by an atom
Consider the radiation emitted from an excited atom. The energy of this atom will decrease when it emits one or more photons of characteristic frequency.
The average period between excitation of the atom and the release of energy is about 10-8 seconds.
Thus, uncertainly in energy is
∆ E 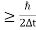
Or ∆ E 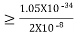 J
J
Or ∆ E 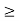 5.3 x 10-27 J
5.3 x 10-27 J
Frequency of light is uncertain by
∆ν = 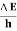 =
= 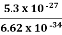 Hz
Hz  0.8 x 107 Hz
0.8 x 107 Hz
As a result, the radiation from an excited atom does not have the noted precise frequency new ν - ∆ν and ν + ∆ν.
We know that in classical physics a particle is well localized in space. We can calculate its position and velocity simultaneously.
But for quantum mechanics, a particle is not well localized in space. We cannot calculate its position and velocity simultaneously.
In Quantum mechanics it is describe that the matter wave associated with the particle. Wave functions depend on the whole space. Hence they cannot be localized.
If the wave function is made to vanish everywhere except in the neighbourhood of the particle or the neighbourhood of the classical trajectory, it can then be used to describe the dynamics of the particle. That is a particle which is localized within a certain region of space can be described by a matter wave whose amplitude is large in that region and zero outside it. This matter wave must then be localized around the region of space within which the particle is confined.
A localized wave function is called a Wave Packet or Wave Group. A wave packet or wave group therefore consists of a group of waves of slightly different wavelengths with phases and amplitudes so chosen that they interfere constructively over a small region of space and destructively elsewhere.
Wave packets are Not only useful in the explanation of isolated particles that are confined to a certain spatial region but they also play a key role in understanding the connection between quantum mechanics and classical mechanics. The wave packet concept also represents a uniting mathematical tool that describes particle-like behaviour and also its wave-like behaviour.
Phase and group velocity are two important and related concepts in wave mechanics. They arise in quantum mechanics in the time development of the state function for the continuous case, i.e. wave packets.
According to the theory of relativity particle velocity (v) is always less than the speed of light c. But according to the De-Broglie wave velocity must be greater than c. This is an unexpected result. According to this the de-Broglie wave associated with the particle would travel faster than the particle itself thus leaving the particle far behind.
The difficulty was recovered by Schrodinger. He proposed that a material particle in motion is equivalent to a wave packet rather than a single wave. Wave packet comprises of a group of waves, each with slightly different velocity and wavelength.
Such a wave packet moves with its own velocity vg called the group velocity.
The individual wave forming the wave packet possesses an average velocity vp called the phase velocity.
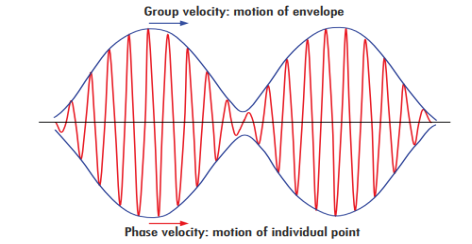
Figure 1
It can be shown that the velocity of the material particle v is the same as group velocity
Let us assume two wave trains have same amplitude but different frequency and phase velocities
u (x, t) =A sin(ωt - kx) …….(1)
u’(x, t) =A sin[(ω+∆ω)t-(k+∆k)x] …….(2)
Where ω and (ω+Δω) are angular frequencies and k and (k +Δk) are propagation constants
The superposition of two waves is of the form
ψ(x,t) = u +u’ = A sin(ωt - kx)sin[(ω+∆ω)t - (k+∆k)x] …….(3)
As Δω and Δk are small therefore (ω+Δω) 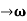 and (k + Δk)
and (k + Δk) 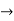 k, equation (3) reduces to
k, equation (3) reduces to
ψ(x,t) = 2Acos[  t -
t -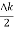 x] sin(ωt - kx) .…….(4)
x] sin(ωt - kx) .…….(4)
This equation represents a vibration of amplitude
2Acos[ 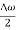 t -
t -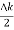 x] …….(5)
x] …….(5)
The phase of the resultant wave moves with the velocity known as phase velocity
vp=  ……..(6)
……..(6)
And the amplitude moves with the velocity known as group velocity
vg =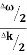 =
= 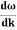
vg =  ….….(7)
….….(7)
In this experiment, Davisson and Germer scattered a 54 eV mono energetic beam of electrons from a nickel (Ni) crystal. The electron source and detector were symmetrically located with respect to the crystal’s normal, as shown in figure this is similar to the Bragg setup for X-ray diffraction by a grating. What Davisson and Germer found was that, although the electrons are scattered in all directions from the crystal, the intensity was a minimum at θ =35°
WAVE ASPECT OF PARTICLES
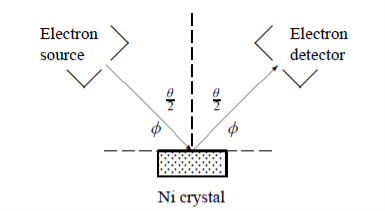
Figure 2
Davisson–Germer experiment: electrons strike the crystal’s surface at an angle ϕ
The detector, symmetrically located from the electron source, measures the number of electrons scattered at an angle θ, where θis the angle between the incident and scattered electron beams.
It is maximum at θ = 50°; that is, the bulk of the electrons scatter only in well-specified directions. They showed that the pattern continued even when the intensity of the beam was so low that the incident electrons were sent one at a time. This can only result from a constructive interference of the scattered electrons. The reflected electrons formed diffraction patterns that were identical
With Bragg’s X-ray diffraction by a grating instead of the diffuse distribution pattern.
In fact, the intensity maximum of the scattered electrons in the Davisson–Germer experiment corresponds to the first maximum (n =1) of the Bragg formula,
nλ=2d sinϕ
Where d is the spacing between the Bragg planes
ϕis the angle between the incident ray and the crystal’s reflecting planes
θis the angle between the incident and scattered beams
d is given in terms of the separation D between successive atomic layers in the crystal by d =D sin θ
For an Ni crystal, we have d =0.091 nm, since D =0.215 nm. Since only one maximum is seen at θ=50°for a mono-energetic beam of electrons of kinetic energy 54 eV, and since 2ϕ+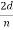 =πand hence sinϕ=cos(θ /2) from figure.
=πand hence sinϕ=cos(θ /2) from figure.
We can obtain the wavelength associated with the scattered electrons:
λ= 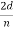 sinϕ=
sinϕ= 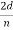 cos
cos  θ=
θ= 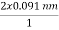 cos 25° = 0.165 nm
cos 25° = 0.165 nm
Now using results from de Broglie’s relation we will calculate the numerical value of λ. Since the kinetic energy of the electrons is K =54 eV, and the momentum is p =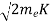 with mec2=0.511 MeV.
with mec2=0.511 MeV.
Where mec2 is the rest mass energy of the electron and hc 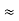 197.33 eV nm, we can show that the de Broglie wavelength is
197.33 eV nm, we can show that the de Broglie wavelength is
λ=  =
=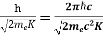 = 0.167 nm
= 0.167 nm
This is in excellent agreement with the experimental value.
We have seen that the scattered electrons in the Davisson–Germer experiment produced interference fringes that were identical to those of Bragg’s X-ray diffraction. Since the Bragg formula provided an accurate prediction of the electrons’ interference fringes, the motion of an electron of momentum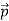 must be described by means of a plane wave
must be described by means of a plane wave
ψ(r, t) =Aei(k·r−ωt) = Aei(p·r−Et)/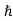
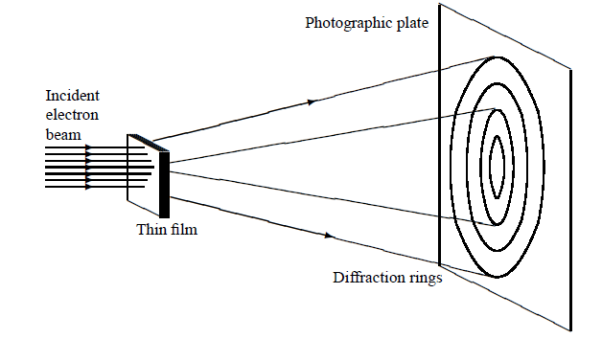
Figure 3
- The state of a quantum mechanical system is completely specified by a function (r,t) that depends on the coordinates of the particle(s) and on time. This function, called the wave function or state function, has the important property that *(r,t) (r,t)d
 is the probability that the particle lies in the volume element d
is the probability that the particle lies in the volume element d located at r at time t
located at r at time t
The wavefunction must satisfy certain mathematical conditions because of this probabilistic interpretation. For the case of a single particle, the probability of finding it somewhere is 1, so that we have the normalization condition

It is customary to also normalize many-particle wavefunctions to 1. The wavefunction must also be single-valued, continuous, and finite.
2. To every observable in classical mechanics, there corresponds a linear, Hermitian operator in quantum mechanics. For example, in coordinate space, the momentum operator 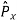 corresponding to momentum px in the
corresponding to momentum px in the 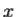 direction for a single particle is -iℏ
direction for a single particle is -iℏ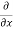 .
.
3. The average value of the observable corresponding to operator  is given by
is given by
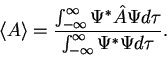
4. The wave function evolves in time according to the time-dependent Schrödinger equation
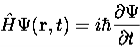
5. In any measurement of the observable associated with operator 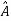 , the only values that will ever be observed are the eigenvalues a which satisfy
, the only values that will ever be observed are the eigenvalues a which satisfy 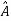 = a. Although measurements must always yield an eigen value, the state does not originally have to be in an eigen state of
= a. Although measurements must always yield an eigen value, the state does not originally have to be in an eigen state of 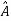 . An arbitrary state can be expanded in the complete set of eigenvectors of
. An arbitrary state can be expanded in the complete set of eigenvectors of 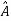 (
(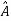 = a) as, =
= a) as, = 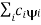 where the sum can run to infinity in principle. The probability of observing eigen value ai is given by ci*ci.
where the sum can run to infinity in principle. The probability of observing eigen value ai is given by ci*ci.
6. The total wave function must be anti symmetric with respect to the interchange of all coordinates of one fermion with those of another. Electronic spin must be included in this set of coordinates. The Pauli exclusion principle is a direct result of this antisymmetry principle.
The wave function, at a particular time, contains all the information that anybody at that time can have about the particle. But the wave function itself has no physical interpretation. It is not measurable. However, the square of the absolute value of the wave function has a physical interpretation. We interpret |ψ(x,t)|2 as a probability density, a probability per unit length of finding the particle at a time t at position x.
The wave function ψ associated with a moving particle is not an observable quantity and does not have any direct physical meaning. It is a complex quantity. The complex wave function can be represented as
ψ(x, y, z, t) = a + ib
And its complex conjugate as
ψ*(x, y, z, t) = a – ib.
The product of wave function and its complex conjugate is
ψ(x, y, z, t)ψ*(x, y, z, t) = (a + ib) (a – ib) = a2 + b2
a2 + b2 is a real quantity.
However, this can represent the probability density of locating the particle at a place in a given instant of time.
The positive square root of ψ(x, y, z, t) ψ*(x, y, z, t) is represented as |ψ(x, y, z, t)|, called the modulus of ψ. The quantity |ψ(x, y, z, t)|2 is called the probability. This interpretation is possible because the product of a complex number with its complex conjugate is a real, non-negative number.
We should be able to find the particle somewhere, we should only find it at one place at a particular instant, and the total probability of finding it anywhere should be one.
For the probability interpretation to make sense, the wave function must satisfy certain conditions.
- The wave function must be single valued at each point.
- The probability of finding the particle at time t in an interval ∆x must be some number between 0 and 1.
- ψ must be finite everywhere.
- ψ must be continuous everywhere and
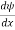 must also be continuous everywhere except where V(x) is infinite.
must also be continuous everywhere except where V(x) is infinite. - ψ (x) must vanish ψ
 0 as x
0 as x .
. - The wave function should satisfy the normalization condition. Normalization condition of a wave function ψ is mathematical statement of existence of the particle somewhere. So that if we sum up all possible values ∑|ψ(xi,t)|2∆xi we must obtain 1. The total probability of finding the particle anywhere must be one. Normalization condition is given as
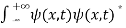 dx =1
dx =1
Only wave function with all these properties can yield physically meaningful result.
Physical significance of wave function
- The wave function ‘Ѱ’ has no physical meaning. It is a complex quantity representing the variation of a matter wave.
- The wave function Ѱ(r,t) describes the position of particle with respect to time .
- It can be considered as ‘probability amplitude’ since it is used to find the location of the particle.
- The square of the wave function gives the probability densityof the particle which is represented by the wave function itself.
- More the value of probability density, more likely to find the particle in that region.
The Schrodinger equation also known as Schrodinger’s wave equation is a partial differential equation that describes the dynamics of quantum mechanical systems by the wave function. The trajectory, the positioning, and the energy of these systems can be retrieved by solving the Schrodinger equation.
All of the information for a subatomic particle is encoded within a wave function. The wave function will satisfy and can be solved by using the Schrodinger equation. The Schrodinger equation is one of the fundamental axioms that are introduced in undergraduate physics.
Schrodinger wave equation, is the fundamental equation of quantum mechanics, same as the second law of motion is the fundamental equation of classical mechanics. This equation has been derived by Schrodinger in 1925 using the concept of wave function on the basis of de-Broglie wave and plank’s quantum theory.
Let us consider a particle of mass m and classically the energy of a particle is the sum of the kinetic and potential energies. We will assume that the potential is a function of only x.
So We have
E= K+V = mv2+V(x) =
mv2+V(x) =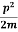 +V(x) ……….. (1)
+V(x) ……….. (1)
By de Broglie’s relation we know that all particles can be represented as waves with frequency ω and wave number k, and that E= ℏω and p= ℏk.
Using this equation (1) for the energy will become
ℏω =  + V(x) ……….. (2)
+ V(x) ……….. (2)
A wave with frequency ωand wave number k can be written as usual as
ψ(x, t) =Aei(kx−ωt) ……….. (3)
The above equation is for one dimensional and for three dimensional we can write it as
ψ(r, t) =Aei(k·r−ωt) ……….. (4)
But here we will stick to one dimension only.
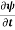 =−iωψ⇒ωψ=
=−iωψ⇒ωψ=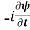 ……….. (5)
……….. (5)
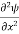 =−k2ψ⇒k2ψ = -
=−k2ψ⇒k2ψ = - 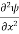 ……….. (6)
……….. (6)
If we multiply the energy equation in Eq. (2) by ψ, and using(5) and (6) , we obtain
ℏ(ωψ) = 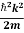 ψ+ V(x)ψ⇒
ψ+ V(x)ψ⇒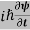 = -
= - 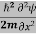 + V(x)ψ……….. (7)
+ V(x)ψ……….. (7)
This is the time-dependent Schrodinger equation.
If we put the x and t in above equation then equation (7) takes the form as given below
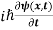 = -
= - 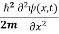 + V(x)ψ(x,t) ……….. (8)
+ V(x)ψ(x,t) ……….. (8)
In 3-D, the x dependence turns into dependence on all three coordinates (x, y, z) and the 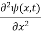 term becomes ∇2ψ.
term becomes ∇2ψ.
The term |ψ(x)|2 gives the probability of finding the particle at position x.
Let us again take it as simply a mathematical equation, then it’s just another wave equation. However We already know the solution as we used this function ψ(x, t) =Aei(kx−ωt) to produce Equations (5), (6) and (7)
But let’s pretend that we don’t know this, and let’s solve the Schrodinger equation as if we were given to us. As always, we will guess an exponential solution by looking at exponential behaviour in the time coordinate, our guess is ψ(x, t) =e−iωtf(x) putting this into Equation (7) and cancelling the e−iωt yields
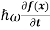 = -
= - 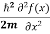 + V(x) f(x) ……….. (9)
+ V(x) f(x) ……….. (9)
We already know that E=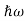 . However ψ(x, t) is general convention to also use the letter ψto denote the spatial part. So we will now replace f(x) with ψ(x)
. However ψ(x, t) is general convention to also use the letter ψto denote the spatial part. So we will now replace f(x) with ψ(x)
Eψ = - 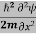 + V(x)ψ ……….. (10)
+ V(x)ψ ……….. (10)
This is called the time-independent Schrodinger equation.
2.8.1 A FREE PARTICLE
We will now apply Schrodinger's wave equation in several examples using various potential functions. These examples will demonstrate the techniques used in the solution of Schrodinger's differential equation and the results of these examples will provide an indication of the electron behaviour under these various potentials.
A Free Particle
A particle is said to be free when no external force is acting on during its motion in the given region of space, and its potential energy V is constant.
Let us consider an electro is freely moving in space in positive x direction and not acted by any force, there potential will be zero. The Schrodinger wave equation reduces to
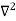 ψ +
ψ +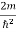 Eψ =0
Eψ =0
Substituting 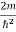 E = k2
E = k2
As the electron is moving in one direction (say x axis), then the above equation can be written as
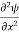 + k2ψ =0
+ k2ψ =0
The general solution of the equation (2) is of the form ψ = ψ0e-iωt
The electron is not bounded and hence there are no restrictions on k. This implies that all the values of energy are allowed. The allowed energy values form a continuum and are given by
E = 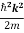
The wave vector k describes the wave properties of the electron.
It is seen from the relation that E 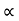 k2 Thus the plot of E as a function of k gives a parabola.
k2 Thus the plot of E as a function of k gives a parabola.
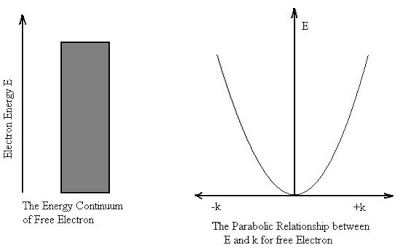
Figure 4
The momentum is well defined and in this case given by
pxψ =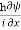
Therefore, according to uncertainty principle it is difficult to assign a position to the electron.
2.8.2 PARTICLE IN A BOX ONE DIMENSIONAL
Particle in a One Dimensional Deep Potential Well
Let us consider a particle of mass ‘m’ in a deep well restricted to move in a one dimension (say x). Let us assume that the particle is free inside the well except during collision with walls from which it rebounds elastically.
The potential function is expressed as
V= 0 for 0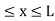 ………. (1)
………. (1)
V= 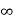 for x <0, x>L ………. (2)
for x <0, x>L ………. (2)
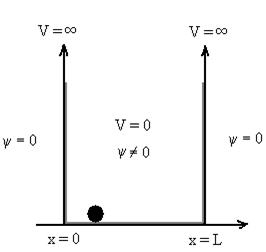
Figure 5: Particle in deep potential well
The probability of finding the particle outside the well is zero (i.e. Ѱ =0)
Inside the well, the Schrödinger wave equation is written as
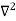 ψ +
ψ +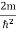 Eψ =0 …………….(2)
Eψ =0 …………….(2)
Substituting 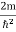 E = k2 …………….(3)
E = k2 …………….(3)
Writing the SWE for 1-D we get
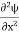 + k2ψ =0 …………….(4)
+ k2ψ =0 …………….(4)
The general equation of above equation may be expressed as
ψ = Asin (kx + ϕ) …………….(5)
Where A and ϕ are constants to be determined by boundary conditions
Condition I: We have ψ = 0 at x = 0, therefore from equation
0 = A sinϕ
As A 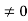 then sinϕ =0 or ϕ=0 …………….(6)
then sinϕ =0 or ϕ=0 …………….(6)
Condition II:Further ψ = 0 at x = L, and ϕ=0, therefore from equation (5)
0 = Asin kL
As A 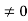 then sinkL =0 or kL=nπ
then sinkL =0 or kL=nπ
k = 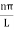 …………….(7)
…………….(7)
Where n= 1,2,3,4………
Substituting the value of k from (7) to (3)
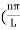 )2 =
)2 = 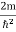 E
E
This gives energy of level
En =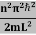 n=1,2,3,4…so on …………….(8)
n=1,2,3,4…so on …………….(8)
From equation En is the energy value (Eigen Value) of the particle in a well.
It is clear that the energy values of the particle in well are discrete not continuous.
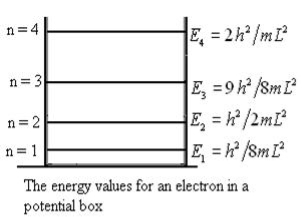
Figure 6
Using (6) and (7) equation (5) becomes, the corresponding wave functions will be
ψ =ψn= Asin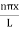 …………….(9)
…………….(9)
The probability density
|ψ(x,t)|2 =ψψ*
|ψ(x,t)|2 = A2sin2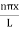 …………….(10)
…………….(10)
The probability density is zero at x = 0 and x = L. Since the particle is always within the well
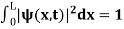 …………….(11)
…………….(11)
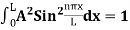

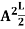 =1
=1
A = 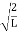
Substituting A in equation (9) we get
ψ =ψn= 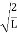 sin
sin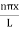 n=1,2,3,4….. …………….(12)
n=1,2,3,4….. …………….(12)
The above equation (12) is normalized wave function or Eigen function belonging to energy value En
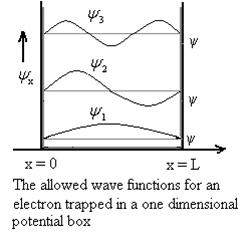
Figure 7: Wave function for Particle
2.8.3 PARTICLE IN A BOX THREE DIMENSIONAL
To illustrate the solution of the time-independent Schrödinger equation (TISE) in three dimensions, we start with the simple problem of a particle in a rigid box. This is the three-dimensional version of the problem of the particle in a one-dimensional, rigid box. In one dimension, the TISE is written as
A particle trapped in a 3D box with three lengths Lx, Ly, and Lz. A real box has three dimensions. Consider a particle which can move freely with in rectangular box of dimensions a × b × c with impenetrable walls. Since the wave function ψ should be well behaved, so, it must vanish everywhere outside the box. By the continuity requirement, the wavefunction must also vanish in the six surfaces of the box. Orienting the box so its edges are parallel to the cartesian axes, with one corner at (0,0,0), the following boundary conditions must be satisfied: ψ(x,y, z) = 0 when x = 0, x = a, y = 0, y = b, z = 0 or z = c Inside the box, where the potential energy is everywhere zero.
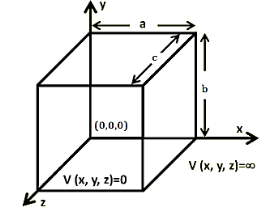
Figure 8
As with the other systems, there is no force (i.e., no potential) acting on the particles inside the box.
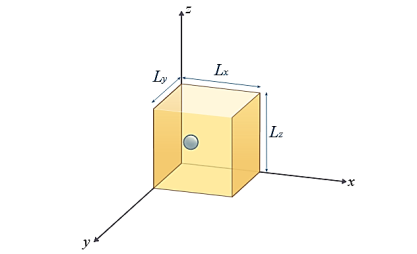
Figure 9: A particle in 3D box with three lengths Lx, Ly, and Lz
The potential for the particle inside the box
V( )=0 ………..(1)
)=0 ………..(1)
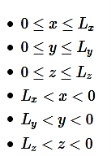
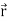 is the vector with all three components along the three axes of the 3-D box:
is the vector with all three components along the three axes of the 3-D box: 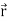 =Lx
=Lx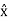 +Ly
+Ly +Lz
+Lz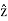 . When the potential energy is infinite, then the wave-function equals zero. When the potential energy is zero, then the wavefunction obeys the Time-Independent Schrödinger Equation
. When the potential energy is infinite, then the wave-function equals zero. When the potential energy is zero, then the wavefunction obeys the Time-Independent Schrödinger Equation
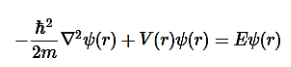 ………..(2)
………..(2)
Since here we are dealing with a 3-dimensional box, we need to modify the above equation as:
 ………..(3)
………..(3)
The easiest way in solving this partial differential equation is by having the wavefunction equal to a product of individual function for each independent variable. We are using the Separation of Variables technique here.
ψ(x,y,z)=X(x)Y(y)Z(z) ………….(4)
Now each function has its own variable:
X(x) is a function of variable x only
Y(y) is a function of variable y only
Z(z) is a function of variable z only
Now substitute Equation (4) into Equation (3) and divide it by the xyz product:
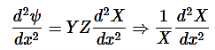 ………….(5)
………….(5)
 ………….(6)
………….(6)
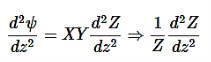 ………….(7)
………….(7)
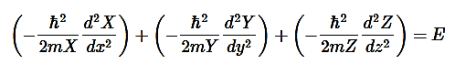 ………….(8)
………….(8)
E is an energy constant, and is the sum of x, y, and z. For this to work, each term must equal its own constant. For example,
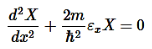 ………….(9)
………….(9)
Now separate each term in Equation 8 to equal zero:
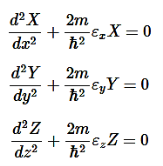
Now we can add all the energies together to get the total energy:
εx+εy+εz=E ………….(10)
These equations look similar with 1D box system.As we have now reduced the 3D box into three particle in a 1D box problems!
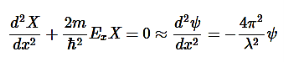 ………….(11)
………….(11)
Now the equations are very similar to a 1-D box and the boundary conditions are identical, i.e.,
n=1,2,3,..∞ ………….(12)
Use the normalization wavefunction equation for each variable:
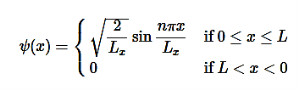 ………….(13)
………….(13)
Normalization wavefunction equation for each variable (that substitute into Equation in 4).
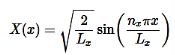 ………….(14)
………….(14)
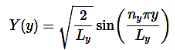 ………….(15)
………….(15)
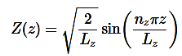 ………….(16)
………….(16)
The limits of the three quantum numbers
nx=1,2,3,...∞
ny=1,2,3,...∞
nz=1,2,3,...∞
For each constant use the de Broglie Energy equation:
εx =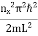 n=1,2,3,4…so on …………….(17)
n=1,2,3,4…so on …………….(17)
Do the same for variables ny and nz. Combine Equation 4 with Equations 14-16 to find the wavefunctions inside a 3D box.
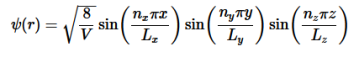 …………….(18)
…………….(18)
V = Lx Ly
Ly Lz …………….(19)
Lz …………….(19)
To find the Total Energy, add Equation (17) and Equation (10)
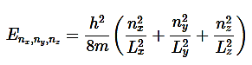 ………….(20)
………….(20)
Degeneracy in a 3D box
Quantum systems with symmetry generally exhibit degeneracy in their energy levels. This means that there can exist distinct eigen functions which share the same eigenvalue. An eigenvalue which corresponds to a unique eigen function is termed non-degenerate while one which belongs to n different eigen functions is termed n-fold degenerate.
The energy of the particle in a 3-D box (i.e., Lx=Ly=Lz) in the ground state is given by Equation 20 with nx=1, ny=1, and nz=1. This energy (E1,1,1) is hence
E1,1,1= ……………(21)
……………(21)
The ground state has only one wavefunction and no other state has this specific energy; the ground state and the energy level are said to be non-degenerate. However, in the 3-D cubical box potential the energy of a state depends upon the sum of the squares of the quantum numbers (Equation 18). The particle having a particular value of energy in the excited state may has several different stationary states or wavefunctions. If so, these states and energy eigenvalues are said to be degenerate.
For the first excited state, three combinations of the quantum numbers (nx,ny,nz)are (2,1,1),(1,2,1),(1,1,2). The sum of squares of the quantum numbers in each combination is same (equal to 6). Each wavefunction has same energy:
E2,1,1=E1,2,1=E1,1,2= …………..(22)
…………..(22)
Corresponding to these combinations three different wavefunctions and three different states are possible. Hence, the first excited state is said to be three-fold or triply degenerate. The number of independent wavefunctions for the stationary states of an energy level is called as the degree of degeneracy of the energy level. The value of energy levels with the corresponding combinations and sum of squares of the quantum numbers
n2=n2x+n2y+n2z …………………(23)
Note
2.8.4 SIMPLE HARMONIC OSCILLATOR (ONE DIMENSIONAL)
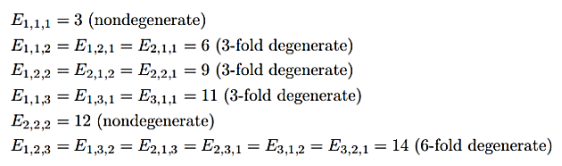
It’s time to study another example of solving the Schrodinger equation for a particular potential energy function V(x). This example is the harmonic oscillator, for which V(x) is quadratic.
For a Classical harmonic oscillator a mass m attached to spring of spring constant k. The motion of classical harmonic oscillator is governed by Hooke’s law
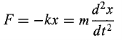 …………(1)
…………(1)
And the solution is given by
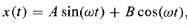 …………(2)
…………(2)
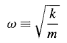 …………(3)
…………(3)
Is the (angular) frequency of oscillation. The potential energy is
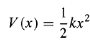 …………(4)
…………(4)
Its graph is a parabola. Of course, there'sno such thing as perfect simple harmonic oscillator-if you stretch it too far the spring is going to break, and typically Hooke's law fails long before that point is reached. But practically any potential is approximately parabolic, in the neighbourhood of a local minimum(Figure 10). Formally, if we expandV(x)in a Taylor series about the minimum:
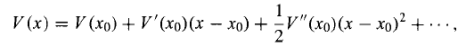
…………(5)
Subtract V (xo) [you can add a constant to V(x) with impunity, since that doesn't change the force], recognize that V'(xo) = 0 (since xois a minimum), and drop the higher-order terms [which are negligible as long as (x - xo) stays small], the potential becomes
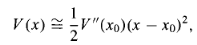 …………(6)
…………(6)
Which describes simple harmonic oscillation (about the point xo), with an effective spring constant k = V” (xo). That's why the simple harmonic oscillator is so important: Virtually any oscillatory motion is approximately simple harmonic, as long as the amplitude is small.

Figure 10: Parabolic approximation (dashed curve) to an arbitrary potential, in the neighbourhood of a local minimum.
The quantum problem is to solve the Schrodinger equation for the potential
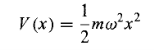 …………(7)
…………(7)
(it is customary to eliminate the spring constant in favour of the classical frequency, using Equation (3). As we have seen, it suffices to solve the time-independent Schrodinger equation:
 …………(8)
…………(8)
In the literature you will find two entirely different approaches to this problem. The first is a straightforward "brute force" solution to the differential equation, using the method of power series expansion; it has the virtue that the same strategy can be applied to many other potentials. The second is a diabolically clever algebraic technique, using so-called ladder operators also known as algebraic method. But we will solve this with the analytic method for now.
Analytic Method
The Schrodinger equation for the harmonic oscillator(Equation 8):
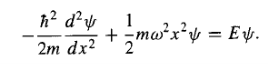
Things look a little cleaner if we introduce the dimensionless variable
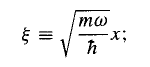 …………(9)
…………(9)
In terms of ,the Schrodinger equation reads
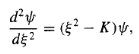 …………(10)
…………(10)
Where K is the energy, in units of (1/2) ℏω:
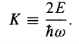 …………(11)
…………(11)
We have to solve Equation (10), and in the process obtain the "allowed" values of K(andhenceofE).
To begin with, note that at very large(which is to say, at very largex),2completelydominatesovertheconstantK,so in this regime
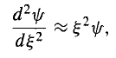 …………(12)
…………(12)
Which has the approximate solution
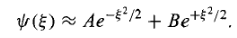 …………(13)
…………(13)
The B term is clearly not normalizable (it blows up as |x|  the physically acceptable solutions, then, have the asymptotic form
the physically acceptable solutions, then, have the asymptotic form
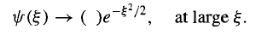 …………(14)
…………(14)
This suggests that we “peel off" the exponential part,
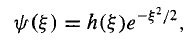 …………(15)
…………(15)
In hopes that what remains[h()]has a simpler functional form than ()itself. Differentiating Equation (15), we have
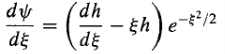
And
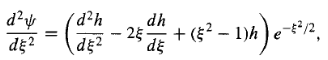
So the Schrodinger equation (Equation 10) becomes
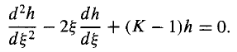 …………(16)
…………(16)
A solution to Equation (16)in the form of a power series in:
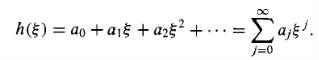 …………(17)
…………(17)
Differentiating the series term by term,
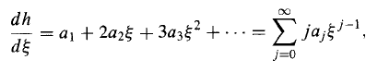
And

Putting these into Equation 16, we find
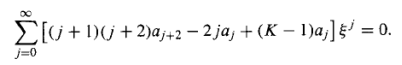 …………(18)
…………(18)
It follows (from the uniqueness of power series expansions) that the coefficient of each power of must vanish,
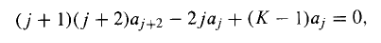
And hence that
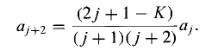 …………(19)
…………(19)
This recursion formula is entirely equivalent to the Schrodinger equation itself. Given ao it enables us (in principle) to generate a2, a4, a6,..., and given a1it generates a3, a5, a7,.... Let us write
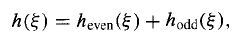 …………(20)
…………(20)
Where
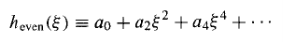
Is an even function of (since it involves only even powers), built on a0, and
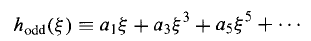
Is an odd function, built on a1. Thus Equation (19) determines h() in terms of two arbitrary constants (a0and a1) –which is just what we would expect, for a second – order differential equation.
However, not all the solutions so obtained are normalizable. For at very large j, the recursion formula becomes (approximately)
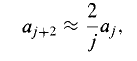
With the (approximate) solution
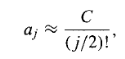
For some constant C, and this yields (at large,where the higher powers dominate)
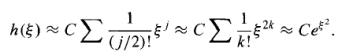
Now, if h goes like exp(2),then (remember ? - that's what we're trying to calculate) goes like exp (2/2) (Equation 15),which is precisely the asymptotic behaviour we don't want. There is only one way to wiggle out of this: For normalizable solutions the power series must terminate. There must occur some "highest"j(callitn)suchthattherecursionformulaspitsoutan+2= 0 (this willtruncateeithertheserieshevenortheserieshodd;theotheronemustbe zero from the start). For physically acceptable solutions, then, we must have
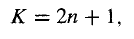
For some positive integer n, which is to say (referring to Equation 11) that the energy must be of the form
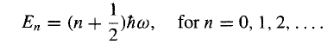 …………(21)
…………(21)
For the allowed values of K, the recursion formula reads
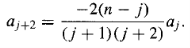 …………(22)
…………(22)
If n=0,thereis only one term in the series(we must pick a1= 0 to kill hodd, and j= 0 inEquation22 yieldsa2=0):
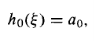
And hence
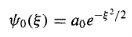
(For n = 1 we pick ao = 0,and Equation (22) with j = 1 yields a3 = 0, so
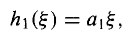
And hence
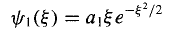
For n = 2, j = 0 yields a2 = -2ao, and j = 2 gives a4 = 0, so
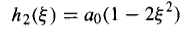
And
And so on.
In general, hn() will be a polynomial of degree n in , involving even powers only, if n is an even integer, and odd powers only, if n is an odd integer. Apart from the overall factor (a0 or a1) they are the so-called Hermite polynomials, Hn(). The first few of them are listed in Table 1. By tradition, the arbitrary multiplicative factor is chosen so that the coefficient of the highest power of is 2n. With this convention, the normalized stationary states for the harmonic oscillator are
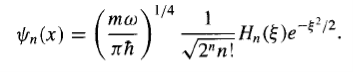 …………(23)
…………(23)
In Figure 11a;We have plotted n(x) for the first few n's.
The quantum oscillator is strikingly different from its classical counterpart-not only are the energies quantized, but the position distributions have some bizarre features. For instance, the probability of finding the particle outside the classically allowed range (that is, with x greater than the classical amplitude for the energy in question) is not zero and in all odd states the probability of finding the particle at the center of the potential well is zero, Only at relatively large n do we begin to see some resemblance to the classical case.
Table 1: The first few Hermite polynomials, Hn (x).
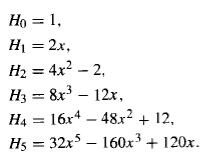
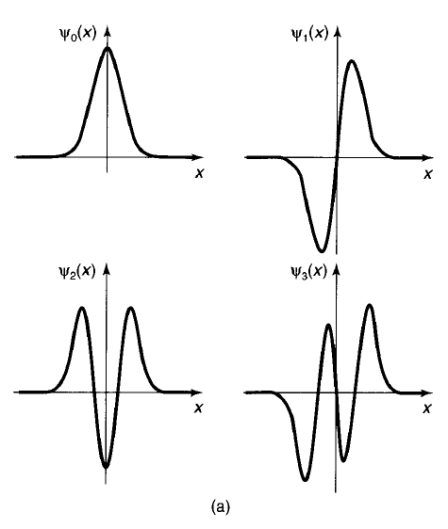
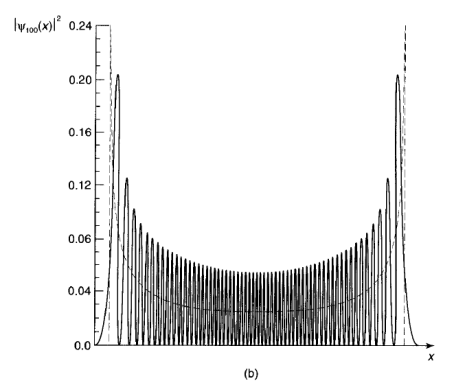
Figure 11: (a) The first four stationary states of the harmonic oscillator.
(b)Graph of |100|2,with the classical distribution(dashed curve)super imposed.
In Figure 11(b)We have superimposed the classical position distribution on the quantum one (for n = 100); if you smoothed out the bumps in the latter, the two would fit pretty well, however, in the classical case we are talking about the distribution of positions over time for one oscillator, whereas in the quantum case we are talking about the distribution over an ensemble of identically-prepared systems.