UNIT–2
Multivariable Calculus
Limits-
The function f(x,y) is said to tend to limit ‘l’ , as x →a and y→b Iff the limit is dependent on point (x,y) as x →a and y→b
We can write this as,

Example-1: evaluate the 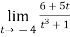
Sol. We can simply find the solution as follows,
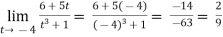
Example-2: evaluate 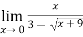
Sol.
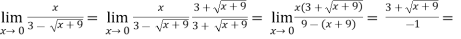 -6.
-6.
Example-3: evaluate 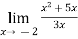
Sol.
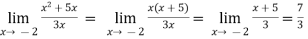
Conitinuity –
At point (a,b) , a function f(x,y) is said to be continuous if,
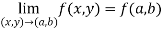
Working rule for continuity-
Step-1: f(a,b) should be well defined.
Step-2: 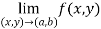 should exist.
should exist.
Step-3: 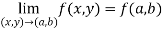
Example-1: Test the continuity of the following function-
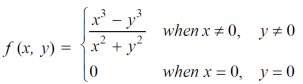
Sol. (1) the function is well defined at (0,0)
(2) check for the second step,
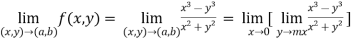
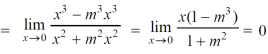
That means the limit exists at (0,0)
Now check step-3:
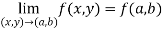
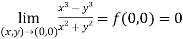
So that the function is continuous at origin.
Example-2: check for the continuity of the following function at origin,
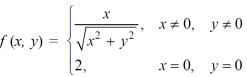
Sol. (1) Here the function is well defined at (0,0)
(2) check for second step-
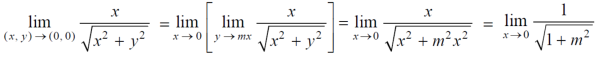
Limit f is not unique for different values of m
So that the limit does not exists.
Therefore the function is not continuous at origin.
Steps to check for existence of limit-
Step-1: find the value of f(x,y) along x →a and y→b
Step-2: find the value of f(x,y) along x →b and y→a
Note- if the values in step -1 and step-2 are same then we can say that the limits exist otherwise not.
Step-3: if a →0 and b→0 then find the limit along y =mx , if the value does not contain m then limit exist, If it contains m then the limit does not exist.
Note-1- put x = 0 and y = 0 in f , then find f1
2 - Put y = 0 and x = 0 In f then find x2
If f1 and f2 are equal then limit exist otherwise not.
3- put y = mx then find f3
If f1 = f2 ≠f3, limit does not exist.
4- put y = mx² and find f4,
If f1 = f2 = f3 ≠ f4 , limit does not exist
If f1 = f2 = f3 = f4 , limit exist.
Example-1: Evaluate 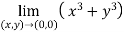
Sol . 1. 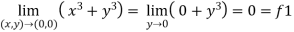
2. 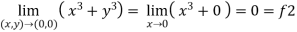
Here f1 = f2
3. Now put y = mx, we get
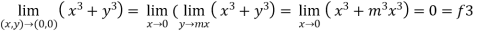
Here f1 = f2 = f3
Now put y = mx²
4. 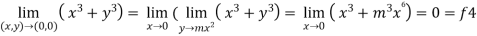
Therefore ,
F1 = f2 = f3 =f4
We can say that the limit exists with 0.
Example-2: evaluate the following-
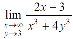
Sol. First we will calculate f1 –
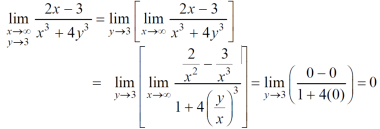
Here we see that f1 = 0
Now find f2,
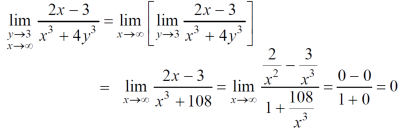
Here , f1 = f2
Therefore the limit exists with value 0.
Partial derivatives-
First order partial differentiation-
Let f(x , y) be a function of two variables. Then the partial derivative of this function with respect to x can be written as 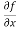 and defined as follows:
and defined as follows:
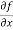 =
= 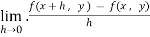
Now the partial derivative of f with respect to f can be written as 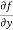 and defined as follows:
and defined as follows:
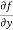 =
= 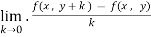
Note: a. While calculating partial derivatives treat all independent variables, other than the variable with respect to which we are differentiating , as constant.
b. We apply all differentiation rules.
Higher order partial differentiation-
Let f(x , y) be a function of two variables. Then its second-order partial derivatives, third order partial derivatives and so on are referred as higher order partial derivatives.
These are second order four partial derivatives:
(a) 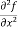 =
= 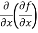
(b)  =
= 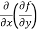
(c)  =
= 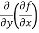
(d) 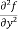 =
= 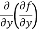
b and c are known as mixed partial derivatives.
Similarly we can find the other higher order derivatives.
Example-1: - Calculate 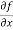 and
and 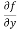 for the following function
for the following function
f(x , y) = 3x³-5y²+2xy-8x+4y-20
Sol. To calculate 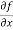 treat the variable y as a constant, then differentiate f(x,y) with respect to x by using differentiation rules,
treat the variable y as a constant, then differentiate f(x,y) with respect to x by using differentiation rules,
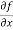 =
= 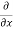 [3x³-5y²+2xy-8x+4y-20]
[3x³-5y²+2xy-8x+4y-20]
= 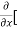 3x³] -
3x³] - 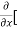 5y²] +
5y²] + 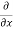 [2xy] -
[2xy] -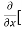 8x] +
8x] +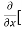 4y] -
4y] - 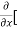 20]
20]
= 9x² - 0 + 2y – 8 + 0 – 0
= 9x² + 2y – 8
Similarly partial derivative of f(x,y) with respect to y is:
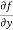 =
= 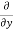 [3x³-5y²+2xy-8x+4y-20]
[3x³-5y²+2xy-8x+4y-20]
= 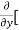 3x³] -
3x³] - 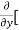 5y²] +
5y²] + 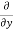 [2xy] -
[2xy] - 8x] +
8x] +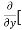 4y] -
4y] - 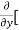 20]
20]
= 0 – 10y + 2x – 0 + 4 – 0
= 2x – 10y +4.
Example-2: Calculate  and
and 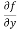 for the following function
for the following function
f( x, y) = sin(y²x + 5x – 8)
Sol. To calculate 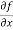 treat the variable y as a constant, then differentiate f(x,y) with respect to x by using differentiation rules,
treat the variable y as a constant, then differentiate f(x,y) with respect to x by using differentiation rules,
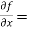
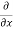 [sin(y²x + 5x – 8)]
[sin(y²x + 5x – 8)]
= cos(y²x + 5x – 8) (y²x + 5x – 8)
(y²x + 5x – 8)
= (y² + 50) cos(y²x + 5x – 8)
Similarly partial derivative of f(x,y) with respect to y is,
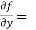
 [sin(y²x + 5x – 8)]
[sin(y²x + 5x – 8)]
= cos(y²x + 5x – 8)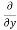 (y²x + 5x – 8)
(y²x + 5x – 8)
= 2xy cos(y²x + 5x – 8)
Example-3: Obtain all the second order partial derivative of the function:
f( x, y) = ( x³y² - xy⁵)
Sol. 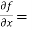 3x²y² - y⁵,
3x²y² - y⁵, 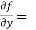 2x³y – 5xy⁴,
2x³y – 5xy⁴,
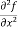 =
= 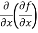 = 6xy²
= 6xy²
 =
= 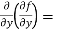 2x³ - 20xy³
2x³ - 20xy³
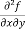 =
= 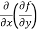 = 6x²y – 5y⁴
= 6x²y – 5y⁴
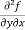 =
= 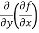 = 6x²y - 5y⁴
= 6x²y - 5y⁴
Example-4: Find
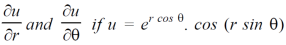
Sol. First we will differentiate partially with repsect to r,
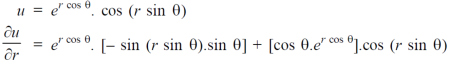

Now differentiate partially with respect to θ, we get
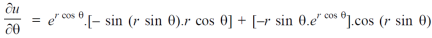
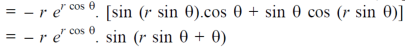
Example-5: if,
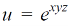
Then find.
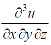
Sol-
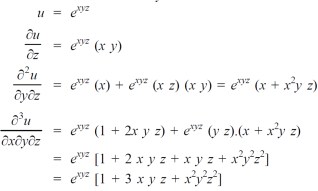
Example-6: if  , then show that-
, then show that- 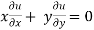
Sol. Here we have,
u = 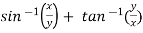 …………………..(1)
…………………..(1)
Now partially differentiate eq.(1) w.r to x and y , we get
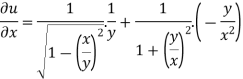
= 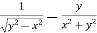
Or
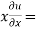
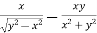 ………………..(2)
………………..(2)
And now,

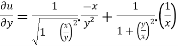
= 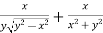
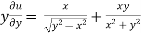 ………………….(3)
………………….(3)
Adding eq. (1) and (3) , we get
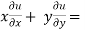
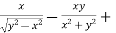
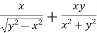 = 0
= 0
Hence proved.
When we measure the rate of change of the dependent variable owing to any change in a variable on which it depends, when none of the variable is assumed to be constant.
Let the function, u = f( x, y), such that x = g(t) , y = h(t)
ᵡ Then we can write,
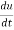 =
= 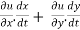
= 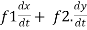
This is the total derivative of u with respect to t.
Change of variable-
If w = f (x, y) has continuous partial variables fx and fy and if x = x (t), y = y (t) are
Differentiable functions of t, then the composite function w = f (x (t), y (t)) is a
Differentiable function of t.
In this case, we get,
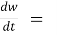 fx (x (t), y (t)) x’ (t) + fy (x(t), y (t)) y’ (t).
fx (x (t), y (t)) x’ (t) + fy (x(t), y (t)) y’ (t).
Example-:1 let q = 4x + 3y and x = t³ + t² + 1 , y = t³ - t² - t
Then find 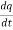 .
.
Sol. : 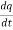 . =
. = 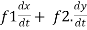
Where, f1 = 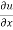 , f2 =
, f2 = 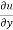
In this example f1 = 4 , f2 = 3
Also, 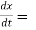 3t² + 2t ,
3t² + 2t , 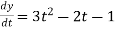
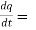 4(3t² + 2t) + 3(
4(3t² + 2t) + 3(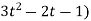
= 21t² + 2t – 3
Example-2: Find 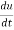 if u = x³y⁴ where x = t³ and y = t².
if u = x³y⁴ where x = t³ and y = t².
Sol. As we know that by definition, 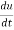 =
= 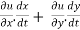
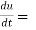 3x²y⁴3t² + 4x³y³2t = 17t¹⁶.
3x²y⁴3t² + 4x³y³2t = 17t¹⁶.
Example-3: if w = x² + y – z + sint and x + y = t, find
(a) 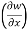 y,z
y,z
(b) 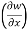 t, z
t, z
Sol. With x, y, z independent, we have
t = x + y, w = x² + y - z + sin (x + y).
Therefore,
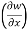 y,z = 2x + cos(x+y)
y,z = 2x + cos(x+y)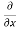 (x+y)
(x+y)
= 2x + cos (x + y)
With x, t, z independent, we have
Y = t-x, w= x² + (t-x) + sin t
Thus 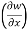 t, z = 2x - 1
t, z = 2x - 1
Example-4: If u = u( y – z , z - x , x – y) then prove that 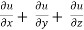 = 0
= 0
Sol. Let,
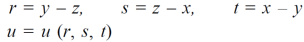
Then,
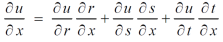
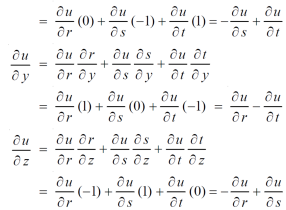
By adding all these equations we get,
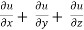 = 0 hence proved.
= 0 hence proved.
Example-5: if φ( cx – az , cy – bz) = 0 then show that ap + bq = c
Where p = 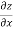 q =
q = 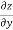
Sol. We have,
φ( cx – az , cy – bz) = 0
φ( r , s) = 0
Where,
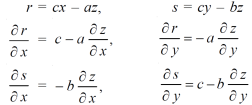
We know that,
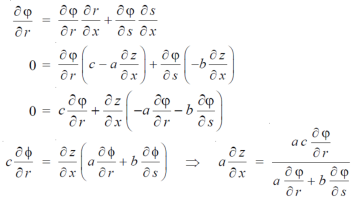
Again we do,
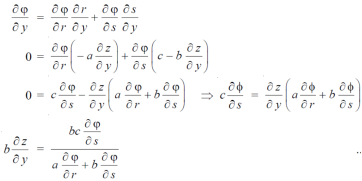
By adding the two results, we get
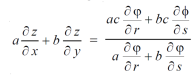

Example-6: If z is the function of x and y , and x = 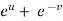 , y =
, y = 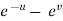 , then prove that,
, then prove that,
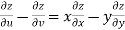
Sol. Here , it is given that, z is the function of x and y & x , y are the functions of u and v.
So that,
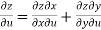 ……………….(1)
……………….(1)
And,
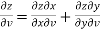 ………………..(2)
………………..(2)
Also there is,
x = 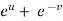 and y =
and y = 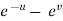 ,
,
Now,
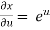 ,
, 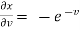 ,
, 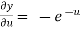 ,
, 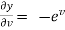
From equation(1) , we get
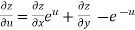 ……………….(3)
……………….(3)
And from eq. (2) , we get
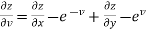 …………..(4)
…………..(4)
Subtracting eq. (4) from (3), we get
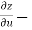
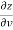 =
= 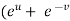 )
) 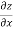 – (
– (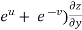
= x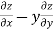
Hence proved.
Differentiability-
Let f(x) be a single valued function of the variable x, then,
f’(x) = 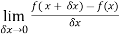
Provided that the limit exists and is dependent of the path along which 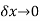
Example: suppose that the function,
f(z) = 4x + y + i( -x + 4y)
Discuss df/dz.
Sol. Here,
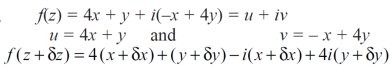
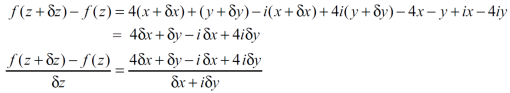
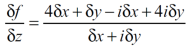
Example-2: if
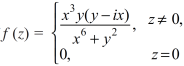
Then df/dz , z = 0.
Sol.
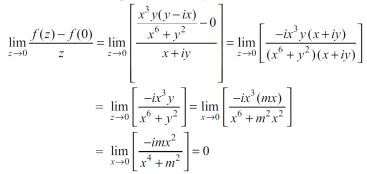
Equation of a tangent plane-
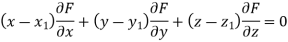
And
Equation of the normal to the plane-
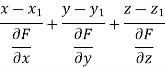
Example: Find the equation of the tangent plane and normal line to the surface 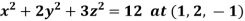
Sol.
Here,
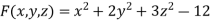
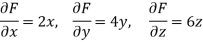
At point (1, 2, -1)-
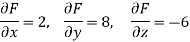
Therefore the equation of the tangent plane at (1, 2, -1) is-
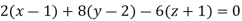
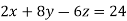
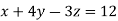
Equation of normal line is-
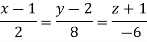
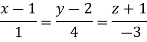
If f(x) is a single valued function defined in a region R then
Maxima is a maximum point 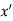 if and only if
if and only if 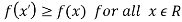
Minima is a minimum point 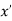 if and only if
if and only if 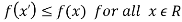
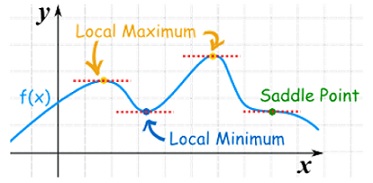


Maxima and Minima of a function of two independent variables
Let 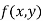 be a defined function of two independent variables.
be a defined function of two independent variables.
Then the point 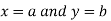 is said to be a maximum point of
is said to be a maximum point of 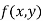 if
if
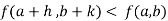
Or  =
= 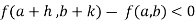
For all positive and negative values of h and k.
Similarly the point 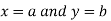 is said to be a minimum point of
is said to be a minimum point of 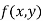 if
if
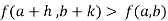
Or 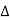 =
= 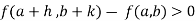
For all positive and negative values of h and k.
Saddle point:Critical points of a function of two variables are those points at which both partial derivatives of the function are zero. A critical point of a function of a single variable is either a local maximum, a local minimum, or neither. With functions of two variables there is a fourth possibility - a saddle point.
A point is a saddle point of a function of two variables if
![2 2 [ 2 ] 2
@f-= 0, @f--= 0, and @-f- @-f-- -@-f- < 0
@x @y @x2 @y2 @x @y](https://glossaread-contain.s3.ap-south-1.amazonaws.com/epub/1643282277_411049.png)
At the point.
Stationary Value
The value 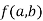 is said to be a stationary value of
is said to be a stationary value of 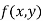 if
if
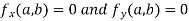
i.e. the function is a stationary at (a , b).
Rule to find the maximum and minimum values of 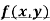
- Calculate
 .
. - Form and solve
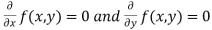 , we get the value of x and y let it be pairs of values
, we get the value of x and y let it be pairs of values 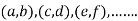
- Calculate the following values :
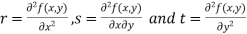
4. (a) If 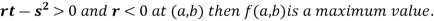
(b) If 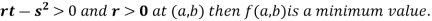
(c) If 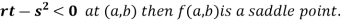
(d) If 
Example1 Find out the maxima and minima of the function
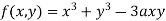
Given 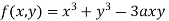 …(i)
…(i)
Partially differentiating (i) with respect to x we get
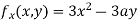 ….(ii)
….(ii)
Partially differentiating (i) with respect to y we get
 ….(iii)
….(iii)
Now, form the equations 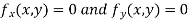
Using (ii) and (iii) we get
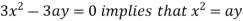
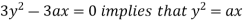
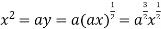 using above two equations
using above two equations
Squaring both side we get

Or 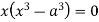
This show that 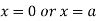
Also we get 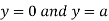
Thus we get the pair of value as 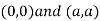
Now, we calculate
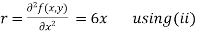
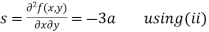
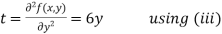
Putting above values in
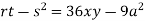
At point (0,0) we get
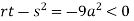
So, the point (0,0) is a saddle point.
At point 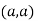 we get
we get
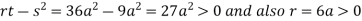
So the point 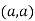 is the minimum point where
is the minimum point where 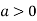
In case 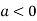
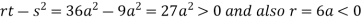
So the point  is the maximum point where
is the maximum point where 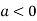
Example2 Find the maximum and minimum point of the function
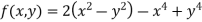
Partially differentiating given equation with respect to and x and y then equate them to zero
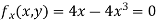
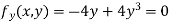
On solving above we get 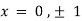
Also 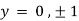
Thus we get the pair of values (0,0), ( ,0) and (0,
,0) and (0,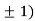
Now, we calculate
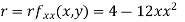
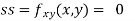
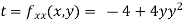
At the point (0,0)
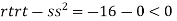
So function has saddle point at (0,0).
At the point (
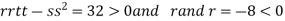
So the function has maxima at this point ( .
.
At the point (0,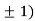
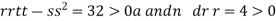
So the function has minima at this point (0,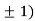 .
.
At the point (
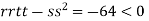
So the function has an saddle point at (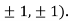
Example3 Find the maximum and minimum value of 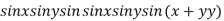
Let 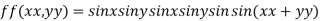
Partially differentiating given function with respect to x and y and equate it to zero
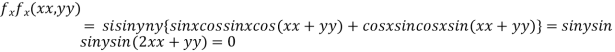 ..(i)
..(i)
 ..(ii)
..(ii)
On solving (i) and (ii) we get
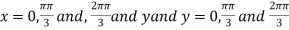
Thus pair of values are 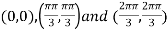
Now, we calculate
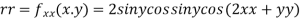
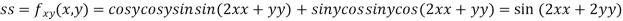
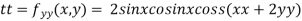
At the point (0,0)
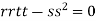
So further investigation is required
On the x axis y = 0 , f(x,0)=0
On the line y=x, 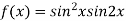
At the point 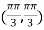
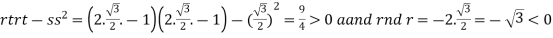
So that the given function has maximum value at 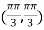
Therefore maximum value of given function
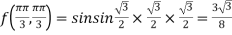
At the point 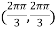
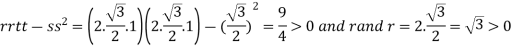
So that the given function has minimum value at 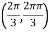
Therefore minimum value of the given function
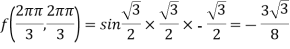
Let 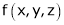 be a function of x, y, z which to be discussed for stationary value.
be a function of x, y, z which to be discussed for stationary value.
Let 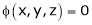 be a relation in x, y, z
be a relation in x, y, z
 for stationary values we have,
for stationary values we have,
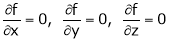
i.e.  … (1)
… (1)
Also from 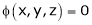 we have
we have
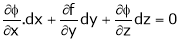 … (2)
… (2)
Let ‘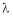 ’ be undetermined multiplier then multiplying equation (2) by
’ be undetermined multiplier then multiplying equation (2) by 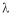 and adding in equation (1) we get,
and adding in equation (1) we get,
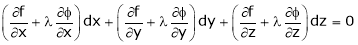
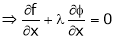 … (3)
… (3)
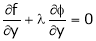 … (4)
… (4)
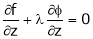 … (5)
… (5)
Solving equation (3), (4) (5) & we get values of x, y, z and 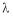 .
.
- Decompare a positive number ‘a’ in to three parts, so their product is maximum
Solution:
Let x, y, z be the three parts of ‘a’ then we get.
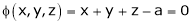 … (1)
… (1)
Here we have to maximize the product
i.e. 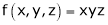
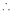 By Lagrange’s undetermined multiplier, we get,
By Lagrange’s undetermined multiplier, we get,
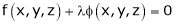
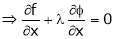 … (2)
… (2)
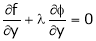 … (3)
… (3)
 … (4)
… (4)
i.e.
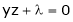 … (2)’
… (2)’
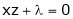 … (3)’
… (3)’
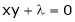 … (4)
… (4)
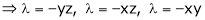
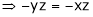

And 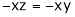
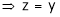
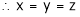
From (1)
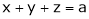
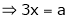
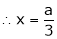
Thus 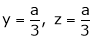 .
.
Hence their maximum product is 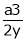 .
.
2. Find the point on plane 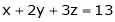 nearest to the point (1, 1, 1) using Lagrange’s method of multipliers.
nearest to the point (1, 1, 1) using Lagrange’s method of multipliers.
Solution:
Let 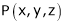 be the point on sphere
be the point on sphere 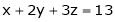 which is nearest to the point
which is nearest to the point 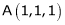 . Then shortest distance.
. Then shortest distance.
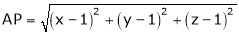
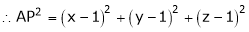
 Let
Let 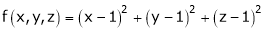
Under the condition  … (1)
… (1)
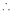 By method of Lagrange’s undetermined multipliers we have
By method of Lagrange’s undetermined multipliers we have
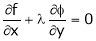
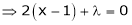 … (2)
… (2)
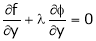
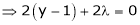 … (3)
… (3)
i.e. 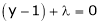 &
&
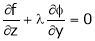
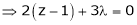 … (4)
… (4)
From (2) we get 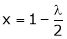
From (3) we get 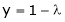
From (4) we get 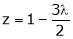
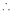 Equation (1) becomes
Equation (1) becomes
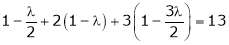
i.e. 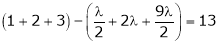


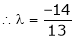
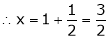
y = 2
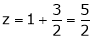
Double integral –
Before studying about multiple integrals , first let’s go through the definition of definition of definite integrals for function of single variable.
As we know, the integral
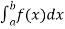
Where is belongs to the limit a ≤ x ≤ b
This integral can be written as follows-
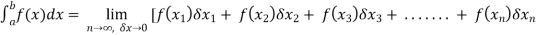
Now suppose we have a function f(x , y) of two variables x and y in two dimensional finite region R in xy-plane.
Then the double integration over region R can be evaluated by two successive integration

Evaluation of double integrals-
If A is described as 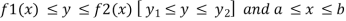
Then,
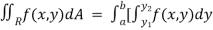 ]dx
]dx
Let do some examples to understand more about double integration-
Example-1: Evaluate 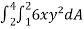 , where dA is the small area in xy-plane.
, where dA is the small area in xy-plane.
Sol. Let , I = 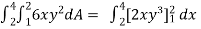
= 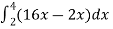
= 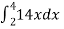
= 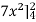
= 84 sq. Unit.
Which is the required area.
Example-2: Evaluate 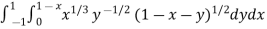
Sol. Let us suppose the integral is I,
I = 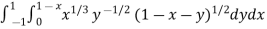
Put c = 1 – x in I, we get
I = 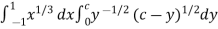
Suppose , y = ct
Then dy = c
Now we get,
I = 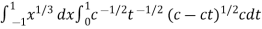
I = 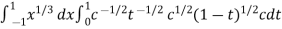
I = 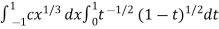
I = 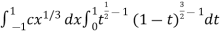
I = 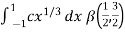
As we know that by beta function,

Which gives,
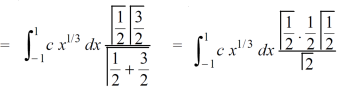
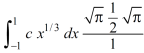
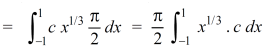
Now put the value of c, we get
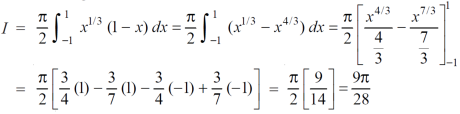
Example-3: Evaluate the following double integral,
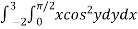
Sol. Let ,
I = 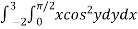
On solving the integral, we get
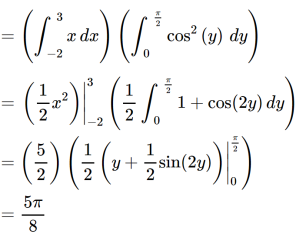
Double Integral over Rectangular and general regions
Consider a function f (x, y) defined in the finite region R of the x-y plane. Divide R into n elementary areas A1, A2,…,An. Let (xr, yr) be any point within the rth elementary are Ar
Fig. 6.1
f (x, y) dA = f (xr, yr) A
Evaluation of Double Integral when limits of Integration are given(Cartesian Form).
Ex. 1 : Evaluate 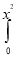 ey/x dy dx.
ey/x dy dx.
Soln. :
Given : I = 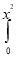 ey/x dy dx
ey/x dy dx
Here limits of inner integral are functions of y therefore integrate w.r.t y,
I = 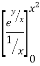
 dx
dx
= 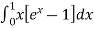
= 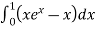
I = 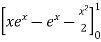
= =
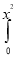 ey/x dy dx =
ey/x dy dx =
Ex. 2 : Evaluate x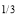 y
y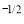 (1 – x –y)
(1 – x –y)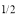 dx dy.
dx dy.
Soln. :
Given : I = x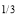 y
y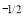 (1 – x –y)
(1 – x –y)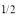 dx dy.
dx dy.
Here the limits of inner integration are functions of y therefore first integrate w.r.t y.
I = x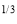 dx
dx 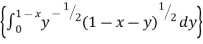
Put 1 – x = a (constant for inner integral)
I = x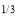 dx
dx 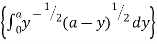
Put y = at dy = a dt
y | 0 | a |
t | 0 | 1 |
I = x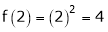 dx
dx 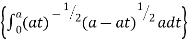
I = x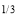 dx
dx 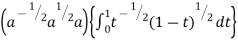
I = x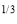 a dx
a dx
I = x (1 – x) dx = (x
(1 – x) dx = (x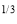 – x4/3) dx
– x4/3) dx
I = 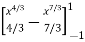
=
I = =
x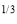 y
y (1 – x –y)
(1 – x –y) dx dy =
dx dy =
Ex. 3 : Evaluate 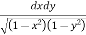
Soln. :
Let, I = 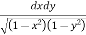
Here limits for both x and y are constants, the integral can be evaluated first w.r.t any of the variables x or y.
I = dy 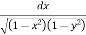
I = 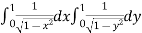
= 
= 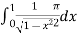
= 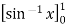
=
= 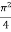
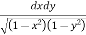 =
=
Ex. 4: Evaluate e–x2 (1 + y2) x dx dy.
Soln. :
Let I = e–x2 (1 + y2) x dy = dy e–x2 (1 + y2) x dy
= dy e– x2 (1 + y2)  dx
dx
= dy [∵ f (x) ef(x) dx = ef(x) ]
= (–1) dy (∵ e– = 0)
= = =
e–x2 (1 + y2) xdx dy =
NOTES:
Type II: Evaluation of Double Integral when region of Integration is provided (Cartesian form)
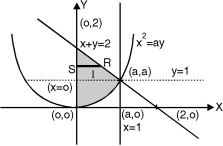
Ex.1: Evaluate y dx dy over the area bounded by x= 0 y =  and x + y = 2 in the first quadrant
and x + y = 2 in the first quadrant
Soln. :
The area bounded by y = x2 (parabola) and x + y = 2 is as shown in Fig.6.2
The point of intersection of y = x2 and x + y = 2.
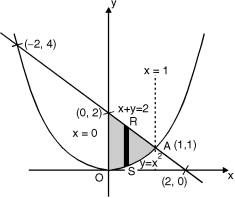
x + x2 = 2 x2 + x – 2 = 0
x = 1, – 2
At x = 1, y = 1 and at x = –2, y = 4
Fig. 6.2
(1, 1) is the point of intersection in Ist quadrant. Take a vertical strip SR, Along SR x constant and y varies from S to R i.e. y = x2 to y = 2 – x.
Now slide strip SR, keeping IIel to y-axis, therefore y constant and x varies from x = 0 to x = 1.
I = 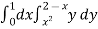
= 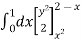
= 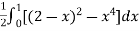
= (4 – 4x + 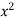 –
– 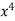 ) dx
) dx
= =
I = 16/15
Ex. 2 : Evaluate 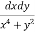 over x 1, y
over x 1, y 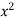
Soln. :
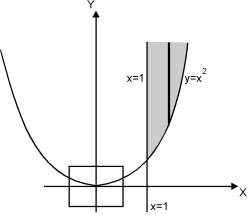 Let I =
Let I = 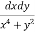 over x 1, y
over x 1, y 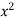
The region bounded by x 1 and y 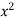
Is as shown in Fig. 6.3.
Fig. 6.3
Take a vertical strip along strip x constant and y varies from y = 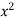
To y = . Now slide strip throughout region keeping parallel to y-axis. Therefore y constant and x varies from x = 1 to x = .
I = 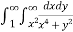
= 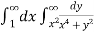
=  [ ∵
[ ∵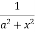 dx = tan–1 (x/a)]
dx = tan–1 (x/a)]
= 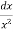 =
=
= – = (0 – 1)
I =
Ex. 3 : Evaluate (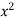 +
+ 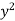 ) dx dy through the area enclosed by the curves y = 4x, x + y = 3 and y =0, y = 2.
) dx dy through the area enclosed by the curves y = 4x, x + y = 3 and y =0, y = 2.
Soln. :
Let I = (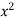 +
+ 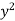 ) dx dy
) dx dy
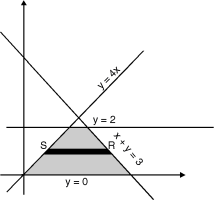 The area enclosed by the curves y = 4x, x + y =3, y = 0 and y = 2 is as shown in Fig. 6.4.
The area enclosed by the curves y = 4x, x + y =3, y = 0 and y = 2 is as shown in Fig. 6.4.
(find the point of intersection of x + y = 3 and y = 4x)
Fig. 6.4
Take a horizontal strip SR, along SR y constant and x varies from x = to x = 3 – y. Now slide strip keeping IIel to x axis therefore x constant and y varies from y = 0 to y = 2.
I = dy (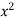 +
+ 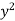 ) dx
) dx
= 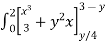
= 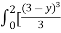 +
+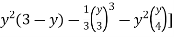 dy
dy
I = 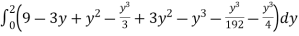
= 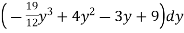
= 
= + – 6 + 18
I =
Triple integral-
Definition: Let f(x,y,z) be a function which is continuous at every point of the finite region (Volume V) of three dimensional space. Divide the region V into n sub regions of respective volumes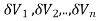 . Let (
. Let (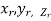 ) be a point in the
) be a point in the 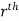 sub region then the sum:
sub region then the sum:
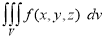 =
= 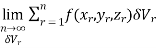
Is called triple integration of f(x, y, z) over the region V provided limit on R.H.S of above Equation exists.
Spherical Polar Coordinates
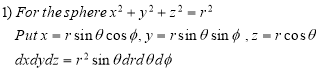
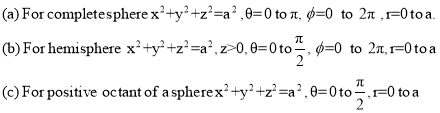
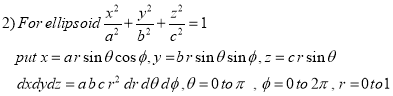
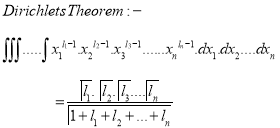
Where the integral is extended to all positive values of the variables subjected to the condition 
Ex.1: Evaluate
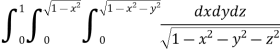
Solution: Let
I = 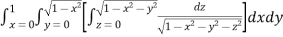
= 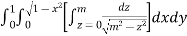
(Assuming m = 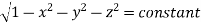 )
)
= 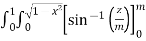 dxdy
dxdy
= 
=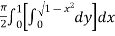
= 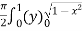 dx
dx
= 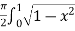 dx
dx
= 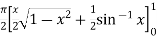
=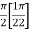
I =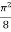
Ex.2: Evaluate 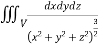 Where V is annulus between the spheres
Where V is annulus between the spheres 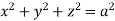
And 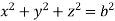 (
(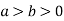 )
)
Solution: It is convenient to transform the triple integral into spherical polar co-ordinate by putting
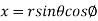 ,
, 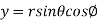 ,
, 
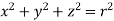 , dxdydz=
, dxdydz=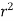 sin
sin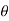 drd
drd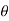 d
d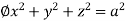 ,
,
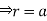 and
and 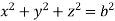
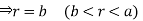
For the positive octant, r varies from r =b to r =a ,  varies from
varies from 
And 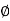 varies from
varies from 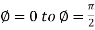
I= 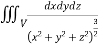
= 8
=8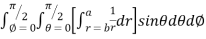
=8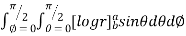
=8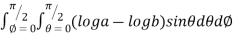
=8 log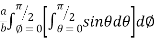
= 8 log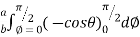
I= 8 log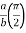 I = 4 log
I = 4 log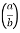
Ex.3: Evaluate 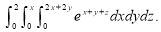
Solution:-
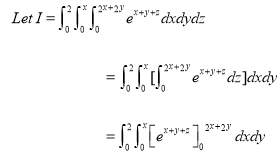
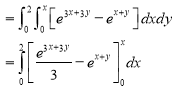
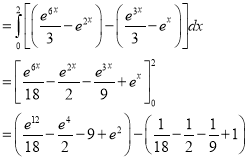
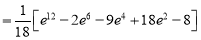
Ex.4:Evaluate 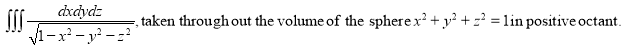
Solution:- 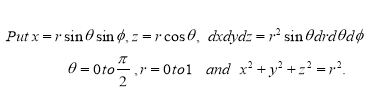
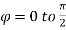
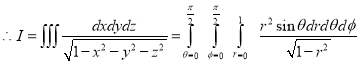
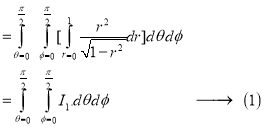
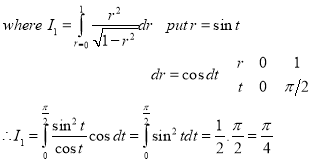
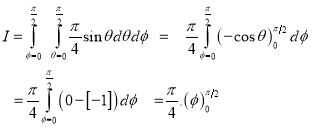
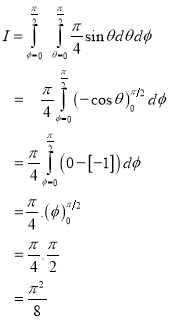
NOTES:
The volume of solid is given by
Volume =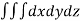
In Spherical polar system
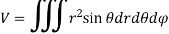
In cylindrical polar system
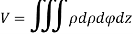
Ex.1: Find Volume of the tetrahedron bounded by the co-ordinates planes and the plane
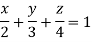
Solution: Volume =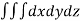 ………. (1)
………. (1)
Put  ,
, 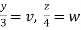
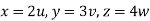
From equation (1) we have
V = 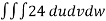
=24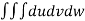
=24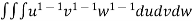 (u+v+w=1) By Dirichlet’s theorem.
(u+v+w=1) By Dirichlet’s theorem.
=24 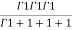
=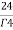 =
=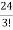 = 4
= 4
Volume =4
Ex.2: Find volume common to the cylinders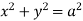 ,
, 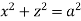 .
.
Solution: For given cylinders,
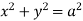 ,
,  .
.
Z varies from
Z=-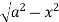 to z =
to z = 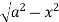
Y varies from
y= -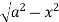 to y =
to y = 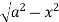
x varies from x= -a to x = a
By symmetry,
Required volume= 8 (volume in the first octant)
=8 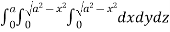
=8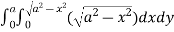
= 8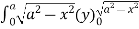 dx
dx
=8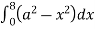
=8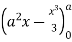
=8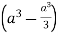
Volume = 16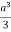
Ex.3: Evaluate
1. 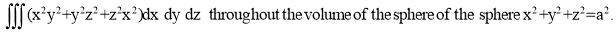 Solution:-
Solution:-
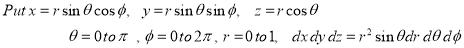
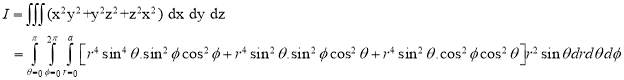
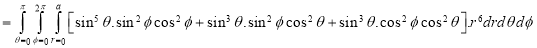
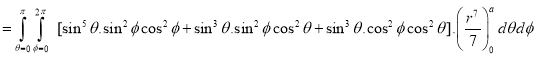
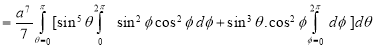
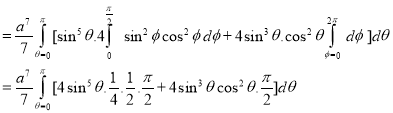
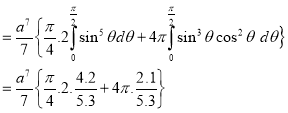
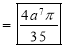
Ex.4: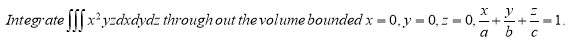
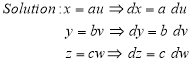
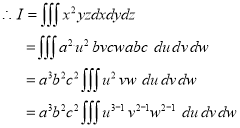

Ex.5: Evaluate 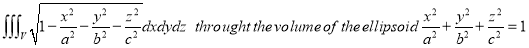
Solution:- Put
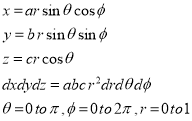
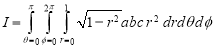
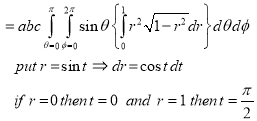

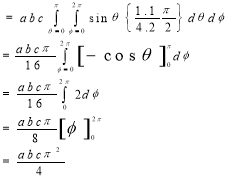
Ex. 1 : Change the order of integration for the integral 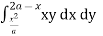 and evaluate the same with reversed order of integration.
and evaluate the same with reversed order of integration.
Soln. :
Given, I = 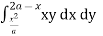 …(1)
…(1)
 In the given integration, limits are
In the given integration, limits are
y = 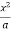 , y = 2a – x and x = 0, x = a
, y = 2a – x and x = 0, x = a
The region bounded by x2 = ay, x + y = 2a Fig.6.5
And x = 0, x = a is as shown in Fig. 6.5
Here we have to change order of Integration. Given the strip is vertical.
Now take horizontal strip SR.
To take total region, Divide region into two parts by taking line y = a.
1 st Region :
Along strip, y constant and x varies from x = 0 to x = 2a – y. Slide strip IIel to x-axis therefore y varies from y = a to y = 2a.
I1 = dy xy dx …(2)
2nd Region :
Along strip, y constant and x varies from x = 0 to x = . Slide strip IIel to x-axis therefore x-varies from y = 0 to y = a.
I2 = dy xy dx …(3)
From Equation (1), (2) and (3),
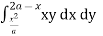 = dy xy dx + dy xy dx
= dy xy dx + dy xy dx
= 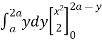 + y dy
+ y dy 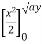
=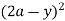 dy + (ay) dy = y (4a2 – 4ay + y2) dy + ay2 dy
dy + (ay) dy = y (4a2 – 4ay + y2) dy + ay2 dy
= (4a2 y – 4ay2 + y3) dy + y2 dy
= 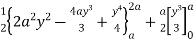
= 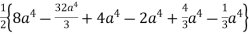 +
+ 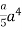
= a4
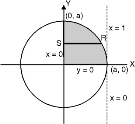
 Ex. 2 : Evaluate
Ex. 2 : Evaluate
I = 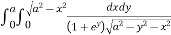
Soln. :
 Given : I =
Given : I = 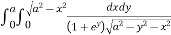 …(1)
…(1)
In the given integration, limits are
x = 0, x = a, y = 0, y = 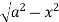
The bounded region is as shown in Fig. **.
In the given, strip is vertical. Now take horizontal strip SR. Along strip y constant and x varies from x = 0 to
x = 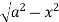 . Slide strip IIel to X-axis therefore y varies from y = 0 to y = a.
. Slide strip IIel to X-axis therefore y varies from y = 0 to y = a.
I = dy 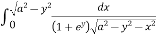
= 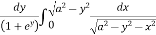
Put a2 – y2 = b2
I = 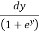
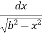
=  =
= 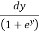
= 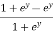 dy =
dy = 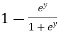 dy
dy
= 
=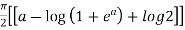
= 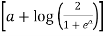
= 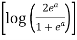 [∵ a = a loge]
[∵ a = a loge]
I = dy 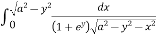 =
= 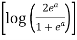
 Ex.3 : Express as single integral and evaluate dy dx + dy dx.
Ex.3 : Express as single integral and evaluate dy dx + dy dx.
Soln. :
Given : I = dy dx + dy dx
I = I1 + I2
The limits of region of integration I1 are
x = – ; x = and y = 0, y = 1 and I2 are x = – 1,
x = 1 and y = 1, y = 3.
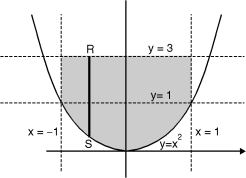
The region of integration are as shown in Fig. 6.7
To consider the complete region take a vertical strip SR along the strip y varies from y = x2 to
y = 3 and x varies from x = –1 to x = 1. Fig. 6.7
I = 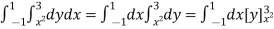

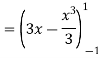
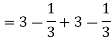
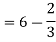
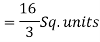
Evaluation of Double Integral by Changing Cartesian to Polar co-ordinates (when limits are given).
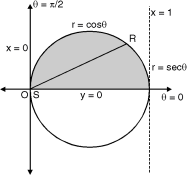 Ex. 1 : Evaluate
Ex. 1 : Evaluate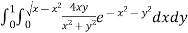
Soln. :
The region of integration bounded by
y = 0, y = 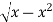 and x = 0, x = 1
and x = 0, x = 1
y = 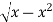 x2 + y2 = x
x2 + y2 = x
The region bounded by these is as shown in Fig. 6.8.
Convert the integration in polar co-ordinates by using x = r cos , y = r sin and dx dy = r dr d
x2 + y2 = x becomes r = cos
y = 0 becomes r sin = 0 = 0
x = 0 becomes r cos = 0 =
And x = 1 becomes r = sec
Take a radial strip SR with angular thickness , Along strip constant and r varies from r = 0 to r = cos . Turning strip throughout region therefore varies from = 0 to =
I = 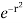 r dr d
r dr d
= 4 cos sin d r 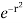 dr
dr
= 4 cos sin d [–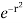 ]
]
= – 2 cos sin [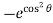 +1] d
+1] d
= – 2 [cos sin 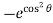 – cos sin ] d
– cos sin ] d
= –2 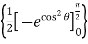 + 2 cos sin d
+ 2 cos sin d
= – + 2
= + 1 =
 Ex. 2: Sketch the area of double integration and evaluate
Ex. 2: Sketch the area of double integration and evaluate
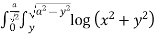 dxdy
dxdy
Soln. :
Let I = 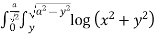 dxdy
dxdy
The region of integration is bounded by the curves
x = y, x = 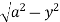 and y = 0, y = Fig. 6.9
and y = 0, y = Fig. 6.9
i.e. x = y, x2 + y2 = a2 and y = 0, y =
The region bounded by these is as shown in Fig. 6.9.
The point of intersection of x = y and x2 + y2 = a2 is x =
Convert given integration in polar co-ordinates by using polar transformation x = r cos , y = r sin and dx dy = r dr d
x = y gives r cos = r sin tan = 1 =
x2 + y2 = a2 r2 = a2 r = a
y = 0 gives r sin = 0 = 0.
y = gives r sin = r = cosec
Take a radial strip SR, along SR constant and r varies from r = 0 to r = a. Turning this strip throughout region therefore varies from = 0 to =
I = log r2 r dr d = 2 d r log r dr
I = 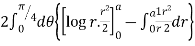
= 2 d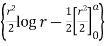
= 2 d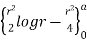
= 2 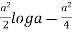 d
d
= 2 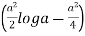 []
[]
I = 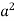 [/4] =
[/4] = 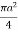
Evaluation of Double Integral when region of Integration is provided (Polar form)
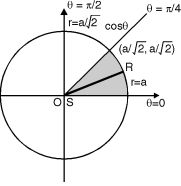
Ex. 1 : Evaluate r4 cos3 dr d over the interior of the circle r = 2a cos
Soln. :
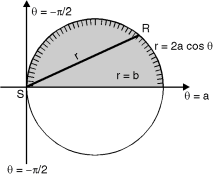 The region of the integration is as shown in Fig. 6.10.Take a radial strip SR, along strip constant and r varies from r = 0 to r = 2a cos. Now turning this strip throughout region therefore varies from = to =
The region of the integration is as shown in Fig. 6.10.Take a radial strip SR, along strip constant and r varies from r = 0 to r = 2a cos. Now turning this strip throughout region therefore varies from = to =
I = r4 cos3 dr d
= cos3 d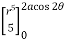
= 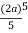 cos3 cos5 d
cos3 cos5 d
= 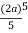 cos8 d Fig. 6.10
cos8 d Fig. 6.10
= 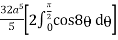
=  2
2
I = 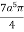
r4 cos3 dr d = 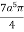
Ex. 2 : Evaluate r sin dr d over the cardioid r = a (1 – cos ) above the initial line.
Soln. :
The cardioid r = a (1 – cos ) is as shown in Fig. 6.11. The region of the integration is above the initial line.
Take a radial strip SR, along strip constant and r Varies from r = 0 to r = a (1 – cos ).
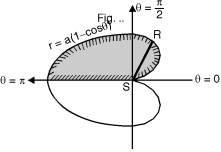 New turning the strip throughout region therefore varies from = 0 to = .
New turning the strip throughout region therefore varies from = 0 to = .
I = r sin dr d
= sin d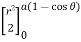
Fig.6.11
= sin [a2 (1 – cos )2]
= 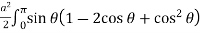
I = 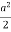 (sin – 2 sin cos + sin
(sin – 2 sin cos + sin 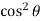 ) d
) d
= 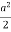 2 (sin – sin2 + sin
2 (sin – sin2 + sin 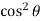 ) d
) d
= 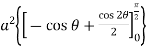 +
+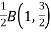
I = a2= a2
I = 
NOTES:


 Area enclosed by plane curves expressed in Cartesian coordinates:
Area enclosed by plane curves expressed in Cartesian coordinates:
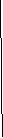



 Y (x,y) P Q (x+dx,y+dy) y=f(x)
Y (x,y) P Q (x+dx,y+dy) y=f(x)


 V U
V U
G H dxdy
 A R S B
A R S B
x=a y=0 x=b X
Consider the area enclosed by the curves 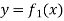
And 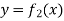 and the ordinates
and the ordinates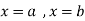
Area =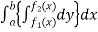
Consider the area enclosed by the curves 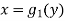
And 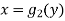 and the ordinates
and the ordinates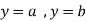
Area =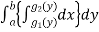
Area enclosed by plane curves expressed in Polar coordinates:
Consider the area enclosed by the polar curves 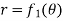
And 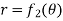 and the line
and the line 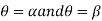
Area =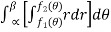
Ex.1: Find the area between the curves 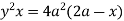 and its asymptote.
and its asymptote.
Solution: The curve is symmetrical about x axis not passing through origin. Also 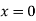 is the asymptote to the curve and intersect x axis at
is the asymptote to the curve and intersect x axis at  for
for 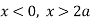 curve doesn’t exists. And for
curve doesn’t exists. And for 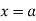 and
and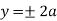 . Because of symmetry
. Because of symmetry
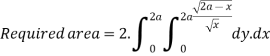
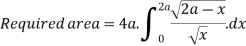
Put 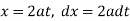
x | 0 | 2a |
t | 0 | 1 |
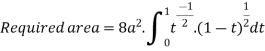
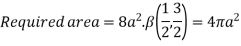
Ex: 2: Show that the area of curve 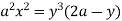 is
is 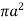
Solution: The curve is symmetrical about Y - axis passing through origin and there exists a cusp at origin. It intersects Y-axis at (0 , 0) , (0 , 2a) .
Also 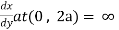 .
.
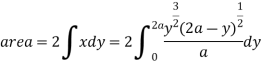
Putting 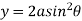
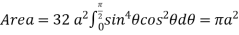 .
.
Ex.3: Find the Area included between the two cardioids 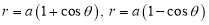
Solution: - Area = 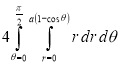
= 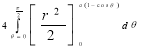
= 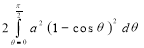
= 
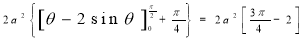
Ex.4: Find the Area common to the two circle 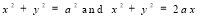
Solution: - By converting the given circle into polar form we get
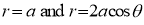
Area = 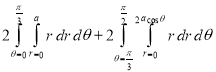
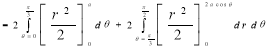
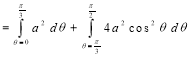
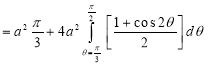

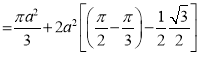
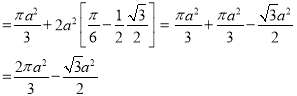
Area in Cartesian coordinates-
Example-1: Find the area enclosed by two curves using double integration.
y = 2 – x and y² = 2 (2 – x)
Sol. Let,
y = 2 – x ………………..(1)
And y² = 2 (2 – x) ………………..(2)
On solving eq. (1) and (2)
We get the intersection points (2,0) and (0,2) ,
We know that,
Area = 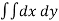

Here we will find the area as below,
Area = 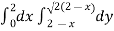
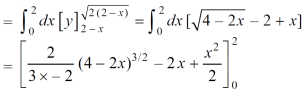
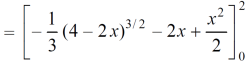
Which gives,
= ( - 4 + 4 /2 ) + 8 / 3 = 2 / 3.
Example-2: Find the area between the parabola y ² = 4ax and another parabola x² = 4ay.
Sol. Let,
y ² = 4ax ………………..(1)
And
x² = 4ay…………………..(2)
Then if we solve these equations , we get the values of points where these two curves intersect
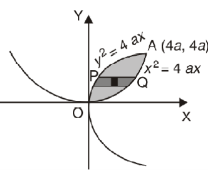
x varies from y²/4a to 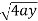 and y varies from o to 4a,
and y varies from o to 4a,
Now using the conceot of double integral,
Area = 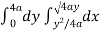
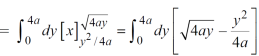
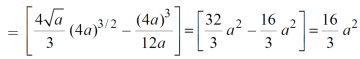
Area in polar coordinates-
Example-3: Find the area lying inside the cardioid r = a(1+cosθ) and outside the circle r = a, by using double integration.
Sol. We have,
r = a(1+cosθ) …………………….(1)
And
r = a ……………………………….(2)
On solving these equations by eliminating r , we get
a(1+cosθ) = a
(1+cosθ) = 1
Cosθ = 0
Here a θ varies from – π/2 to π/2
Limit of r will be a and 1+cosθ)
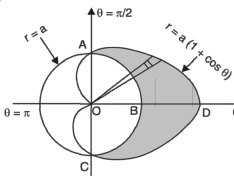
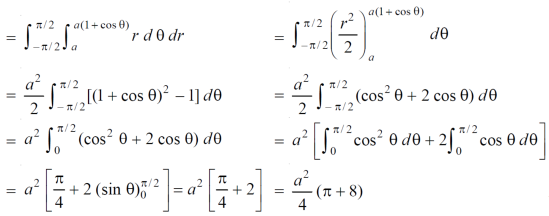
Which is the required area.
Example-4: Find the are lying inside a cardioid r = 1 + cos θ and ouside the parabola r(1 + cos θ) = 1.
Sol. Let,
r = 1 + cos θ ……………………..(1)
r(1 + cos θ) = 1……………………..(2)
Solving these equestions , we get
(1 + cos θ )( 1 + cos θ ) = 1
(1 + cos θ )² = 1
1 + cos θ = 1
Cos θ = 0
θ = ±π / 2
So that, limits of r are,
1 + cos θ and 1 / 1 + cos θ
The area can be founded as below,
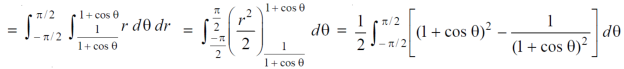
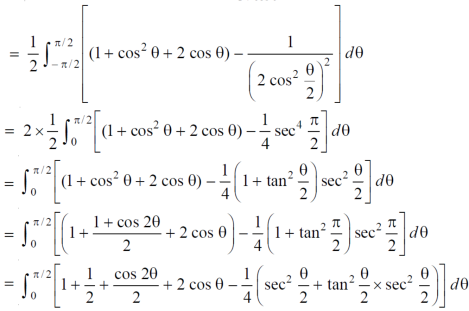
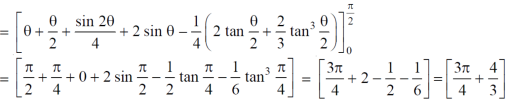
The centre of gravity of a lamina is the point where it balances perfectly which means the lamina’s centre of mass.
If 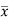 and
and 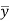 are the coordinates of the centroid C of area A, then-
are the coordinates of the centroid C of area A, then-
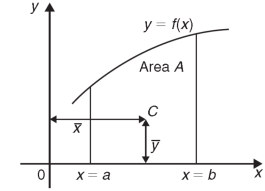
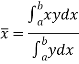
And
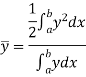
Example: Determine the coordinates of the centroid of the area lying between the curve y = 5x - x² and the x-axis.
Sol.
Here y = 5x - x² when y = 0, x = 0 or x = 5
Therefore the curve cuts the x-axis at 0 and 5 as in the figure-
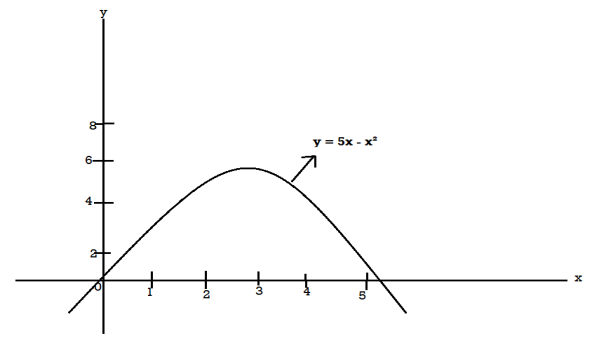
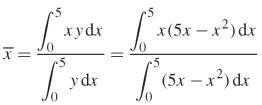
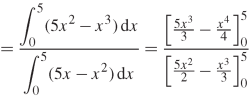
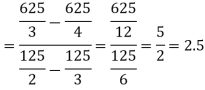
Now
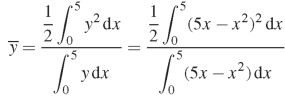
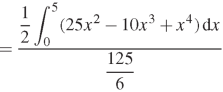
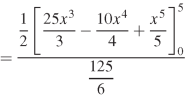
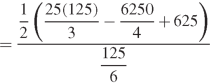
= 2.5
Therefore the centroid of the area lies at (2.5, 2.5)