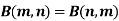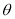UNIT-4

Calculus

4.1. Mean value theorem: Rolle’s theorem

Important definitions-

Continuity- suppose that a function f(x) is defined in the interval I , then it is said to be continuous at x=a ,  if

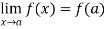
Differentiability- A function f(x) is said to be differentiable at x=a if

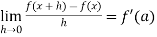exists      where ‘a’ belongs to I

Rolle’s theorem-

Suppose f(x) is a function defined on [a , b] and it satisfies the following conditions

1. f(x) is continuous in [a , b]

2. f(x) is differentiable in (a , b)

3. f(a) = f(b)

Then there exists atleast a point point c ϵ (a , b) , where a<b , such that f’(c) = 0

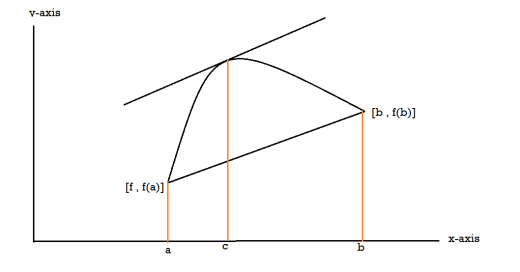
Proof: suppose y = f(x) is a function and A(a , f(a)) , B(b , f(b)) be two points on the curve f(x) and a,b are two end points. Now conditions for Rolle’s theorem-

1.f(x) is a continuous function in[a , b] , from the figure without breaks in between  A&B on y = f(x).

2. f(x) is differentiable in (a , b), because joining A and B we get a line AB.

Slope of the line AB=0 then a point C at P also a tangent at P, or Q,R,S is parallel to x –axis.

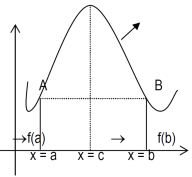
Slope of the tangent at P or Q,R,S , will be 0 ,even the curve y = f(x) decreases or increases, that means f(x) is constant.

Derivative of f(x),

f’(c) = 0

That’s why,     f’(c) = 0

3. The slope of the line AB is equal to zero, that means the line AB is parallel to x-axis.

So that,       f(a) = f(b)

Example: Verify Rolle’s theorem for the function f(x) = x(x+3) in interval [-3 , 0].

Sol. First we will differentiate the given function with respect to x, we get

f’(x) = (x²+3x) 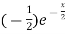+ (2x + 3)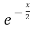

=

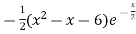
This shows that f’(x) exists for all x, therefore f(x) is continuous for all x.

Now, f(-3) = 0 and f(0) = 0 , so that f(-3) = f(0).

Here f(x) satisfies all the conditions of Rolle’s theorem,

Then,

f’(x) = 0  , which gives

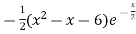= 0

We get,

X = 3 and x = -2

Here  we can see that clearly -3<-2<0 , therefore there exists   -2 ∈ (-3,0) such that

f’(-2) = 0

That means the Rolle’s theorem is true for the given function.

Example: Verify Rolle’s theorem for the given functions below-

1.  f(x) = x³ - 6x²+11x-6  in the interval [1,3]

2. f(x) = x²-4x+8  in the interval [1,3]

Sol. (1)

As we know that every polynomial is continuous and differentiable for all points, so that the given function is continuous and differentiable in the interval [1,3]

Also, f(1) = f(3) = 0

Now we find f’(x) = 0

3x² - 12x +11 = 0

We get, x = 2+ 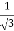and 2 -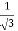

Hence both of them lie in (1,3).

Hence the theorem holds good for the given function in interval [1,3]

(2) As we know that every polynomial is continuous and differentiable for all points, so that the given function is continuous and differentiable in the interval [1,3]

Also,  f(1) = 1 -4 +8 = 5 and f(3) = 9 – 12 + 8 = 5

Hence f(1) = f(3)

Now the first derivative of the function,

f’(x) = 0

2x – 4 = 0 , gives

X = 2

We can see that 1<2<3, hence there exists 2 between 1 and 3. And f’(2) = 0.

This means that the Rolle’s theorem holds good for the given function and given interval.

Example: Verify the Rolle’s theorem for sin x in the interval []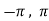

Sol. Suppose f(x) = sin x

We know that sin x is continuous for all x.

Now , f’(x) = cos x exists for all x in (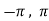) and

f(0

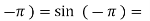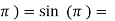f(0

Thus f(x) satisfies all the conditions of Rolle’s theorem.

Now,

f’(x) = 0 that gives ,    cos x = 0

x =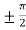  

Here we notice that both intervals lie in (.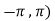

There exists, c =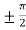

So that, f’(c) = 0

The Rolle’s theorem has been verified.

4.2. Lagrange’s mean value theorem with their geometrical interpretation and applications

Suppose that f(x) be a function of x such that,

1. If it is continuous in [a , b]

2. If it is differentiable in (a , b)

Then there atleast exists a value cϵ (a , b)

f’(c) =

Proof:

Lets define a function g(x),

g(x) = f(x) – Ax  ………………..(1)

Here A is a constant which is to be determined,

So that,  g(a) = g(b)

Now,

g(a) = f(a) – Aa

g(b) = f(b) – Ab

So,

g(a) = g(b),

f(a) – Aa = f(b) – Ab ,

Which gives,

A = 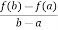    …………………..(2)

As right hand side of eq.(1) is continuous in [a,b] , so that g(x) is continuous.

And right hand side of eq.(1) is differential in (a,b) , so that g(x) is differentiable in (a,b).

And g(a) = g(b) , because of the choice of A.

Hence g(x) satisfies all the conditions of Rolle’s theorem.

So that,

There exists a value c  such that a<c<b at which g’(c) = 0

Now, differentiate eq. (1) with respect to x, we get

g’(x) = f’(x) – A

Here we know that, x = c,

g’(c) = f’(c) – A

As g’(c) = 0, then

f’(c) – A =0

So that,, f’(c) = A,

From equation (2) , we get

f’(c) = 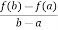                                            hence proved.

Example:  Verify Lagrange’s mean value theorem for f(x) = (x-1)(x-2)(x-3) in [0,4].

Sol. As we see that the given function is a polynomial and we know that the polynomial is continuous in [0,4] and differentiable in (0,4).

f(x) = (x-1)(x-2)(x-3)

f(x) =  x-6x²+11x-6

Now at x = 0, we get

f(0) = -6  and

At x = 4, we get.

f(4) = 6

Diff. The function w.r.t.x , we get

f’(x) = 3x²-6x+11

Suppose x = c, we get

f’(c) = 3c²-6c+11

By Lagrange’s mean value theorem,

f’(c) = 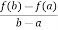   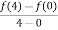 =  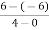   =   = 3                                                                                                                        

Now we get,

3c²-6c+11 = 3

3c²-6c+8 = 0

On solving the quadratic equation, we get

C = 2   

Here we see that the value of c lies between 0 and 4

Therefore the given function is verified.

Example: :  Verify Lagrange’s mean value theorem for f(x) = log xin [1,e].

Sol. We already know that the function which is log x is continuous for all x>0.

So that this is the continuous function In [1,e]

Now,

f’(x) = 1/x

Which is exists for all x in (1,e)

So that f(x) is differentiable in (1,e).

By Lagrange’s mean value theorem, we get

f’(c) = 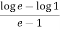, let x = c,

Then ,

f’(c) =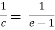       

We get,

c = e-1

e-1 will always lies between 1 and e .

Hence the function is verified by Lagrange’s mean value theorem.

4.3. Cauchy’s mean value theorem

Suppose we have two functions f(x) and g(x) of x, such that,

1. Both functions are continuous in [a,b]

2. Both functions are differentiable in (a,b)

3. g’(x) ≠ 0 for any x ϵ (a,b)

These three exists atleast , x = c ϵ (a,b) , at which

Proof: suppose ,we define a functions,

h(x) = f(x) – A.g(x) …………………….(1)

So that h(a) = h(b) and A is a constant to be determined.

Now ,

h(a) = f(a) – Ag(a)

h(b) = f(b) – A.g(b)

So that,

f(a) – Ag(a) = f(b) – A.g(b) , which gives

A =     …………………………….(2)

Now , h(x) is continuous in [a,b] as RHS of eq. (1) is continuous in [a,b] and h(x) is diff. In (a,b) as RHS of eq. (1) is diff. In (a,b)

Also,

h(a) = h(b)

Therefore all the conditions of Rolle’s theorem are satistfied then there exists a value  x = cϵ (a,b)

So that h’(c) = 0

Differentiate eq.(1) w.r.t. x , we get

h’(x) = f’(x) – A.g’(x)

At x = c

h’(c) = f’(c) – A.g’(c)

0 = f’(c) – A.g’(c)

A =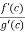

So that , we get

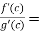
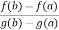    where a<c<b

Hence the Cauchy’s mean value theorem is proved.

Example: Verify Cauchy’s mean value theorem for the function f(x) = sin x and g(x) = cos x  in [ 0 , π/2]

Sol.  It is given that ,

f(x) = sin x and g(x) = cos x

Now ,

f’(x) = cos x and g’(x) = - sin x

We know that both the functions are continuous in [ 0 , π/2] and differentiable in     ( 0 , π/2 )

Also , g’(x) = -sin x  ≠ 0 for all x ϵ( 0 , π/2 )

By Cauchy’s mean value theorem , we get

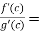
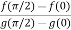       for some c: 0< c <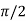

That means

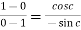  which gives,

Cot c = 1

C =

Now we see that lies between 0 and

Example: Verify Cauchy’s mean value theorem for the function f(x) = x⁴ and g(x) = x² in the interval [1,2]

Sol. We are given, f(x) = x⁴ and g(x) = x

Derivative of these fucntions ,

f’(x) = 4x³ and g’(x) = 2x

Put these values in Cauchy’s formula, we get

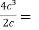
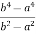
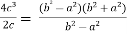
2c² =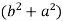

c² =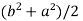

c =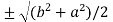

Now put the values of a = 1 and b = 2 ,we get

c = 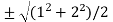= =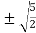 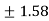(approx..)

Hence the Cauchy’s theorem is verified.

4.4. Taylor’s series

Taylor’s theorem for a function f(x) can be expressed as an infinite series as follows-

f(x) = f(a) + (x – a)f’(a) +  + ……….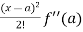

Example: Expand the function 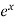in ascending powers of (x – 1) by using Taylor’s theorem.

Sol. We know that the Taylor’s theorem for the function f(x) in ascending powers of (x – a) is,

f(x) = f(a) + (x – a)f’(a) + 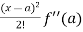 + ………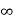.                ………….(1)

Here f(x) = 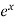and a = 1

Now,

f’(x) = 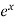and f’(a) = e

f’’(x) = 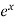and f’’(a) = e

Put these values in equation (1),

…………….

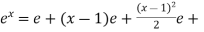Take ‘e’ as common,

…………….}

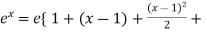Which is the required expansion.

Example: Find the Taylor’s series for the function f(x) = 3x² - 6x + 5 in ascending powers of x-3.

Sol. We know that the Taylor’s theorem for the function f(x) in ascending powers of (x – a) is,

f(x) = f(a) + (x – a)f’(a) +  + ………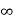.                ………….(1)

Here ,

f(x) = 3x² - 6x + 5 and a = 3.   So that f(a) = 14

Now , we will find out the derivatives ,

f’(x) = 6x – 6  and  f’(3) = 12

f’’(x) = 6  and f’’(3) = 6

f’’’(x) = 0 and f’’’(x) = 14

Put these values in Taylor’s theorem, we get

3x² - 6x + 5 = 14 + 12(x-3) + 6 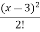+…………. Ans.

4.5. Applications of definite integrals to evaluate surface areas and volumes of revolutions of curves (only in Cartesian coordinates)

There is wide range of definite integrals such as, from geometric applications to physical world problems.

We will study about these applications one by one-

(1) Area between the curves-

Suppose we want to find out the area between y = f(x) and y = g(x) on interval [a,b] such that f(x) ≥ g(x). See the figure

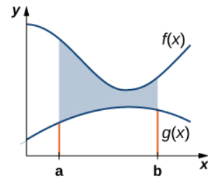
Let the area between the two curve is A, then

A =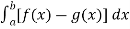

Lets do some examples,

Example-1: find the area under the curves where f(x) =  x+4 and g(x) =  3 – x/2 over the interval [1,4]

Sol. Here limits are given a = 1 , b = 4,

We know that, area under the curve,

A =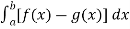

= 

=

= 

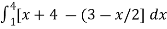
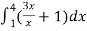
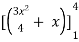
=  (16 – 7/4) =  57/4

So that the area of the region is 57/4 unit square.

Example-2: find the area under the curves where y =  x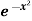 and y =  x + 1,         x = 2 and y-axis.

Sol. When we draw the graph, curve does not meet, but depend on two vertical lines,

Here boundary is [0 , 2]

Area under the curve,

A =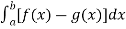

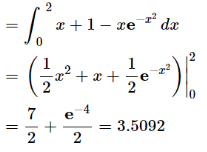
So that the area under the curve is 3.2092 unit square.           

Example-2: Determine the area under the curves y = 2x² + 10 and y = 4x + 16.

Sol. Here we will find out the intersection, which are the boundaries of the curve,

2x² + 10 =  4x + 16

2x² - 4x – 6 = 0

2 (x + 1) ( x – 3 ) = 0

We get, x = -1  and x = 3,

We know that, Area under the curve,

A =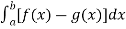

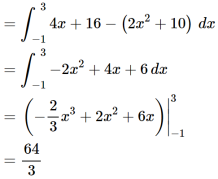
(2) Average function value-

We can use definite integrals to find out the average value of a function.

The average value of a function over the interval [a , b]  is given by the following expression ,

f( avg.) =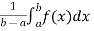      

Example-1: find the average value of the function f(x) = x³ over the interval [0,1].

Sol.  We know that

f( avg.) =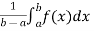      

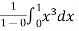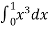=      =       = 1 / 4

Example-2: find the average value of f(x) = cos x over the interval [0 , 𝛑/2]

Sol. We know that,

f( avg.) =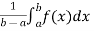      

Put the values ,  we get

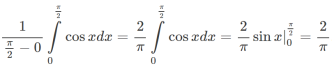
(3) Work-

This one is the easiest use of definite integral

We know that work done, when a constant force F is applied to move the object over a distance d.

W = Fd

Now suppose that the force at any point x is F(x) , the the work done by to move the object from x = a to x = b is,

W =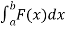      

Example-1: Find the work done on a spring when we compress it from its natural length of 1m to the 0.75m , if the spring constant k = 16 N / m.

Sol. The force is given by,

F =16x

Here we start to compress it at x = 0 and finish at x= 0.25 from its natural length.

So we take lower limit and upper limit 0 and 0.25 respectively.

We know that,

W =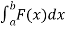      

=       

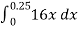
= 0.5 N.m

Example-2: A force of 1200 N compresses a spring from its natural length of 18 cm to a length of 16 cm. How much work is done in compressing it from 16 cm to 14 cm?

Sol.  Here,

F = kx

So that,

1200 = 2k

K = 600 N/cm

In that case,

F = 600x

We know that,

W =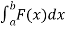      

W = 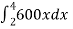      , which gives

W  = 3600 N.cm

Method for volume-

Suppose A(x) represents the area of the cross section at any point x over the interval [a,b], then the volume of the solid is represented by,

V(S)  =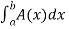      

This method is known as slicing method.

Example-1: find out the volume of pyramid with square base by slicing method.

Sol.

Here pyramid has square base , then its volume will be V = a²h , where a is the length of side of base.

We will derive this formula by slicing method.

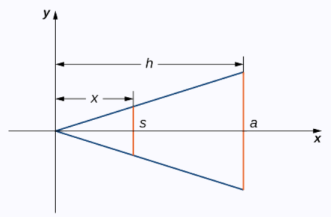
In the above figure , there is a pyramid on x-axis,

Here we see that there are similar tirangles,

Then by basic proportionality theorem( BPT) that we have studied in high school,

We get,

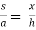
Which gives,

s =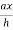

Then the area of cross-sectional squares,

A(x) =  s² =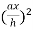

To find the volume of pyramid, we integrate from 0 to h,

V= == ,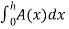

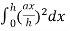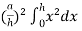Which gives,

V = a²h.

This is formula for volume of pyramid with square base.

Example-2: Using slicing method, find out the volume of solid of revolution bounded by f(x) = x² - 4x +5 , x = 1 and x = 4, rotated about the x-axis.

Sol. Intervals are given.   [ 1 , 4]

Here the solid is formed by revolving the region about x-axis , the cross – sections are circle .

Area of the cross section is , then is the area of circle, here radius of the circle is given by f(x).

We know that,

Area of the circle= π r²

= π [f(x)]²

= π[x² - 4x +5]²

Volume will be,

V =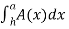       

=       

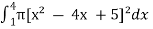
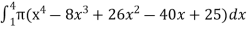=       which gives,

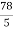=  π

So the volume is 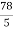π

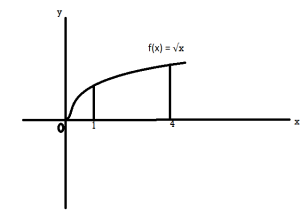
Example-3: Find the volume of the solid of revolution generated by rotating the region between the graph of f(x) = √x over the interval [1,4] around x-axis.

Sol. The graph of the function will look like as follow,

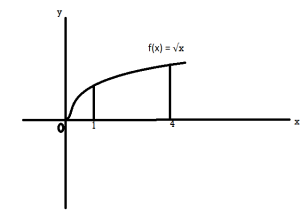
On rotation it will make circle ( cross-section)

We know that,

V =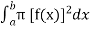       

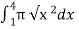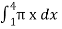=     =     , which gives

= 

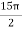
The volume is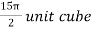

We can use definite integrals to find out the length of the arc of a curve.

Suppose a rocket launched along a parabolic path and if we are interested to find the length of the how much distance has rocket covered the we can use the concept of arc length of curve.

Arc length of the curve y = f(x) –

Let f(x) be a smooth function over the interval [a,b]. Then the arc length of the proportion of f(x) from point (a,f(a)) to the point (b, f(b))  is given by,

Length of Arc  =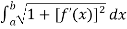 

Note -  smooth functions- functions which are continuous derivative are called smooth.

Example-1:  let f(x) = 2 x³/² , then calculate the arc length of the graph over interval [ 0 , 1].

Sol. Here we can find its first derivative

f’(x) = 3 x¹/²

Such that,    

[f’(x)]² = 9x

Then we know that,

Length of Arc  = 

=

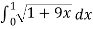
Now,

Let u = 1 + 9x , then du =  9 dx , when x = 0 then u = 1 and x = 1 then u = 10,

So,

=  

=   

= 1/9 ×2/3 × 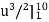=  2.268 units

Example-2: suppose a wire hanging on two poles follows the curve,

f(x) = a cosh(x/a)  

Find the length of the wire.

Sol. We will find the first derivative of f(x),

f’(x) =  sinh(x/a)

The curve of f(x) will look like,

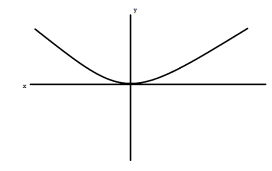
Hence the curve os symmetric, we will measure the length of one side first,

The limits on one side will be, 0 to b

We know that

Length of Arc  =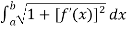 

Put  f’(x) =  sinh(x/a), we get

Length of Arc  = 

Use the identity,

=

=

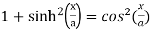
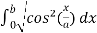
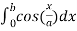
We get on solving,

a sinh(b/a)

On both sides, the length of the curve will be,

2 a sinh(b/a)

Example_3: Find the length of f(x) = x between  x = 2 and x = 3.

Sol.  The derivative of f(x) will be,

f’(x) = 1

And we know that,

Length of Arc  =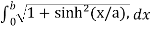 

So that,

Length of Arc  =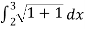 

=   

On solving the integral, we get

=  (3 – 2) = .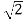

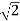Area of surface of revolution-

Suppose we have a curve y = f(x) and if we revolve this curve around x – axis then the area formed is known as surface area of revolution.

Let f(x) be a non-negative smooth function over the interval[ [ a , b] , then the surface area of the surface of revolution formed by revolving the graph of f(x) around x-axis is given by,

S =

Example-1: find the surface of the surface generated by revolving the graph f(x) = 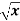over the interval [1,4] around x-axis.

Sol.  The graph of the function will look like

f(x) = 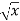then f’(x) = 1/2 (

(f’(x))² = 1/4x

Then,

S =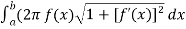

S =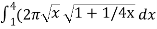

S = ,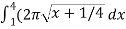

Let u = 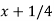  , then du =  dx, when  x = 1 , u = 5/4 and when x = 4 , u = 17/4,

This will give,

=

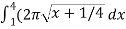On solving the integral we get,

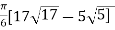= 30.84 (approx.)

Example-1: find the surface of the surface generated by revolving the graph g(y) = 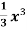over the interval [0,2] around y-axis.

Sol.  Hint -  use formula

S =

Ans. = 24.118

4.6. Definition of improper integral: Beta & Gamma functions and their properties.

(1) Let f is function defined on [a , ∞) and it is integrable on [a , t] for all t >a, then

If 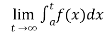exists , then we define the improper integral of f over [a , ∞) as follows-

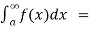
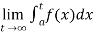
(2) Let f is function defined on (-∞,b] and it is integrable on [t , b] for all t >b, then

If 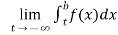exists , then we define the improper integral of f over (-∞ , b] as follows-

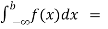
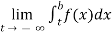
(3) ) Let f is function defined on (-∞, ∞] and it is integrable on [a , b] for every closed and bounded interval [a , b] which is the subset of R., then

If 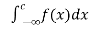and 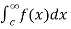exist for some c belongs to R , then we define the improper integral of f over (-∞ , ∞ ) as follows-

= +

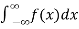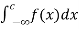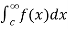
(4) Let f is function defined on (a , ∞) and 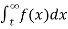exists for all t>a , then

If 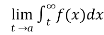exists , then we define the improper integral of f over (a , ∞) as follows-

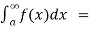
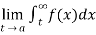
(5)  ) Let f is function defined on (-∞ , b) and 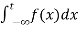exists for all t<b  , then

If 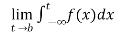exists , then we define the improper integral of f over (-∞ , b) as follows-

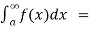
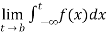
Improper integrals over finite intervals-

(1) Let f is function defined on (a, b] and 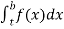exists for all t ∈(a,b)  , then

If 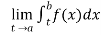exists , then we define the improper integral of f over (a , b] as follows-

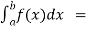
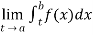
(2) Let f is function defined on [a, b) and 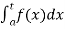exists for all t ∈(a,b)  , then

If 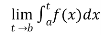exists , then we define the improper integral of f over [a , b) as follows-

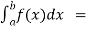
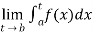
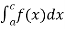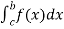(3) Let f is function defined on [a, c) and (c , b] . If and   exist

Then we define the improper integral of f over [a , b] as follows-

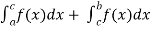
Beta & gamma functions and their properties-

A function,

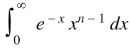
Is called gamma function of n , which can be written as,

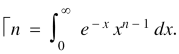
Some important results-

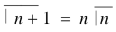
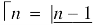
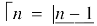
Ex.1: Evaluate   0∞  x3/2  e -x dx

Solution:               0∞  x3/2  e -x dx          =   0∞  x 5/2-1  e -x dx  

=  γ(5/2)

=   γ(3/2+ 1) 

=    3/2   γ(3/2 )  

=   3/2 .  ½   γ(½ ) 

=  3/2 . ½ .π 

=    ¾ π  

Ex. 2: Find  γ(-½) 

Solution:       (-½) + 1  =  ½
γ(-1/2)  =   γ(-½ + 1)  / (-½)   

=   - 2   γ(1/2 )  

=  - 2 π  

Ex. 3.  Show that      

Solution : =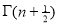

=

= )  .......................

=

=

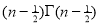
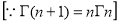
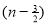
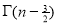
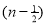
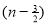
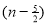
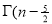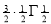
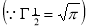
Ex. 4: Evaluate 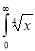 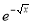dx.

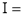
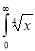
Solution :  Let   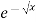 dx 

X	0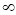
t	0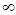

Put 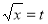or 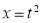;dx =2t 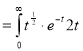dt .

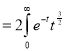dt

dt

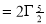
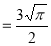
Ex. 5: Evaluate 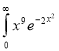  dx.

Solution :   Let 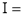 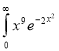 dx.

x	0
t	0

Put 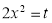or 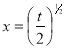 ;             4x dx = dt

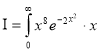dx

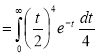
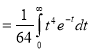
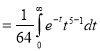
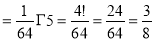
Definition : Beta function

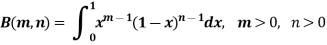
Properties of Beta function :

2.

3.

4.

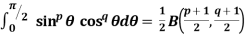Example(1):  Evaluate    I   =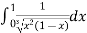  

Solution: 

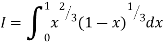
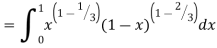
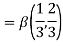
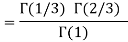
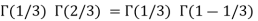
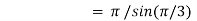
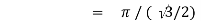
= 2 π/3

Example(2): Evaluate:  I =  02  x2   / (2 – x )   . Dx

Solution:

Letting   x  = 2y, we get

I   =   (8/2) 01  y 2  (1 – y ) -1/2dy

=  (8/2) . B(3 , 1/2 )

= 642 /15 

BETA FUNCTION MORE PROBLEMS

Relation between Beta and Gamma functions :

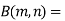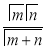Example(1):   Evaluate:  I =  0a  x4   (a2 – x2 )   . Dx Solution:                      Letting   x2  = a2 y , we get I   =   (a6  / 2) 01  y 3/2  (1 – y )1/2dy =  (a6  / 2) .  B(5/2 , 3/2 ) =  a6  /3 2 Example(2):   Evaluate:  I =  02 x (8 – x3 )   . Dx Solution:                Let   x3  = 8y I =  (8/3) 01  y-1/3      (1 – y ) 1/3   . Dy   = (8/3) B(2/3 , 4/3 ) =  16 π / ( 9 3 )   Example(3):   Prove that 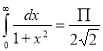Solution : Let 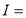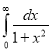Put 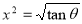 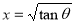 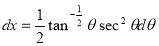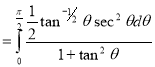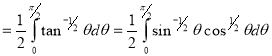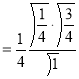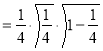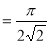or  ,  Example(4):  Evaluate 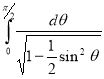Solution :Let 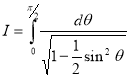Put 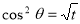 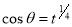 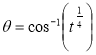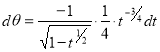or  , , When 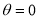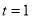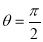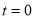, ; , o 1 0 Also 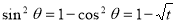 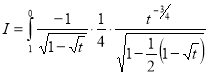 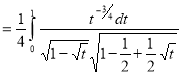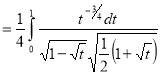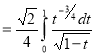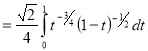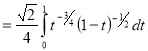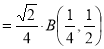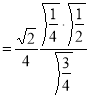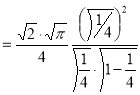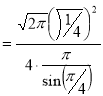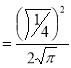Example(5):   Show that 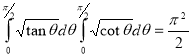Solution : 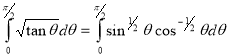= 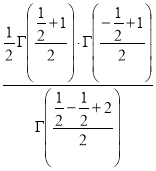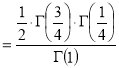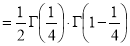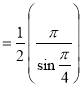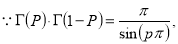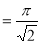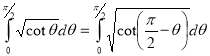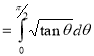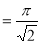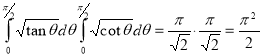( 0<p<1) (by above result)