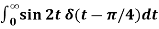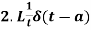Unit - 1
Laplace Transformation
Let f(t) be any function of t defined for all positive values of t. Then the Laplace transform of the function f(t) is defined as-
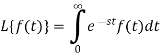
Provided that the integral exists, here ‘s’ is the parameter that could be real or complex.
The inverse of the Laplace transform can be defined as below-
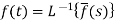
Here 
f(t) is called the inverse Laplace transform of 
L is called the Laplace transformation operator.
Conditions for the existence of Laplace transform-
The Laplace transform of f(t) exists for s>a, if
1. f(t) is a continuous function.
2. 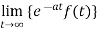 is finite.
is finite.
Important formulae-
1. 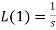
2. 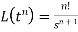
3. 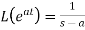
4. 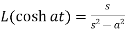
5. 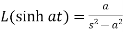
6. 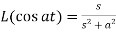
7. 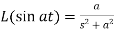
Example-1: Find the Laplace transform of the following functions-
1. 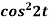 2.
2. 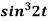
Sol. 1.
Here 
So that we can write it as-

Now-
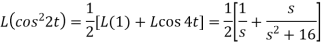
2. Since 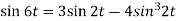
Or 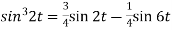
Now-
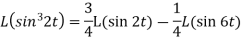
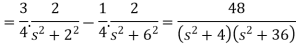
Example-2: Find the Laplace transform of (1 + cos 2t)
Sol. 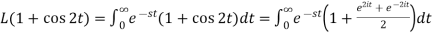
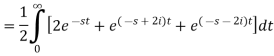
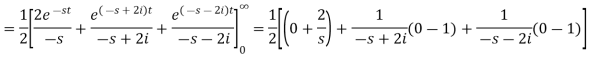
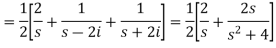
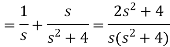
So that-
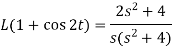
Properties and theorems of LT
1. Linearity property-
Let a and b be any two constants and 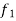 ,
, 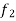 any two functions of t, then-
any two functions of t, then-
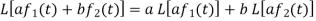
Proof: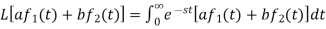
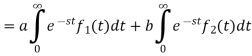
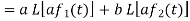
Hence proved.
2. First shifting property (Theorem)- If 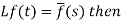
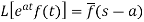
Proof: By definition-
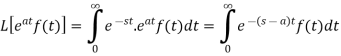
Let (s – a) = r
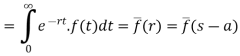
Hence proved.
We can find the following results with the help of the above theorem-
1. 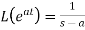
2. 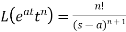
4. 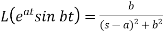
5. 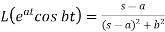
6. 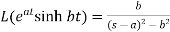
7. 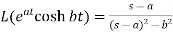
Here s>a in each case.
Example-1: Find the Laplace transform of t sin at.
Sol. Here-

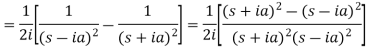
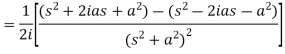
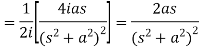
Example-2: Find the Laplace transform of 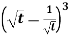
Sol. Here-
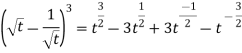
So that-


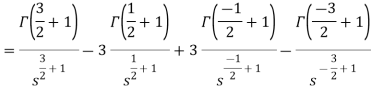
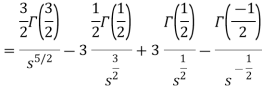
As we know that- 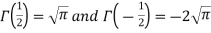
So that-
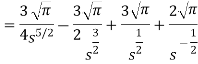
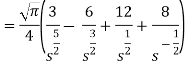
Hence-
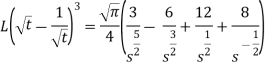
Example-3: Find the Laplace transform of the following function-
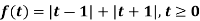
Sol. The given function f(t) can be written as-
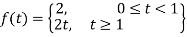
So, by definition,
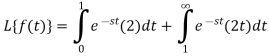
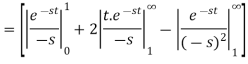
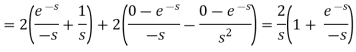
Existence theorem
The Laplace transform of f(t) exists for s>a if –
- F(t) is continuous
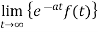 is finite.
is finite.
The above conditions are not necessary but sufficient.
Key Takeaways:
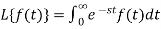
- The inverse of the Laplace transform can be defined as below-
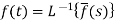
3. If 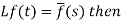
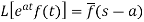
Suppose the function f(t) be periodic with period 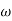 , then-
, then-
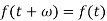
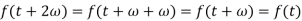
Similarly-
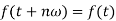
Now-
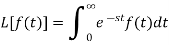
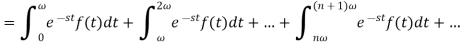
Put t = z +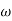 in the second integral of the above equation and t = z + 2
in the second integral of the above equation and t = z + 2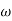 in the third integral and so on.
in the third integral and so on.
We get-
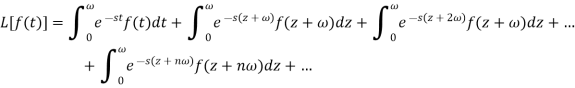
F(t) is periodic with period 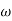 we can write-
we can write-
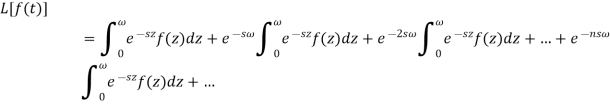
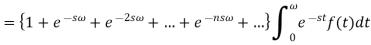
Here 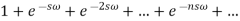 is a geometric progression with a common ratio
is a geometric progression with a common ratio 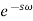 , and we know that the sum of infinite terms in G.P. Is given by
, and we know that the sum of infinite terms in G.P. Is given by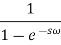
Then-
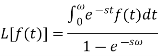
Laplace transform of periodic functions-
If f(t) is a periodic function with period T, which means f(t + T) = f(t), then-
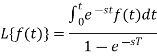
Proof: we have-
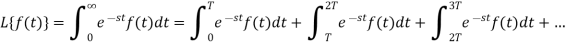
Put t = u + T in the second integral, t = u + 2T in third integral and so on…
Then-
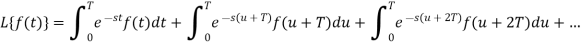
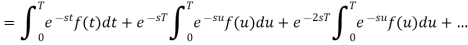
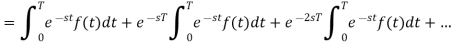
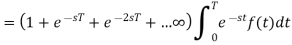
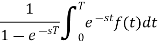
Key takeaways:
- If f(t) is a periodic function with period T, which means f(t + T) = f(t), then-
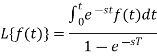
Unit step function
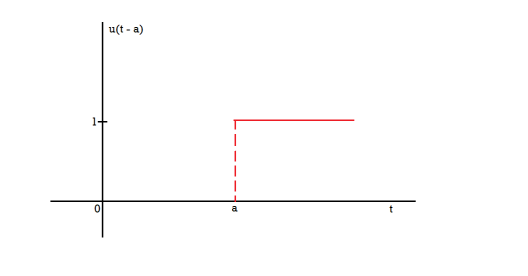
The unit step function u(t – a) is defined as-
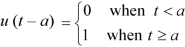
Laplace transform of unit functions-

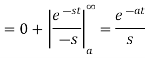
Example-1: Express the function given below in terms of a unit step function and find it's Laplace transform as well-
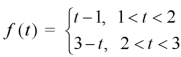
Sol. Here we are given-
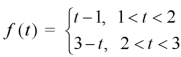
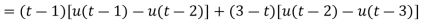
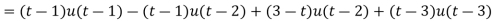
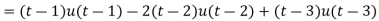
So that-
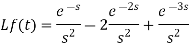
Example-2: Find the Laplace transform of the following function by using unit step function-

Sol. 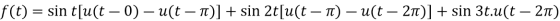
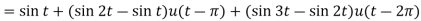
Since 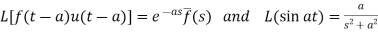

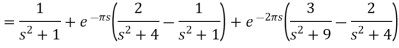
Key takeaways:
- The unit step function u(t – a) is defined as-
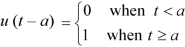
2. 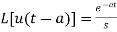
The impulse function is also known as the Dirac-delta function.
Impulse- When a large force acts for a short time, then the product of the force and the time is called impulse.
The unit impulse function is the limiting function.
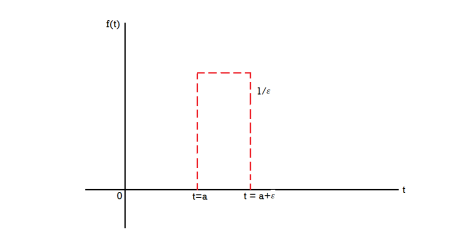
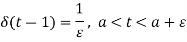
= 0, otherwise
The unit impulse function can be defined as-
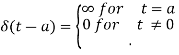
And
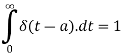
Laplace transform of unit impulse function-
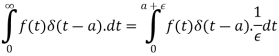
We know that-
Mean value theorem- 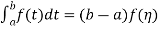
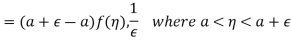
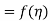
As 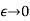 , then we get-
, then we get- 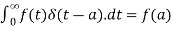
When 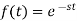 then we have
then we have 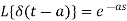
Example-1: Evaluate-
Sol.1. As we know that- 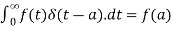
So that-

2. As we know that-

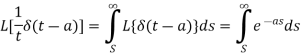
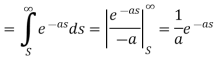
Example-2: 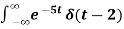
Sol. 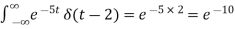
Key takeaways:
- When a large force acts for a short time, then the product of the force and the time is called impulse.
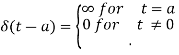

Inverse Laplace transforms-
The inverse of the Laplace transform can be defined as below-

Here 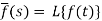
f(t) is called the inverse Laplace transform of 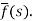
L is called the Laplace transformation operator.
Important formulae-
1. 
2. 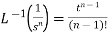
3. 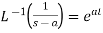
4. 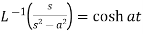
5. 
6. 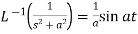
7. 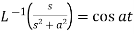 8.
8. 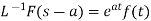
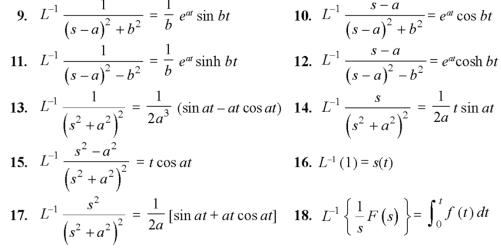
Example: Find the inverse Laplace transform of the following functions-
1. 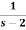
2. 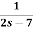
Sol.
1.
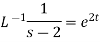
2.
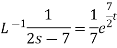
Example: Find the inverse Laplace transform of-
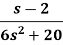
Sol.
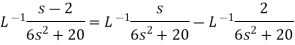
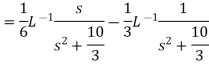
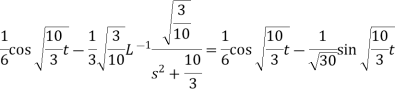
Multiplication by ‘s’ -
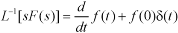
Example: Find the inverse Laplace transform of-
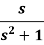
Sol.
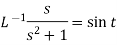
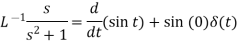
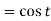
Division by s-
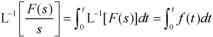
Example: Find the inverse Laplace transform of-
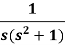
Sol.
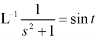
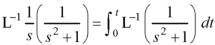
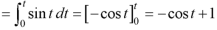
Inverse Laplace transform of derivative-
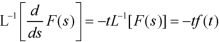
Example: Find 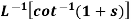
Sol.
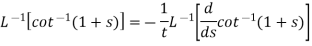
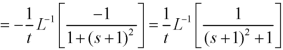
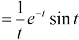
Inverse Laplace transform by using partial fraction
We can find the inverse Laplace transform by using the partial fractions method described below-
Example: Find the Laplace inverse of-
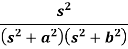
Sol.
We will convert the function into partial fractions-

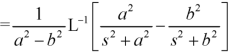
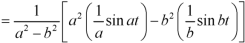
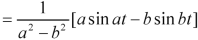
Example: Find the inverse transform of-
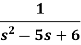
Sol.
First, we will convert it into partial fractions-
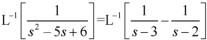
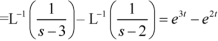
Inverse Laplace transform by convolution theorem-
According to the convolution theorem-
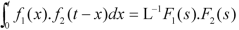
Example: Find
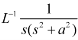
Sol.
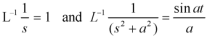
Therefore by the convolution theorem-
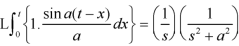

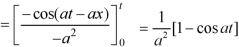
Convolution theorem
If 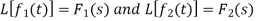
Then
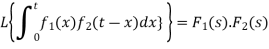
Proof:
We have
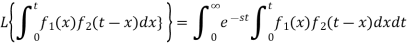
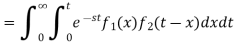
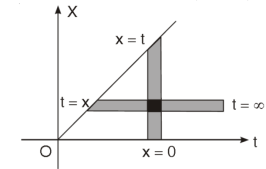
Where the double integral is taken over the infinite region in the first quadrant lying between the lines x = 0 and x = t.
If we change the order of integration, the above integrals changes to-
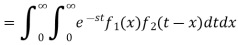
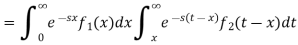
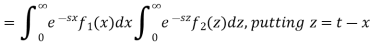
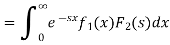

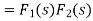
Hence proved.
Example: Apply convolution theorem to evaluate-
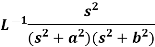
Sol.
Since
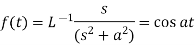

By convolution theorem, we get-
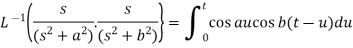
Because- f(u) = cos au, g(t-u) = cos b(t – u)
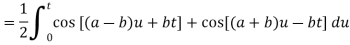
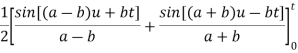
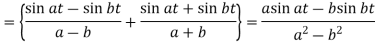
Key takeaways:
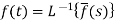
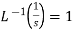 2.
2. 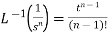
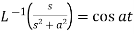 8.
8. 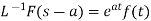
- If
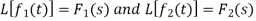
Then

Step by step procedure to solve a linear differential equation by using Laplace transform-
1. Take the Laplace transform of both sides of the given differential equation.
2. Transpose the terms with a negative sign to the right.
3. Divide by the coefficient of 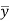 , getting
, getting 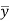 as a known function of s.
as a known function of s.
4. Resolve the function of s into partial fractions and take the inverse transform of both sides.
We will get y as a function of t. Which is the required solution.
Example-1: Use Laplace transform method to solve the following equation-
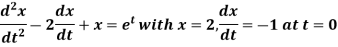
Sol. Here we have-
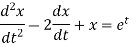
Take Laplace transform of both sides, we get-
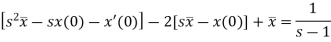
It becomes-
(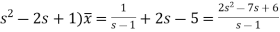
So that-
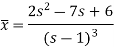
Now breaking it into partial fractions-
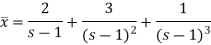
We get the following results on inversion-
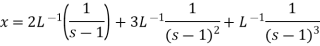
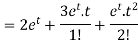
Example-2: Use Laplace transform method to solve the following equation-
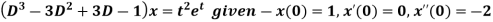
Sol.
Here, taking the Laplace transform of both sides, we get
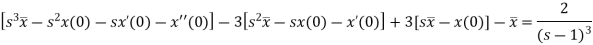
It becomes-
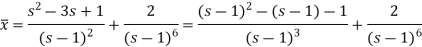
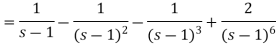
On inversion, we get-
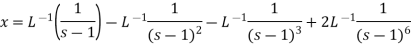
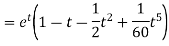
Example-3: Use Laplace transform method to solve the following equation-
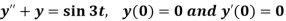
Sol. Here we have-
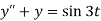
Taking Laplace transform of both sides, we get-
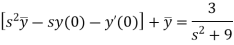
We get on putting given values-
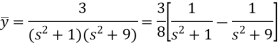
On inversion, we get-
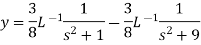

Example-4: Find the solution of the initial value problem by using Laplace transform-
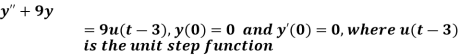
Sol. Here we have-
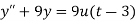
Taking Laplace transform, we get-
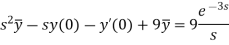
Putting the given values, we get-
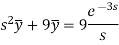
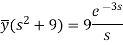
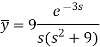
On inversion, we get-
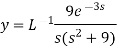
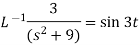
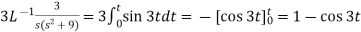 4
4
Now-
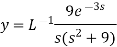
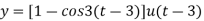
The solution of simultaneous differential equations by using Laplace transform-
Example: solve the following differential equation by using Laplace transform-
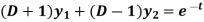
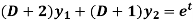
Here D = d/dt and 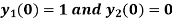
Sol.
Here we have-
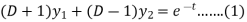
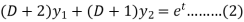
Now multiply (1) by D+1 and (2) by D – 1 we get-
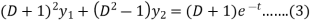
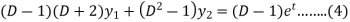
Now subtract (4) from (3), we get-
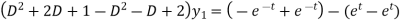
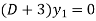
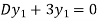
By taking Laplace to transform we get-
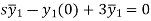
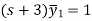
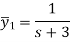
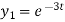
Put the value of 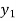 in (1) we get-
in (1) we get-
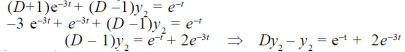
By taking Laplace to transform we get-
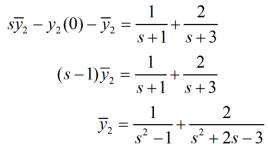
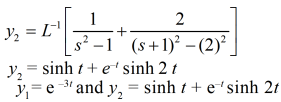
Which is the required answer.
References:
1. R. J. Beerends H. G. Ter Morsche, J. C. Van Den Berg. L. M. Van De Vrie, Fourier and Laplace Transforms, Cambridge University Press.
2. Sastry S.S. Introductory Methods of Numerical Analysis, PHI.
3. B.S. Grewal: Higher Engineering Mathematics; Khanna Publishers, New Delhi.
4. B.V. Ramana: Higher Engineering Mathematics; Tata McGraw- Hill Publishing Company Limited, New Delhi.
5. Peter V.O’ Neil. Advanced Engineering Mathematics, Thomas (Cengage) Learning.