Unit - 1
Fluids and Their Properties
Mechanics is the oldest physical science, dealing with both fixed and moving entities subjected to forces. Statics is the branch of mechanics that deals with bodies at rest, whereas dynamics is the branch that deals with moving bodies. The study of bodies in motion is known as dynamics. Fluid mechanics is a subcategory. Defined as the science of the behaviour of fluids at rest (fluid statics) or in motion (fluid dynamics), as well as fluid-solid interactions or other fluids at the edges Fluid mechanics is also known as fluid dynamics. Dynamics by treating fluids at rest as a particular example of motion with zero v Fluid mechanics is separated into various categories as well. Hydrodynamics is the study of the motion of fluids that are virtually incompressible (such as liquids, particularly water, and gases at low speeds).
Hydraulics is a subfield of hydrodynamics that deals with liquid flows in pipes and open channels. Gas dynamics is concerned with the flow of fluids that undergo considerable density changes, such as the flow of gases through high-speed nozzles. Aerodynamics is concerned with the movement of gases (particularly air) across things such as aeroplanes, rockets, and vehicles at high or low speeds velocity. Other subfields, such as meteorology, oceanography, and hydrology, deal with naturally occurring flows.
As you may know from physics, a substance exists in three basic states: solid, liquid, and gas. (It also occurs as plasma at very high temperatures.)
A fluid is a material that is in the liquid or gas phase. The capacity of a material to resist an applied shear (or tangential) stress that tends to change its form distinguishes it from a fluid. A solid can deform in response to applied shear stress, but a fluid deforms continually under the effect of shear stress, no matter how little. Stress in solids is proportional to strain, whereas stress in fluids is proportional to strain rate.
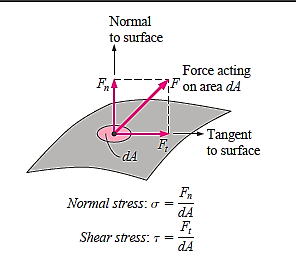
In statics, stress is defined as force per unit area and is calculated by dividing the force by the area on which it operates. Normal stress is the normal component of a force acting on a surface per unit area, while shear stress is the tangential component of a force acting on a surface per unit area. The typical tension in a fluid at rest is referred to as pressure. Because the supporting walls of a fluid minimise shear stress, a fluid at rest has zero shear stress. When the walls are removed or a liquid container is tilted, shear develops and the liquid spills or travels in order to achieve a horizontal free surface.
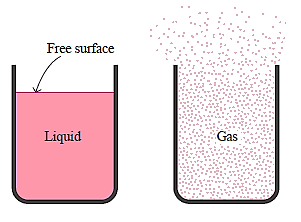
Because of the strong cohesive interactions between the molecules, pieces of molecules can move relative to one other in a liquid, but the volume remains essentially constant. As a result, in a gravitational field, a liquid adopts the shape of the container it is in and creates a free surface in a bigger container. A gas, on the other hand, expands until it comes into contact with the container's walls and fills the whole available area. This is due to the fact that the gas molecules are widely dispersed and the cohesive interactions between them are relatively weak. Gases, unlike liquids, cannot form a free surface.
- Viscous versus Inviscid Flow
When two fluid layers travel in opposite directions, a friction force arises between them, and the slower layer attempts to slow down the faster layer. The fluid property viscosity, which measures the fluid's internal stickiness, quantifies this internal resistance to flow. Cohesive interactions between molecules in liquids and molecular collisions in gases generate viscosity. Because there is no such thing as a fluid with zero viscosity, all fluid flows entail viscous effects to some extent.
Viscous flows are those in which frictional effects are considerable. However, in many real flows, there are places (usually those not near to solid surfaces) where viscous forces are negligibly tiny in comparison to inertial or pressure forces. In such inviscid flow zones, ignoring the viscous factors considerably simplifies the analysis without sacrificing much accuracy.
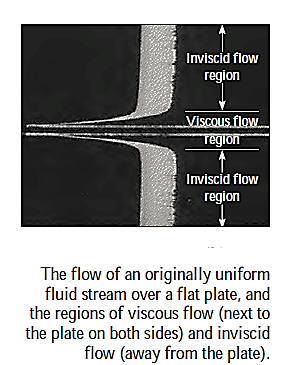
Figure depicts the formation of viscous and inviscid flow areas as a result of putting a flat plate parallel into a uniform velocity fluid stream. Because of the no-slip condition, the fluid adheres to the plate on both sides, and the viscous flow zone is the thin boundary layer at the plate surface where viscous effects are substantial. The inviscid flow area is the region of flow on both sides away from the plate that is unaffected by the presence of the plate.
- Internal versus External Flow
Depending on whether the fluid is pushed to flow in a limited channel or over a surface, a fluid flow is characterised as internal or external.
External flow is the flow of an unbounded fluid over a surface such as a plate, a wire, or a pipe. Internal flow occurs when a fluid is totally limited by solid surfaces in a pipe or duct. Water flow in a pipe, for example, is an example of internal flow, but airflow over a ball or over an open pipe on a windy day is an example of external flow.
If the duct is only half filled with liquid and there is a free surface, the flow is called open-channel flow. Water flows in rivers and irrigation canals are two examples of such flows.
Throughout the flow field, the impact of viscosity dominates internal flows. The viscous effects in external flows are restricted to boundary layers near solid surfaces and wake areas downstream of bodies.
- Compressible versus Incompressible Flow
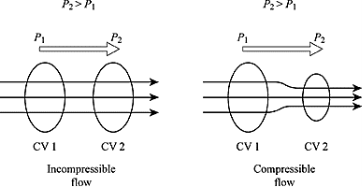
Depending on the degree of density fluctuation during flow, a flow is characterised as compressible or incompressible. Incompressibility is a rough approximation; a flow is considered to be incompressible if the density remains roughly constant throughout. When the flow (or the fluid) is incompressible, the volume of every section of the fluid remains constant during the duration of its motion.
Because liquid densities are largely constant, liquid flow is often incompressible. As a result, liquids are often referred to as incompressible substances. A pressure of 210 atm, for example, changes the density of liquid water at 1 atm by only 1%. Gases, on the other hand, may be compressed quite easily. A pressure shift of 0.01 atm, for example, generates a 1% change in atmospheric air density.
When evaluating rockets, spacecraft, and other systems with high-speed gas flows, the flow speed is frequently stated in terms of the dimensionless Mach number, which is defined as
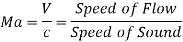
Where c is the speed of sound whose value is 346 m/s in air at room temperature
At sea level. A flow is called sonic when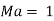 
, subsonic when 
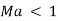, supersonic when 
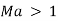, and hypersonic when 
.
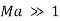To a great degree of precision, liquid flows are incompressible, but the degree of change in density in gas flows, and hence the amount of approximation used when modelling gas flows as incompressible, depends on the Mach number. Gas flows are frequently approximated as incompressible if density variations are less than roughly 5%, which is commonly the case when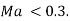 
As a result, at speeds less than 100 m/s, the compressibility effects of air may be ignored. It is important to note that a gas flow is not always a compressible flow. Small changes in liquid density correlating to huge pressure changes can nonetheless have significant repercussions. The annoying "water hammer" in a water pipe, for example, is created by vibrations in the pipe induced by the reflection of pressure waves when the valves are suddenly closed.
- Laminar versus Turbulent Flow
Some flows are smooth and organised, whilst others are chaotic. Laminar fluid motion is defined as highly organised fluid motion characterised by smooth layers of fluid. The term "laminar" refers to the movement of neighbouring fluid particles in "laminates." At low velocities, the flow of high-viscosity fluids such as oils is often laminar. Turbulent fluid motion is defined as highly disordered fluid motion that happens at high speeds and is characterised by velocity variations.
At high velocities, the flow of low-viscosity fluids such as air is often turbulent. The necessary power for pumping is heavily influenced by the flow regime. A transitional flow is one that fluctuates between being laminar and turbulent. Osborn Reynolds' work in the 1880s resulted in the dimensionless Reynolds number, Re, being established as the primary metric for determining the flow regime in pipes.
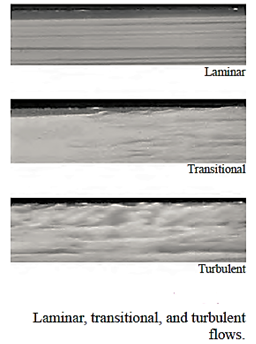
- Natural (or Unforced) versus Forced Flow
Depending on how the fluid motion is begun, a fluid flow is considered to be natural or forced. Forced flow occurs when a fluid is forced to flow over a surface or through a pipe by an external force such as a pump or a fan. Any fluid motion in natural flows is caused by natural forces such as the buoyancy effect, which expresses itself as the rise of warmer (and therefore lighter) fluid and the fall of cooler (and thus denser) fluid. The thermosiphoning effect, for example, is widely employed to replace pumps in solar hot-water systems by positioning the water tank sufficiently above the solar collectors.
- Steady versus Unsteady (Transient) Flow
Because the phrases steady and uniform are frequently used in engineering, it is critical to grasp their definitions. The phrase steady suggests that there is no change at a certain moment in time. Unsteady is the inverse of steady. The phrase uniform suggests that there is no variation with location within a certain region. These definitions are congruent with their common usage (steady girlfriend, uniform distribution, and so forth).
Although the phrases unsteady and transitory are frequently used interchangeably, they are not synonyms. Unsteady is the most generic word in fluid mechanics for any flow that is not steady, while transitory is often used for developing flows. When a rocket engine is ignited, for example, there are transitory effects (pressure rises up inside the rocket engine, flow increases, and so on) until the engine settles down and functions steadily. The word periodic flow refers to an unstable flow that oscillates around a steady mean.
Many devices, such as turbines, compressors, boilers, condensers, and heat exchangers, run under the same conditions for extended periods of time and are classed as steady-flow equipment. (It should be noted that the flow field near the moving blades of a turbomachine is unstable, but when classifying devices, we evaluate the total flow field rather than the details at particular places.) The fluid characteristics of a device can fluctuate from point to point during steady flow, but they stay constant at any given location. As a result, in steady operation, the volume, mass, and total energy content of a steady-flow device or flow section stay constant.
Devices designed for continuous operation, such as turbines, pumps, boilers, condensers, and heat exchangers in power plants or refrigeration systems, can closely simulate steady-flow conditions. Some cyclic devices, such as reciprocating engines or compressors, may not meet the steady-flow criteria because the flow at the inlets and exits is pulsing rather than steady. The fluid characteristics, on the other hand, fluctuate with time in a periodic fashion, and the flow through these devices may still be evaluated as a steady-flow process by utilising time-averaged values for the attributes.
- One-, Two-, and Three-Dimensional Flows
The velocity distribution best characterises a flow field, and so a flow is considered to be one-, two-, or three-dimensional if the flow velocity changes in one, two, or three principal dimensions, respectively. A normal fluid flow has a three-dimensional geometry, and the velocity can change in all three dimensions, causing the flow to be three-dimensional.
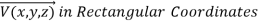
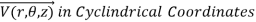
However, if the variation in velocity in one direction is modest in comparison to the variance in another, it can be ignored with little inaccuracy. In such circumstances, the flow may be readily described as one- or two-dimensional, making it easier to evaluate.
It should be noted that the dimensionality of the flow is also affected by the coordinate system chosen and its orientation. Also, because of the no-slip criterion, the velocity cannot be uniform across the cross section of the pipe even in this basic flow. However, at a well-rounded pipe entry, the velocity profile may be estimated as almost uniform across the pipe, because velocity is essentially constant at all radii except extremely close to the pipe wall.
When the aspect ratio is large and the flow does not change much over the longer dimension, a flow can be represented as two-dimensional.
In the gas phase, matter is made up of atoms that are widely dispersed. However, it is highly easy to dismiss a substance's atomic nature and see it as a continuous, homogenous mass with no holes, i.e. a continuum. Using the continuum idealisation, we may consider qualities as point functions and assume that they fluctuate continuously in space with no jump discontinuities.
This idealisation holds true as long as the size of the system in question is big in comparison to the space between the molecules.
Except for a few specific issues, this is true for almost all problems. Many of our claims, such as "the density of water in a glass is the same at every position," are implicit in the continuum idealisation.
Consider a container filled with oxygen under atmospheric conditions to get a feel of the distances involved at the molecular level.
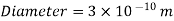
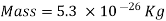
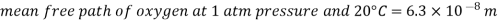
That is, an oxygen molecule travels, on average, a distance of 6.3×10^(-8) m (about 200 times its diameter) before it collides with another molecule.
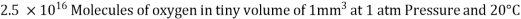
The continuum model is valid as long as the system's characteristic length (such as its diameter) is significantly greater than the mean free path of the molecules. The mean free path can grow quite enormous at very high vacuums or very high heights (for example, atmospheric air at 100 km elevation has a mean free path of around 0.1 m). In such instances, the rarefied gas flow theory should be applied, with the influence of individual molecules considered.
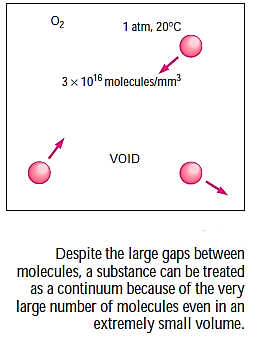
Consider a fluid layer separated by a distance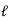 
between two extremely big parallel plates (or, equivalently, two parallel plates submerged in a vast body of a fluid). The top plate is now subjected to a constant parallel force F while the bottom plate remains stationary.
Following the first transients, the top plate travels continually under the influence of this force at a constant velocity V. The fluid in contact with the top plate adheres to the plate surface and flows at the same velocity as it, and the shear stress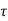 
acting on this fluid layer is
Consider the movement of a fluid across a plate's surface. The fluid layer in contact with the surface will use friction to draw the plate along, putting a friction force on it. Similarly, due of the friction between the two layers, a quicker fluid layer will try to drag the neighbouring slower layer and impose a friction force. Shear stress is defined as friction force per unit area and is denoted by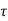 
.
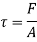
Where A denotes the area of contact between the plate and the fluid. It is worth noting that the fluid layer deforms continually as a result of shear stress.
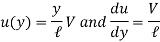
Where y is the vertical distance from the lower plate.
During a differential time, interval dt, the sides of fluid particles along a
Vertical line MN rotate through a differential angle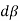 
while the upper plate
Moves a differential distance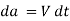 
. The angular displacement or deformation (or shear strain) can be expressed as,
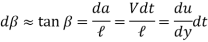
Rearranging, the rate of deformation under the influence of shear stress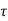 
Becomes
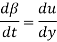
As a result, we infer that a fluid element's rate of deformation is identical to the velocity gradient du/dy. Furthermore, it can be demonstrated experimentally that the rate of deformation (and consequently the velocity gradient) is exactly proportional to the shear stress in most fluids.
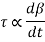
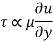
Newtonian fluids are fluids in which the rate of deformation is proportional to the shear stress. Newtonian fluids include most ordinary fluids such as water, air, gasoline, and oils. Non-Newtonian fluids include blood and liquid polymers. Shear stress may be described linearly in one-dimensional shear flow of Newtonian fluids.
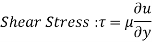
For most fluids, shear stress is proportional to velocity gradient, and shear stress at the wall surface is as where the constant of proportionality is termed the fluid's dynamic viscosity.
The fluids that that obey the linear relationship above are called Newtonian fluids,
In fluid flow and heat transfer studies, the ratio of dynamic viscosity to density appears frequently. For convenience, this ratio is given the name kinematic viscosity,
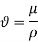
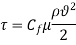
Where Cf is the dimensionless friction coefficient.
The shear force operating on a Newtonian fluid layer (or, according to Newton's third law, the force acting on the plate) is defined as
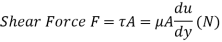
There, once more A denotes the area of contact between the plate and the fluid. The force F necessary to move the top plate at a constant velocity of V while the bottom plate remains stationary is then calculated.
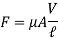
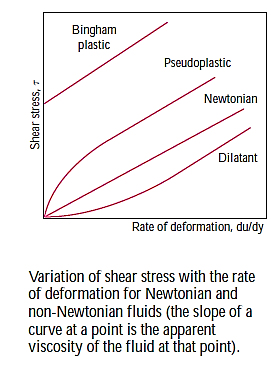
Fluids with dilatant or shear thickening behaviour (the fluid becoming less viscous as it is sheared harder, such as some paints, polymer solutions, and fluids with suspended particles) have an apparent viscosity that increases with the rate of deformation. Fluids with pseudoplastic or shear thinning behaviour (the fluid becoming less viscous as it is sheared harder, such as some paints, polymer solutions, and fluids with suspended particles) have the opposite behaviour (the Some materials, such as toothpaste, can withstand a finite shear stress and so act as a solid, yet continually deform when the shear stress exceeds the yield stress and hence behave as a fluid. Bingham plastics are the name given to such materials.
Newtonian Fluid (Fluid Mechanics)
- The Newtonian fluid is the fluid in which the shear stress is directly proportional to the rate of shear strain or velocity gradient.
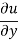
- All gases are Newtonian fluids. E.g.: Glycerine, kerosene. Air, water, alcohol etc.
- The linear relationship between shear stress and the rate of deformation is called as Newtonian fluid.
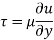
Non-Newtonian Fluid (Rheology)
- The fluid in which the shear stress is not directly proportional to the rate of shear strain or Velocity gradient, is called as Non-Newtonian Fluid.
- e.g., Slurries, toothpaste, gels, polymer solution paint, lubricant oils.
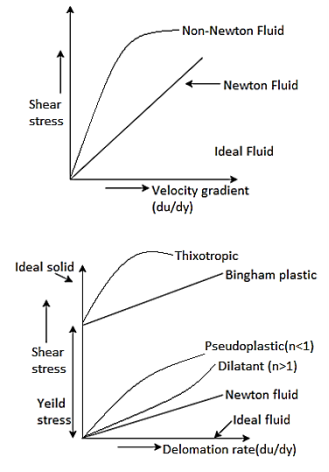
- The non-linear relationship between shear stress and the rate of deformation is called as non-Newtonian fluid.
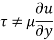
Pseudo plastic Fluid or Shear Thinning Fluid:
- Fluid in which the apparent viscosity decreases with increasing deformation rate (n< 1) is called as Pseudoplastic fluid.
- Examples are slurries, mud, polymer solution, quicksand, gums, blood, milk, colloidal suspensions paper pulp in water, ketchup.
Dilatant Fluid or Shear Thickening fluid:
- Fluid in which the apparent viscosity increases with increasing deformation rate (n>1) is called as dilatant fluids.
- e.g., Suspension of sand, starch, butter, sugar solution.
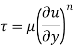
Bingham Plastic Fluid
- The fluid which possesses a definite yield stress but then the relationship between shear stress and angular deformation is linear is called as Bingham plastic fluid.
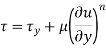
- e.g., Sewage sludge, toothpaste, oil paint, jellies, applesauce, drilling mud.
Thixotropic Fluid or Plastic Fluid:
- The fluid which possesses a definite yield stress but then the relationship between shear stress and angular deformation is nonlinear are called as Thixotropic fluid. e.g., Printer ink, lipstick.
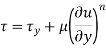
Ideal Fluid:
The fluid which has zero viscosity er shear stress is always zero is called as ideal fluid, that's why ideal fluid is represented by the horizontal axis.
Key Takeaway:
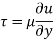
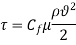
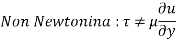
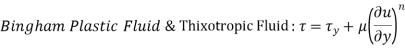
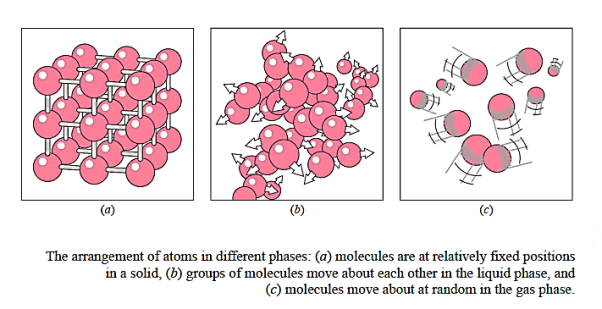
Solids have the strongest intermolecular bonds, whereas gases have the weakest. One reason for this is because molecules in solids are densely packed together, whereas molecules in gases are separated by comparatively great distances.
A solid's molecules are organised in a pattern that is repeated throughout.
Because of the tiny distances between molecules in a solid, the attractive forces between molecules are strong, keeping the molecules in fixed locations. The molecular spacing in the liquid phase is like that in the solid phase, except that the molecules are no longer in fixed locations relative to each other and can freely spin and translate. The intermolecular forces in a liquid are smaller than in a solid but remain substantial when compared to gases. Water is an exception to the rule that the distances between molecules increase somewhat as a solid turn’s liquid.
The molecules in the gas phase are widely away from one another, and there is no molecular order. Gas molecules move randomly, hitting with one another and the walls of the container in which they are confined. Intermolecular forces are extremely weak, especially at low concentrations, and collisions are the sole way for molecules to interact.
Those in the gas phase have a far greater energy level than molecules in the liquid or solid phases. As a result, the gas must expend a significant amount of energy before it can condense or freeze.
Gas and vapour are frequently used interchangeably. When a substance's vapour phase is above the critical temperature, it is commonly referred to as a gas.
Vapor generally refers to a gas that is on the verge of condensing.
Any realistic fluid system is made up of a huge number of molecules, and the system's attributes are naturally determined by the behaviour of these molecules.
Density is defined as mass per unit volume
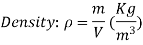
The reciprocal of density is the specific volume 
, which is defined as volume per unit mass
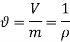
For a differential volume element of mass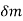 
& 
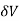
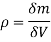
In general, the density of a material is affected by temperature and pressure.
Most gases have a density that is proportional to pressure and inversely proportional to temperature. Liquids and solids, on the other hand, are basically incompressible substances with minor fluctuation in density with pressure.
The density of a material is sometimes provided relative to the density of a well-known substance. Then it is referred to as specific gravity, or relative density, and is defined as the ratio of a material's density to the density of some reference substance at a certain temperature.
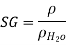
Substances having specific gravities less than one float on water because they are lighter than water.
Specific weight is the weight of a unit volume of a material and is stated as
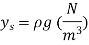
Key Takeaway:
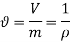
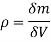
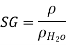
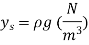
Viscosity may be defined as the property of fluid which determines its resistance to shearing stresses. It is a measure of the internal fluid friction which causes resistance to flow.
Viscosity of fluids is due to cohesion and interaction between particles.
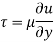
= Constant of proportionality and is known as co-efficiency of dynamic viscosity.
Du/dy = Rate of shear stress or rate of shear deformation or velocity gradient.
We have,
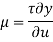
Thus, viscosity may also be defined as the shear stress required to produce unit rate of shear strain.
Units Of Viscosity:
In S.I. Units: N.s/m2
Kinematic Viscosity:
Kinematic Viscosity is defined as the ratio between the dynamic viscosity and density of fluid. It is denoted by v (called nu).
v = Viscosity / Density
Units Of Kinematic Viscosity:
In SI units: m2/s
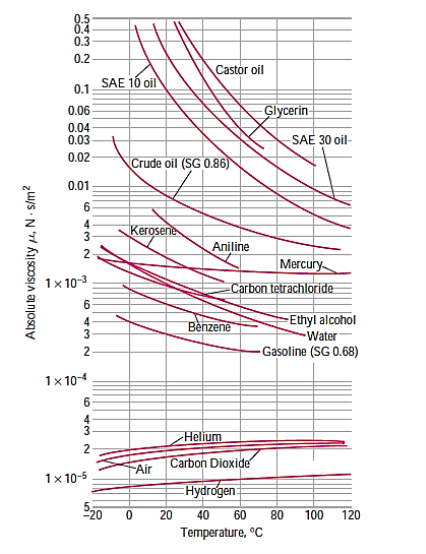
In general, the viscosity of a fluid is affected by both temperature and pressure, albeit the influence of pressure is rather minor. Except at extremely high pressures, the dynamic and kinematic viscosities of liquids are virtually independent of pressure, and any tiny fluctuation with pressure is typically ignored. This is also true for dynamic viscosity (at low to moderate pressures) for gases, but not for kinematic viscosity, because a gas's density is proportional to its pressure.
A fluid's viscosity is a measure of its "resistance to deformation."
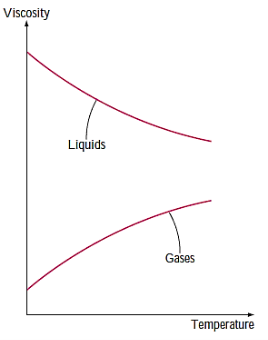
The internal frictional force that develops between distinct layers of fluids when they are compelled to move relative to one other causes viscosity. The cohesive forces between molecules in liquids and molecular collisions in gases generate viscosity, which varies dramatically with temperature. The viscosity of liquids reduces as temperature rises, but the viscosity of gases rises as temperature rises.
This is because at higher temperatures, the molecules in a liquid have more energy and may more firmly fight the massive cohesive intermolecular forces. As a result, the electrified liquid molecules have greater freedom to move.
In a gas, on the other hand, intermolecular interactions are insignificant, and gas molecules move randomly at increasing velocities at high temperatures.
As a result, there are more molecule collisions per unit volume per unit time, resulting in increased flow resistance.
The viscosity of a fluid is proportional to the amount of pumping force required to move a fluid in a pipe or a body (such as an automobile in the air or a submarine in the sea) through a fluid.
According to the kinetic theory of gases, the viscosity of gases is related to the square root of temperature.
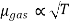
Practical observations validate this prediction, however differences for various gases must be compensated for by integrating some correction factors. The Sutherland correlation (from The United States Standard Atmosphere) expresses gas viscosity as a function of temperature as
Gases:
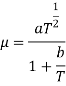
Where T denotes absolute temperature and a and b denote experimentally established constants It's worth noting that measuring viscosities at two distinct temperatures is enough to calculate these constants.
For air, the values of these constants
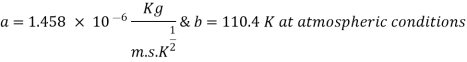
At low to moderate pressures, gas viscosity is pressure independent (from a few percent of 1 atm to several atm). However, viscosity rises at high pressures owing to density increase.
For liquids, the viscosity is approximated as
Liquids:
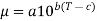
Where again T is absolute temperature and a, b, and c are experimentally
Determined constants.
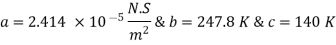
Results in less than 2.5 percent error in viscosity
In the temperature range of 0°C to 370°
Key Takeaway:
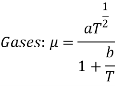
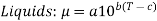
A drop of blood forms a hump on a horizontal glass; a drop of mercury forms a near-perfect sphere and can be rolled over a smooth surface just like a steel ball; water droplets from rain or dew hang from branches or leaves of trees; liquid fuel injected into an engine forms a mist of spherical droplets; water dripping from a leaky faucet falls as spherical droplets; a soap bubble released into the air forms.
In these and other observations, liquid droplets behave like little spherical balloons filled with the liquid, and the liquid's surface behaves like a stretched elastic membrane under stress. The attraction interactions between the liquid molecules generate the pulling force that causes this tension to act parallel to the surface. Surface tension is the magnitude of this force per unit length.
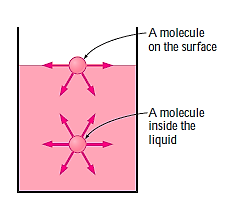
Consider two liquid molecules, one on the surface and the other deep into the liquid body. Because of symmetry, the attractive forces exerted on the inside molecule by the surrounding molecules balance each other. However, the attractive forces exerted on the surface molecule are not symmetric, and the attractive forces provided by the gas molecules above are often negligible. As a result, there is a net attractive force acting on the molecule at the liquid's surface, which tends to drag the molecules on the surface into the liquid's centre.
This force is countered by the repulsive forces generated by the compressed molecules under the surface.
As a result of the compression effect, the liquid's surface area is reduced. This explains why liquid droplets prefer to take on a spherical form, which has the least surface area for a given volume.
Consider a liquid film (such as the film of a soap bubble) hung on a U-shaped wire frame with a moveable side to better comprehend the surface tension effect. Normally, the liquid film draws the moveable wire inward to reduce its surface area. To counteract this pulling action, a force F must be supplied to the moveable wire in the opposite direction. Because the thin film in the device has two sides accessible to air (the top and bottom surfaces), the length along which the tension operates in this situation is 2b.
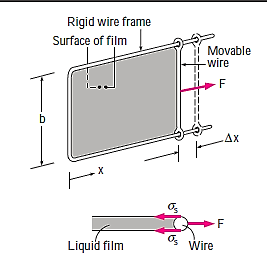
Then a force balance on the movable wire gives
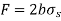
Thus, surface Tension,
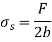
The force F stays constant in the U-shaped wire while the moveable wire is pushed to stretch the film and increase its surface area.
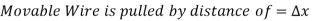
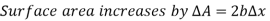
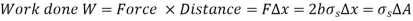
Because the force is constant in this scenario. This finding may alternatively be understood as the film's surface energy increasing by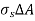 
during the stretching process, which is consistent with the alternate interpretation of 
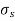as surface energy. As a result, surface tension may alternatively be defined as the work done per unit increase in the liquid's surface area.
The surface tension of a material changes substantially depending on its composition and temperature. Mercury droplets produce spherical balls that can be rolled on a surface like a solid ball without soaking it.
In general, the surface tension of a liquid falls with temperature until it reaches zero at the critical point (thus, there is no distinguishable liquid–vapor barrier at temperatures above the critical point). Pressure has a minor influence on surface tension.
Impurities can significantly alter a substance's surface tension. As a result, some compounds known as surfactants can be added to a liquid to reduce its surface tension. Soaps and detergents, for example, reduce the surface tension of water and allow it to permeate through the microscopic gaps between fibres for more efficient cleaning. However, because of poor craftsmanship, equipment whose performance is dependent on surface tension (such as heat pipes) might be ruined by the presence of contaminants.
Only at liquid–liquid or liquid–gas interfaces do we talk about surface tension for liquids. As a result, while defining surface tension, it is critical to include the neighbouring liquid or gas. Surface tension also influences the size of the liquid droplets that form. A droplet that continues to develop by adding additional mass will collapse when the surface tension can no longer hold it together. This is analogous to a balloon that will explode during inflation if the pressure within exceeds the strength of the balloon material.
A curved interface denotes a pressure differential (or "pressure jump") across the interface, with pressure greater on the concave side. Consider the free-body diagram of half a droplet or bubble to estimate the surplus pressure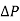 
inside a droplet or bubble above atmospheric pressure. Given that surface tension works around the circumference and pressure acts on the area, horizontal force balances for the droplet and bubble result in
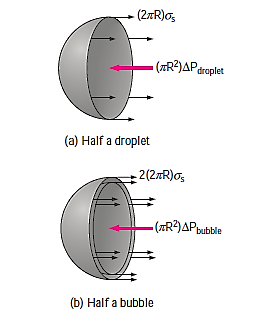
Where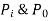 
denotes the pressures inside and outside the droplet or bubble. 
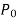is just atmospheric pressure while the droplet or bubble is in the atmosphere. The bubble has a factor 2 in the force balance because it is made up of a film with two surfaces (inner and outer surfaces) and hence two circumferences in the cross section.
Excess pressure in a droplet (or bubble) may also be calculated by taking into account a differential increase in the radius of the droplet owing to the addition of a differential quantity of mass and interpreting the surface tension as an increase in surface energy per unit area. The rise in the surface energy of the droplet as a result of this differential expansion process is thus
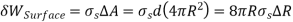
The expansion work performed during this differential process is calculated by multiplying the force by the distance.
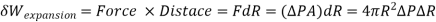
Equating the two expressions above gives
Key Takeaway:
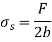
The capillary effect, which is the rise or fall of a liquid in a small-diameter tube put into the liquid, is another intriguing result of surface tension. Capillaries are narrow tubes or limited flow pathways.
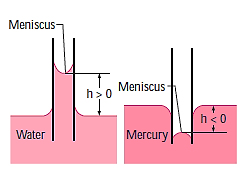
This phenomenon is responsible for the ascent of kerosene through a cotton wick put into the reservoir of a kerosene lamp. The capillary effect is also responsible for water rising to the tops of tall trees. The meniscus is the curved free surface of a liquid in a capillary tube.
Water in a glass container is usually observed to curve up somewhat at the margins where it hits the glass surface; however, mercury does the opposite: it bends down at the edges. This phenomenon is commonly represented by stating that water wets the glass (by adhering to it), but mercury does not.
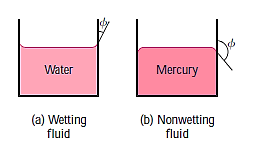
The contact (or wetting) angle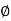 
, defined as the angle formed by the tangent to the liquid surface with the solid surface at the point of contact, quantifies the strength of the capillary action. The surface tension force acts toward the solid surface along this tangent line.
When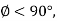 
a liquid is said to wet the surface, and when 
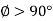, it is said not to wet the surface. The contact angle of water (and most other organic liquids) with glass in atmospheric air is almost zero, 
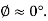As a result, the surface tension force operates upward throughout the circle of water in a glass tube, tending to pull the water up. As a result, water rises in the tube until the weight of the liquid above the reservoir's liquid level balances the surface tension force.
Microscopically, the capillary effect may be explained by considering cohesive forces (the forces between similar molecules, such as water and water) and adhesive forces (the forces between unlike molecules, such as water and glass). At the solid–liquid interface, liquid molecules are susceptible to both cohesive forces from other liquid molecules and adhesive forces from solid molecules. The magnitudes of these forces govern whether or not a liquid wet a solid surface.
Water molecules are obviously more strongly attracted to glass molecules than to other water molecules, and hence water tends to climb along the glass surface.
The converse is true for mercury, which suppresses the liquid surface towards the glass wall.
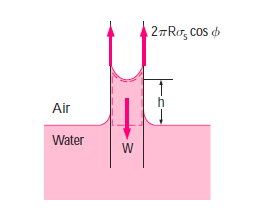
A force balance on the cylindrical liquid column of height h in the tube can be used to calculate the amount of the capillary rise in a circular tube. Because the bottom of the liquid column is at the same level as the reservoir's free surface, the pressure there must be atmospheric. As a result, the air pressure acting at the top surface is balanced, and the two effects cancel each other out.
The weight of the liquid column is approximately
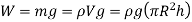
Equating the vertical component of the surface tension force to the weight gives
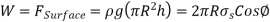
Solving for h gives the capillary rise to be,
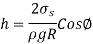
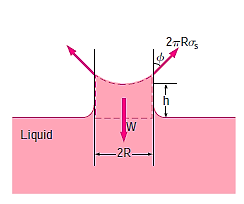
This relationship applies to nonwetting liquids (such as mercury in glass) and yields the capillary drop. In this example,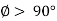 
and therefore 
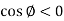, resulting in a negative h. As a result, a negative capillary rise value equates to a capillary drop.
It's worth noting that the capillary rise is inversely proportional to the tube's radius. As a result, the higher the rise (or fall) of the liquid in the tube, the thinner the tube. In reality, the capillary effect is generally minimal in tubes larger than 1 cm in diameter. When measuring pressure using manometers and barometers, it is critical to use appropriately big tubes to reduce the capillary effect.
As predicted, the capillary rise is inversely proportional to the density of the liquid. As a result, lighter liquids have larger capillary increases. Finally, keep in mind that Equation is calculated for constant-diameter tubes and should not be utilised for variable-cross section tubes.
Temperature and pressure are well-established dependent qualities for pure substances during phase-change processes, and temperatures and pressures have a one-to-one connection. The saturation temperature is the temperature at which a pure material changes phase at a given pressure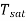 
. Similarly, the pressure at which a pure material changes phase at a particular temperature is known as the saturation pressure 
.
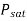The saturation temperature of water is 100°C at an absolute pressure of one standard atmosphere (1 atm or 101.325 kPa). In contrast, at 100°C, the saturation pressure of water is 1 atm.
The vapor pressure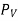 
.of a pure substance is defined as the pressure
Exerted by its vapor in phase equilibrium with its liquid at a given temperature.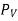 
is a property of the pure substance and turns out to be identical to the saturation pressure 
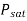of the liquid (
).
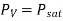We must be cautious not to mix up vapour pressure with partial pressure. The pressure of a gas or vapour in a combination with other gases is defined as partial pressure. For example, atmospheric air is a mixture of dry air and water vapour, and atmospheric pressure is the sum of dry air and water vapour partial pressures. Because air is mainly nitrogen and oxygen, the partial pressure of water vapour is a minor fraction (typically less than 3%) of the atmospheric pressure. If no liquid is present, the partial pressure of a vapour must be less than or equal to the vapour pressure. When both vapour and liquid are present and the system is in phase equilibrium, the vapour partial pressure must equal the vapour pressure, and the system is said to be saturated.
Because the vapour is pure, the saturation pressure and vapour pressure are comparable for phase-change processes between the liquid and vapour phases of a pure material. It should be noted that the pressure value is the same whether measured in the vapour or liquid phase (given that it is recorded near to the liquid–vapor contact to prevent hydrostatic effects). Temperature causes a rise in vapour pressure. As a result, at greater temperatures, a material boils at higher pressures.
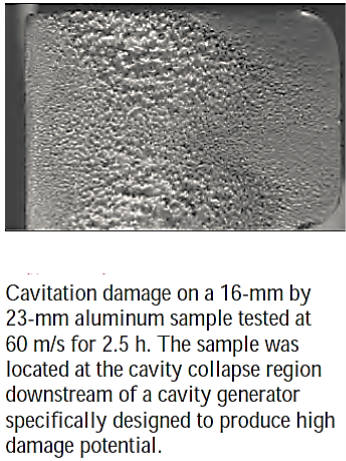
The reason for our interest in vapour pressure is the likelihood that the liquid pressure in liquid-flow systems will drop below the vapour pressure at some points, resulting in unintended vaporisation. Water at 10°C, for example, will flash into vapour and create bubbles if the pressure drops below 1.23 kPa (such as the tip regions of impellers or the suction sides of pumps). The vapour bubbles (dubbed cavitation bubbles because they generate "cavities" in the liquid) burst when they are carried away from low-pressure areas, causing very destructive, extremely high-pressure waves.
This condition is a typical cause of decreased performance and
Cavitation refers to the erosion of impeller blades and is a significant concern in the design of hydraulic turbines and pumps.
Cavitation should be avoided (or at least minimised) in flow systems because it decreases performance, creates irritating vibrations and noise, and damages equipment. Pressure spikes caused by a high number of bubbles bursting near a solid surface over time may cause erosion, surface pitting, fatigue failure, and eventually the destruction of the components or machinery. Cavitation in a flow system can be detected by its characteristic tumbling sound.
Compressibility:
The volume (or density) of a fluid change as its temperature or pressure changes. Fluids often expand when heated or depressurized and shrink when chilled or pressured. However, the amount of volume change varies depending on the fluid, and we must establish parameters that connect volume changes to pressure and temperature changes i.e., bulk modulus of elasticity k and the coefficient of volume expansion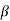 
.
It is a well-known fact that a fluid contracts as additional pressure is applied to it and expands when the pressure on it is lessened. In other words, fluids behave like elastic solids when subjected to pressure. As a result, similar to Young's modulus of elasticity for solids, it is suitable to define a compressibility coefficient k (also known as the bulk modulus of compressibility or bulk modulus of elasticity) for fluids as
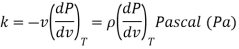
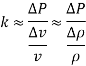
As, both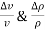 
are dimensionless , k has dimension of pressure.
Furthermore, the coefficient of compressibility reflects the change in pressure caused by a fractional change in the volume or density of the fluid while the temperature stays constant. As a result, the compressibility coefficient of a completely incompressible substance (v! constant) equals infinite.
A high k number implies that a substantial change in pressure is required to generate a tiny fractional change in volume, implying that a fluid with a high k is effectively incompressible. This is normal for liquids and explains why they are commonly thought to be incompressible. For example, to compress water by 1% at normal atmospheric circumstances, the pressure must be raised to 210 atm, resulting to a coefficient of compressibility value of k= 21,000 atm.
Small variations in liquid density can nonetheless induce intriguing phenomena in piping systems, such as water hammer, which is characterised by a sound similar to that produced when a pipe is "hammered." This happens when a liquid in a pipe network hits a sudden flow constraint (such as a shutting valve) and is squeezed locally. As the acoustic waves travel and reflect along the pipe, they impact the pipe surfaces, bends, and valves, causing the pipe to vibrate and make the characteristic sound.
Note that volume and pressure are inversely proportional (volume
Decreases as pressure is increased and thus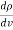 
is a negative quantity),
And the negative sign in the definition ensures that k is a positive
Quantity. Also, differentiating
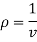
We get,
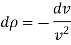
Rearranging,
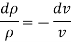
That is, the fractional changes in the specific volume and the density of a
Fluid is equal in magnitude but opposite in sign.
The isothermal compressibility and is defined as the inverse of the compressibility coefficient.
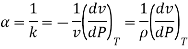
A fluid's isothermal compressibility reflects the fractional change in volume or density that corresponds to a unit change in pressure.
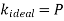
Bulk Modulus/Coefficient of volume expansion:
In general, the density of a fluid depends on temperature more than pressure, and the variation of density with temperature is responsible for many natural phenomena such as winds, ocean currents, the rise of plumes in chimneys, the operation of hot-air balloons, heat transfer by natural convection, and even the rise of hot air, giving rise to the phrase "heat rises." We need a characteristic that describes the fluctuation of a fluid's density with temperature under constant pressure in order to measure these impacts.
That information is provided by the coefficient of volume expansion (or volume expansivity) b, which is defined as
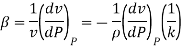
It can also be expressed approximately in terms of finite changes as
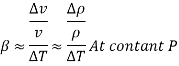
A big value of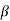 
for a fluid indicates a large change in density with temperature, and the product 
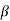&T reflects the proportion of volume change that corresponds to a temperature change of 
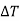at constant pressure.
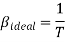
The state of the main fluid body that surrounds the finite hot or cold areas is represented by the subscript "infinity" in the study of natural convection currents to serve as a reminder that this is the value at a distance where the presence of the hot or cold region is not sensed. In such instances, the volume expansion coefficient may be roughly represented as
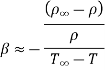
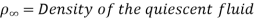
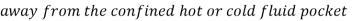
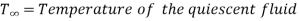
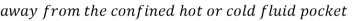
The buoyancy force, which is proportional to the density difference, which is proportional to the temperature difference at constant pressure, initiates natural convection currents. As a result, the greater the temperature differential between the hot or cold fluid pocket and the surrounding main fluid body, the greater the buoyancy force and, consequently, the stronger the natural convection currents.
The combined effects of pressure and temperature changes on a fluid's volume change may be calculated by treating the specific volume as a function of T and P.
Differentiating,
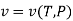
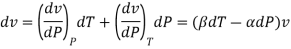
The fractional change in volume (or density) due to pressure and temperature variations may therefore be approximated as
Key Takeaway:
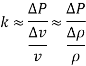
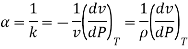
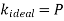
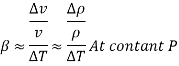
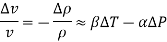
Solved Examples
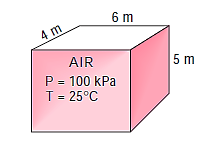
Q.1. Determine the density, specific gravity, and mass of the air in a room whose dimensions are 4 m ! 5 m ! 6 m at 100 kPa and 25°C .
Solution:
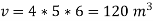
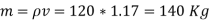
Q.2. The temperature of water in a water distribution system has been measured to reach as high as 30°C. Determine the system's minimal pressure to minimise cavitation.
Solution:
The vapor pressure of water at 30°C is 4.25 kPa.
Analysis To avoid cavitation, the pressure anywhere in the flow should not be allowed to drop below the vapor (or saturation) pressure at the given temperature.
That is, 4.25 kPa
Therefore, the pressure should be maintained above 4.25 kPa everywhere in the flow.
Q.3. Consider water at 20°C and 1 atm at first. Determine the ultimate density of water if it is heated to 50°C at a constant pressure of 1 atm and compressed to 100-atm pressure at a constant temperature of 20°C. Assume that the isothermal compressibility of water is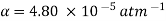 
Solution:
The density of water at 20°C and 1 atm pressure is = 998.0 Kg/m3
The coefficient of volume expansion at the average temperature
Of ( 20+50)/2 = 35 is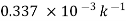 
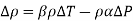
The change in density due to the change of temperature from 20°C to 50°C at constant pressure is,
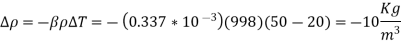
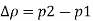
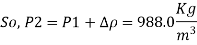
The change in density due to a change of pressure from 1 atm to 100
Atm at constant temperature is
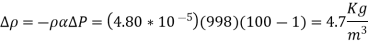
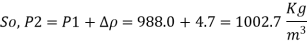
Q.4. In a cup, a 0.6-mm-diameter glass tube is immersed in 20°C water. Calculate the capillary rise of the water in the tube.
Given: The surface tension of water at 20°C is 0.073 N/m
Solution:
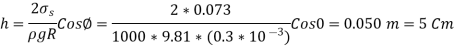
References
- Fluid Mechanics: Fundamentals and Applications by Y. A. Çengel and J. M. Cimbala, by McGraw-Hill
- Fluid Mechanics and Hydraulic Machines by R.K. Bansal, Laxmi Publications
- Fluid Mechanics by Frank .M. White, mcgraw Hill Publishing Company Ltd.
- Fundamentals of Fluid Mechanics by munson, Wiley India Pvt. Ltd
