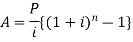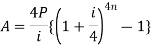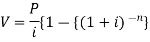Unit-2
Compound interest and Annuities
Calculation of interest is one of the most basic uses of mathematics in finance.
We can define the simple interest as- “the price has to be paid for the use of a certain amount of money or principal for certain period, is called interest”
Amount- This is the sum of the principal and the interest at any time.
The rate of interest is denoted by ‘i’.
Let the principal is 100 rs. And the interest is 8 then we can say that the rate of interest is 8 percent per annum or we can write it as r = 8%
Suppose ‘P’ is the principal and ‘n’ is the time for which the principal is given and ‘r’ be the rate of interest per annum
‘I’ be the amount of interest
And ‘i’ be the rate of interest per unit
Then-

Here- i = r/100
We can calculate the amount as-

Example: Sushmita invested 5000rs. At the rate of 8 per cent per annum then what will be the value of the amount she invested in 5 years.
Sol.
Here P = 5000, i = 8/100 = 0.08, n = 5, now

Hence the required amount is - 7000
Example: Find out the simple interest on 5600 rs. At 12 percent per annum from July 15 to September 26, 2020.
Sol.
Here we calculate the time-
Total days from july 15 to sept 26 = 73 days or 73/365 = 1/5 years
And P = 5600, i = 12/100 = 0.12
Simple interest = P.i.n. = 5600 
Hence the simple interest is – 134.40
Example: Harpreet invests 1200rs. At 10 percent per annum for some time and it becomes 1560 then find the principal when that will become 2232 at 8 percent p.a. in the same time.
Sol.
Here in first situation- P = 1200, A = 1560 and i = 0.10
So that,

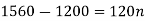
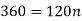
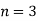
In second situation-
A = 2232, n = 3, i = 0.08
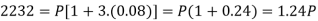
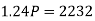
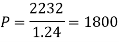
Compound interest-
In compound interest, the principal does not remain same but increases at the end of each interest period.
Let-
P- Principal
A - Amount
i = interest on re. 1 for a year
n = interest period
Then the amount can be calculated as-

And
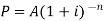
Note- By using algorithm the above formula can be written as-

Note- if the compound interest is paid half-yearly, quarterly, monthly instead of a year the there will be different formulae as given in the table below-
Time | Amount |
Annually |
|
Half-yearly |
|
Quarterly |
|
Example: Aman invests 1000 rupees at 5 percent p.a for four years then find the compound interest on it.
Sol.
Here P = 1000, i = 0.05 and n = 4
Then we know that-
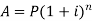
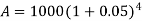
On taking log, we get-


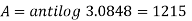
Compound interest will be-
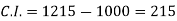
Which is the required answer.
Example: A sum of money invested at C.I. payable yearly amounts to 10, 816 rs. at the end of the second year and to 11,248.64 rs. at the end of the third year. Find the rate of interest and the sum.
Sol.
Here A1 = 10,816, n = 2, and A2 = 11,248.64, n = 3
We know that
A = P (1 + i)n we get,
10,816 =  … (i)
… (i)
11,248.64 = … (ii)
… (ii)
Here on dividing equation (2) by (1)-

We get-


And

Hence the rate is 4 percent.
Now from first equation-
10,816 = 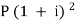
Or

Now-

P = antilog 4.000 = 10,000
Therefore the require answer- 10,000
Nominal and effective rate of interest-
Nominal rate of interest is the rate of interest per annum which is compounded yearly, half yearly, quarterly, monthly, n times in a year or continuously.
Effective rate of interest is the rate of interest per annum compounded only once in a year.
There is a relationships between nominal and effective rate of interest under two different conditions-


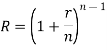
Where ‘r’ is nominal rate and ‘R’ is effective rate.
2. If compounding is continuous-

Or

Relationship between two nominal rates-
If interest is compounded quarterly at  percent and the interest is compounded half yearly at
percent and the interest is compounded half yearly at  percent, then the relationship between the two is-
percent, then the relationship between the two is-
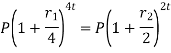
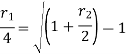

Example: Ronak deposited Rs. 10,000 in a bank for 3 years. Bank gives two offers either 10 percent compounded quarterly or 8% compounded continuously, then which offer is preferable for Ronak?
Sol.
Balance after three years under first offer-

Balance after 3 years under second offer-
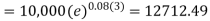
So that we can conclude that the first offer is preferable for Ronak.
Key takeaways-

3. Compound interest- 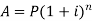
4. Nominal rate- Nominal rate of interest is the rate of interest per annum which is compounded yearly, half yearly, quarterly, monthly, n times in a year or continuously.
5. Effective rate- Effective rate of interest is the rate of interest per annum compounded only once in a year.
6. 
Present value-
Present value of a given sum due at the end of a given period is that sum which together with its interest of the given period equals to the given sum.
Or in other words “Present value describes how much a future sum of money is worth today”.
Let the objective is to have an amount A after
n years from today. If the interest rate is ‘i’, then the amount is required to deposited now
so as to achieve the set target is-

Which is called the present value of A.
Note-
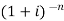
Present value (Continuous compounding)-
We use the following formula if there is continuous compounding-
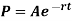
Where-
P = principal amount
A = Amount at future point of time
t = time
r = rate of interest
Example: Find out the present value of Rs. 1000 due in 2 years at 5% per annum compound interest, If the interest is paid yearly.
Sol.
Here, A = 1000, n = 2, i = 0.05
We have to find P-
By the formula-

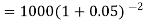


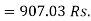
Therefore the present value is 
Example: Find the present value of Rs. 4,500 due after 3 years from now. the interest is compounded continuously at the interest rate of 6%.
Sol.
Here we have- A = 4500, t = 3, r = 0.06,
To find P,





Annuity-
An annuity is a sequence of equal payment or a sequence of regular payment at regular intervals or in other words, An annuity is a fixed sum paid at regular intervals under certain conditions.
The length of time during which the annuity is paid can either be until the death of the recipient or for a guaranteed minimum term of years, irrespective of whether the annuitant is alive or not.
The time between payments is called the payment interval, and the time which the money is to be paid is called the term of the annuity.
Amount of an annuity:
Amount of an annuity is the total of all the installments left unpaid together with the compound interest of each payment for the period it remains unpaid.
The formulas to find the amount of annuity are given below-
| When annuity is payable annually and interest is also compounded annually |
| annuity is payable half-yearly and interest is also compounded half-yearly |
| annuity is payable quarterly and interest is also compounded quarterly |
Present value of an annuity-
Present value of an annuity is the sum of the present values of all payments (or installments) made at successive annuity periods
The formulas to calculate present value ‘V’ of an annuity P are given below-
| When V of an annuity P payable annually |
| When V of an annuity P payable half-yearly |
| When V of an annuity P payable quarterly |
The future value is calculated as-
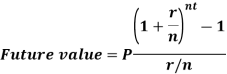
Example: Sundar decides to deposit 20,000rs. at the end of each year in a bank which pays 10% p.a. compound interest.
If the installments are allowed to accumulate, what will be the total accumulation at the end of 9 years?
Sol.
Suppose A rs. be the total accumulation at the end of 9 years. Then we get-
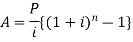
Here P = 20,000 rs., i = 10/100 = 0.1 and n = 9
Then
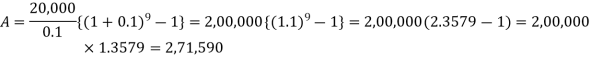
Hence the total required accumulation is 2,71,590 rs.
Example: Rajeev purchased a flat valued at 3,00,000rs. He paid 2,00,000 rs. at the time of purchase and agreed to pay the balance with interest of 12% per annum compounded half yearly in 20 equal half yearly installments.
If the first installment is paid after six months from the date of purchase, find the amount of each installment.
[Given log 10.6 = 1.0253 and log 31.19 = 1.494]
Sol.
Here 2,00,000 has been paid at the time of purchase when cost of the flat was 3,00,000, we have to
consider 20 equated half yearly annuity payment P when 12% is rate of annual interest compounded half
yearly for present value of 1,00,000rs.
So that-

Or



Then-

Hence the amount of each installment = 8,718.40
Suppose,

Taking log-

Hence
X = 0.3119
Stated annual rate and effective annual rate-
Stated annual interest rate is the rate expressed as a per year percentage, by which interest payments are determined.
Or in other words, the stated annual interest rate is the return on an investment that is expressed as a per-year percentage.
It is also called quoted interest rate
It is the simple interest rate that the bank gives as the interest rate on loan.
Effective rate of interest-(EAR)
It is the equivalent annual rate of interest which is compounded annually.
Effective annual interest rate = 
Where i =actual rate of interest, n = compounding period.
Example: Rohan invests Rs. 5,000 in a term deposit plan. The plan offers an interest rate of 6% p.a., compounded quarterly. How much interest will John earn after one year? Also, what is the effective rate of interest?
Sol. Here we have-
P = 5000, i = 6% per annum or 0.06 p.a. or 0.015 per quarter
Compounding period = n = 4
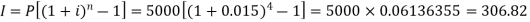
Rohan earns Rs. 306.82 interest after a year
Now the effective rate of interest is-
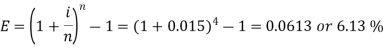
Example: What is the effective rate of interest on a CD that has a nominal rate of 9.5 percent with interest compounded monthly?
Sol.
Here we have,
i = 9.5% or 0.095
Now the effective rate of interest is-

Example: Amitabh invested Rs. 100 in a scheme that pays out a nominal annual interest rate of 10% compounded on a quarterly basis. Find the Effective Annual Rate.
Sol.
Here we have,
i = 10% or 0.1
Now the effective rate of interest is-
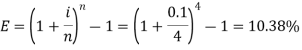
Key takeaways-
An annuity is a fixed sum paid at regular intervals under certain conditions.
The length of time during which the annuity is paid can either be until the death of the recipient or for a guaranteed minimum term of years, irrespective of whether the annuitant is alive or not.
2. Amount of an annuity:
Amount of an annuity is the total of all the installments left unpaid together with the compound interest of each payment for the period it remains unpaid.
3. Present value of an annuity- Present value of an annuity is the sum of the present values of all payments (or installments) made at successive annuity periods
4. the stated annual interest rate is the return on an investment that is expressed as a per-year percentage.
5. Effective annual interest rate = 
Where i =actual rate of interest, n = compounding period.
Depreciation-
Depreciation is defined as the expensing of the cost of an asset involved in producing revenues throughout its useful life.
Depreciation expense affects the values of businesses and entities because the accumulated depreciation disclosed for each asset will reduce its book value on the balance sheet. Depreciation expense also affects net income. Generally the cost is allocated as depreciation expense among the periods in which the asset is expected to be used. Such expense is recognized by businesses for financial reporting and tax purposes.
Depreciation does not actually represent any kind of cash transaction. Instead, it simply represents how much of an asset's value has been used up over time and can be deducted as an expense.
The total depreciation over a period of time is known as "accumulated depreciation". The "book value" of an asset is calculated by deducting the accumulated depreciation from the original purchase price. The book value is what is reflected as the asset's value on the balance sheet.
Depreciation calculation-
There are four criteria used to calculate depreciation-
1- The initial cost of the asset.
2- The expected residual value (also known as salvage value) - this is the value of asset at the end of its useful life, which may be zero.
3- The estimated useful life of the asset.
4- An appropriate method of apportioning the cost of the useful life of the asset.
Equated monthly instalments-
An equated monthly installment(EMI) is a fixed payment amount made by a borrower to a lender at a specified date e ach calendar month. Equated monthly installments are used to pay off both interest and principal each month so that over a specified number of years, the loan is paid off in full.
Note-
The EMI flat-rate formula is calculated by adding together the principal loan amount and the interest on the principal and dividing the result by the number of periods multiplied by the number of months.
The EMI reducing-balance method is calculated using the formula shown below, in which P is the principal amount borrowed, I is the annual interest rate, r is the periodic monthly interest rate, n is the total number of monthly payments, and t is the number of months in a year.
Example: A man buys a machine for Rs.20,00. What its value be after 6 years, if it is assumed to depreciate at a fixed rate of 12% per annum.
Sol.
Here we have P = 20,000, n = 6 and r = -0.12 (rate of interest is negative in depreciation)
Then,

So that the value of the machine in 6 years will be = 9288.08
Example: A machine valued at 500,000 depreciates at 6% per annum then in how many year its value will reduce to 100,000?
Sol. Here P = 500,000, r = 0.06, n = ? and the final value is = 100,000
We know that-


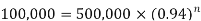

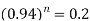
Taking log on both sides-

So that-

Therefore approximately it will take 26 years for the value decline to 100,000
Example: Ayesha invests Rs. 3000 initially and then Rs. 1800 at the end of the first, second and third years and finally Rs. 600 at the end of 4th year.
If the interest is paid annually at the rate of 6.5% then find the value of the investment at the end of 5th year.
Sol.
Rs. 3000 is invested for 5 years and grows to-

The three sums of Rs. 1800 are invested for 4, 3 and 2 years and grow in total-

And Rs. 600 is invested for 1 year and grows to-

Then the total value at the end of 5 years will be 11,280.81
Sinking funds-
A sinking fund is a special type of investment in which a constant amount is invested each year, usually with a view to reaching a specified value at a given point in the future. Questions need to be read carefully in order to be clear about exactly when the first and last installments are paid.
Key takeaways-
References-