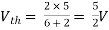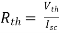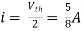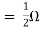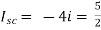UNIT 1
DC CIRCUITS

# In case of capacitor: -
At time t= 0-
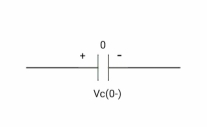
:. Capacitor is initially uncharged
Q (0-) =0
Q=cv
Q (0-) = cvc (0-)
Vc (0-) =0
At time t= 0+
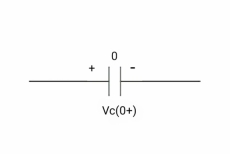
By low of conservation of charges
Q (0-) = Q (0+)
Cvc (0-) =cvc (0+)
Vc (0+) =0
Hence acts as
If capacitor is initially uncharged at t= 0- than voltage across capacitor is zero at t=0+ capacitorwill follow low of consvation of charges and Vc (0+) =0 it means at t=0+ capacitor behaves as short circuit in other words, voltage across capacitor cannot change instantaneously.
At time t= infinity,
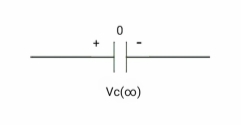
I = cdvc/dt constant and maximum
Hence, i= 0
At t= infinity capacitor will be fully charged or voltage across capacitor is constant and current through capacitor is zero so, capacitor will behave as open –circuit
Say capacitor is initially charged

At time t= 0-,
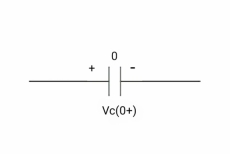
At time t= 0+,
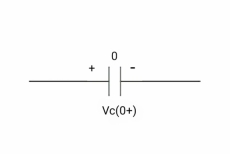
At time t= 0+
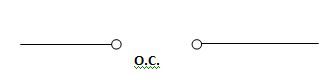
Q (0-) = Q (0+)
Cvc (0-) =cvc (0+)
V1 = Vc (0+)
Hence acts as
Its capacitor is initially charged at t= 0- as voltage across capacitor is V1 than at t=0+ capacitor behaves as a voltage as a voltage source with value V1
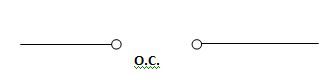
At time t = infinity
If capacitor is initially charged and it is connected to any independent source for long time than current through capacitor is 3 etw and capacitor will be fully charged and it behaves as o.c
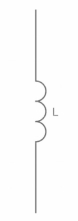
# for Inductor: -
At time t= 0-
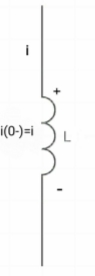
Ø= Li =0
Ø (0) = Li (0-)
i (0-) =0
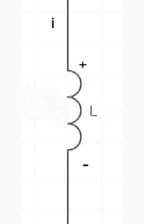
At t= 0+,
By low of conservation of flux
Ø (0-) = Ø (0+)
Li (0-) = Li (0+)
0 =i (0+)
Hence, acts as short circuit
Ideal and practical Voltage and Current source:
A voltage source is a device which provides a constant voltage to load at any instance of time and is independent of the current drawn from it. This type of source is known as an ideal voltage source. Practically, the ideal voltage source cannot be made. It has zero internal resistance. It is denoted by this symbol.

Fig: Voltage source symbol
Ideal Voltage Source
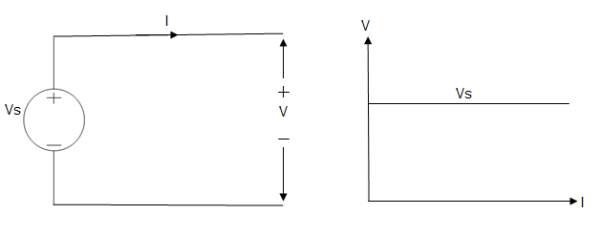
Fig: Ideal Voltage Source
The graph represents the change in voltage of the voltage source with respect to time. It is constant at any instance of time.
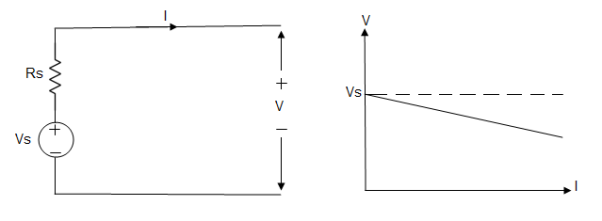
Voltage sources that have some amount of internal resistance are known as a practical voltage source. Due to this internal resistance, voltage drop takes place. If the internal resistance is high, less voltage will be provided to load and if the internal resistance is less, the voltage source will be closer to an ideal voltage source. A practical voltage source is thus denoted by a resistance in series which represents the internal resistance of source.
Practical Voltage source
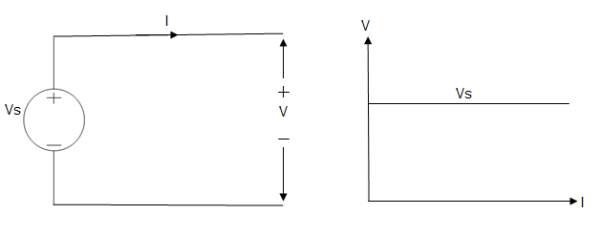
Fig: Practical Voltage source
The graph represents the voltage of the voltage source with respect to time. It is not constant but it keeps on decreasing as the time passes.
Current source
A current source is a device which provides the constant current to load at any time and is independent of the voltage supplied to the circuit. This type of current is known as an ideal current source; practically ideal current source is also not available. It has infinite resistance. It is denoted by this symbol.
Ideal Current source
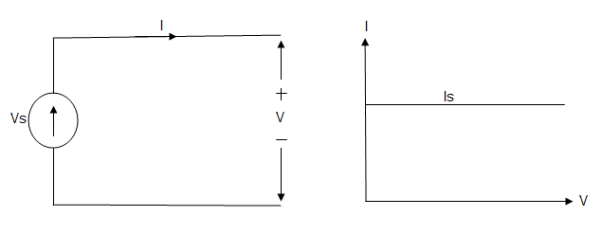
Fig: Ideal Current source
The graph represents the change in current of the current source with respect to time. It is constant at any instance of time.
Why ideal Current source has infinite resistance?
A current source is used to power a load, so that load will turn on. We try to supply 100% of the power to load. For that, we connect some resistance to transfer 100% of power to load because the current always takes the path of least resistance. So, in order for current to go to the path of least resistance, we must connect resistance higher than load. This is why we have the ideal current source to have infinite internal resistance. This infinite resistance will not affect voltage sources in the circuit.
Practical Current source
Practically current sources do not have infinite resistance across there but they have a finite internal resistance. So the current delivered by the practical current source is not constant and it is also dependent somewhat on the voltage across it.
A practical current source is represented as an ideal current source connected with resistance in parallel.
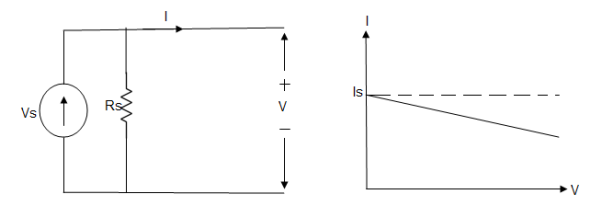
Fig; Practical Current source
The graph represents the current of the current source with respect to time. It is not constant but it also keeps on decreasing as the time passes.
Examples of current and voltage sources
The examples of current source are solar cells, transistors and examples of some voltage sources are batteries and alternators.
This was all about ideal and practical sources of power. The ideal sources are very useful for calculations in theory but as ideal sources are not practically possible, only practical sources are used in practical circuits. The batteries we use are a practical source of power and the voltage and current decreases as we use it. Thus both are useful to us in their own ways.
The algebraic sum of currents meeting at a junction or node in a electric circuit is zero or the summation of all incoming current is always equal to summation of all outgoing current in an electrical network.
Explanation
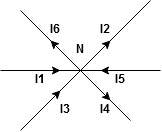
Assuming the incoming current to be positive and outgoing current negative we have
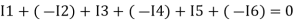
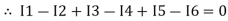
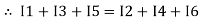
I e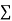 incoming current = ∑ outgoing current thus, the above Law can also be stated as the sum of current flowing towards any junction in an electric circuit is equal to the sum of currents flowing away from that junction
incoming current = ∑ outgoing current thus, the above Law can also be stated as the sum of current flowing towards any junction in an electric circuit is equal to the sum of currents flowing away from that junction
Kirchhoff’s Voltage Law (KVL)
Statement : the algebraic summation of all Voltage in any closed circuit or mesh of loop zero.
Ie ∑ Voltage in closed loop = 0 the summation of the Voltage rise (voltage sources) is equal to summation of the voltage drops around a closed loop in 0 circuit for explanation from here
Determination of sigh and direction of currents (Don’t write in exams just for understanding)

Current entering a resistor is +ve and leaving should be –ve
Now
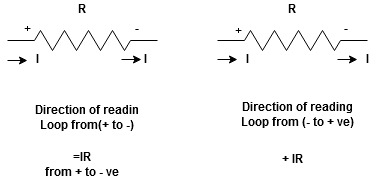
Potential Rise Potential Drop
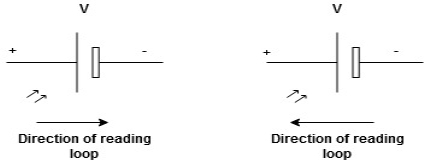
We are reading from +V to –V we are reading from –V to +V
 potential drops
potential drops  potential rise
potential rise
 -V
-V  +V
+V
Given Circuit
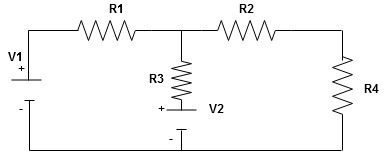
First identify no of loops and assign direction of current flowing in loop
Note : no of loops in circuit = No, of unknown currents = no, of equations in the circuit
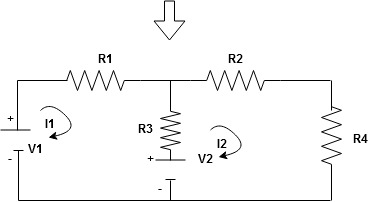
Note : keep loop direction and current direction same ie either clockwise or anticlockwise for all loops I1 I2
Now according to direction of direction assign signs (+ve to –ve) to the resistors
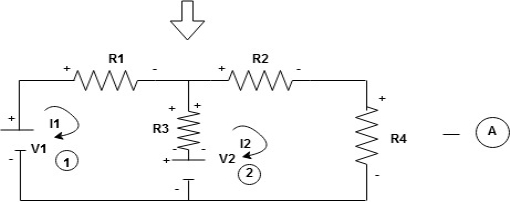
Note : voltage sources (V) polarities does not change is constant.
Note: for common resistor between 2 loops appearing in the circuit like R3 give signs according to separate loops as shown
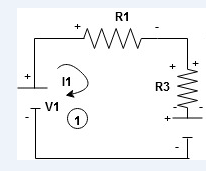
When considering only loop no 1 (+ R3 - )
- B
Now consider diagram A and write equations
Two loops  two unknown currents
two unknown currents  two equation
two equation
Apply KVL for loop ①
(+ to drop -) = - sign and (- to rise +) = + sign
 for drop = -sign
for drop = -sign
 for rise = + sign
for rise = + sign
-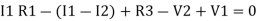
-(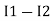 ) R2 is considered because in R3,2 currents are flowing
) R2 is considered because in R3,2 currents are flowing 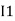 and
and 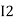 and we have taken (
and we have taken ( ) because we are considering loop no 1 and current flowing is
) because we are considering loop no 1 and current flowing is 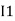 in loop no 1
in loop no 1
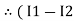 )
)
Similarly for loop no. 2 currents flowing is 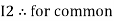 resistor R3 it should be
resistor R3 it should be 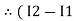 )R3
)R3
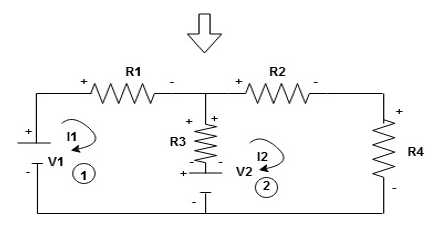
Consider loop no. 1 apply KVL
-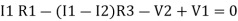 …….①
…….①
-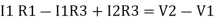
Consider loop no. 2 apply KVL
-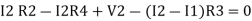 …….②
…….②
-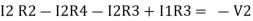
After solving equation ① and ② we will get branch current  and
and 
Consider the following series RL circuit diagram.
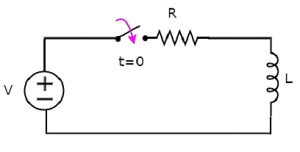
In the above circuit, the switch was kept open up to t = 0 and it was closed at t = 0. So, the DC voltage source having V volts is not connected to the series RL circuit up to this instant. Therefore, there is no initial current flows through inductor.
The circuit diagram, when the switch is in closed position is shown in the following figure.
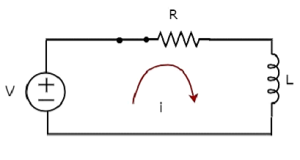
Now, apply KVL around the loop.
V=Ri+ L di/dt
Di/dt+⟮R/L⟯i=VL Equation 1
The above equation is a first order differential equation and it is in the form of
Dy/dt+Py=Q Equation 2
By comparing Equation 1 and Equation 2, we will get the following relations.
x=t
y=i
P=R/L
Q = V/L
The solution of Equation 2 will be
Ye∫pdx=∫Qe∫pdxdx+k-------------------------Equation 3
Where, k is the constant.
Substitute, the values of x, y, P & Q in Equation 3.
Ie∫⟮R/L⟯dt=∫(V/L)⟮e∫⟮R/L⟯dt⟯dt+k
⇒ie⟮R/L⟯t=V/L∫e⟮R/L⟯tdt+k
⇒ie⟮R/L⟯t=V/L{e⟮R/L⟯t/R/L}+k
⇒i=V/R+ke−⟮R/L⟯t--------------------------Equation 4
We know that there is no initial current in the circuit.
Hence, substitute, t = 0 and 𝑖 = 0 in Equation 4 in order to find the value of the constant k.
0=V/R+ke−⟮R/L⟯(0)0=
0=VR+k(1)
k=−V/R
Substitute, the value of k in Equation 4.
i=V/R+⟮−V/R⟯e−⟮R/L⟯t
i=V/R−V/Re−⟮R/L⟯t
Therefore, the current flowing through the circuit is
i=−V/R e−⟮R/L⟯t+V/R Equation 5
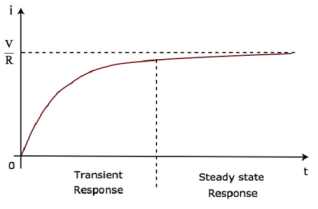
So, the response of the series RL circuit, when it is excited by a DC voltage source, has the following two terms.
- The first term −V/ R e−⟮R/L⟯t corresponds with the transient response.
- The second term V/R corresponds with the steady state response.
- These two responses are shown in the following figure.
We can re-write the Equation 5 as follows −
i=V/R⟮1−e−⟮R/L⟯t⟯
⇒i=VR⟮1−e−⟮tτ ) Equation 6
Where, τ is the time constant and its value is equal to LR.
Both Equation 5 and Equation 6 are same. But, we can easily understand the above waveform of current flowing through the circuit from Equation 6 by substituting a few values of t like 0, τ, 2τ, 5τ, etc.
If a sinusoidal signal is applied as an input to a Linear electric circuit, then it produces a steady state output, which is also a sinusoidal signal. Both the input and output sinusoidal signals will be having the same frequency, but different amplitudes and phase angles.
We can calculate the steady state response of an electric circuit, when it is excited by a sinusoidal voltage source using Laplace Transform approach.
The s-domain circuit diagram, when the switch is in closed position, is shown in the following figure.
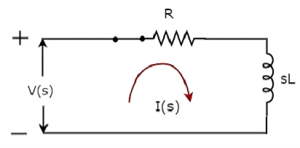
In the above circuit, all the quantities and parameters are represented in s-domain. These are the Laplace transforms of time-domain quantities and parameters.
The Transfer function of the above circuit is
H(s)=I(s)V(s)
⇒H(s)=1Z(s
⇒H(s)=1R+sL
Substitute s=jω in the above equation.
H(jω)=1R+jωL
Magnitude of H(jω) is
|H(jω)|=1R2+ω2−−−−−−−√L2|H(jω)|=1R2+ω2L2
Phase angle of H(jω) is
∠H(jω)=−tan−1⟮ωL/R)
We will get the steady state current iss(t) by doing the following two steps −
- Multiply the peak voltage of input sinusoidal voltage and the magnitude of H(jω)
- Add the phase angles of input sinusoidal voltage and H(jω).
The steady state current iss(t) will be
Iss(t)=Vm/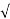 R2+ω2L2 (sin⟮ωt+φ−tan−1⟮ωL/R⟯⟯
R2+ω2L2 (sin⟮ωt+φ−tan−1⟮ωL/R⟯⟯
Substitute the value of iss(t) in Equation 2.
i(t)=Ke−⟮t/τ⟯+Vm/ 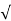 R2+ω2L2 sin⟮ωt+φ−tan−1⟮ωL/R⟯⟯ --------------Equation 3
R2+ω2L2 sin⟮ωt+φ−tan−1⟮ωL/R⟯⟯ --------------Equation 3
We know that there is no initial current in the circuit.
Hence, substitute t = 0 & i(t) = 0 in Equation 3 in order to find the value of constant, K.
0=Ke−⟮0τ⟯+Vm / 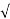 R2+ω2L2 [sin⟮ω(0)+φ−tan−1⟮ωL/R⟯
R2+ω2L2 [sin⟮ω(0)+φ−tan−1⟮ωL/R⟯
⇒0=K+Vm/ 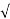 R2+ω2L2 sin⟮φ−tan−1⟮ωL/R)
R2+ω2L2 sin⟮φ−tan−1⟮ωL/R)
⇒K=−Vm/ 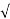 R2+ω2L2 sin⟮φ−tan−1⟮ωL/R)
R2+ω2L2 sin⟮φ−tan−1⟮ωL/R)
Substitute the value of K in Equation 3.
i(t)=−Vm/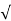 R2+ω2L2sin⟮φ−tan−1⟮ωL/R⟯⟯e−⟮t/τ⟯+Vm/
R2+ω2L2sin⟮φ−tan−1⟮ωL/R⟯⟯e−⟮t/τ⟯+Vm/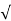 R2+ω2L2sin⟮ωt+φ−tan−1⟮ωL/R⟯⟯
R2+ω2L2sin⟮ωt+φ−tan−1⟮ωL/R⟯⟯
-----------------Equation 4
Equation 4 represents the current flowing through the series RL circuit, when it is excited by a sinusoidal voltage source. It is having two terms. The first and second terms represent the transient response and steady state response of the current respectively.
We can neglect the first term of Equation 4 because its value will be very much less than one. So, the resultant current flowing through the circuit will be
i(t)=Vm/ 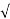 R2+ω2L2 sin⟮ωt+φ−tan−1⟮ωL/R⟯
R2+ω2L2 sin⟮ωt+φ−tan−1⟮ωL/R⟯
It contains only the steady state term. Hence, we can find only the steady state response of AC circuits and neglect transient response of it.
Transient response:
The transient response time T is measured in terms of τ = R x C, in seconds, where R is the value of the resistor in ohms and C is the value of the capacitor in Farads. This then forms the basis of an RC charging circuit were 5T can also be thought of as “5 x RC”.
RC Charging Circuit
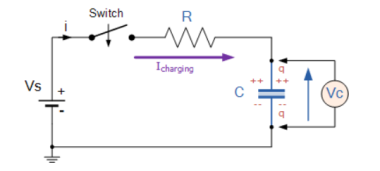
The figure below shows a capacitor, (C ) in series with a resistor, ( R ) forming a RC Charging Circuit connected across a DC battery supply ( Vs ) via a mechanical switch. At time zero, when the switch is first closed, the capacitor gradually charges up through the resistor until the voltage across it reaches the supply voltage of the battery.
RC Charging Circuit
Let us assume above, that the capacitor, C is fully “discharged” and the switch (S) is fully open. These are the initial conditions of the circuit, then t = 0, i = 0 and q = 0. When the switch is closed the time begins at t = 0 and current begins to flow into the capacitor via the resistor.
Since the initial voltage across the capacitor is zero, ( Vc = 0 ) at t = 0 the capacitor appears to be a short circuit to the external circuit and the maximum current flows through the circuit restricted only by the resistor R.
Then by using Kirchhoff’s voltage law (KVL), the voltage drops around the circuit are given as:
Vs – R x i(t) – Vc(t) =0.
The current now flowing around the circuit is called the Charging Current and is found by using Ohms law as: i = Vs/R.
RC Charging Circuit Curves
As the capacitor charges up as shown, the rise in the RC charging curve is steeper at the beginning because the charging rate is fastest at the start and then tapers off as the capacitor takes on additional charge at a slower rate.
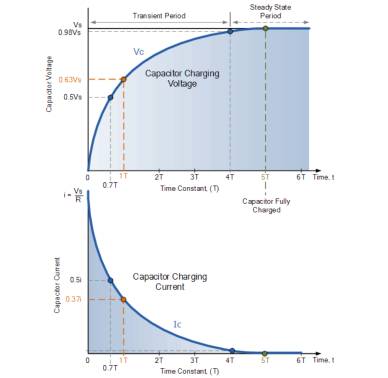
As the capacitor charges up, the potential difference across its plates slowly increases with the actual time taken for the charge on the capacitor to reach 63% of its maximum possible voltage, in our curve 0.63Vs being known as one Time Constant, ( T ).
This 0.63Vs voltage point is given the abbreviation of 1T, (one time constant).
The capacitor continues charging up and the voltage difference between Vs and Vc reduces, so too does the circuit current, i. Then at its final condition greater than five time constants ( 5T ) when the capacitor is said to be fully charged, t = ∞, i = 0, q = Q = CV.
At infinity the charging current finally diminishes to zero and the capacitor acts like an open circuit with the supply voltage value entirely across the capacitor as Vc = Vs.
So mathematically we can say that the time required for a capacitor to charge up to one time constant, ( 1T ) is given as:
RC Time Constant,
τ= R x C
This RC time constant only specifies a rate of charge where, R is in Ω and C in Farads.
Since voltage V is related to charge on a capacitor given by the equation, Vc = Q/C, the voltage across the capacitor ( Vc ) at any instant in time during the charging period is given as:
Vc = Vs(1- e(-t/RC))
Where:
Vc is the voltage across the capacitor
Vs is the supply voltage
t is the elapsed time since the application of the supply voltage
RC is the time constant of the RC charging circuit.
After a period equivalent to 4 time constants, ( 4T ) the capacitor in this RC charging circuit is virtually fully charged and the voltage across the capacitor is now approx 98% of its maximum value, 0.98Vs. The time period taken for the capacitor to reach this 4T point is known as the Transient Period.
After a time of 5T the capacitor is now said to be fully charged with the voltage across the capacitor, ( Vc ) being equal to the supply voltage, ( Vs ). As the capacitor is fully charged no more current flows in the circuit. The time period after this 5T point is known as the Steady State Period.
Series R-L-C circuit:
The circuit shown is called series R-L-C circuit. When the switch is closed the equation describes the response of the system by applying KVL around the mesh.
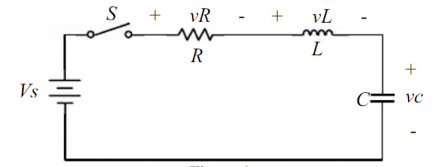
VR + vL + vc = Vs -----------------------------(1)
The current flowing in the circuit is
i = C dvc/dt -----------------------------(2)
Thus, the voltages vR and vL are given by
VR = iR = RC dvc/dt ----------------------------------(3)
VL = L di/dt = LC d2vc/dt2 -----------------------------------------(4)
Substituting eq (3) and (4) in (1) we get
d 2 vc/dt 2 + R/L dvc/dt + 1/LC vc = 1/LC Vs ---------------------------(5)
The solution to equation(5) is the linear combination of the homogeneous and the particular solution vc = vcp + vch
The particular solution is
Vcp=vs --------------------------------------------(6)
And the homogeneous solution satisfies the equation
d 2 vch/dt2 + R/L d vch/dt + 1/LC vch =0 ----------------------------(7)
Assuming the homogeneous solution is of the form Ae st and by substituting into equation(7) we get
s 2 + R/L s + 1/LC =0 ------------------------------(8)
By defining α = R/2L ; Damping rate ----------------------------(9)
w0 = 1/ 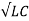 : Natural frequency.-------------------------(10)
: Natural frequency.-------------------------(10)
The characteristic equation becomes
s 2 + 2 α s + wo 2 = 0 -------------------------------------(11)
The roots of the characteristic equation are :
s 1 = -α + 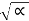 2 – wo 2 --------------------------------------------(12)
2 – wo 2 --------------------------------------------(12)
s2 = -α - 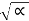 2 – wo 2 --------------------------------------------------------------------(13)
2 – wo 2 --------------------------------------------------------------------(13)
And the homogeneous solution becomes
Vch = A1 e s1t + A2 e s2t ----------------------------------------------------------(14)
The total solution is
Vc = Vs + A1 e s1t + A2 es2t ------------------------------------------------------------------(15)
The parameters A1 and A2 are constants and can be determined by the application of the initial conditions of the system vc(t=0) and dvc(t)/dt
The value of the 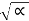 2 – wo 2 determine the behaviour of the response.
2 – wo 2 determine the behaviour of the response.
α = wo then s1 and s2 are equal and real numbers ; no oscillatory behaviour
Critically Damped System.
α>wo and s1 and sr are real numbers but not equal and no oscillatory behaviour then it is over damped system.
Vc = Vs + A1 es1t + A2 e s2t
α<wo 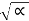 2 – wo 2 = j
2 – wo 2 = j 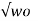 2 – α 2 . In this case the roots s1 and s2 are complex numbers.
2 – α 2 . In this case the roots s1 and s2 are complex numbers.
s 1 = - α + j 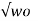 2 – α 2
2 – α 2
s2 = - α - j 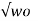 2 – α 2
2 – α 2
The system exhibits underdamped system.
This is only applicable to circuits with linear elements.
If two or more than two independent sources (voltage or current) are operating in the circuit than voltage across any element or current through any element is sum of current and voltages due to individual sources.
Question 1. Find the current through 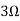 resistance.
resistance.
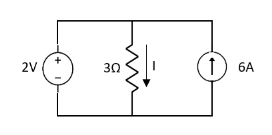
Solution:
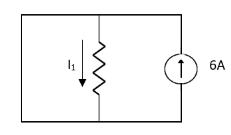
 1= 0
1= 0
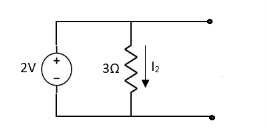
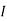 2=
2=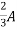
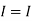 1 +
1 + 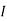 2
2 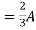
Special Case:
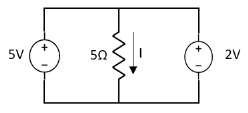
Since two voltage sources with different magnitude in parallel which cannot be connected as in single branch two different current is not possible (if 5V than I = zero).
Question:
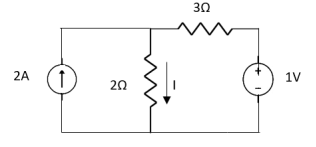
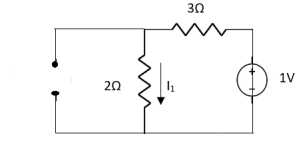
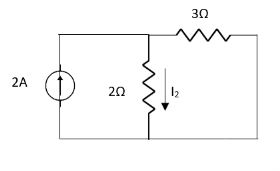
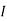 1 =
1 = 
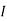 2 =
2 = 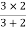
=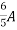
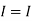 1 +
1 +  2
2
= 
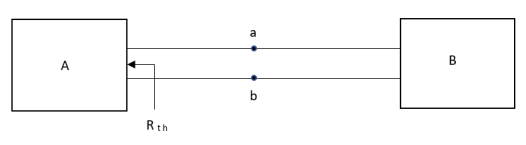
Thevenin’s equivalent of A
Norton’s equivalent of A
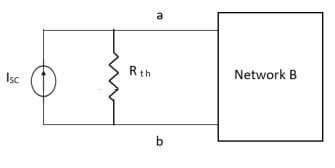
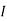 sc = Vth/Rth
sc = Vth/Rth
- Norton’s equivalent is obtained by source conversion of thevenin’s equivalent circuit.
CONDITIONS FOR APPLICATION
- For network A:
- Network A should contain linear elements.
- Network A can have independent and dependent current and voltage source.
- If network A has dependent source than controlling parameter must lie in network A itself.
- Network A should not have any source coupling and magnetic coupling.
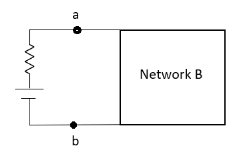
- For network B:
- It can have linear and non linear elements.
- It can have dependent and independent voltage and current sources.
- It should not have any source and magnetic coupling with network A.
Method for finding Rth :
Firstly, open circuit terminal A and B.
- If network is operating with only independent sources:
- Make all sources zero in network A.
- Find out the equivalent resistance across terminal A and B.
2. If network A is operating with independent and dependent sources:
- Make all independent sources zero in network A.
- Connect a generation between A and B.
3. If network is operating with only dependent sources:
Connect generation between A and B
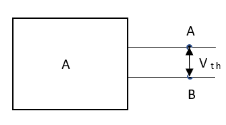
Method for Vth:
First open circuit terminal A and B.
Find out the voltage between A and B this is Vth
Method for Isc:
- Isc =
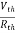
- Remove network B and S.C. The terminal A and B and current from terminal A to B Isc.
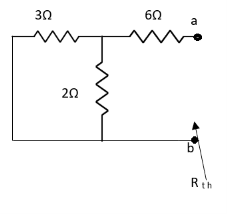
Question:
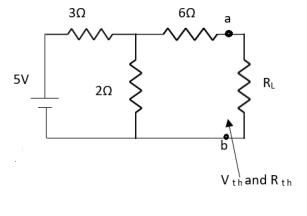
Answer:
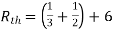
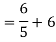
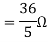
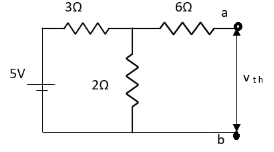
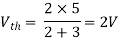
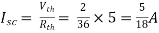
Finding Isc from circuit directly:

By KCL,
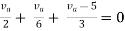
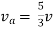

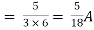
Question:
Answer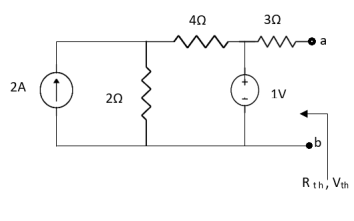
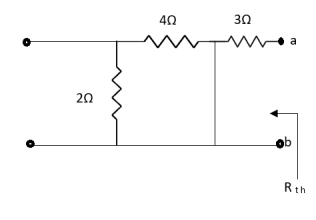
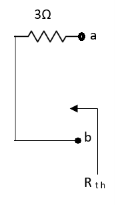
Also, clear from circuit that Vth = 1V.
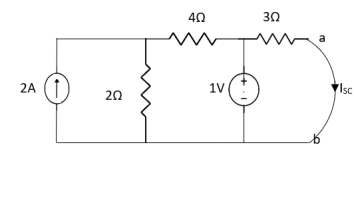
By applying KVL we get,
1-3Isc=0
Isc= A
A
Que:
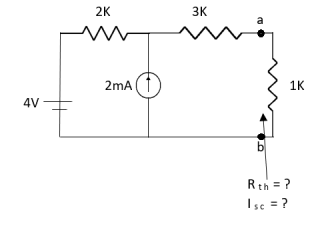
Ans;
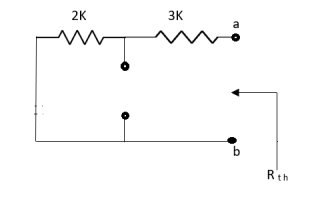
Rth=3k+2k=5k
By applying KVL we get

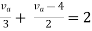
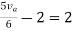
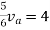
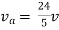
Therefore, 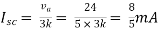
Question:
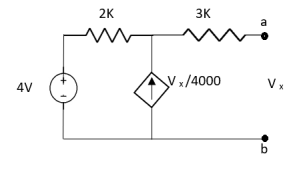
Solution: For Rth

By KCL,
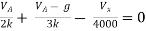
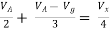
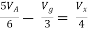
But, 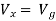
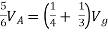
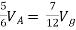
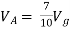

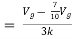
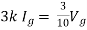
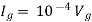
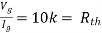
By KVL,
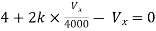
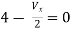
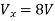
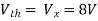
Question:
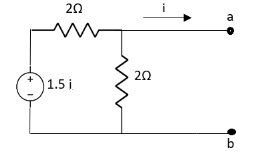
Solution: Since, no independent source is present so,
Isc = 0
And we know that,
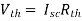
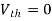
Since Rth cannot be zero

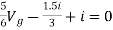
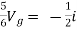
But 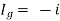
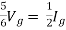
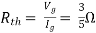
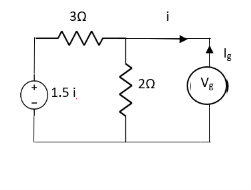
Question: Find out the Norton’s equivalent
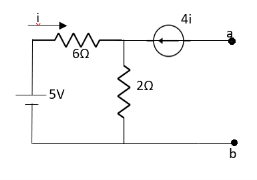
Solution:
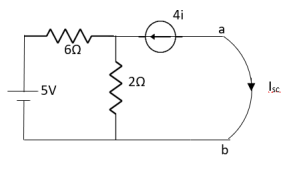
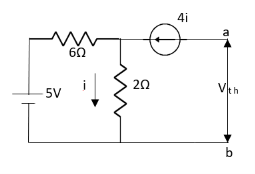
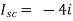
Since, there is no significance of current source