Unit - 4

Symmetrical Fault Analysis

4.1 Symmetrical Fault Analysis

INTRODUCTION

A direct axis quantity is one whose magnetic effect is along the field pole axis. Field pole axis is also known as direct axis. A direct axis synchronous reactance is defined as the reactance offered to the armature flux when the peak of armature mmf is directed along the direct axis.

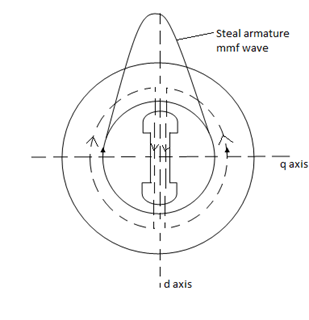
Under this condition, the air gap length is minimum and hence the reluctance is also minimum. Thus, the armature flux (Flux= mmf/reluctance) is maximum in this case.

Under this condition, the flux linkage per ampere in the armature winding is called direct axis synchronous inductance Ld.

Where Nph is the number of turns per phase of armature winding.

Hence, Xd = WLd = 2πfLd   ohm

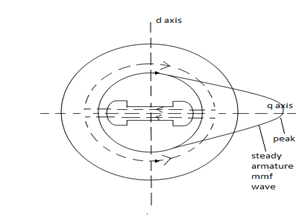
Quadrature axis synchronous reactance

A quadrature axis is one whose magnetic effect is along the perpendicular to field pole axis. When the peak of armature mmf coincides with the quadrature axis., the reactance offered to the armature mmf is called quadrature axis reactance. In the above fig, the peak of armature mmf is along the perpendicular to the field poleaxis or direct axis.

Under this condition, the reactance offered by the two large air gap is maximum. For a given armature current, the armature mmf is constant and hence under this case the armature flux (FLUX =mmf/reluctance) is minimum.

The flux linkage per armature ampere is called quadrature axis or q-axis synchronous inductance Lq.

Xq = WLq= 2πfLq   ohm

In case of salient pole synchronous machine, the air gap is non-uniform. Therefore, Xd> Xq. But due to uniform air gap in cylindrical pole synchronous machine, both direct and quadrature axis synchronous reactance are equal. i.e. Xd = Xq. In fact, due to presence of rotor slots along q-axis, Xq is slightly less than Xd for cylindrical pole synchronous machine.

In short circuit studies, only the direct axis reactances are involved.

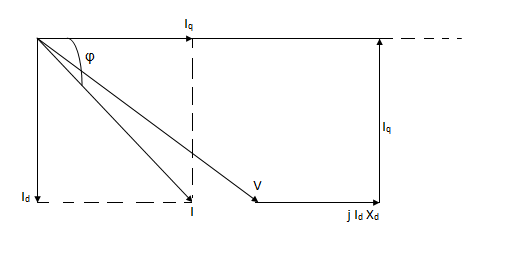
Short circuit current in general feed through lines or transformers where the inductive reactance is quite large in comparison to resistance, making the power factor approach zero as currents go lagging and when the armature current is in quadrature (lagging) with the excitation voltage (or no load voltage) the entire armature mmf acts directly upon the magnetic paths through the salient pole field windings.

Fault–Any defect in the electrical circuit on network which deviate the current from its intended path is called as fault.

The fault which results into symmetrical currents (3 line currents of equal magnitude with 120° displacement) is called as symmetrical fault. In other words, “A fault involving all the three phases is known as symmetrical fault.”

The reasons of faults in electrical power system are-

1. Failure of insulation
2. Mechanical failures
3. Excessive internal and external electric stress
4. Heavy winds
5. Lightening strokes
6. Falling of trees on the lines
7. Over voltage/ under voltage due to switching surges.
8. Vehicle accidents with poles/towers, etc

All electrical installation have to be protected against short circuits without exception whenever there is an electrical discontinuity which more generally corresponds to a change in conductor cross-section.

The short circuit current shall be calculated at each stage in the installation for the various configurations that are possible within the network, in order to determine the characteristics of the equipment that has to with stand or break this fault current.

4.2 3 Phase short circuit analysis of unloaded alternator

In case of symmetrical short circuit i.e. short circuit on all the output terminals of a three phase alternator, the flux linkages in the armature circuit and the field circuit cannot change suddenly by the application of short circuit to the armature winding. For maintaining these flux linkages constant, large changes of current may take place in both of the windings when the short circuit occurs in order to keep their respective flux linkages constant.

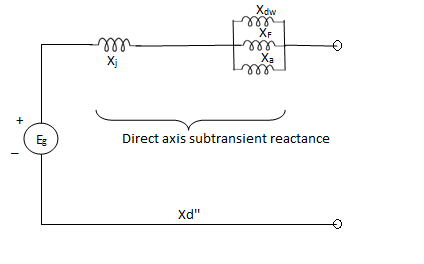
In case of an RL series circuit, reactance X(wl) is constant quantity whereas in case of the synchronous generator, the reactance is not a constant one but it is a function of time. Thus, we have three reactance’s- direct axis sub transient reactance (Xd’’), direct axis transient reactance(X’d) and direct axis synchronous reactance(Xd).

Under normal no load condition of operation, there is no mmf due to armature reaction. When a sudden 3 phase short circuit occurs at the terminals of a synchronous generator, the current in the armature circuit increases suddenly to a large value (the symmetrical short circuit current is limited only by the leakage reactance of the machine) and since the resistance of the circuit is negligible as compared to its reactance, the current is highly lagging and the power factor is approximately zero. A sudden increase in armature current is accompanied by armature reaction. Since the air gap flux cannot change instantaneously, to counter the demagnetization of the armature short circuit current, currents appear in the field winding as well as in the damper winding in a direction to help the main flux. Thus, during initial part of the short circuit, the equivalent cut appears as

The effect of damper windings is to increase the initial value of short circuit current and to maintain a slightly increased current for three or four cycles after the short cut. When the main flux assumes the value it would have had if there has been no damper windings falls to zero after three or four cycles.

As the damper winding currents are first to die out, Xdw effectively becomes open circuited and at later stage Xf becomes open circuited.

The reactance represented by the machine in the initial period of the short circuit is called sub transient reactance Xd.

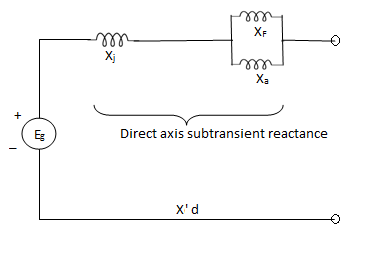
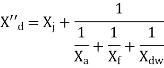
While the reactance effective after the damping winding currents have died out is called the transient reactance X'd.

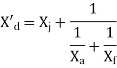
The reactance under steady state short circuit conditions is the synchronous reactance of the machine.

X”d<X'd<Xd

Analysis of short circuit current in three phases of a synchronous generator

When a short circuit occurs across the terminals of a synchronous generator, the initial short circuit current is limited by the sub transient reactance for a few cycles, later on it is controlled by the transient reactance. Finally, the short circuit current settles down to the steady state short circuit value limited by the synchronous reactance of the machine. Depending upon the value of the phase voltage at the instant of short circuit will have least asymmetry in the short circuit waves.

4.3 Sub-transient, Transient and steady state current and impedances

a) Sub transient current and impedance-

During the initial part of the short circuit on an unloaded alternator, the damper and field windings have transformer currents induced in them.

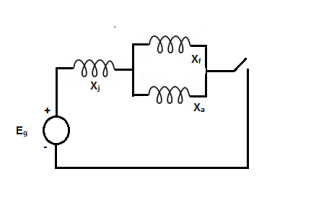
So that the circuit model includes the damper winding and field winding reactance in parallel with armature reactance.

The approximate circuit model during sub transient period of short circuit is shown below-

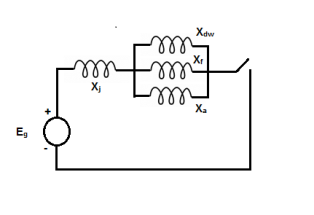
The sub transient reactance and current is given by-

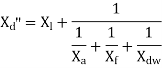
excluding dc component of current.

b) Transient current and impedance

After first three to four cycles of short circuit, the current in damper winding first die out and the damper winding reactance effectively becomes open circuited.

The approximate circuit model during transient period of short circuit is shown below-

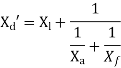
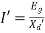  excluding dc component of current.

c) Steady state current and impedance

In the last stage, when the main flux attain the original value before the short circuit occurs. The field winding reactance is cut out from the circuit. The armature current settles to steady state value.

The approximate circuit model during steady state period is shown below-

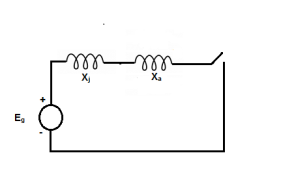
Xd = Xl + Xa

4.4 D.C. Offset and effect of the instant of short-circuit on the waveforms

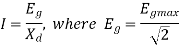
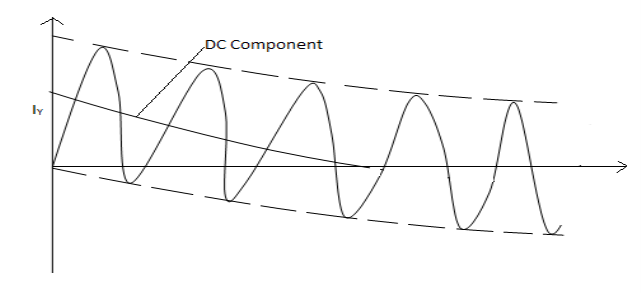
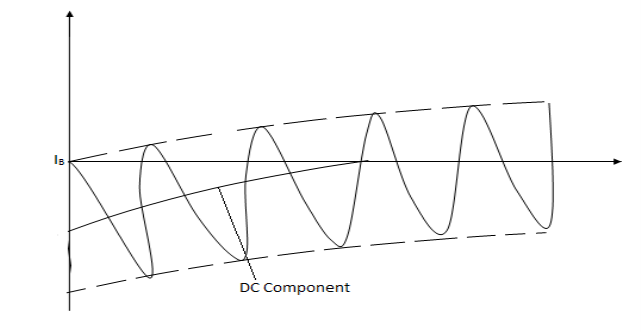
The current can be split up into unidirectional or asymmetrical component and an alternating component of supply frequency. The DC component represents the displacement of the AC wave from the zero axis and is therefore sometimes known as DC offset current.

The short circuit period can be divided into three periods - initial sub transient period, lasting only for the first few cycles during which the current decrement is very rapid; the middle transient period, covering a relatively longer time during which the current decrement is moderate and finally the steady-state period. The short circuit current for 1 phase with DC component eliminated is shown in following figure.

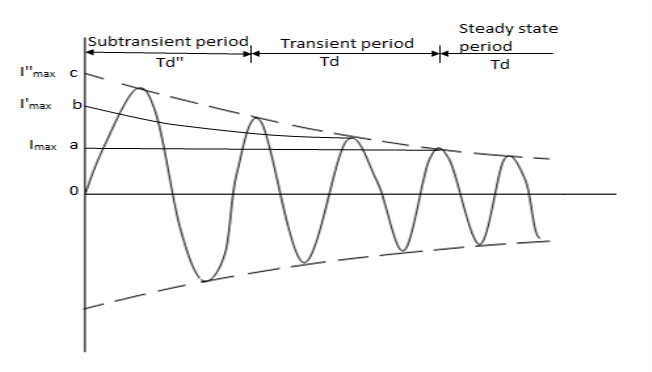
Sub transient current (maximum) excluding dc component,

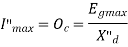
Transient current (maximum) excluding dc component,

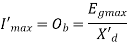
Steady state current (maximum)

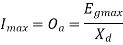
Where Egmax is the maximum voltage from one terminal to neutral on no load.

Sub transient current (rms) excluding dc component

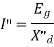
Transient current (rms) excluding dc component

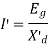
And steady state current (rms)

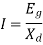
WhereEg = 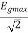  , the rms voltage from one terminal to neutral.

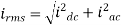
Where maximum dc current,

And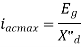
 

Thus,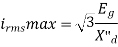
 

The momentary shortcut which a breaker should be capable of carrying is given by above equation.

4.5 Estimation of fault current without pre-fault current for simple power systems

Limitations to fault current

An occurrence of a short circuit at any point in a system the short circuit current is limited by the impedance of the system up to the point of fault.

Also, the short circuit current depends on the size of the power system which supplies the short circuit current. Larger the supply source, greater will be the short circuit current. This is because of-

1. Large kVA of the generating plant.
2. Smaller impedance of the generating plant. Larger the size of the generating plant, smaller will be impedance.
3. Smaller impedance between the generating station and the point of fault, larger the current carrying capacity of the line, the lower will be the impedance.

Example 1-

A three phase 400V 75kW induction motor connected to infinite bus bar

i) 100kVA transformer 

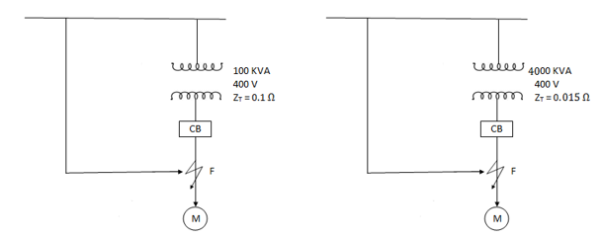
(ii) 4000 kVA transformer.

Full load motor current,

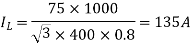
Assuming pf to be 0.8 lagging,

a)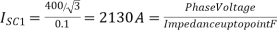

b)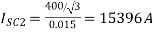

Thus, it us observed that as the system grows, the capability of supply of short circuit will be increased along with it.

The analysis of symmetrical (L-L-L) faults includes determination of the voltage at any point in the power system network, the current in any branch and the value of reactance necessary to limit the fault current to any desired value. Such calculations provide the necessary data for selection of circuit breakers and design of protective scheme. The circuit breaker MVA breaking capacity is based on a three phase fault MVA.

The following assumptions are made during the short circuit analysis:

1. Load currents are considered negligible as compared to fault currents.
2. The transformer is represented by the reactance in series as Transformer resistance is quite low in comparison with its reactance.
3. Shunt capacitances of the transmission lines are neglected
4. System resistance is neglected and only inductive reactance of the system is taken into account. A transmission line is represented by series reactance and resistance.
5. The EMFs of all the generators are assumed to be equal to 1<0° per unit.
6. The effect of DC component is accounted for by using correction factors.

Network Reduction Technique

Because of the balanced nature of fault and the system, any condition which applies to 1 phase supply apply equally to the remaining two phases.

1. Make out a single line diagram of the complete network indicating on each component its rating, voltage, resistance and reactance.
2. Choose a common base kVA (or MVA) and convert all the resistances and reactance in per unit values as referred to common base kVA(or MVA).
3. From single line diagram, draw single line reactance diagram showing 1 phase and neutral. In this diagram, write down reactance or impedances of the elements in per unit values.
4. Reduce the reactance diagram by network reduction techniques keeping the identity of the point intact. Find the reactance of the system as seen from the fault Point (Thevenin reactance)
5. Determine the fault current and fault MVA in per unit. Convert these per unit values to actual values.

Fault current and Fault MVA (steady state)

Isc per unit =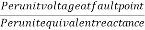

Per unit fault MVA = √3 × per unit fault current × per unit source voltage

Or Fault MVA = 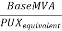  MVA lagging

4.6 Selection of Circuit-Breakers and Current Limiting Reactors and Their Location in Power System (Descriptive Treatment Only)

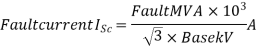
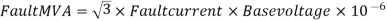
Current limiting Reactors

A Current limiting reactor, also sometimes called a series reactor is an inductive coil having a large inductive reactance in comparison to its reactance and is used for limiting short circuit current during fault conditions.

These are installed in feeder and ties, in generator leads and between bus sections to reduce the magnitude of short circuit currents and the effect of the resulting voltage disturbances.

Their cost is often more than offset by the savings in circuit breaker cost as a result of the lower short circuit ratings that can be used. Therefore, their use is restricted to the interconnection of large power systems.

The reactors allow free interchange of power under normal conditions but under short circuit conditions the disturbances are confined to the faulty section.

Principle of operation of current limiting reactors-

Short circuit currents are reduced by an increase of the percentage reactance in the system. The shortcut current depends upon the generating capacity, voltage at the fault point and the total reactance between the generators and the fault.

The circuit breaker should have enough breaking current capacity more than the expected fault currents.

If the fault currents are beyond the capacity of the circuit breaker, the fault currents may not be interrupted.

In large interconnected systems, the total ratings of the generator is very high and therefore, the fault currents are very high. There is possibility that the circuit breakers of suitable breaking capacity may not be available.

Also, when the system is extended by the addition of more generating units or by a supply from the grid, the fault currents to be interrupted by the same circuit breaker will become greater than before.

As it is uneconomical to scrap the existing switchgear, which was adequate for the former output but is not of sufficient capacity to meet the extensions.

In such a case, there is a need of providing a protective reactor between the old system and the extensions for limiting the short circuit currents to permissible value.

Primary functions-

1. Protective reactors are used to reduce the flow of current into a short circuit.
2. It is used to reduce the magnitude of voltage disturbances caused by short circuit.
3. They also localise the fault by limiting the current that flows into the fault from other healthy feeders or parts of the system.
4. They reduce the duty imposed on switching equipment during short circuits to be within economical ratings.

Drawbacks –

With the introduction of the reactors, total percentage reactance of the circuit increases, thereby causing increase in reactive voltage drop and decrease in power factor due to increased angle of lag. Thus, regulation becomes poorer.

Selection of reactors-

1. Reactance in ohms or in percentage
2. Normal current rating
3. Short time current rating
4. Rated voltage
5. Rated through-put KVA
6. Type (dry or oil immersed, air cored or iron cored)
7. Number of phases
8. Indoor or outdoor
9. Circuit characteristics (frequency voltage).

Location of reactors-

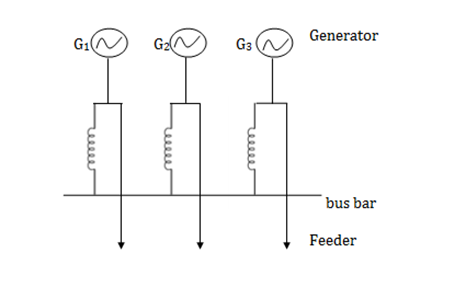
i) Generator reactors (in series with generators) -These reactors are inserted between the generator and the generator bus. Such reactor protect the machines individually.

Magnitude of such reactors very about 5% or 0.05pu.

A short circuit occurs on 1 feeder, the voltage at the generator bus may drop to such a value that the synchronous machine connected to this common bus bar may fall-out of step.

During normal operation, a full load current is always flowing through these reactors during normal operation resulting in a constant voltage drop and constant power loss.

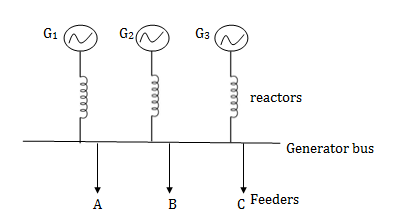
Ii) Feeder reactors-

These reactors are connected in series with feeders.

In an event of fault on anyone feeder, the main voltage drop is in its reactor only and the bus bar voltage is not affected much, hence other machines continue supplying load.

Also fault on a feeder will not affect other feeders and thus the effects of false are localised

These reactors do not provide protection to the generators against short circuit fault across the bus bars.

The constant voltage and power drop under normal operation is also drawback.

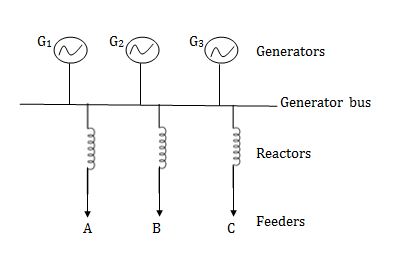
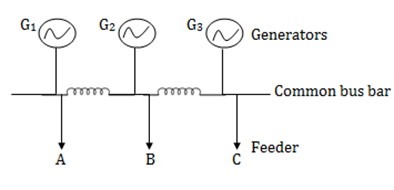
Iii) Bus bar reactors-

The constant voltage drop and constant power loss in reactors may be avoided by inserting the reactors in the bus bars.

(a)  Bus bar reactors ring system

(b) Bus bar reactors tie bar system

4.7 Numerical Based on symmetrical fault analysis

Example 1-

A 3 phase 10000 kVA, 11 KV alternator has a sub transient reactance of 8℅. A three phase short circuit occurs at its terminals. Determine fault current and fault MVA.

Soln. - Base current,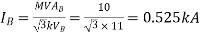

Isc per unit =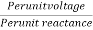

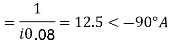
Fault current, Isc =Isc per unit × IB

=12.5 × 525 =6562 A

Fault power = 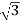× Isc× source voltage × 10-6 MVA

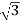= × 6562 × 11000 × 10-6 MVA

=125 MVA

Example-2

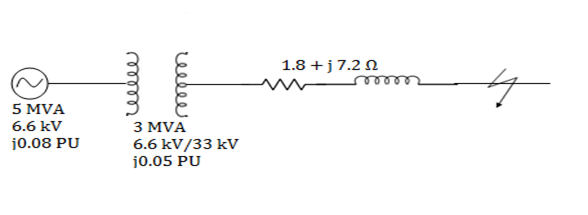
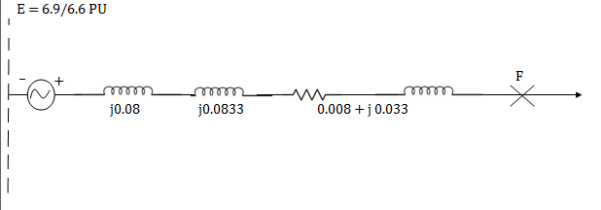
A 3 phase, 5 MVA, 6.6 kV alternator with a reactance of 8% is connected to a feeder of series impedance (0.12+i0.48) ohm/phase/km. The transformer is rated at 3 MVA, 6.6 kV /33 kV and has a reactance of 5%. Determine fault current supplied by the generator operating under no load with a voltage of 6.9kV, when a three phase symmetrical fault occurs at a point 15 km along the feeder.

Soln. Base MVA for complete system = 5MVA

Base kV = 33kV for feeder side

Xalternator (pu) = 0.08 pu

XT (New per unit) = XT (old pu) ××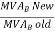

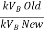
= 0.05 × (5/3) = i0.08333 pu

Impedance of feeder of length 15 km,

Zf = 15 × (0.12+i0.48) = 1.8 + i7.2 ohm = 7.42 < 76˚ ohm

Per unit reactance of feeder, Zfpu = Actual impedance ×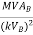

= (7.42<76)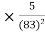

=0.034 pu< 76˚

=0.008 + i0.033 pu

Per unit fault current, Iscpu =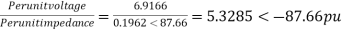

Base current IB =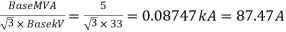

Fault current = Iscpu × IB=(5.3285< -87.66) ×87.47 = 466 < -87.66˚A

Example -3

Two 3 phase 11 kV generators of capacities 8MVA and 4MVA and sub transient reactances of 8% and 4% respectively operate in parallel. The generating station is connected to a transmission line of 200 km length through a step up Transformer of capacity 4 MVA and having percentage reactance of 3.5%. The resistance and reactance of the transmission line per km of its length are 0.025 ohm and 0.1 ohm respectively and it operates at 66 kV. Calculate the short circuit MVA for a phase to phase faults at the receiving end of the transmission line and at the sending end.

Soln.

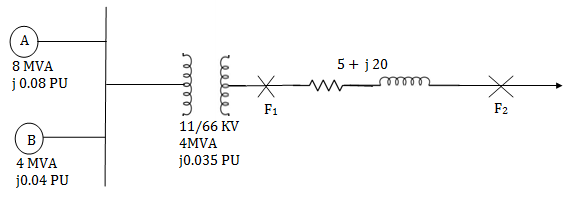
Base MVA for complete system = 8 MVA

Base kV for generator side = 11 kV

Thus, per unit reactance of generator A and B

XA(pu) = i0.08 pu

XB(pu) =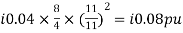

Per unit reactance of transformer = 0.035 × 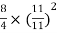= i0.07 pu

Impedance of transmission line = 200 × (0.025 + i0.1) = 5 + i20

Per unit impedance of transmission line = (5 + i20) ×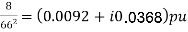

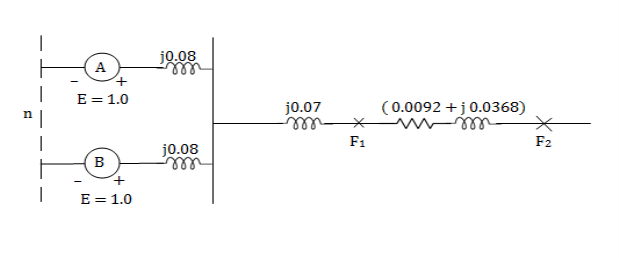
The single line reactance diagram can be drawn as-

Reduced reactance diagram can be drawn

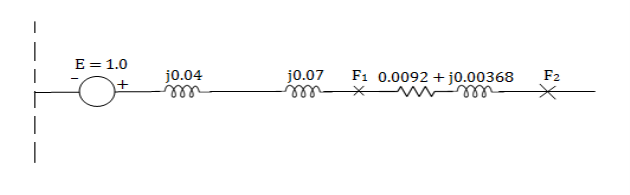
For a 3 phase fault at the sending end (F1) side of the transmission line, the total impedance upto the fault point

= i0.04 + i0.07 = i0.11

Fault MVA at F1 = =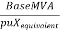

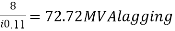
For a 3 phase fault at the receiving end(F2) side of the transmission line, the total impedance upto the fault point = i0.04 +i0.07 + (0.0092 +  i0.0368)

= 0.0092 + i0.1468

Fault MVA at F2 = = 54.38 MVA

Example-4

Two generators A and B are identical and rated 11kV, 10mVA and have a transient reactance of 20% at their own MVA base. Two transformers T1 and T2 are also identical and are rated 5mVA, 11166 kV and have a reactance of 5% at their own MVA base. The tie line is 100km and has a reactance of  0.1 ohm/km. A 3 phase fault occurs at a distance of 25km. From one end of the line when the system is on no load but at rated voltage. Determine fault MVA and fault current.

Soln. –

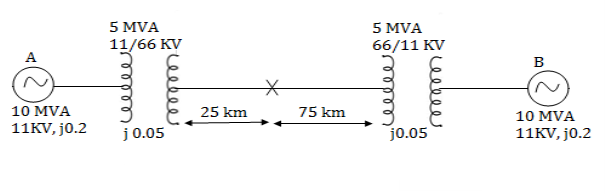
Let base MVA of the system be 10MVA, base KV of the generator side 11kV and for transmission circuit side 66kV.

For generator A, B

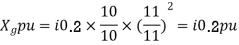
For transformer T1 and T2,

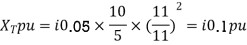
Per unit reactance of 25 km line =

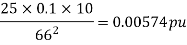
Per unit reactance of 75 km line =

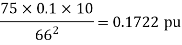
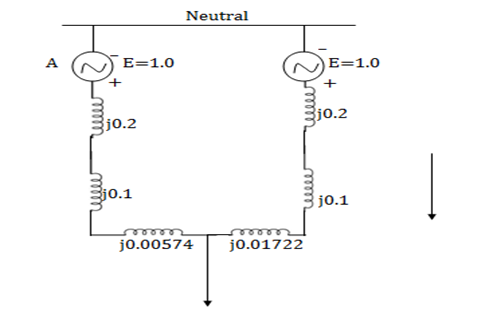
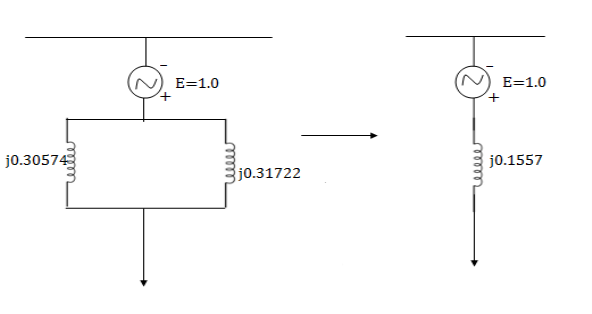
Xeqpu = i30574 || i0.31722

= i0.1557 pu

Fault MVA =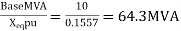

Fault current, Isc=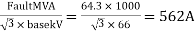

Example-5

Determine the required MVA rating of the circuit breaker CB for the system shown in fig. Consider the grid as infinite bus. Choose 6MVA as base.

Transformer: 3 phase, 33111kV, 6MVA,

0.01+i0.08 pu impedance

Load: 3 phase, 11kV, 5800kVA, 0.8 lag, i0.2 pu impedance

Impedance of each feeder: 9+i18

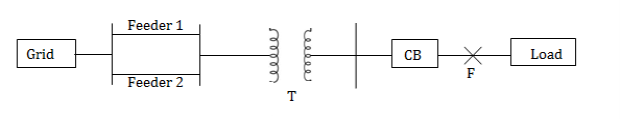
Soln. Base MVA =6, Base kV for grid side is 33 kV and for load side is 11 kV.

Per unit impedance of transformer= 0.01   i0. 08 pu

Per unit impedance of feeder =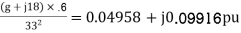

Per unit impedance of load =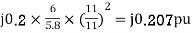

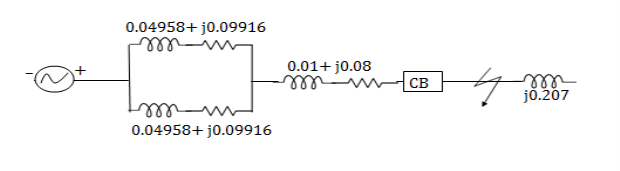
Equivalent impedance upto fault point,

Zeqpu = [(0.04958+j0.09916)||(0.04958+j0.09916)]+(0.01+j0.08)

=(0.02479+j0.4958)+(0.01+j0.08)

=0.03479+j0.12958

=0.13417<74.97˚

Short circuit rating of CB =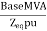 = = 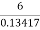44.72MVA

References:

1. H. Hadi Sadat: Power System Analysis, Tata McGraw-Hill New Delhi.
2. G. W. Stagg and El- Abiad – Computer Methods in Power System Analysis – Tata McGraw Hill, New Delhi.
3. M.E. El-Hawary, Electric Power Systems: Design and Analysis, IEEE Press, New York.
4. Rakash Das Begamudre, “Extra High voltage A.C. Transmission Engineering”, New age publication.
5. M.A.Pai, Computer Techniques in Power System Analysis, Tata McGraw Hill Publication.
6. Stevenson W.D. Elements of Power System Analysis (4th Ed.) Tata McGraw Hill, New Delhi.