Unit-4
Concept of Boundary layer
Boundary layer is the thickness of that layer next to a surface in which the velocity grows from zero to a maximum value (or so close to a maximum as to be of no practical difference). This thickness is usually given the symbol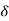 
(small delta).
The boundary layer, once established may have a constant thickness but, for example, when a flow meets the leading edge of a surface, the boundary layer will grow as shown in figure below.
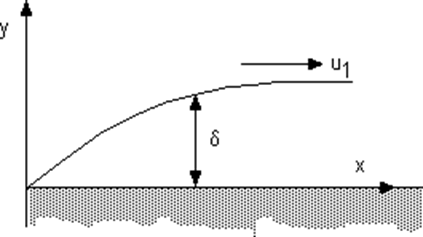
Figure 1: Boundary layer
Boundary layer is defined as:
Boundary Layer Thickness, δ Boundary layer thickness is defined as that distance from the surface where the local velocity equals 99% of the free stream velocity.
δ = y(u=0.99Us)
Thickness of Boundary Layer in A Laminar Flow:
It has been experimentally found, that the thickness of the boundary layer is zero at the leading-edge, and increases to the trailing edge, the flow is laminar.
Thickness of Boundary Layer in a Turbulent Flow:
As the boundary layer continuous further downstream, it expands and the transition flow changes into turbulent flow and the transition boundary layer changes into turbulent boundary layer, which continuous over the remaining length of the plate.
According to Prandtl-Blassious,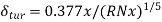 
Some other definitions of Boundary layer Thickness:
Many other definitions of boundary layer thickness has been introduced at different times and provide important concepts based on mathematical calculations and logic These definitions are:
Key Takeaways:
The flow rate within a boundary layer is less than that for a uniform flow of velocity u1. The reduction in flow is equal to the area under the curve in figure given below. If we had a uniform flow equal to that in the boundary layer, the surface would have to be displaced a distance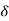 
* inorder to produce the reduction. This distance is called the displacement thickness and it isgiven by:
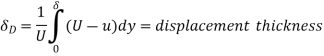
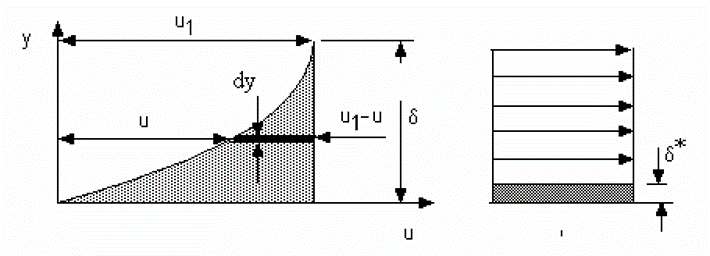
Another interpretation of the displacement thickness is that because the volumetric flow in the boundary layer is actually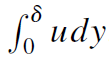
the same flow would be achieved if the wall was displaced upward into the flow in an amount
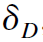since the definition can be written in the form
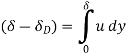
Key Takeaways:
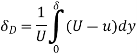
Momentum Thickness, θ Momentum thickness is the distance that, when multiplied by the square of the free stream velocity, equals the integral of the momentum defect. Alternatively, the total loss of momentum flux is equivalent to the removal of momentum through a distance θ. It is a theoretical length scale to quantify the effects of fluid viscosity near a physical boundary.
Momentum thickness is given by:
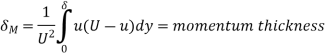
The momentum in a flow with a boundary layer present is less than that in a uniform flow of the same thickness. The momentum in a uniform layer at velocity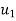 
and height h is 
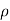h
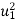. When a boundary layerexists, this is reduced by 
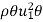. Where 
is the thickness of the uniform layer that contains the equivalent to the reduction.
If density is constant, this simplifies to
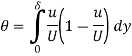
Key Takeaways:
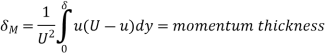
Required formula for momentum thickness
Energy thickness (δe) is defined as the thickness of an imaginary layer in free stream flow which has energy equal to the deficiency of energy caused to actual mass flowing inside the boundary layer.
By equating the energy transport rate for velocity defect to that for ideal fluid
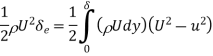
If density is constant, this simplifies to
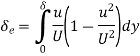
Kinetic energy of fluid, if plate is placed = ½ m V2 = (ρ u b dy) u2
Kinetic energy of fluid if no plate is placed = ½ m V2 = (ρ u b dy) U2
Loss of Kinetic energy = ½ ρ u b (U2 - u2) dy
Total loss of Kinetic energy in BC
= 1/2 ρ u b ∫ ( U2 - u2) dy
Loss of Kinetic energy through ( ) of fluid flowing with velocity U.
= ½ mass x velocity2 = ½ (ρ b δe U) U3
= ½ (ρ b δe) u2
Equating Equations
1/2 ρ b δe
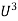
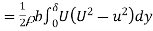
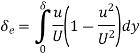
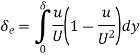
Key Takeaways:
It is the distance perpendicular to solid boundary, by which the boundary should be displaced to compensate for the reduction in kinetic energy of the flowing fluid in the formation of boundary layers.
References:
