Unit - 5
Effects of inertia of reciprocating masses on engine frame
Figure 5.1: Reciprocating engine mechanism.
Let,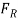
= Force required to accelerate thereciprocating parts
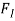= Inertia force due to reciprocating parts
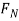= Force on the sides of the cylinder walls or normal force acting onthe cross-head guides
= Force acting on the crankshaft bearing or main bearing
Since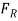 
and 
are equal in magnitude but opposite in direction, therefore they balanceeach other. The horizontal component of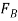 
(i.e.,
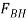) acting along the line of reciprocation is alsoequal and opposite to 
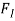. This force 
= 
is an unbalanced force or shaking force and required to be properly balanced.
The force on the sides of the cylinder walls (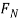
) and the vertical component of 
(i.e.,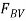
) are equal and opposite and thus form a shaking couple of magnitude 
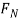× x or 
× x.
Thus, the purpose of balancing the reciprocating masses is to eliminate the shaking force and a shaking couple. Therefore, the reciprocating masses are only partially balanced.
Key takeaway:
The masses rotating with the crankshaft are normally balanced and they do not transmit any unbalanced or shaking force on the body of the engine.
Let, m = Mass of the reciprocating parts,
l = Length of the connecting rod PC,
r = Radius of the crank OC,
= Angle of inclination of the crank with the line of stroke PO,
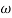= Angular speed of the crank,
n = Ratio of length of the connecting rod to the crank radius = l / r.
The acceleration of the reciprocating parts is-
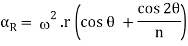
Inertia force due to reciprocating parts =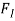 
= 
= Mass × acceleration =
The horizontal component of the force exerted on the crank shaft bearing (i.e.,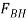
) is equal and opposite to inertia force (
). This force is an unbalanced one and is denoted by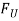 
.
Unbalanced force,
Here,
(
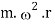cos θ )is known as primary unbalanced force and
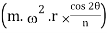is called secondary unbalanced force.
Key takeaway Point:
- The primary unbalanced force is maximum, when
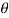= 0° or 180°. Thus, the primary force ismaximum twice in one revolution of the crank. The maximum primary unbalanced force is given by
2. The secondary unbalanced force is maximum, when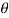 
= 0°, 90°,180° and 360°. Thus, the secondaryforce is maximum four times in one revolution of the crank. The maximum secondary unbalanced force isgiven by
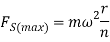
3. We observed that secondary unbalanced force is 1/n times the maximum primary unbalanced force.
4. In case of moderate speeds, the secondary unbalanced force is so small that it may be neglected as compared to primary unbalanced force.
5. The unbalanced force due to reciprocating masses varies in magnitude but constant in direction while due to the revolving masses, the unbalanced force is constant in magnitude but varies in direction.
5.3.1. Balancing of Primary Forces of Multi-cylinder In-line Engines:
In-line engines: The multi-cylinder engines with the cylinder centre lines in the same plane and on the same side of the centre line of the crankshaft, are known as In-line engines.
The following two conditions must be satisfied in order to give the primary balance of the reciprocating parts of a multi-cylinder engine:
1. The algebraic sum of the primary forces must be equal to zero. In other words, the primary force polygon must close.
2. The algebraic sum of the couples about any point in the plane of the primary forces must be equal to zero. In other words, the primary couple polygon must close.
KEYWAY POINTS:
1. For a two-cylinder engine with cranks at 180°, condition (1) maybe satisfied, but this will result in an unbalanced couple. Thus, the above method of primary balancing cannot be applied in this case.
2. For a three-cylinder engine with cranks at 120° and if the reciprocating masses per cylinder are same, then condition (1) will be satisfied because the forces may be represented by the sides of an equilateral triangle. However, by taking a reference plane through one of the cylinders center lines, two couples with non-parallel axes will remain and these cannot vanish vectorially. Hence the above method of balancing fails in this case also.
3. For a four-cylinder engine, similar reasoning will show that complete primary balance is possible and it follows that- ‘For a multi-cylinder engine, the primary forces may be completely balanced by suitably arranging the crank angles, provided that the number of cranks are not less than four’.
5.3.2. Balancing of Secondary Forces of Multi-cylinder In-line Engines:
When the connecting rod is not too long (i.e., when the obliquity of the connecting rod is considered), then the secondary disturbing force due to the reciprocating mass arises. The secondary force,
i.e.
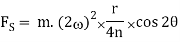
Figure 5.2: Secondary force
In multi-cylinder in-line engines, each imaginary secondary crank with a mass attached to the crankpin is inclined to the line of stroke at twice the angle of the actual crank. The values of the secondary forces and couples may be obtained by considering the revolving mass.
The following two conditions must be satisfied in order togive a complete secondary balance of an engine:
1. The algebraic sum of the secondary forces must be equal to zero. In other words, the secondary force polygon must close.
2. The algebraic sum of the couples about any point in the plane of the secondary forces must be equal to zero. In other words, the secondary couple polygon must close.
Key takeaway:
The closing side of the secondary force polygon gives the maximum unbalanced secondary force and the closing side of the secondary couple polygon gives the maximum unbalanced secondary couple.
The locomotives, usually, have two cylinders with cranks placed at right angles to each other in order to have uniformity in turning moment diagram.
The two-cylinder locomotives maybe classified as:
1. Inside cylinder locomotives: In this locomotive, the two cylinders are placed in between the planes of two driving wheels as shown in Fig.5(a).
2. Outside cylinder locomotives: In this locomotive, the two cylinders are placed outside the driving wheels, one on each side of the driving wheel, as shown inFig.5(b).
The locomotives may be:
(a) Single or uncoupled locomotives: This locomotive is one, in which the effort is transmitted to one pair of the wheels only.
(b) Coupled locomotives: In this locomotive, the driving wheels are connected to the leading and trailing wheel by an outside coupling rod.
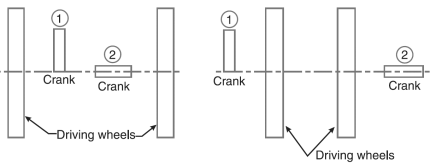
Figure 5.3(a): Inside cylinder locomotives Figure 5.3(b): Outside cylinder locomotive.
References:
- Dr. V. P. Singh, Theory of Machine, Dhanpatrai, and sons.
- C. S. Sharma & Kamlesh Purohit, “Theory of Machine and Mechanism”, PHI.
- Sadhu Singh, Theory of Machines, Pearson
