Unit - 1

Introduction

1.1 Introduction to three modes of heat transfer

Introduction

• Heat is from of energy that flows due to difference in temperature.
• Heat flows from higher temperature to lower temperature.
• Heat energy can neither be observed nor measured directly. However, effects produced by it’s transfer can be measured and observed.

Modes of Heat Transfer

The process of heat transfer takes place through 3 distinct modes: Conduction, Convection and radiation.

Conduction

The mechanism of heat transfer due to temperature gradient in a stationary medium is called conduction. The medium may be solid or fluid. In fluids, conduction is due to collision of molecules in the course of their random motion. In solids, conduction is attributed to:

• Flow of free electrons
• Vibration motion of molecules at their fixed position.

Fourier’s law of heat conduction states that the rate of heat transfer is linearly proportional to the temperature gradient. For one-dimensional or unidirectional heat conduction

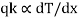
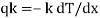..(1.1)

Where, qk is the rate of heat flux (a vector) in W/m2, dT/dx is the temperature gradient in the direction of heat flow x and k is the constant of proportionality, which is a property of the material through which heat propagates. This property of the material is called thermal conductivity (W/m K).

Convection

• The mode by which heat is transferred between a solid surface and adjacent fluid when there is a temperature difference is known as convection heat transfer.
• Convection is a process involving mass movement of fluids. When a temperature difference produces a density difference which results in mass movement the process is called free or natural convection.
• When the mass motion of the fluid is caused by an external device like a pump, compressor, blower or fan, the process is called forced convection.
• Rate of convective heat transfer is determined by Newton’s law of cooling.

It states that rate of heat transfer is proportional surface area perpendicular to heat flow direction and temperature difference between wall surface (Tw) and fluid temperature (T∞) in direction perpendicular to flow.                           

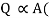Tw - T∞)

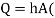Tw - T∞) ..(1.2)

Where, Q is rate of heat transfer (W), A is area in m2, h is constant known as coefficient of convective heat transfer or film coefficient with unit W/m2K.

Radiation

• Radiation is heat transfer in the form of electromagnetic waves.
• The phenomenon is identical to the emission of light.
• The rate at which energy is radiated by a black body at the absolute temperature T is given by the Stefan-Boltzmann law

..(1.3)

Where, is the Stefan-Boltzmann constant, 5.67  X 10-8 W/m2 K4.

1.2 Derivation of heat balance equation- Steady one-dimensional solution for conduction of heat transfer in Cartesian, Cylindrical and spherical geometry

Let us consider an infinitesimal volume element of side dx, dy and dz (Fig. 1). The considerations here will include the non-steady condition of temperature variation with time t.

Let T be temperature at the center of element.

Let the material be anisotropic, hence thermal conductivities have different values kx

ky and kz in x,y and z direction respectively.

Consider heat leaving and entering this volume through six faces.

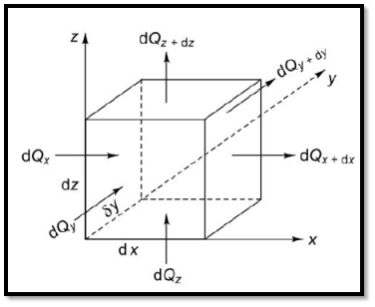
Fig. 1 Volume element for determining heat conduction equation

According to the Fourier heat conduction law, the heat flowing into the left-most face of the element in the x-direction

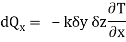
The value of the heat flow out of the right face of the element can be obtained by expanding dQx in a Taylor series and retaining only the first two terms as an approximation:

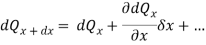
The net heat flow by conduction in the x-direction is therefore

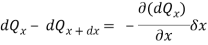
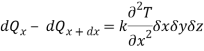
Similarly,

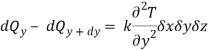
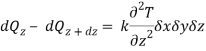
Here, the solid has been assumed to be isotropic and homogeneous with properties uniform in all directions. Let us consider that there is some heat source within the solid, and heat is produced internally as a result of the flow of electrical current or nuclear or chemical reactions. Let qG is the rate at which heat is generated internally per unit volume (W/m3). Then the total rate of heat generation in the elemental volume is

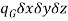
The net heat flow owing to conduction and the heat generated within the element together will increase the internal energy of the volume element. The rate of accumulation of internal energy within the control volume is

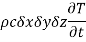
Where c is the specific heat and 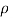 is the density of the solid.

An energy balance can be achieved on the volume element as:

Rate of energy storage within the solid = Rate of heat influx – Rate of heat outflux + Rate of heat generation

Or

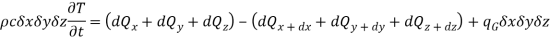
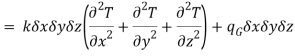
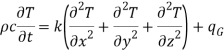
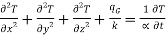…(1.4)

Where 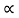is the thermal diffusivity of the solid given by 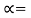k/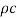. The larger the

Value of 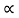, the faster heat will diffuse through the material.

This is heat balance equation in Cartesian form.

If the temperature of a material is not a function of time, the system is in the steady state and does not store any energy. The steady state form of a three-dimensional conduction equation in rectangular coordinates is

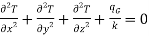…(1.5)

It is known as Poisson’s equation.

If the system is in steady state and no heat is generated internally, the conduction equation further simplifies to

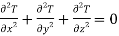…(1.6)

This is known as Laplace equation.

Consider heat flow in x-direction only with no heat generation. Hence Laplace equation reduces to

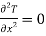…(1.7)

This equation is known as one dimensional heat conduction equation with no heat generation.

Heat conduction equation in cylindrical co-ordinates

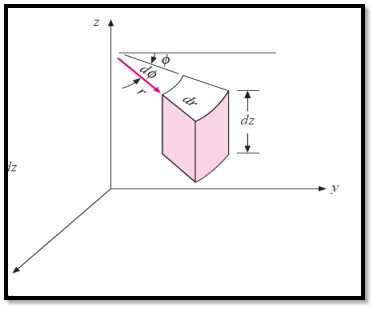
Fig 2 Heat conduction in cylindrical element

For a general transient three-dimensional heat conduction problem in the cylindrical coordinates with T = T (r, q, z, t)

By substituting x=rcos, y=rsin and z=z equation (1.4) becomes

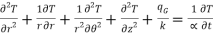…(1.8)

Considering material as isotropic and heat transfer occurring to be one dimensional in steady state equation reduces to

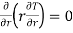…(1.9)

This equation is known as one dimensional heat conduction equation with no heat generation in cylindrical co-ordinate system.

Heat conduction equation in spherical co-ordinates

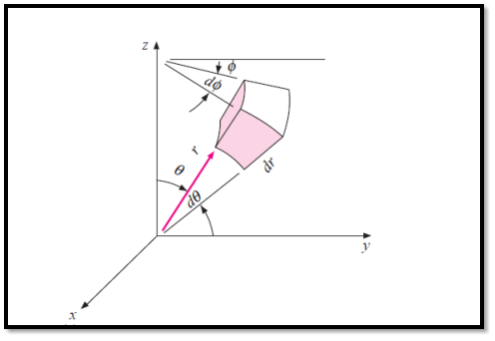
Fig 3. Heat conduction in spherical element

Consider a spherical element with co-ordinate (r,,)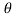

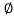Substitute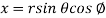

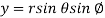
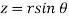
…(1.10)

Considering material as isotropic and heat transfer occurring to be one dimensional in steady state equation reduces to

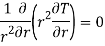
This equation is known as one dimensional heat conduction equation with no heat generation in spherical co-ordinate system.

1.3 Concept of conduction and film resistances

• Heat flow has an analogy in the flow of electricity. Ohm’s law states that the current I (Ampere) flowing through a wire is equal to the voltage potential E1 – E2 (V), divided by the electrical resistance R

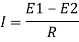
• Since the temperature difference and heat flux in conduction are similar to the potential difference and electric current respectively, the rate of heat conduction through the wall can be written as

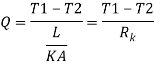
Where Rk=L/KA is conductive thermal resistance offered by thermal wall.

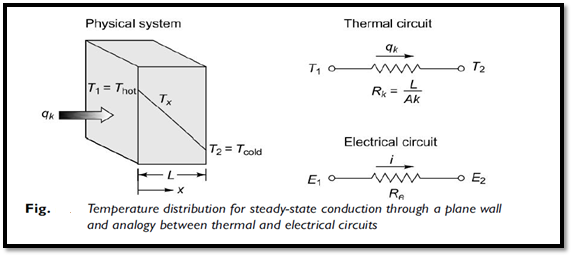
Fig 4.

Heat transfer in plane wall

From Fourier law,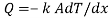

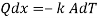
From fig 4, at x=0, T=T1

At x=L, T=T2

On integrating,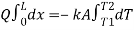

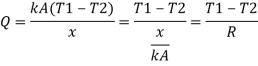
Conductive resistance R=

Heat transfer by convection

By Newton’s law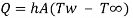

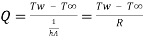
Where R=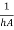 is a convective resistance.

Overall heat transfer coefficient

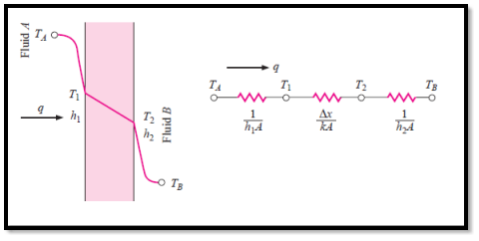
Fig. 5 Overall heat transfer through plane wall

Consider the plane wall shown in Figure 5 exposed to a hot fluid A on one side and a cooler fluid B on the other side. The heat transfer is expressed by

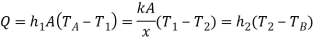
The heat-transfer process may be represented by the resistance network in Figure 5, and the overall heat transfer is calculated as the ratio of the overall temperature difference to the sum of the thermal resistances

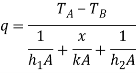
The overall heat transfer by combined conduction and convection is frequently expressed in terms of an overall heat-transfer coefficient U, defined by the relation

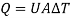
Where A is area of heat flow and

…(1.11)

Resistances connected in series

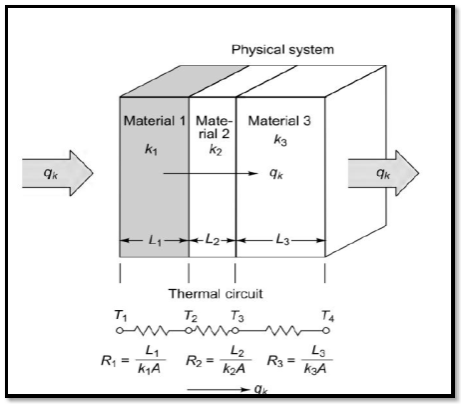
Fig 6 Conduction through three resistances in series

Consider 3 slabs connected in series with endpoints insulated to avoid loss of heat due to convection. This system is equivalent to electric system. Hence,

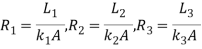
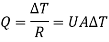
Resistances connected in parallel

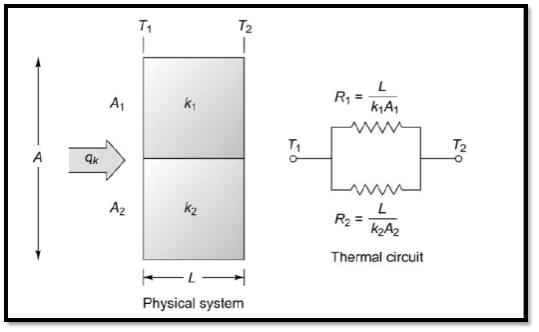
Fig 7 Conduction through three resistances in parallel

Consider 2 slabs connected in parallel with endpoints insulated to avoid loss of heat due to convection. This system is equivalent to electric system connected in parallel. Hence,

,

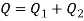
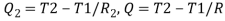
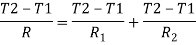
Therefore,

1.4 Critical thickness of insulation

• Heat insulating material is one which has low thermal conductivity.
• Thermal insulation is provided in thermal system to reduce heat losses.
• In case of plane wall, heat transfer rate always decreases with insulation. However, in case of cylinder and sphere, thickness of insulation should be higher than a particular value to reduce heat loss. This value is known as critical radius of insulation

Critical radius of insulation in cylinders

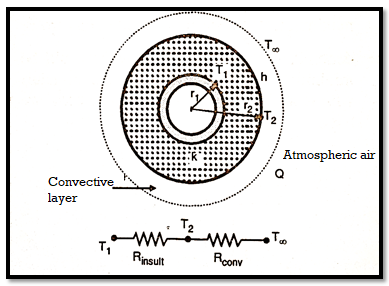
Fig 8 Critical thickness of cylinder

Consider heat flow from metal tube of outside radius r1.It is insulated by layer of insulation with outer radius r2. Let temperature of outside surface of metal tube is T1, conductivity of insulation be k(W/m K). Insulation is exposed to atmosphere at temperature T∞ with convective heat transfer coefficient h(W/m2K) and length L in meters. Now the heat transfer rate through metal tube is

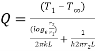…(1.14)

From above equation, it can be seen that with increase in insulation (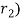 heat flow rate Q may decrease or increase with increase in since conductive resistance increase logarithmically but conductive resistance 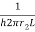decrease linearly.

To find value of for which Q is maximum should be equated to zero. Hence differentiating 1.14 with respect to 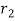and equating equal to zero.

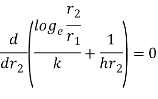
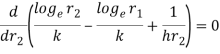
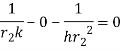
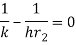
…(1.15)

Where 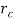is critical radius of insulation.

Therefore, at 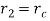heat loss will be maximum.

At , heat transfer will increase until increases and becomes equal to .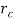

If , heat transfer will go on decreasing and insulation effect will be achieved.

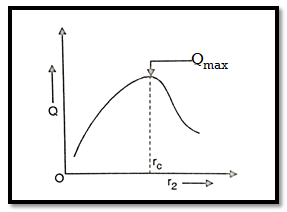
Fig 9 Variation of heat transfer with insulation radius

Critical radius of insulation of Sphere

Consider a hollow sphere with outer radius r1 at temperature T1 which is covered with insulation of outer radius r2. Insulation is exposed to atmosphere at temperature T∞ with convective heat transfer coefficient h(W/m2K). Let temperature of outside surface of metal sphere is T1, conductivity of insulation be k(W/m K).

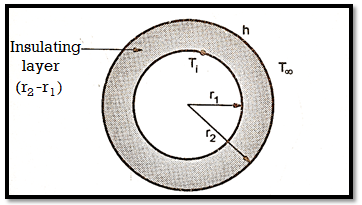
Fig 10 Critical thickness of sphere

From 1.13,

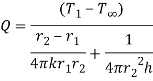
For maximum heat transfer,

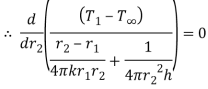
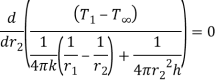
Differentiating denominator for it’s minimum value

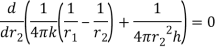
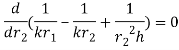
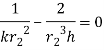
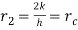(1.16)

1.16 is equation for critical radius of insulation for sphere.

Variation in heat transfer with change in radius in insulation is same as of cylinder.

1.5 Heat Transfer through pin fins

Convection heat transfer between a surface (at T1) and the fluid surrounding it (at ) is given by

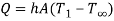
Where h is the heat transfer coefficient and A is the surface area of heat transfer.

Consider a plane wall. If T1 is fixed, the heat transfer rate may be increased by increasing the surface area across which convection occurs. This may be done by using fins that extend from the wall into the surrounding fluid.

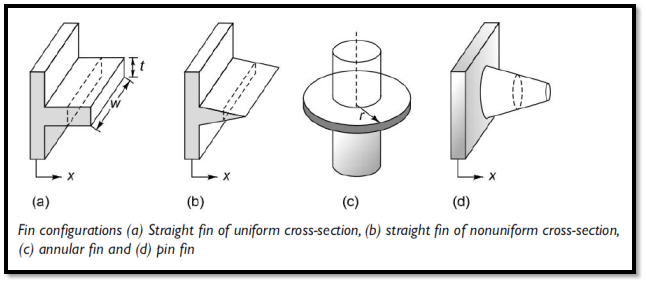
Fig 11 Types of Fins

Analysis of fin of uniform cross-section area(pin-fin)

Consider a thin rectangular fin of uniform cross-section. The fin is attached to base surface at temperature To. Let Ac be cross-sectional area and P be perimeter of fin, is atmospheric temperature and L is length of fin. Following assumptions are made in this analysis:

• Heat conduction is along x-axis under steady-state.
• Convective heat transfer coefficient over the fins is uniform.
• There is no heat generation in fin.
• Material of fin is isotropic, hence k is constant.

Heat is conducted from base surface to fin by conduction and from fin to atmosphere by convection simultaneously.

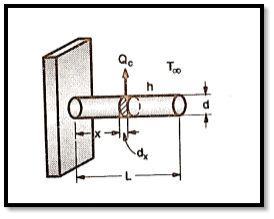
Fig 12 Pin fin

Consider an element section of thickness dx at distance x from base. Let T be be temperature at section.

Heat entering in elementary section,

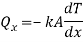
Heat conducted out from element

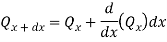
Heat convected out of element through thickness dx

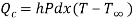
By energy balance equation,

Heat conducted into element = Heat conducted out + Heat convected out

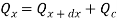
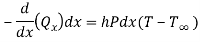
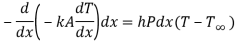
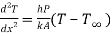…(1.17)

Let 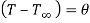and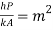

By differentiating

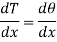
And

Hence 1.17 becomes

Above equation is second order differential equation. General solution for this equation is

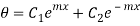…(1.18)

And slope is

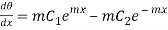…(1.19)

In order to find solution of above differential equations we need values of 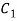and . We can obtain solution for following three cases:

1. Infinitely long fin

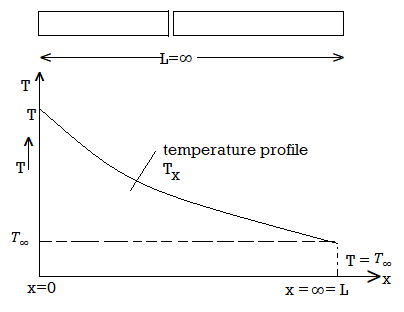
Fig 13 Infinitely long fin

Consider a infinitely long fin as in figure such that temperature at the end of fin approaches surrounding temperature  .

At hence .

At

Applying boundary condition 1 to 1.18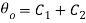…(1.20)

Applying boundary condition 2 to 1.18

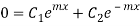
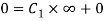
Hence 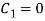. Putting this value in 1.20

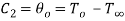
Substituting values of 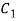and 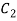in 1.18

…(1.21)

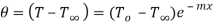
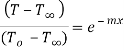
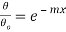…(1.22)

This represents temperature distribution on infinitely long fin.

Heat transfer at base is equal to heat transfer through fin. Hence, at base(x=0)

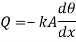
From 1.21

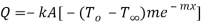
As x=0

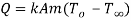
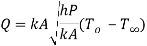
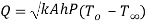…(1.23)

2.     Adequately long fin with insulated fin

Boundary conditions are

At hence .

At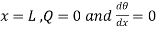

Applying first boundary condition on 1.18

…(1.24)

Fig 14 Insulated fin

Applying second boundary condition on 1.19

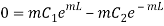
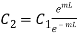…(1.25)

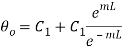
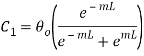
From 1.25

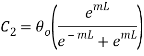
Substituting C1 and C2 in 1.18

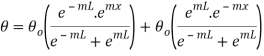
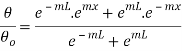
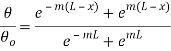
But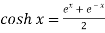

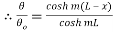…(1.26)

This represents temperature distribution on insulated long fin.

Heat transfer at base is equal to heat transfer through fin. Hence, at base(x=0)

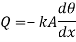
From 1.26

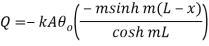
Therefore at x=0

…(1.27)

3.     Short Fin

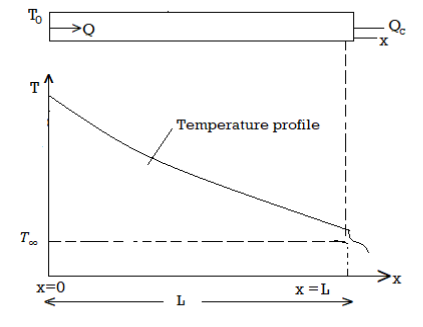
Fig. 15 Short fin

Boundary conditions are

At hence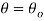

At

is heat convected away to surrounding.

From second boundary condition at x=L,

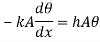
From 1.18 and 1.19

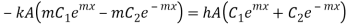
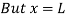
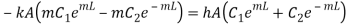
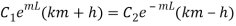…(1.28)

Putting boundary condition 1 in 1.18

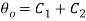
Solving this equation and 1.28

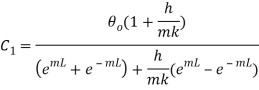
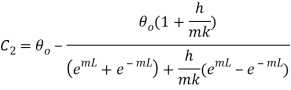
Putting these values in 1.18

At x=L and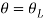

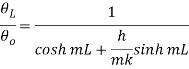
This represents temperature distribution on short fin.

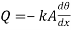at x=0

Substituting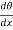

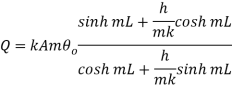
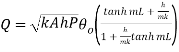…(1.29)

Effectiveness of fin

• Fins are used to increase heat transfer but fin itself blocks the base area on surface and acts as resistance to heat conduction.
• It not sure whether fin is helping in heat transfer or not hence the term effectiveness is used.
• Effectiveness is ration of actual heat transfer rate by fin to heat transfer by the area blocked by fin.

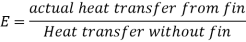
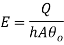
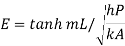
For infinitely long fin tanhmL=1

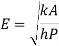
Fins are used only if

1.6 Lumped system approximation and Biot Number

• After the heat transfer process is initiated some time must elapse before steady-state is reached. During this period the temperature changes until body attains steady-state. This period during which temperature changes with time is called transient state or unsteady state.
• Conduction of heat in this state is transient heat conduction.

Lumped system approximation

• Even though no material in nature has an infinite thermal conductivity, many transient heat flow problems can be readily solved by assuming that the internal conductive resistance of the system is very small and the temperature within the system at any instant is uniform. This method is called lumped system approximation.
• This is justified when the external thermal resistance between the surface of the system and the surrounding fluid is so large compared to the internal thermal resistance that it controls the rate of heat transfer.
• In order know whether not to use lumped mass approximation, a dimensionless number is used known as Biot number (Bi).
• Biot number is measure of the relative importance of the thermal resistance within a solid body, which is the ratio of internal to external thermal resistance.
• 

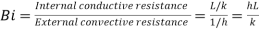
Where h is the average heat transfer coefficient, L is a characteristic dimension obtained by dividing the volume of the body by its surface area and k is the thermal conductivity of the solid body. For Bi < 0.1, i.e. when the internal resistance is less than 10% of the external resistance, the internal resistance can be ignored and lumped system approximation can be used.

Heisler charts

• Solution to unsteady state problems is worked out by lumped method if internal resistance of solid can be neglected. It can be done only if Bi<0.1.
• In case Bi>0.1, some another method needs to be applied. Heisler worked out analytical solutions of such problems in form of charts for standard shapes like cylinder, slabs and spheres.
• If 0.1<Bi<100, Heisler charts give very good accuracy.

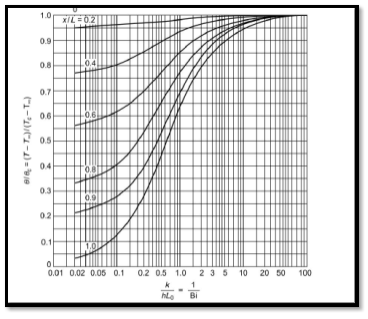
Fig 16 Temperature as a function of centre temperature in an infinite plate of thickness 2L

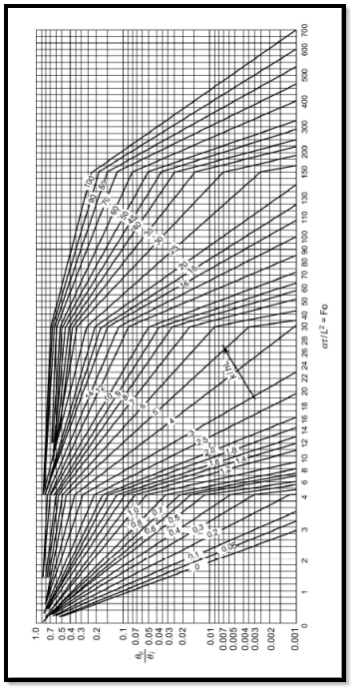
Fig 17 Axis temperature for infinite plate with thickness 2L

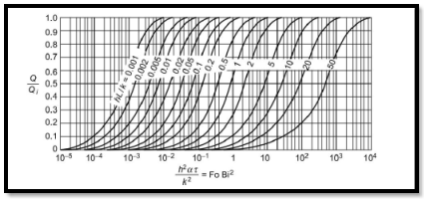
Fig 18 Heat loss of an infinite plate of thickness 2L with time

These are Heisler charts for cylinder where,

Fo = Fourier number

Bi=Biot number

=Thermal diffusivity

h= convective heat transfer coefficient

Q=Heat transfer rate

Qi=Heat transfer rate at t=0

t=time

1.7 Two- dimensional conduction solutions for steady heat transfer-approximate solution to unsteady conduction

When the boundaries of a system are irregular or the temperature along a boundary is non-uniform, a one-dimensional treatment may not be satisfactory. Temperature here may be a function of two or even three coordinates.

The components of the heat flow per unit area (heat flux) q in the x and y-direction are obtained from Fourier’s law:

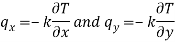
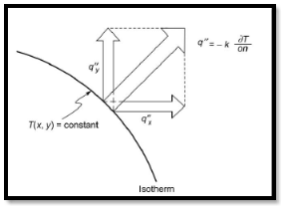
Fig 19 Heat flow in two dimensions

The heat flux q at a given point (x, y) is the resultant of the components qx and qy at that point and is directed perpendicular to the isotherm (Fig. 20). If the temperature distribution in a system is known, the rate of heat flow can easily be calculated.

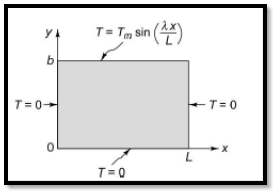
Fig. 20 Rectangular adiabatic plate with sinusoidal temperature distribution on one edge

Let us consider a simple case of a thin rectangular plate (Fig. 20), free of heat sources and insulated at the top and bottom surfaces. Since ∂T/∂z =0, the temperature is a function of x and y only. If k is uniform, the temperature distribution must satisfy Eq. (2.134), a linear and homogeneous partial differential equation that can be integrated by assuming a product solution for T(x, y) of the form

…(1.30)

Where X = X(x), a function of x only, and Y = Y(y), a function of y alone. Differentiating above equation twice, first with respect to x and then with respect to y.

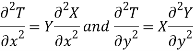
Substituting this values in 1.30

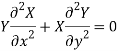
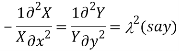
The variables are now separated. The LHS is a function of x only, while the RHS is a function of y alone. Since neither side can change as x and y vary, both must be equal to a constant, say  . We have, therefore, two ordinary differential equations

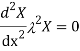
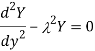
Solutions to above differential equations are

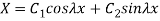
And

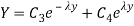
Respectively. Substituting these values in 1.30

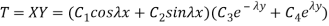…(1.31)

Where C1, C2, C3 and C4 are constants to be evaluated from the boundary conditions. As shown in Fig, the boundary conditions to be satisfied are

at x=0

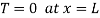
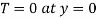
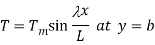
Substituting third condition in 1.31

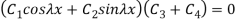…(1.32)

Substituting first condition in 1.31

…(1.33)

Substituting second condition in 1.31

…(1.34)

Solving 1.33, , solving 1.32, we get

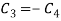
Using these results in 1.34

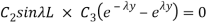
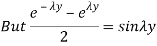
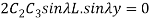
To satisfy this condition, sin 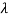L = 0 or, =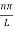, where n = 1, 2, 3, …. There exists, therefore, a different solution for each integer n, and each solution has a separate integration constant Cn. Summing these solutions

…(1.35)

The last boundary condition needs that at y=b

Putting this value in 1.35

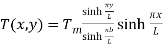  …(1.36)

1.8 Two-Dimensional conduction solutions for both unsteady heat transfer-approximate solution to unsteady conduction

For regular shaped bodies, analytical methods in the form of Heisler’s charts can be used to determine the time-temperature history.

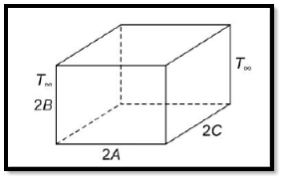
Fig. 21 A parallelepiped being heated or cooled

Let us consider a rectangular parallelepiped with dimensions 2A, 2B and 2C, which is initially at a uniform temperature Ti and then subjected to a constant surrounding temperature (Fig. 21).

Fourier’s heat conduction equation in absence of any heat source is given by

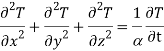
The solution may be broken up into factors so that

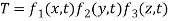
Substituting above mentioned factors in fourier equation

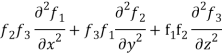
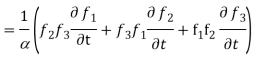
By rearranging

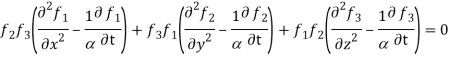
…(1.37)

Equation 1.37 will be satisfied only if

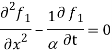
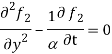
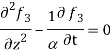
But these are simply three one-dimensional forms of Fourier’s equation, so that the solution to the three-dimensional problem may be expressed as the product of three solutions of one-dimensional problems.

From Heisler’s charts for infinite plates, (/)A, (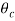/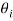))B and (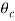/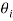))C are found out. The product of these three will give the centre temperature of the parallelepiped

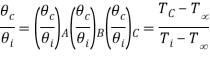
From which 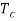at any time t can be estimated.

Similarly, for any other point inside the parallelepiped the time-temperature history can be found out by using the position-correction charts.

1.9 Heat transfer by the use of Heissler charts

• Heisler charts are a graphical analysis tool for the evaluation of heat transfer in thermal engineering. They are a set of two charts per included geometry introduced in 1947 by M. P.
• Heisler which were supplemented by a third chart per geometry in 1961 by H. Gröber. Heisler charts permit evaluation of the central temperature for transient heat conduction through an infinitely long plane wall of thickness 2L, an infinitely long cylinder of radius ro, and a sphere of radius ro.
• These first Heisler-Gröber charts were based upon the first term of the exact Fourier Series solution for an infinite plane wall:

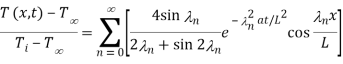
Where Ti is the initial temperature of the slab, T∞ is the constant temperature imposed at the boundary, x is the location in the plane wall, λn is π(n+1/2), and α is thermal diffusivity. The position x=0 represents the center of the slab.

Limitations

Although Heisler-Gröber charts are a faster and simpler alternative to the exact solutions of these problems, there are some limitations. First, the body must be at uniform temperature initially. Additionally, the temperature of the surroundings and the convective heat transfer coefficient must remain constant and uniform. Also, there must be no heat generation from the body itself.

Importance of numerical methods

• The overall goal of the field of numerical analysis is the design and analysis of techniques to give approximate but accurate solutions to hard problems, the variety of which is suggested by the following:
  • Advanced numerical methods are essential in making numerical weather prediction feasible.
  • Computing the trajectory of a spacecraft requires the accurate numerical solution of a system of ordinary differential equations.
  • Car companies can improve the crash safety of their vehicles by using computer simulations of car crashes. Such simulations essentially consist of solving partial differential equations numerically.

The Biot and Fourier Numbers

The temperature profiles and heat flows may all be expressed in terms of two dimensionless parameters called the Biot and Fourier numbers:

Biot number (Bi)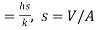

Fourier number

All value of the Bi no. Means that internal conduction resistance is negligible in comparison with surface-convection resistance.

All value of Fo no. Means that along period of time is required to heat or cool the body.

Example: A steel plate (k=41 W/m.K, 5cm thick) is initially at a temperature of 425°C. Its suddenly exposed on path side to a convention environment with h = 285 W/m2 .K and T∞ = 65°C. Determine the centre line temperature and the temperature inside the body 1.25cm from the surface after 24min. α=1.172 ×10-5 m2 /s

Solution

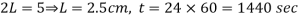
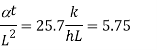
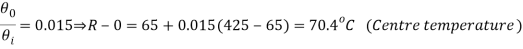
At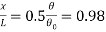

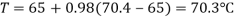
Example: Along 6.5cm diameter solid cylinder steel (k=16.3 W/m.K, ρ=7817 kg/m3 , cp=460 J/kg.K). It's initially a uniform temperature Ti = 150°C. It's suddenly exposed to a convective environment at T∞ = 50°C and h = 285 W/m2.K. Calculate the temperature at 1) the axis of cylinder, and 2) a 2.5cm radial distance after 5min of exposure to the cooling flow 3) determine the total energy transferred from the cylinder per meter length during the first five min. Of cooling.

Solution

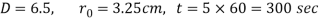
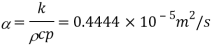
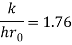
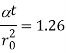
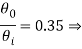
1)

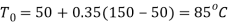
2) At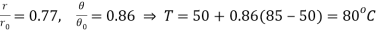

3)

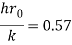
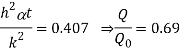
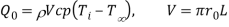
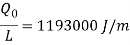
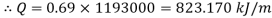
Example: A fused quartz sphere has a thermal diffusivity of 9.5 ×10-7 m 2 /s. a diameter of 25mm and k=1.52 W/m.K. The sphere is initially at a uniform temperature Ti = 25°C. It's suddenly subjected to a convection environment at T∞ = 200°C and h = 110 W/m2.K. Calculate the temperature at the centre and at the radius of 6.4mm after 4min.

Solution

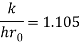
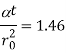
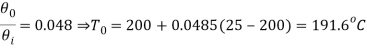
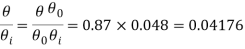
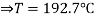
Important Formulae

Conductive heat transfer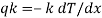
Convective heat transfer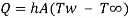
Radiation heat transfer
Heat balance equation
Conductive thermal resistance
Convective thermal resistance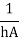
Total thermal resistance of bodies connected in series
Total thermal resistance of bodies connected in parallel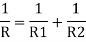
Heat transfer through cylinder
Heat transfer through sphere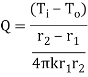
Critical insulation radius of cylinder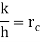
Critical insulation radius of sphere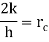
Heat transfer through infinitely long fin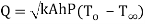
Heat transfer through fin with insulated tip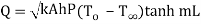
Heat transfer through short fin	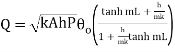Or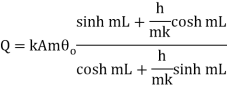
Effectiveness of fin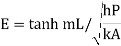
Biot number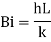

References:

1. A. Bejan, Heat Transfer John Wiley, 1993

2. J.P. Holman, Heat Transfer, Eighth Edition, McGraw Hill, 1997.

3. F.P. Incropera, and D.P. Dewitt, Fundamentals of Heat and Mass Transfer, John Wiley, Sixth Edition, 2007.

4. Massoud Kaviany, Principles of Heat Transfer, John Wiley, 2002