Unit -6
Step–Less-Regulation (Theoretical Treatment only) & Gyroscope
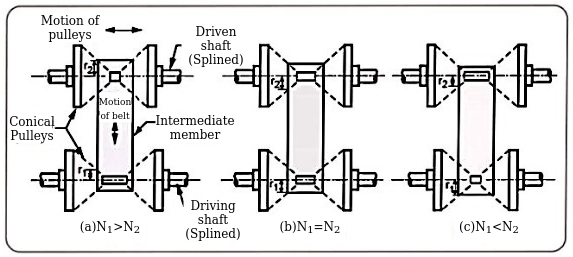
Continuous variable transmission
Principle of Pulley based CVT system
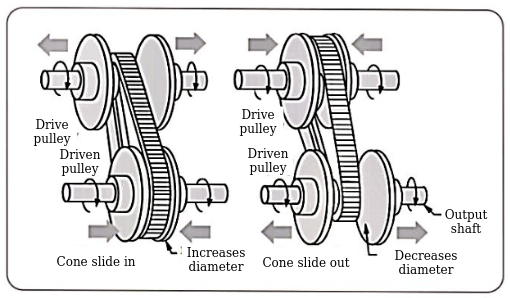
Advantages of CVT system:
Limitations of CVT system:
Applications of CVT system:
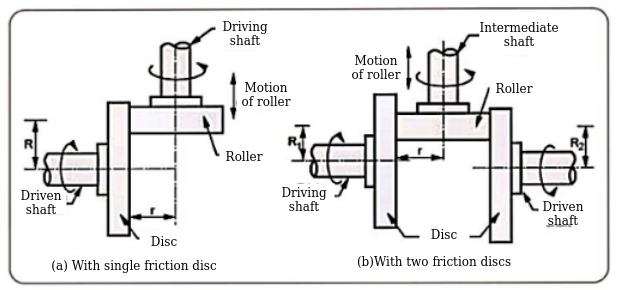
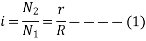
Where i=instantaneous transmission ratio
 speed of driving shaft
speed of driving shaft
 speed of driven shaft
speed of driven shaft
r= contact radius of roller
R=contact radius of disc
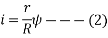
Where 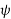 =Slip factor=0.97 to 0.98
=Slip factor=0.97 to 0.98
Specifications
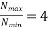
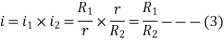
Where. r=contact radius of roller
 contact radii of discs
contact radii of discs
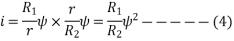
Specifications
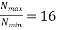
Advantages of faceplate variators
Limitation of faceplate variators
Application of faceplate variators
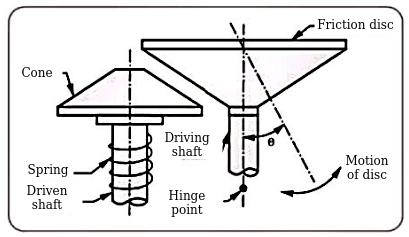
Cone variator with non-parallel shafts
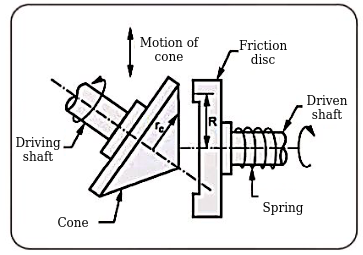

Where 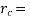 radius of friction cone at the point of contact
radius of friction cone at the point of contact
R= radius of friction disc
Specifications
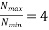
Cone variator with parallel shafts

Specifications
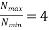
Cone variator with planetary mechanism

Specifications
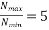
Cone variator with swivelling disc
Specifications
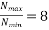
Advantages of conical variator
Limitations of conical variator
Applications of conical variator
Spheroidal variator with swivelling discs
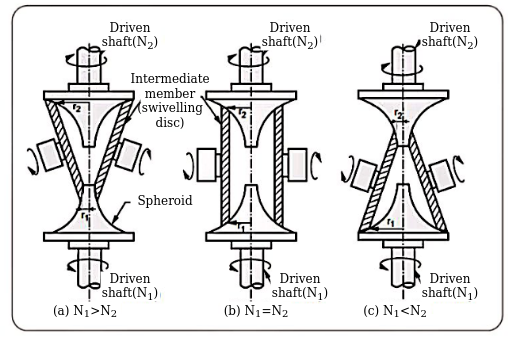
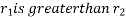
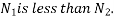
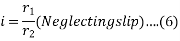
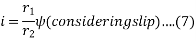
Specifications
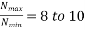
Cone variators with spheres supported on shafts
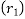
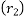
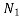
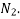


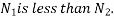
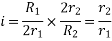
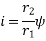
Specifications
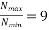
Advantages of spheroidal and cone variators
Limitations of spheroidal and cone variators
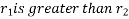
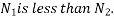
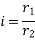
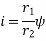
V belt:-
Specifications
Nylon belt
Steel ring
Specifications

Chain
Specifications
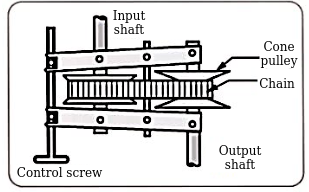
Applications:
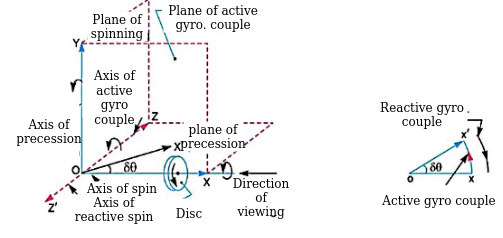
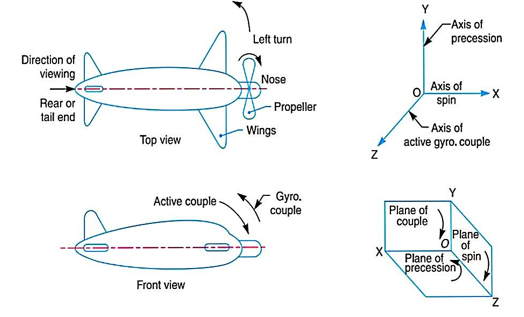
Precessional angular motion
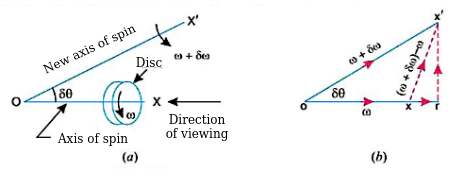
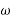
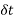
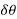
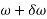
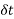
Component of angular acceleration in the direction of ox.

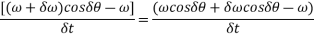
Since 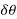 is very small, therefore substituting
is very small, therefore substituting 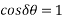 we have
we have
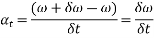
In the limit, when 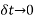
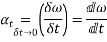
Component of angular acceleration in the direction perpendicular to ox,
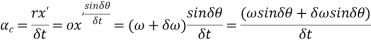
Since 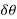 is very small, therefore substituting,
is very small, therefore substituting, 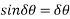 , we have
, we have
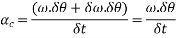
In the limit when 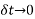
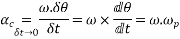
Total angular acceleration of the disc
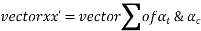
= 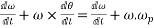

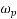
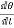
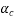
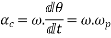
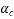
Gyroscopic couple

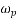
Let. I=mass moment of inertia of the disc about OX, and
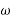 = Angular velocity of the disc.
= Angular velocity of the disc.
Angular momentum of the disc 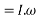
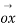
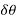
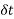
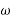
Change in angular momentum
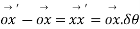
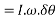
And rate of change of angular momentum
=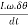
Since the rate of change of angular momentum will result by the application of a couple to the disc, therefore the couple applied to the disc causing precession.
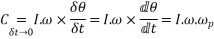
Where 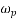 = angular velocity of precision of the axis of spin or the speed of rotation of the axis of the spin about the axis of precision OY.
= angular velocity of precision of the axis of spin or the speed of rotation of the axis of the spin about the axis of precision OY.
In SI units, the units of C in N-m when I is in 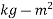
It may be noted that
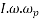
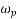
Let.  = Angular velocity of the engine in rad/s
= Angular velocity of the engine in rad/s
m= mass of the engine and the propeller in kg.
k= its radius of gyration in metres.
I=mass momentum of inertia of the engine and the propeller in kg-m^2
= 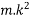
v= linear velocity of the aeroplane in m/s
R= radius of curvature in metres, and
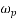 = Angular velocity of precision=v/R rad/s
= Angular velocity of precision=v/R rad/s
Gyroscopic couple acting on the aeroplane,
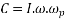
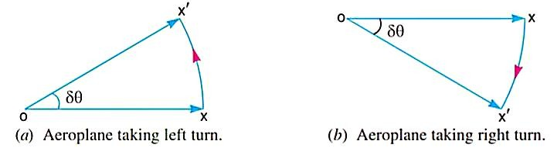
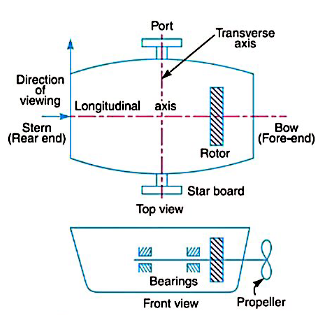
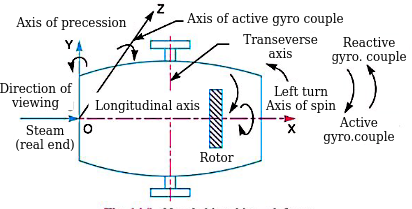
Terms used in a naval ship
Effect of gyroscopic couple on a naval ship during steering
Effect of gyroscopic couple on a naval ship during pitching
 = Amplitude of Swing maximum angle turned from the mean position in radians, and
= Amplitude of Swing maximum angle turned from the mean position in radians, and
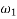 = Angular velocity of S.H.M.
= Angular velocity of S.H.M.
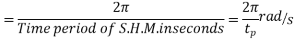
Angular velocity of precision
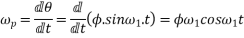
The angular velocity of precession will be maximum if  =1
=1
Maximum angular velocity of precession,

Let I=Moment of inertia of the rotor in 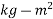 and
and
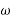 = Angular velocity of the rotor in rad/sec
= Angular velocity of the rotor in rad/sec
Maximum gyroscopic couple,
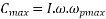
Effect of gyroscopic couple on a naval ship during rolling
Let m=mass of the vehicle in kg.
W= weight of the vehicle in Newton = m.g
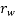 = radius of the wheels in metres.
= radius of the wheels in metres.
R= radius of curvature in metres
h= distance of centre of gravity, vertically above the road surface in metres,
x= width of track in metres,
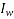 = mass moment of inertia of one of the wheels in kg-m^3
= mass moment of inertia of one of the wheels in kg-m^3
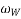 = Angular velocity of the wheels for velocity of spin in rad/s.
= Angular velocity of the wheels for velocity of spin in rad/s.
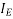 = mass moment of inertia of the rotating parts of the engine
= mass moment of inertia of the rotating parts of the engine
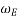 = Angular velocity of the rotating parts of the the engine in rad/s.
= Angular velocity of the rotating parts of the the engine in rad/s.
G= gear ratio=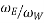
v= linear velocity of the vehicle in m/s =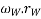
Therefore
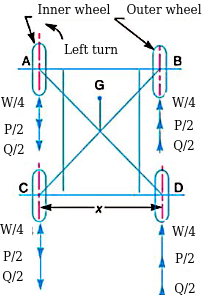
Road reaction over each wheel =W/4=m.g/4 Newton’s
Let us now consider the effect of the gyroscopic couple and centrifugal couple on the vehicle.
Since the vehicle takes a turn towards left due to the precession and other rotating parts, therefore a gyroscopic couple will act.
We know that velocity of precision,
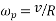
Gyroscopic couple due to four wheels
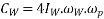
And gyroscopic couple due to rotating parts of the engine,
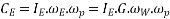
Net gyroscopic couple,
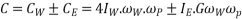
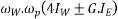
P × x = C. Or. P=C/x
Vertical reaction at each of the outer or inner wheels,
P/2 =C/2x
This gyroscopic couple is balanced by vertical reactions, which are vertically upwards on the outer wheels & vertically downwards on the inner wheels.
2. Effect of the centrifugal couple
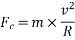
The couple tending to overturn the vehicle or overturning couple,
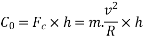
This overturning couple is balanced by vertical reactions, which are vertically upwards on the outer wheels & vertically downwards on the inner wheels.
Vertical reaction at each of the outer or inner wheels,
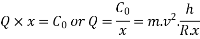
Vertical reaction at each of the outer or inner wheels,
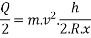
Total vertical reaction at each of the outer wheel,
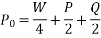
And total vertical reaction at each of the inner wheel,
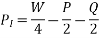
Stability of a two wheeler vehicle taking a turn
Consider a two wheeler taking a right turn as shown in figure
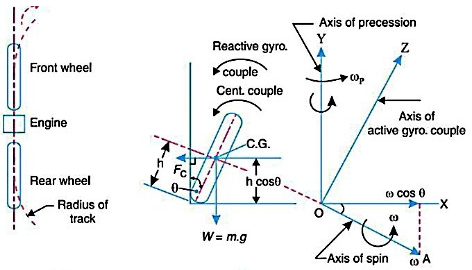
Let m=mass of the vehicle and its rider in kg.
W=weight of the vehicle and its rider in Newton’s =m.g
h= height of the centre of gravity of the vehicle and rider.
 =radius of the wheels,
=radius of the wheels,
R= radius of track or curvature.
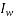 =mass moment of inertia of each wheel,
=mass moment of inertia of each wheel,
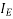 =mass moment of inertia of the rotating parts of the engine,
=mass moment of inertia of the rotating parts of the engine,
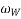 = Angular velocity of the wheels,
= Angular velocity of the wheels,
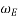 = Angular velocity of the engine,
= Angular velocity of the engine,
G= gear ratio = 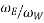
v= linear velocity of the vehicle= 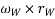
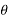 = Angle of heel. It is inclination of the vehicle to the vertical for equilibrium.
= Angle of heel. It is inclination of the vehicle to the vertical for equilibrium.
Let us now consider the effect of the gyroscopic couple and centrifugal couple on the vehicle, as discussed below.
We know that 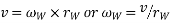
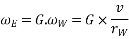
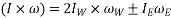
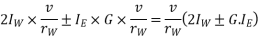
and velocity of precession 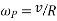
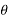
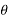
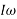
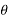
Gyroscopic couple,
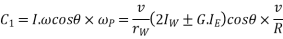
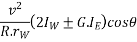
2. Effect of centrifugal couple
We know that centrifugal force,
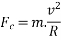
This force acts horizontally through the centre of gravity along the outward direction,
Centrifugal couple,
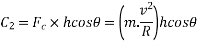
Since the centrifugal couple has a tendency to overturn the vehicle, therefore total overturning couple,
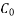 = Gyroscopic couple +centrifugal couple
= Gyroscopic couple +centrifugal couple

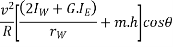
We know that balancing couple = m.g.h.sin
The balancing couple acts in clockwise direction when seen from the front of the vehicle.
Therefore for stability, the overturning couple must be equal to the balancing couple, i.e.
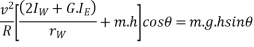
From this expression, the value of the angle of heel may be determined, so that the vehicle does not skid.
Numericals
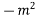
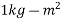
Solution Given m=250Kg , h=0.6m . v=90km/h = 25m/s, R=50m
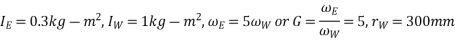
Let 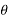 =Angle of inclination with respect to the vertical of a two wheeler
=Angle of inclination with respect to the vertical of a two wheeler
We know that gyroscopic couple,
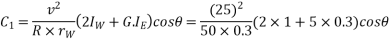
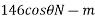
And centrifugal couple 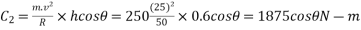
Total overturning couple,
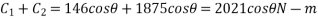
We know that balancing couple,
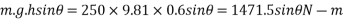
Since the overturning couple must be equal to the balancing couple for equilibrium condition therefore
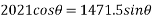

2. A ship propelled by a turbine rotor which has a mass of 5 tonnes and a speed of 2100 rpm. The rotor has a radius of gyration of 0.5 metre and rotates in clockwise direction when viewed from the stern. Find the gyroscopic effects in the following conditions:
Determine also the maximum angular acceleration during pitching. Explain how the direction of motion due to gyroscopic effect is determined in each case.
Solution Given m=5 t = 5000kg N=2100r.p.m or 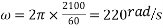 k=0.5m
k=0.5m
Given: v=30km/h =8.33m/s : R=60m
We know that angular velocity of precession
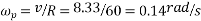
And mass moment of inertia of the rotor
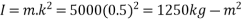
Gyroscopic couple,
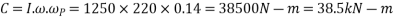
When the rotor in a clockwise direction when viewed from the stern and the ship steers to the left, the effect of reactive gyroscopic couple is to raise the bow and lower the stern.
2. When the ship pitches with the bow descending
Given: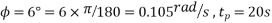
We know that angular velocity of simple harmonic motion
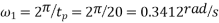
And maximum angular velocity of precession
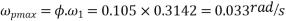
Maximum gyroscopic couple
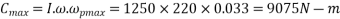
Since the ship is pitching with the bow descending therefore the effect of this maximum gyroscopic couple is to turn the ship towards port side.
3.When the ship rolls
Since the ship rolls at an angular velocity of 0.03rad/s therefore angular velocity of precession when the ship rolls,
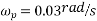
Gyroscopic couple
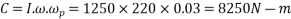
In case of rolling of a ship the axis of precession is always parallel to the axis of spin for all positions, therefore there is no effect of gyroscopic couple.
Maximum angular acceleration during pitching
We know that maximum angular acceleration during pitching.
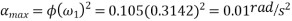
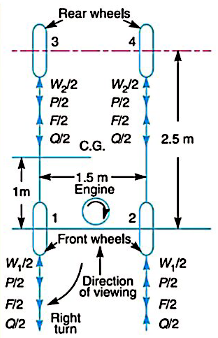
3. A four wheeled motor car of mass 2000 kg has a wheelbase 2.5 m, track with 1.5 m and height of centre of gravity 500 mm above the ground level and lies at 1 metre from the front Excel. Each wheel has an effective diameter of 0.8m and a moment of inertia of 0.8 kg-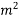 the drive shaft, engine flywheel and transmission are rotating at four times the speed of road wheel, in a clockwise direction when viewed from the front, and is equivalent to a mass of 75kg having a radius of gyration of 100mm. If the car is taking a right turn of 60m radius at 60km/h. Find the load on each wheel.
the drive shaft, engine flywheel and transmission are rotating at four times the speed of road wheel, in a clockwise direction when viewed from the front, and is equivalent to a mass of 75kg having a radius of gyration of 100mm. If the car is taking a right turn of 60m radius at 60km/h. Find the load on each wheel.
Solution Given m=2000kg: b=2.5m: x=1.5m: h=500mm = 0.5m: L=1m: R=60m: v=60km/h =16.67m/s
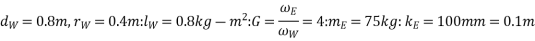
Since the centre of gravity of car lies at 1 m from the front axle and the weight of the car lies at the centre of gravity, therefore weight on the front wheels and rear wheels will be different.
Let 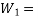 Weight of the front wheels, and
Weight of the front wheels, and
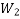 =Weight on the rear wheels,
=Weight on the rear wheels,
Taking moment about the front wheels,
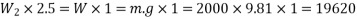
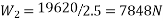
We know that weight of the car or on the four wheels,
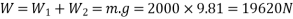

Weight on each of the front wheels
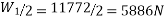
And weight on each of the rear wheels
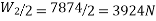
Since the weight of the car over the four wheels will act downwards, therefore the reaction between each wheel and the road surface of the same magnitude will act upwards as shown in Fig.
Let us now consider the effect of gyroscopic couple due to four wheels and rotating parts of the engine.
We know angular velocity of wheels
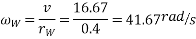
And angular velocity of precession
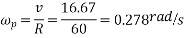
Gyroscopic couple due to four wheels

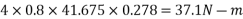
This gyroscopic couple tends to lift the inner wheels and to press the outer wheels. In other words, the reaction will be vertically downward on the inner wheels and vertically upward on the outer wheels as shown in fig.
Let P/2 newtons be the magnitude of this reaction at each of the inner or outer wheel.
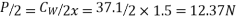
We know that mass moment of inertia of rotating parts of the engine,
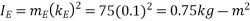
Gyroscopic couple due to rotating parts of engine
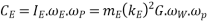

This gyroscopic couple tends to lift the front wheels and to press the outer wheels. In other words, the reaction will be vertically downwards on the front wheels and vertically upwards on the rear wheels as shown in Fig.
Let F/2 newtons be the magnitude of this reaction on each of the front and rear wheels

Now let us consider the effect of centrifugal couple acting on the car. We know that centrifugal force,
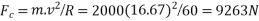
Centrifugal couple tending to overturn the car or over turning couple,
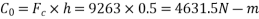
This overturning couple tends to reduce the pressure on the inner wheels and to increase on the outer wheels. In other words, the reactions are vertically downward on the inner wheels and vertically upwards on the outer wheels. Let Q/2 be the magnitude of this reaction on each of the inner and outer wheels.
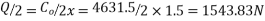
From figure we see that
Load on the front wheel 1
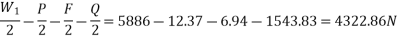
Load on the front wheel 2
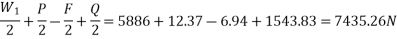
Load on the front wheel 3
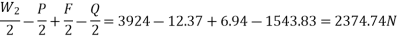
Load on the rear wheel 4
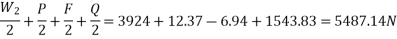
4. A rear engine automobile is travelling along a track of 100 meters mean radius. Each of the four road wheels has a moment of inertia of 2.5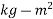 and an effective diameter of 0.6m. The rotating parts of the engine have a moment of inertia of
and an effective diameter of 0.6m. The rotating parts of the engine have a moment of inertia of 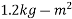 . The engine axis is parallel to the rear axle and the crankshaft rotates in the same sense as the road wheels. The ratio of engine speed to back axle speed is 3:1 .The automobile has a mass of 1600kg and has its centre of gravity 0.5m above road level. The width of the track of the vehicle is 1.5m
. The engine axis is parallel to the rear axle and the crankshaft rotates in the same sense as the road wheels. The ratio of engine speed to back axle speed is 3:1 .The automobile has a mass of 1600kg and has its centre of gravity 0.5m above road level. The width of the track of the vehicle is 1.5m
Determine the limiting speed of the vehicle around the curve for all four wheels to maintain contact with the road surface. Assume that the road surface is not cambered and centre of gravity of automobile lies centrally with respect to the four wheels.
Solution Given R=100m: m=1600kg: h=0.5m: x=1.5m

The weight of the vehicle will be equally distributed over the four wheels which will act downwards. The reaction between the wheel and the road surface of the same magnitude will act upwards.
Road reaction over each wheel
=W/4=m.g/4=1600*9.81/4=3924N
Let v=Limiting speed of the vehicle in m/s
We know that angular velocity of the wheels
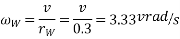
And angular velocity of precession
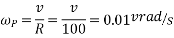
Gyroscopic couple due to 4 wheels

And gyroscopic couple due to rotating parts of engine
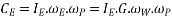
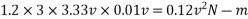
Total gyroscopic couple
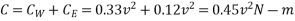
Due to this gyroscopic couple the vertical reaction on the rails will be produced . The reaction will be vertically upwards on the outer wheels and vertically downwards on the inner wheels. Let the magnitude of this reaction at each of the outer or inner wheel be P/2newtons.
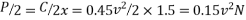
We know that centrifugal force
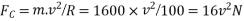
Overturning couple acting in the outward direction
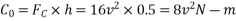
This overturning couple is balanced by vertical reactions which are vertically upwards on the outer wheels and vertically downwards on the inner wheels Let the magnitude of this reaction at each of the outer or inner wheels be Q/2 newtons.
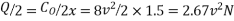
We know that total vertical reaction at each of the outer wheels
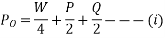
And total vertical reaction at each of the inner wheels
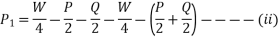
From equation (i) we see that there will always be contact between the outer wheels and the road surface because W/4 P/2 and Q/2 are vertically upwards, In order to have contact between the inner wheels and road surface the reactions should also be vertically upwards which is only possible if
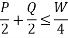
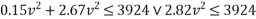
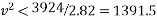
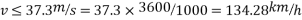
Reference: