Unit 5
Applications of Multivariable Differential Calculus
If u and v are functions of the two independent variables x and y , then the determinant,
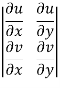
Is known as the jacobian of u and v with respect to x and y, and it can be written as,
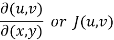
Suppose there are three functions u , v and w of three independent variables x , y and z then,
The Jacobian can be defined as,
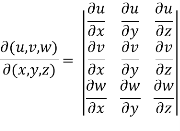
Important properties of the Jacobians-
Property-1-
If u and v are the functions of x and y , then
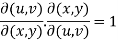
Proof- Suppose u = u(x,y) and v = v(x,y) , so that u and v are the functions of x and y,
Now,
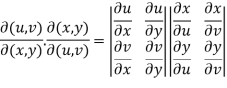
Interchange the rows and columns of the second determinant, we get
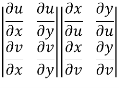
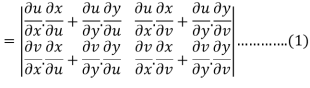
Differentiate u = u(x,y) and v= v(x,y) partially w.r.t. u and v, we get
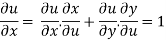
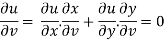
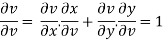

Putting these values in eq.(1) , we get
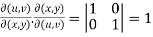 hence proved.
hence proved.
Property-2:
Suppose u and v are the functions of r and s, where r,s are the fuctions of x , y, then,

Proof: 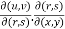 =
= 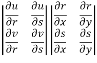
Interchange the rows and columns in second determinant
We get,
= 
= 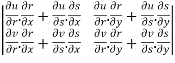
= 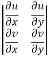 =
= 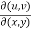
Similarly we can prove for three variables.
Property-3
If u,v,w are the functions of three independent variables x,y,z are not independent , then,
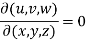
Proof: here u,v,w are independent , then f(u,v,w) = 0 ……………….(1)
Differentiate (1), w.r.t. x, y and z , we get
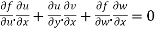 …………(2)
…………(2)
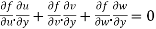 ………………(3)
………………(3)
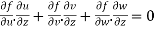 ………………..(4)
………………..(4)
Eliminate 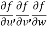 from 2,3,4 , we get
from 2,3,4 , we get
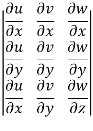
Interchanging rows and columns , we get
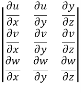 = 0
= 0
So that,
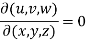
Example-1: If x = r sin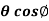 , y = r sin
, y = r sin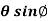 , z = r cos
, z = r cos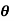 , then show that
, then show that
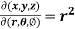 sin
sin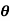 also find
also find 
Sol. We know that,
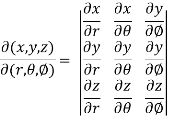
= 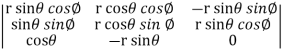
= 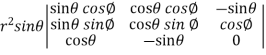
= 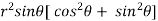 ( on solving the determinant)
( on solving the determinant)
= 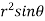
Now using first propert of Jacobians, we get
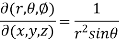
Example-2: If u = x + y + z ,uv = y + z , uvw = z , find 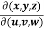
Sol. Here we have,
x = u – uv = u(1-v)
y = uv – uvw = uv( 1- w)
And z = uvw
So that,
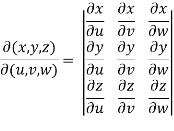
=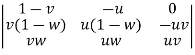
Apply 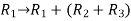
=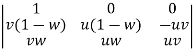
Now we get,
= u²v(1-w) + u²vw
= u²v
Example-3: If u = xyz , v = x² + y² + z² and w = x + y + z, then find J = 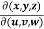
Sol. Here u ,v and w are explicitly given , so that first we calculate
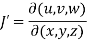
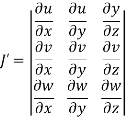
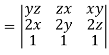
= yz(2y-2z) – zx(2x – 2z) + xy (2x – 2y)
= 2[yz(y-z)-zx(x-z)+xy(x-y)]
= 2[x²y - x²z - xy² + xz² + y²z - yz²]
= 2[x²(y-z) - x(y² - z²) + yz (y – z)]
= 2(y – z)(z – x)(y – x)
= -2(x – y)(y – z)(z – x)
By the property,
JJ’ = 1
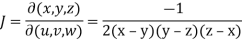
Jacobian of Implicit functions-
If the variables u,v, x, and y are connected by the following equations-
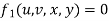 ………………… (1)
………………… (1)
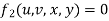 ………………….. (2)
………………….. (2)
u,v are the implicit functions of x and y.
Now differentiate equation (1) and (2) with respect to x and y-
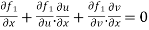 ………… (3)
………… (3)
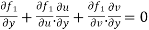 ……………… (4)
……………… (4)
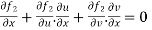 ………………. (5)
………………. (5)
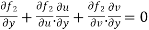 ………………. (6)
………………. (6)
Now,
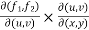 =
= 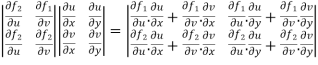
Using equation (3), (4), (5), (6), we get-
= 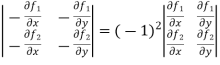
So that-
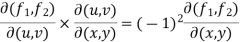
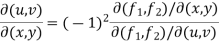
We can find this for three variables as well.
Example-1: If u = 2axy, v = 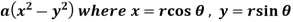 then prove that-
then prove that-
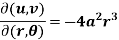
Sol. Here we have,
u = 2axy, v = 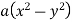
Then
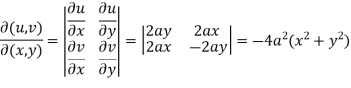
Here - 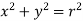
So that
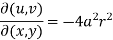
Now,
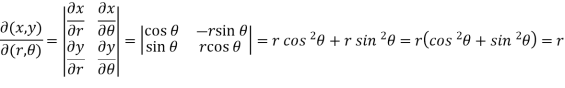
Hence-
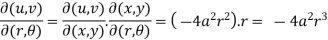
Hence proved.
Example-2: If 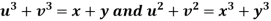 , then prove that-
, then prove that-
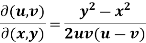
Sol. Suppose 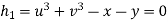
And 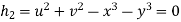
Now
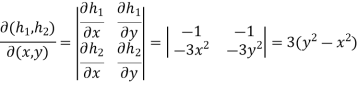
And
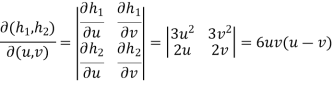
So that,
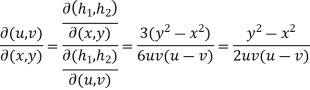
Hence proved.
Example-3: If x + y + z = u, y + z = uv , z = uvw, then prove that-
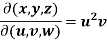
Sol. Suppose
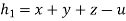
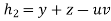
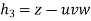
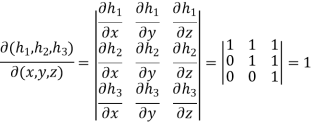
And
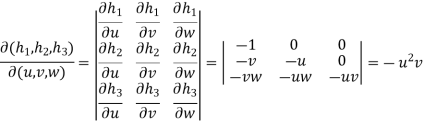
Hence,
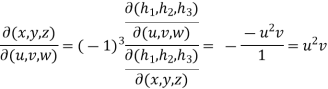
Hence proved.
Jacobians for functional dependence functions-
Note- two functions u and v said to be functionally dependent if their jacobian is equals to zero. That means J (u , v) = 0
Suppose u and v are functionally dependent functions, then
f( u, v) = 0
Differentiate this equation with respect to x and y-
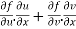 = 0
= 0
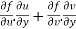 = 0
= 0
There will be a non-trivial solution for 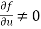 ,
, 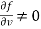 to this system exists.
to this system exists.
So that-
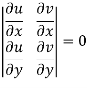
On transposing, we get-
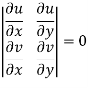
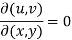
Example-1: Show that 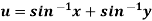 and
and 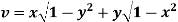 are functionally dependent.
are functionally dependent.
Sol. Here we have-
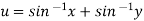 and
and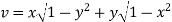
Now we will find out the Jacobian to check the functional dependence.
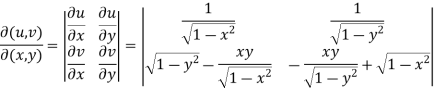
= 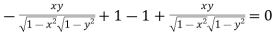
Here Jacobian is zero, so we can conclude that these functions are functionally dependent.
Example-2: Prove that u, v, w are functionally dependent, where-
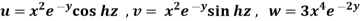
Sol. Here we have
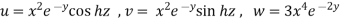
Now we will find out the Jacobian of the given functions-
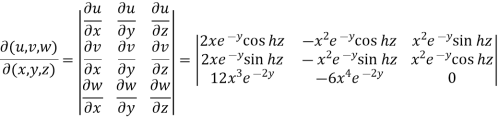
= 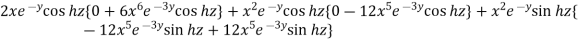
= 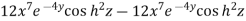
= 0
Therefore, u, v, w are functionally dependent.
Key takeaways-
- If u and v are functions of the two independent variables x and y , then the determinant,

Is known as the jacobian of u and v with respect to x and y, and it can be written as,
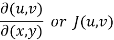
2. If u and v are the functions of x and y , then
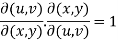
3. Suppose u and v are the functions of r and s, where r,s are the fuctions of x , y, then,
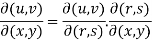
4. If u,v,w are the functions of three independent variables x,y,z are not independent , then,
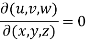
Let f(x, y) be a continuous function of x & y. Let 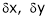 be the increments x & y resp. Then the value of f(x, y) will be
be the increments x & y resp. Then the value of f(x, y) will be
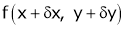
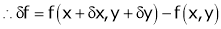
Expanding above expression by taylor’s theorem & since 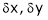 are very small Hence their product and higher powers are negligible and hence neglected we get,
are very small Hence their product and higher powers are negligible and hence neglected we get,
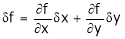
i.e. 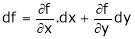
Similarly,
If f be a function of variables x, y, z, t, ………. Then we have
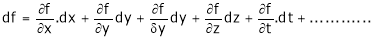
Note that
- Dx, dy, dz, ……. May be taken as actual errors or interments in x, y, z ……… resp. While df is the approximate error in f.
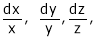 ……….. Denotes relative errors in x, y, z,………….. Resp.
……….. Denotes relative errors in x, y, z,………….. Resp.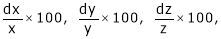 ………….
………….- Denotes percentage errors in, x, y, z, …………. Resp.
Ex.
- Find the percentage error in the area of an ellipse where error of ly is made in measuring it’s major and minor axes.
Solution:
Let A be the area of an ellipse and ‘a’ & ‘b’ are its semi major and minor axes
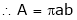
Taking log on both sides.
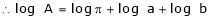
Differentiating we get,
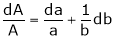
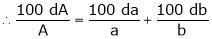


 Percentage error ion the area of an ellipse = 2%
Percentage error ion the area of an ellipse = 2%
2. The density 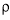 of a body is calculated from its’s weight W in air and win water if error
of a body is calculated from its’s weight W in air and win water if error  are made in
are made in 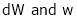 , find the error in
, find the error in 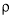 .
.
Solution:
We know that,

Taking log on both sides we get, 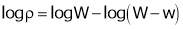
Diff. We get,

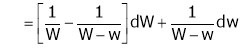
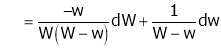
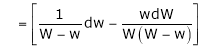
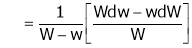
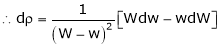
Is the required error in density.
3. Find the percentage error in computing the parallel resistance r of three resistances r1, r2, r3 from the formula.
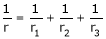
Where
r1, r2, r3 are each in error of 1 & 2y.
4. Find the approximate value of 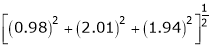
Solution:
Let 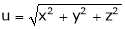
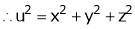
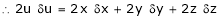
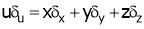 … (1)
… (1)
Take x = 1, y = 2, z = 2
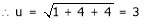
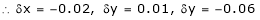
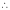 From equation (1)
From equation (1)

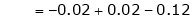
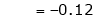
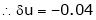
 approximate value is
approximate value is 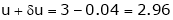
In calculating the volume of right circular cone, error of 2y & 1.5y are made in measuring height and radius of base resp. Find they error in the calculated volume.
If 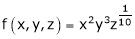 , find the approximate value of f if x = 1.99, y = 3.01, z = 0.98.
, find the approximate value of f if x = 1.99, y = 3.01, z = 0.98.
Key takeaways-
- If f be a function of variables x, y, z, t, ………. Then we have
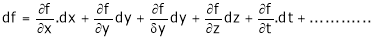
5. dx, dy, dz, ……. May be taken as actual errors or interments in x, y, z ……… resp. While df is the approximate error in f.
6. 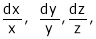 ……….. Denotes relative errors in x, y, z,………….. Resp.
……….. Denotes relative errors in x, y, z,………….. Resp.
If f(x) is a single valued function defined in a region R then
Maxima is a maximum point  if and only if
if and only if 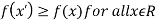
Minima is a minimum point 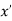 if and only if
if and only if 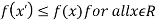
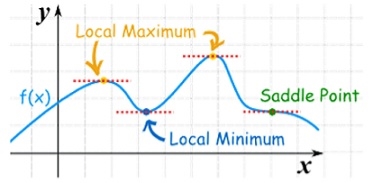


Maxima and Minima of a function of two independent variables
Let 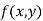 be a defined function of two independent variables.
be a defined function of two independent variables.
Then the point 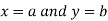 is said to be a maximum point of
is said to be a maximum point of 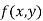 if
if
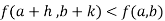
Or  =
= 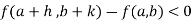
For all positive and negative values of h and k.
Similarly the point 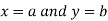 is said to be a minimum point of
is said to be a minimum point of 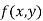 if
if
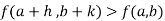
Or 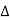 =
= 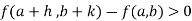
For all positive and negative values of h and k.
Saddle point: Critical points of a function of two variables are those points at which both partial derivatives of the function are zero. A critical point of a function of a single variable is either a local maximum, a local minimum, or neither. With functions of two variables there is a fourth possibility - a saddle point.
A point is a saddle point of a function of two variables if
![2 2 [ 2 ] 2
@f-= 0, @f--= 0, and @-f- @-f-- -@-f- < 0
@x @y @x2 @y2 @x @y](https://glossaread-contain.s3.ap-south-1.amazonaws.com/epub/1642980373_1435342.png)
At the point.
Stationary Value
The value 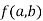 is said to be a stationary value of
is said to be a stationary value of 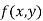 if
if
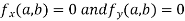
i.e. the function is a stationary at (a , b).
Rule to find the maximum and minimum values of 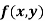
- Calculate
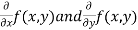 .
. - Form and solve
 , we get the value of x and y let it be pairs of values
, we get the value of x and y let it be pairs of values 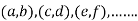
- Calculate the following values :
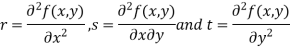
4. (a) If 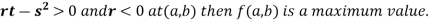
(b) If 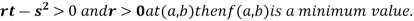
(c) If 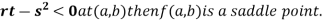
(d) If 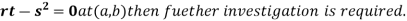
Example-1: Find out the maxima and minima of the function
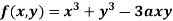
Sol.
Given
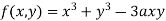 …(i)
…(i)
Partially differentiating (i) with respect to x we get
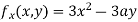 ….(ii)
….(ii)
Partially differentiating (i) with respect to y we get
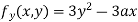 ….(iii)
….(iii)
Now, form the equations 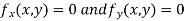
Using (ii) and (iii) we get
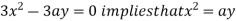
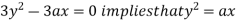
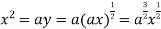 using above two equations
using above two equations
Squaring both side we get
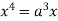
Or 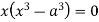
This show that 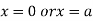
Also we get 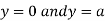
Thus we get the pair of value as 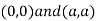
Now, we calculate
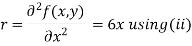
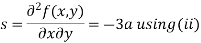

Putting above values in
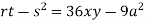
At point (0,0) we get
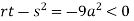
So, the point (0,0) is a saddle point.
At point 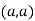 we get
we get
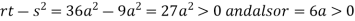
So the point 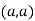 is the minimum point where
is the minimum point where 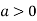
In case 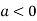
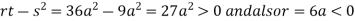
So the point 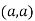 is the maximum point where
is the maximum point where 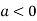
Example2 Find the maximum and minimum point of the function

Partially differentiating given equation with respect to and x and y then equate them to zero
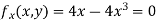
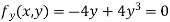
On solving above we get 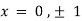
Also 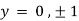
Thus we get the pair of values (0,0), (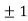 ,0) and (0,
,0) and (0,
Now, we calculate



At the point (0,0)
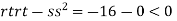
So function has saddle point at (0,0).
At the point (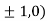
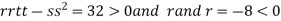
So the function has maxima at this point (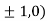 .
.
At the point (0,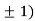
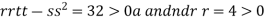
So the function has minima at this point (0,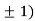 .
.
At the point (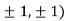
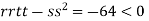
So the function has an saddle point at (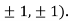
Example-3: There is rectangular box which is open at the top, is to have a volume of 32 c.c.
Find the dimensions of the box requiring least (minimum) material to construct it.
Sol. Here it is given that-
Volume (V) = 32 c.c.
Suppose ‘l’ , ‘b’, ‘h’ are the length, breadth, height of the rectangular box respectively and its surface area is ‘S’.
As we know that-
Volume (V) = l b h = 32
b (breadth) = 32/lh
And the surface area of the rectangular box which is open at the top is-
S = 2 (l + b) h + l b …………… (1)
On putting the value of ‘b’ in (1), we get-
S = 2 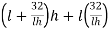
S =  ……………… (2)
……………… (2)
Differentiate partially equation (2) with respect to l and h respectively, we get-
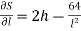 …………… (3) and
…………… (3) and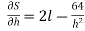 …………….. (4)
…………….. (4)
For Max. And Min. S, we get-
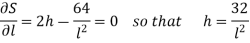
And 
The values of ‘l’, ‘h’ and ‘b’ will be-
L = 4, h = 2 and b = 4
Now-
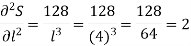
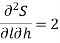
And
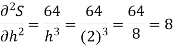
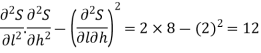
So that-
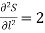 , then S is minimum for l = 4, b = 4, and h = 2
, then S is minimum for l = 4, b = 4, and h = 2
Key takeaways-
- A point is a saddle point of a function of two variables if
![2 2 [ 2 ] 2
@f-= 0, @f--= 0, and @-f- @-f-- -@-f- < 0
@x @y @x2 @y2 @x @y](https://glossaread-contain.s3.ap-south-1.amazonaws.com/epub/1642980376_7901099.png)
At the point.
2. The value 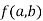 is said to be a stationary value of
is said to be a stationary value of  if
if
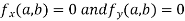
Suppose 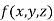 is a function of three independent variables, where x,y,z are related to a constraint
is a function of three independent variables, where x,y,z are related to a constraint
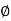 (x, y, z) = 0 …………………… (1)
(x, y, z) = 0 …………………… (1)
So that for stationary values-
 ,
, 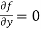 ,
, 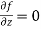
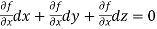 ………………….. (2)
………………….. (2)
By total differentiation of (1), we get-
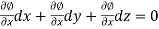 …………………… (3)
…………………… (3)
Now multiply by 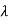 and adding to (2), we get-
and adding to (2), we get-
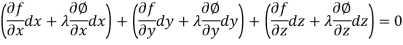
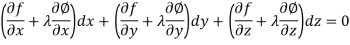
That equation holds good if-
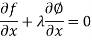
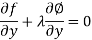
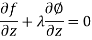
We can find the values of x, y, z and 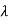 by solving these equations.
by solving these equations.
Example-1: Determine the maxima and minima of 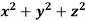 when
when 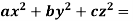 1
1
Sol. Suppose 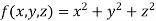 ……….. (1)
……….. (1)
And 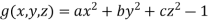 =0 …………… (2)
=0 …………… (2)
Differentiate partially equation (1) and (2) w.r.t. x and y respectively, we get-
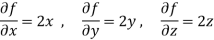
And 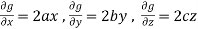
Now using Lagrange’s equations, we get-
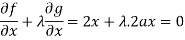
Which gives-
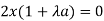 or
or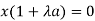 ……… (3)
……… (3)
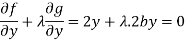
Which gives-
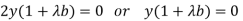 …… (4)
…… (4)
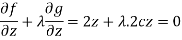
Which gives-
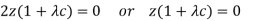 ……. (5)
……. (5)
Multiply these equations by x, y, z respectively and adding, we get-
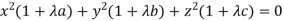
(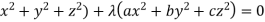
Hence we get-
f + 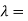 0 then
0 then 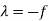
Put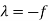 in (3) , (4) and (5), we get-
in (3) , (4) and (5), we get-
x(1 – fa) = 0 , y(1 – fb) = 0 and z(1 – fc) = 0
We get-
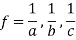
These gives the maximum and minimum values of f.
Example: Decampere a positive number ‘a’ in to three parts, so their product is maximum
Solution:
Let x, y, z be the three parts of ‘a’ then we get.
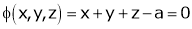 … (1)
… (1)
Here we have to maximize the product
i.e. 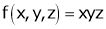
 By Lagrange’s undetermined multiplier, we get,
By Lagrange’s undetermined multiplier, we get,
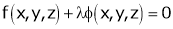
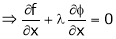 … (2)
… (2)
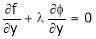 … (3)
… (3)
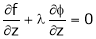 … (4)
… (4)
i.e.
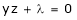 … (2)’
… (2)’
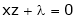 … (3)’
… (3)’
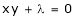 … (4)
… (4)
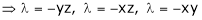
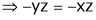
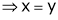
And 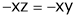
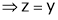
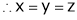
From (1)
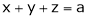
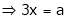
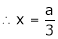
Thus 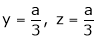 .
.
Hence their maximum product is 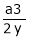 .
.
Example: Find the point on plane 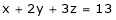 nearest to the point (1, 1, 1) using Lagrange’s method of multipliers.
nearest to the point (1, 1, 1) using Lagrange’s method of multipliers.
Solution:
Let 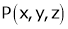 be the point on sphere
be the point on sphere 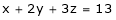 which is nearest to the point
which is nearest to the point 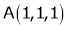 . Then shortest distance.
. Then shortest distance.
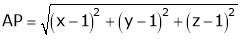
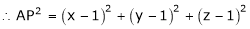
 Let
Let 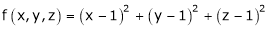
Under the condition 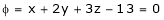 … (1)
… (1)
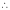 By method of Lagrange’s undetermined multipliers we have
By method of Lagrange’s undetermined multipliers we have
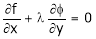
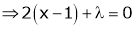 … (2)
… (2)
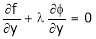
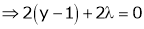 … (3)
… (3)
i.e. 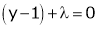 &
&
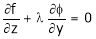
 … (4)
… (4)
From (2) we get 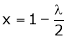
From (3) we get 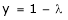
From (4) we get 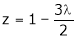
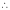 Equation (1) becomes
Equation (1) becomes
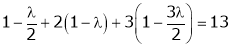
i.e. 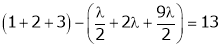
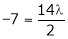
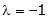
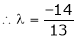
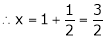
y = 2
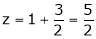
Maxima and minima of function of two variables and three variables using method of Lagrange’s multiplier-
Generally we calculate the stationary value of a function with some relation by converting the given function into the least possible independent variables and then solve them.
When this method fails we use Lagrange’s method.
This method is used to calculate the stationary value of a function of several variables which are all not independent but are connected by some relation.
Let  be the function in the variable x, y and z which is connected by the relation
be the function in the variable x, y and z which is connected by the relation 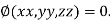
Rule: a) Form the equation
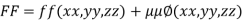
Where  is a parameter.
is a parameter.
b) Form the equation using partial differentiation is
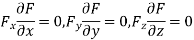
(We always try to eliminate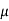 )
)
c) Solve the all above equation with the given relation 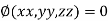
These give the value of 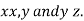
These value obtained when substituted in the given function will give the stationary value of the function.
Example1 Divide 24 into three parts such that the continued product of the first, square of second and cube of third may be maximum.
Let first number be x, second be y and third be z.
According to the question
Let the given function be f
And the relation 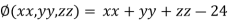
By Lagrange’s Method
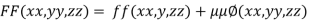
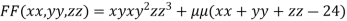 ….(i)
….(i)
Partially differentiating (i) with respect to x,y and z and equate them to zero
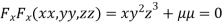 ….(ii)
….(ii)
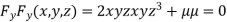 ….(iii)
….(iii)
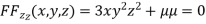 ….(iv)
….(iv)
From (ii),(iii) and (iv) we get
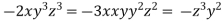
On solving
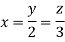
Putting it in given relation we get
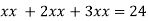
Or 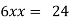
Or 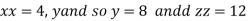
Thus the first number is 4 second is 8 and third is 12
Example2-The temperature T at any point 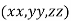 in space is
in space is 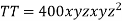 .Find the highest temperature on the surface of the unit sphere.
.Find the highest temperature on the surface of the unit sphere.
Given function is 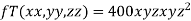
On the surface of unit sphere given  [
[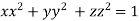 is an equation of unit sphere in 3 dimensional space]
is an equation of unit sphere in 3 dimensional space]
By Lagrange’s Method
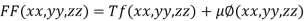
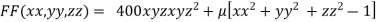 ….(i)
….(i)
Partially differentiating (i) with respect to x, y and z and equate them to zero
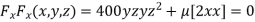 or
or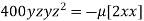 …(ii)
…(ii)
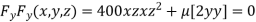 or
or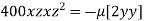 …(iii)
…(iii)
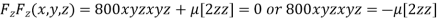 …(iv)
…(iv)
Dividing (ii) and (ii) by (iv) we get
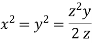
Using given relation 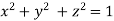
Or  Or
Or 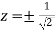
So that 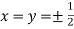
Or 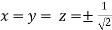
Thus points are 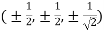
The maximum temperature is 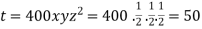
Example3 If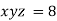 ,Find the value of x and y for which
,Find the value of x and y for which 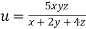 is maximum.
is maximum.
Given function is 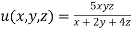
And relation is 
By Lagrange’s Method
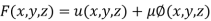
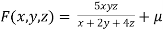 [
[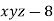 ] ..(i)
] ..(i)
Partially differentiating (i) with respect to x, y and z and equate them tozero
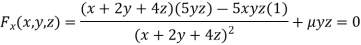
Or 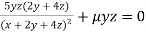 …(ii)
…(ii)
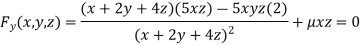
Or 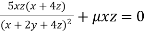 …(iii)
…(iii)
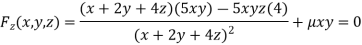
Or 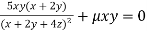 …(iv)
…(iv)
On solving (ii),(iii) and (iv) we get
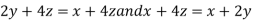
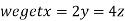
Using the given relation we get 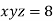
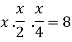
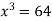
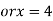
So that 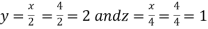
Thus the point for the maximum value of the given function is 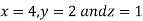
Example-4: Find the points on the surface 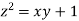 nearest to the origin.
nearest to the origin.
Let 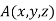 be any point on the surface, then its distance from the origin
be any point on the surface, then its distance from the origin 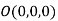 is
is
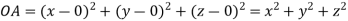
Thus the given equation will be
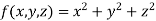
And relation is 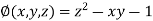
By Lagrange’s Method
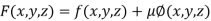
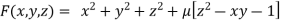 ….(i)
….(i)
Partially differentiatig (i) with respect to x, y and z and equate them to zero
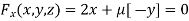
Or 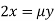 …(ii)
…(ii)
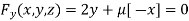
Or 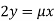 …(iii)
…(iii)
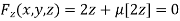
Or 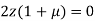
Or 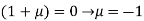
On solving equation (ii) by (iii) we get
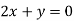
And 
On subtracting we get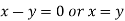
Putting in above 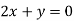
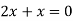 Or
Or 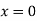
Thus 
Using the given relation we get
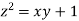 = 0.0 +1=1
= 0.0 +1=1
Or 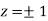
Thus point on the surface nearest to the origin is 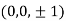
References
1. Erwin Kreyszig, Advanced Engineering Mathematics, 9thEdition, John Wiley & Sons, 2006.
2. N.P. Bali and Manish Goyal, A textbook of Engineering Mathematics, Laxmi Publications.
3. Higher engineering mathematic, Dr. B.S. Grewal, Khanna publishers
4. HK dass, engineering mathematics.