Unit 2

Wave packet and Wave equation

2.1 Concept of Group and phase velocities

To understand the difference between phase and group velocity of waves, consider the following analogy.

A group of people, say city marathon runners, start from the starting at the same time. Initially it would appear that all of them are running at the same speed. As time passes, group spreads out (disperses) simply because each runner in the group is running with different speed.

If you think of phase velocity to be like the speed of an individual runner, then the group velocity is the speed of the entire group as a whole. Obviously and most often, individual runners can run faster than the group as a whole.

To stretch this analogy, we note that the phase velocity vp of waves are typically larger than the group velocity vg of waves. However, this really depends on the properties of the medium. The media in which vg = vp is called the non-dispersive medium. But the media in which vg< vp is called normal dispersion. The media in which vg > vp is called anomalous dispersive media. It must be emphasized that dispersion is a property of the medium in which a wave travel. It is not the property of the waves themselves. The relation between phase and group velocity is given by

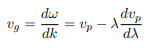
Generally, ω(k) is called the dispersion relation and indicates the dispersion properties of a medium.

As this formula predicts, if the phase velocity does not depend on the wavelength of the propagating wave, then vg = vp.

For example, sound waves are non-dispersive in air, i.e., all the individual components that make up the sound wave travel at same speed. Phase velocity of sound waves is independent of the wavelength when it propagates in air.

Monochromatic wave with angular frequency

ω = 2πν (ν is the frequency) travelling in +ve   x-direction is given by

y = A sin(ωt − kx).

On the other hand, group velocity refers to a group composed of waves within a frequency band ∆ω.

Group velocity is the velocity with which the entire group of waves would travel.

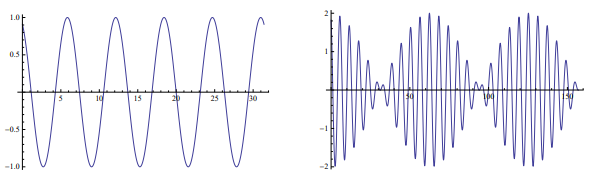
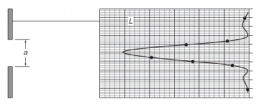
In above fig--

y = sin (2 +t)

y = sin (2 +t) + sin (2 + 1.1t).

The last form is the sum of two waves whose frequencies differ by 0.1.

Notice that the amplitude of the group is modulated as a function of t. The example here shows the waves as a function of t, but similar scenario holds good for waves as a function of x. For the travelling wave shown in the left panel of the figure, phase velocity is the velocity with which any one of the peaks progresses. However, for the right panel of the figure, the speed of any of the peaks would give the group velocity.

Relation between group velocity and phase velocity:

For a wave group formed by the superposition of number of waves, the group velocity and phase velocity are given by

For any propagating wave packet –

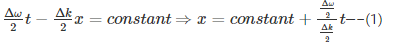
Velocity is the rate of change of displacement given by--

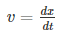
Hence, group velocity is got by differentiating equation (1) with respect to time…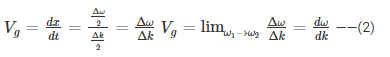

We know that phase velocity is given by

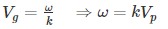
Substituting?? = kVp in equation (2) we get-

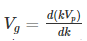
Thus, we arrive at the equation relating group velocity and phase velocity –

2.2 Wave Packet: --

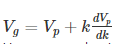
We know that by Heisen­berg’s prin­ci­ple, in or­der to get some lo­cal­iza­tion of the po­si­tion of a par­ti­cle, some un­cer­tainty must be al­lowed in mo­men­tum. That means that you must take the ini­tial mo­men­tum space wave func­tion  to be nonzero over at least some small in­ter­val of dif­fer­ent mo­men­tum val­ues . Such a com­bi­na­tion of com­po­nent waves is called a wave packet.

The wave func­tion for a typ­i­cal wave packet is sketched below. The red line is again the real part of the wave func­tion, and the black lines are the en­ve­lope en­clos­ing the wave; they equal plus and mi­nus the mag­ni­tude of the wave func­tion.

The real part (red) and mag­ni­tude or en­ve­lope (black) of a wave packet. (Schematic).

If the en­ve­lope changes lo­ca­tion with time, and it does, then so does the re­gion where the par­ti­cle can be found. This then fi­nally is the cor­rect pic­ture of mo­tion in quan­tum me­chan­ics: the re­gion in which the par­ti­cle can be found prop­a­gates through space.

The clas­si­cal de­scrip­tion also re­quires that the par­ti­cle moves with ve­loc­ity

u=p/m

Which is twice the speed p/2m of the wave. So, the en­ve­lope should move twice as fast as the wave. Like as in fig. Below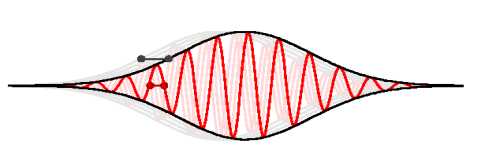

That the en­ve­lope does in­deed move at speed p/m can be seen if you de­fine the rep­re­sen­ta­tive po­si­tion of the en­ve­lope to be the ex­pec­ta­tion value of po­si­tion. That po­si­tion must be some­where in the mid­dle of the wave packet.

2.3 Heisenberg’s uncertainty principle:

Statement: It is impossible to determine simultaneously both the position and momentum of a quantum mechanical particle moving inside the wave packet with equal accuracy. In any simultaneous determination of the position and momentum of a particle, the product of the corresponding uncertainties inherently present in the measurement is equal to, greater than (h/4π).

Explanation: Consider a quantum particle moving inside a wave packet of width Δx. Then inside the wave packet it is not possible to find the exact position of the particle and any attempt to measure it will have an uncertainty (error) which less than or equal to Δx.

The maximum uncertainty involved in measuring the position of the particle within the wave packet is Δx. Since position cannot be measured accurately, there will be an uncertainty in measuring momentum also. According to Heisenberg’s uncertainty principle, the product of uncertainty involved in the measurement of these two quantities is given by the relation,

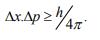
Where ∆x = uncertainty in position.

∆p = uncertainty in momentum.

It can be applied to any conjugate physical quantities such as energy and time, angular position and angular momentum etc.

Other forms of Uncertainties are:

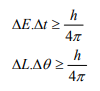
Where, ΔE, Δt are the uncertainties in the measurement of energy and time, and ΔL, Δθ are the uncertainties in the measurement of angular momentum and angular position.

2.4 Thought experiment on single slit electron diffraction: -

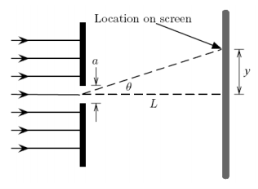
When coherent light of monochromatic wavelength is incident upon a slit, the light diverges as it passes through the slit in a process known as diffraction. A laser produces coherent light, which means all the light striking the slit is in phase. If the light then falls on a screen placed at a large distance from the slit, it produces a pattern of alternating bright and dark images of the slit. This pattern is referred to as a Fraunhofer diffraction pattern, which is the simplest case of diffraction

At each point on the screen, the light from the different portions of the slit will have a different phase due to the different path length of light from each portion of the slit to the point on the screen. Light from the different portions of the slit will interfere with each other and the resultant intensity will vary at different places on the screen

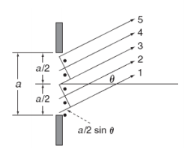
Consider wave 1 from near the bottom of the slit and wave 3 from the center of the slit. They differ in path length by the amount (a sin θ)/2 as shown, where a is the width of the slit and θ is the angle each of the rays makes with the horizontal. It is also true that the path difference between rays 2 and 4 is (a sin θ)/2. If this path difference is exactly equal to half a wavelength, i.e., λ/2, the two waves will be 180◦ out of phase and will interfere destructively and cancel each other. Therefore, waves from the upper half of the slit will be 180◦ out of phase with waves from the lower half of the slit when

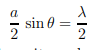
Condition for destructive interference will be satisfied at angles θ on the screen above and below the center of the pattern given by

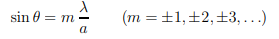
The diffraction pattern that appears on a screen will have an intensity variation above and below the slit as

In the theoretical description of the diffraction pattern, however, it is more convenient to quantify the light intensity as a function of the sine of the angle θ defined accordingly by

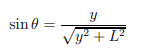
The theory of diffraction predicts that the spatial pattern of light intensity on the viewing screen by a light wave passing through a single rectangular-shaped slit is given by

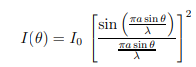
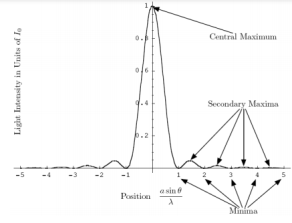
The plot is shown in Fig. It can be seen that the diffraction pattern formed when light wave passes through a rectangular-shaped slit consists of a set of bright spots (the principal maximum and many secondary maxima) interspersed with regions of darkness (the minima). A summary of the key features of this rectangular-slit diffraction pattern is as follows:

• Minima. The minima (locations of zero light intensity) occur at the angle θ given by α = a sin θ/λ = ±1, ±2, ±3, ±4 ... And are called first, second, third, fourth, . . . Minima, respectively. It is to be noted that the condition for minima is  from a simpler geometrical argument.

• Principal Maxima. The central peak, bracketed by the two “first minima” (which are located at α = a sin θ/λ = ±1), is the region of highest light intensity and most of the diffracted wave’s energy is concentrated in this region.

• Secondary Maxima. A detailed analysis of (which involves taking derivatives to find the maxima of this expression) reveals that the secondary maxima occur at angles θ given by α = a sin θ/λ = ±1.43030, ±2.45902, ±3.47089, ±4.47741 and are called the first, second, third and fourth secondary maxima are only 4.7%, 1.6%, 0.8%, 0.5% respectively of I0 (the intensity of the principal maximum).

2.5 Wave function and its probability interpretation:

The quantity that characterizes the de–Broglie wave or matter wave is called the wave function. It is usually denoted as Ψ = (x, y, z, t). This gives complete information about the state of a physical system at a particular time.

It is also called the state function and represents the probability amplitude. If ‘Ψ’ is large, the probability of finding the particle is also large and if ‘Ψ’ is small then the probability of finding the particle is small. The wave function gives the likelihood of finding the particle at a given instant and at a given position inside the wave packet.

Properties of wave functions:

There are certain properties that an acceptable wave function ‘Ψ ‘must satisfy:

1. In order to avoid infinite probabilities, Ψ must be finite for all values of x, y, z.

2.     In order to avoid multiple values of the probability, Ψ must be single valued. i.e., for each set of x, y and z, Ψ must have a unique value.

3.     For finite potentials, Ψ and 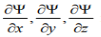must be continuous in all regions.

4.     In order to normalize the wave function, Ψ must approach to zero as ‘x’ approaches to ± infinity.

In any physical wave if ‘A’ is the amplitude of the wave, then the energy density i.e., energy per unit volume is equal to ‘A2. Similar interpretation can be made in case of mater wave also. In matter wave, if ‘Ψ ‘is the wave function of matter waves at any point in space, then the particle density at that point may be taken as proportional to ‘Ψ2’ . Thus, Ψ2 is a measure of particle density.

According to Max Born ΨΨ* = |Ψ|2 gives the probability of finding the particle in the state ‘Ψ’.  i.e., ‘Ψ2’ is a measure of probability density. The probability of finding the particle in a volume dv (= dxdydz) is given by |Ψ|2 dv or |Ψ |2 dx.dy.dz.

Since the particle has to be present somewhere, total probability of finding the particle somewhere is unity i.e., particle is certainly to be found somewhere in space.

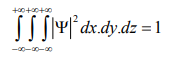or

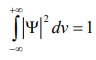
This condition is called Normalization condition. A wave function which satisfies this condition is known as normalized wave function.

2.6 Schrödinger’s Time dependent & time independent equations:

Time dependent equation:

It was observed that the wave function of a particle of fixed energy E could most naturally be written as a linear combination of wave functions of the form:

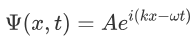
Now the hemiltonian of this system:

H=T+V

Where V is the potential energy and T is the kinetic energy. As we already know that ‘H’ is the total energy, we can rewrite the equation as:

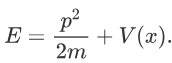
Now taking the derivatives:

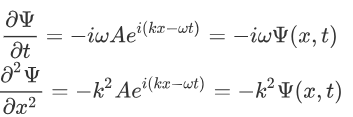
We know that:

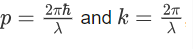
Where ‘λ’ is the wavelength and ‘k’ is the wavenumber.

We have:

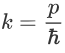
Therefore:

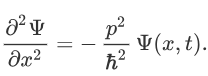
Now multiplying Ψ (x, t) to the Hamiltonian we get:

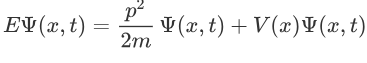
The above expression can be written as:

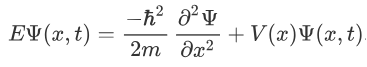
We already know that the energy wave of a matter wave is written as:

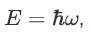
So, we can say that

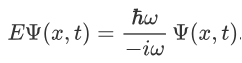
Now combining the right parts, we can get the Schrodinger Wave Equation.

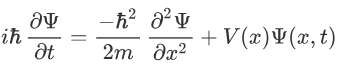
Time independent equation:

According to de-Broglie theory, for a particle of mass ‘m’, moving with a velocity ‘v’, the wavelength associated with it is

λ=h/p

The wave equation for a de-Broglie wave can be written in complex notation as:

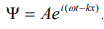…………1

Where, A is the amplitude, ω is angular frequency and k is the wave vector.

Differentiate equation (1) with respect to ‘t’ twice, we get

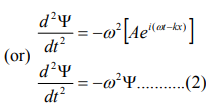
We have differential equation for the traveling wave as,

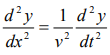……….3

Where, y is displacement and ‘v’ is velocity of wave.

By analogy, we can write the wave equation for de-Broglie wave associated with the motion of a free particle as,

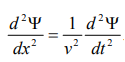………4

This represents the de-Broglie wave propagating along x-direction with a velocity ‘v’ and ‘Ψ’ is the displacement.

But we have from equation (1), p = (h/λ)

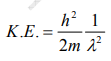
Substitute for (1/ λ)2 from equation (5),

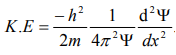…………….6

Let there be a field where the particle is present. Depending on its position in the field, the particle will possess certain potential energy. Then we can write:

Total energy = Kinetic energy + Potential energy

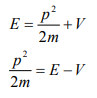
From equation (6), we can write

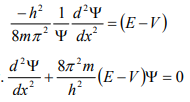
This is the time independent Schrödinger’s wave equation in one-dimension.

2.7 Solution of Schrödinger’s equation for one dimensional infinite              potential well

We will now look at the solutions of a particle of mass m confined to move along the x-axis between 0 to L. This is achieved by making the potential 0 between x = 0 and x = L and V = ∞ for x < 0 and x > L. In quantum mechanics this model is referred to as particle in a box (PIB) of length L.

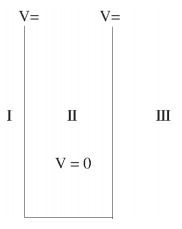
The one-dimensional infinite potential well of length L. It is divided into three regions, with regions I and III having V = ∞ and region II having V = 0

Before we set up and solve the Schrodinger equation let us apply de Broglie’s approach to this problem. De Broglie associates a wave with every material particle traveling with momentum p. The wavelength of the wave is

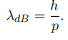
The classical analog of a particle in a box is a string that is fixed at both ends. When such a string is plucked, we know that the amplitude of the oscillations at the fixed ends is zero. In other words, an integer number of half-wavelengths must fit in the length of the box. Applying this idea to the present case, we find that

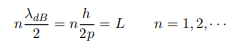
Solving for p we get

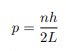
The particle feels no potential energy so all its energy is in the form of kinetic energy. As a result

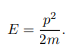
Substituting for p, we get

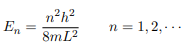
The Schrodinger equation is-

Hψˆ (x) = Eψ(x)

Where V (x) = ∞ in regions I and III and V (x) = 0 in region II. The results are in no way affected if in region II the potential is V because it only has the effect of altering the zero of energy and so without of generality, we will assume that V = 0 within the box. In regions I and III the wave function is identically zero since the potential is infinite so we will only have to consider the solution within the box. We are looking for the solutions to the equation.

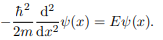
We rewrite this differential equation in the form:

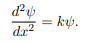
Wave equation

ψ(x) = A cos(kx) + B sin(kx)

The boundary conditions that the wave function should satisfy are

ψ (0) = 0 and ψ(L) = 0. The

First of these conditions implies that A = 0.

The second condition yields

B sin(kL) = 0

Which implies that kL = nπ, n = 1, 2, 3, · · ·

Or in other words k = nπ/L. Because E is related to k we hence obtain that

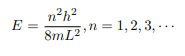
You will notice that the application of the boundary conditions naturally leads to quantization. In addition, observe that we do not consider n = 0 as a possibility because that would imply that the wave function is identically zero for all x or in other words there is no particle! The constant B is yet to be determined. The normalization condition on the wave function yields B.

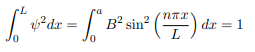
In cases where the wave function is not an eigen function of the operator corresponding to the observable, we will be concerned with the statistical mean of the measured values, averaged over a large number of measurements, the expectation value. The expectation value is defined of an operator is defined a: 

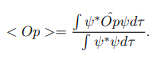
The average momentum is the

2.8 Barrier Tunneling:

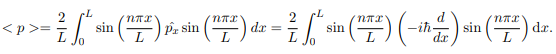
In quantum mechanics the solutions in the classically forbidden regions are exponentially decaying solutions, and they fall to zero rapidly.  But if the region in which the kinetic energy is negative is narrow, then it is possible for the exponential solution to smoothly connect two oscillating solutions on both sides of the region, and for the particle to have a finite probability of presence in that region.  This leads to the phenomena of barrier penetration and tunneling.

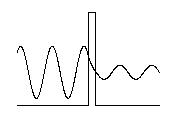
Tunneling in two dimensions:

We can consider the surface of a metal as a two-dimensional system.  Electrons cannot escape, but due to “barrier penetration”, the electron density of a metal actually extends outside the surface of the metal.  The distance x outside the surface of the metal at which the electron probability density drops to 1/1000 of that just inside the metal is on the order of 0.1 to 1 nanometer

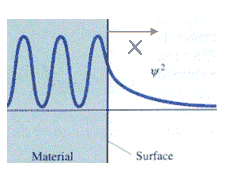
The transmittance T is the probability that an electron will tunnel through a barrier.  The transmittance T is approximately given by the simple exponential form T = exp(-2bL) with b = (2m(U0-E)/ħ2)1/2.  T depends on the difference of the electron energy E and the height of the barrier U0, and on the barrier width L.  For a metal (U0-E) is approximately equal to the work function of the metal.

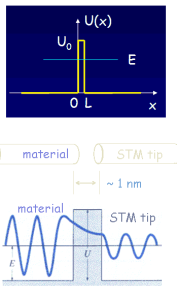
Tunneling in three dimensions:

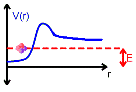
Let us consider alpha decay.  The nucleus decays by emitting an alpha particle.  An alpha particle is a Helium nucleus consisting of two protons and two neutrons.

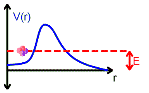
The alpha particle is trapped inside the nucleus in a potential well.  But the well walls do not extend to infinity.  If the shape of the well is of the form shown in the figure above, then there is a finite chance that he alpha particle can tunnel through the potential barrier.  The decay of an nucleus via alpha decay takes a long time if the barrier is high and thick.  Some nuclei, like lead, are considered stable but may have lifetimes greater than the age of the universe.  If the barrier is not very high and narrow, alpha decay can happen very rapidly.  If the shape of the barrier, however, is of the form shown in the figure below, then the alpha particle cannot tunnel through the barrier and the nucleus is stable against alpha decay.