Unit - 4
D'Alembert’s Principle
D’Alembert’s principle states that
For a system of mass of particles, a sum of difference of the force acting on system and time derivatives of the moment is zero when they projected onto any virtual displacement.
It is also known as Lagrange-d’Alembert principle, named after the French mathematician and physicist Jean le Rond d’Alembert. It is an alternative form of Newton’s second law of motion which states that Newton’s second law states that a acceleration of an object depends upon two variables – the net force acting on a object and the mass of the object. The acceleration of the body is directly proportional to the net forces acting on the body and inversely proportional to the mass of a body. This means that as force acting upon an object is increased, an acceleration of the object is increased. Likewise, as mass of an object is increased, the acceleration of the object is decreased. According to the 2nd law of motion, F = ma while it is represented as F – ma = 0 in D’Alembert’s law. So it is said that an object is in equilibrium when a real force is acting on it. Here, F is a real force while -ma is the fictitious force known as inertial force.
D’Alembert’s Principle Mathematical Representation
D’Alembert’s principle can be explained in mathematically the following way:
∑i(Fi−miai)δri=0
Where,
i is the integral used for a identification of variable corresponding to a particular particle in the system
Fi is a total applied force on the ith place
mi is a mass of the ith particles
ai is a acceleration of ith particles
miai is a time derivative representation
𝜹ri is a virtual displacement of ith particle
Derivation of D’Alembert Principle
Using D’Alembert’s mathematical formula, virtual work can be shown equal to the D’Alembert’s principle, which is equal to zero.
Following is the derivation:
Fi(T)=miai (total force on each particle)
Fi(T)=miai=0 (inertial force is moved left, representing quasi-static equilibrium)
δW=∑iF(T)i.δri−∑imiai.δri=0 (equated to virtual work )
δW=∑iFi.δri+∑iCi.δri−∑imiai.δri=0 (separation of applied force and constraint force)
δW=∑i(Fi−miai).δri=0 (final equation)
Examples of D’Alembert Principle
1D motion of rigid body: T – W = ma or T = W + ma where T is tension force of wire, W is weight of sample model and ma is the acceleration force.
The 2D motion of rigid body: For an object moving in an x-y plane the following is a mathematical representation: Fi= -mrc where Fi is a total force applied on a ith place, m mass of the body and arc is the position vector of the centre of mass of the body. This is the D’Alembert’s principle.
Applications
- Mass falling under gravity
- Parallel axis theorem
- Frictionless vertical hoop with a bead.
NUMERICALS ON D’-ALEMBERTS PRINCIPLE FOR CURVILENAR MOTION
1) A pendulum bob has a mass of 10 kg and released from rest when 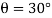 as shown in fig. Determine the tension in the chord at
as shown in fig. Determine the tension in the chord at 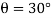 . Neglect the size of bob.
. Neglect the size of bob.
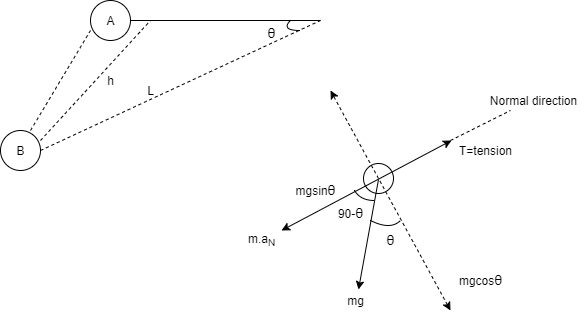
h = L sin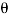
Consider the pendulum is initially at A and when it is released it occupies new position at B.
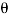 = angle displaced
= angle displaced
L = Length of string of pendulum = radius of curvature = 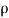 {rho}
{rho}
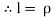
Now at point B apply D’- Alemberts Principle in normal direction ∑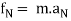
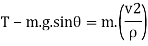
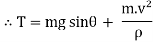
Here,
V = velocity at B = 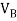
 Using equation of motion
Using equation of motion
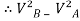 = 2gs
= 2gs
∴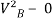 = 2gh
= 2gh
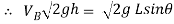
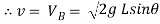
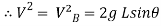 ……….. Put in equation 1
……….. Put in equation 1
We get,

At
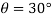
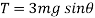
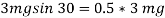 N
N
2) A 0.5 kg balls revolves in horizontal circle as shown in figure. If L = 1m and maximum allowable tension in the string is 100N determine maximum allowable speed and corresponding angle 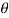 .
.
Consider the body diagram of ball as shown in figure

Apply D’-ALEMBERTS principle in x direction
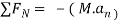
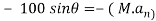
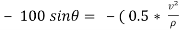 )
)

∴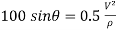
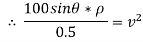
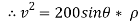
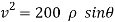 …………… (1)
…………… (1)
Apply D’- alemberts principle in y direction
∑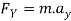
But as there is no velocity in y direction thus
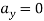
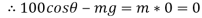
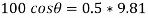
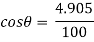
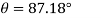 …………………………. Put in equation 1.
…………………………. Put in equation 1.
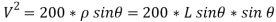
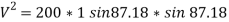
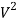 = 199.52
= 199.52
Therefore,
V= 14.12 m/s
This is max allowable speed.
Work and work done:
The work is said to be done when a force acts on a body and moves through certain distance.
W.D = Force * distance
U = F * S
Unit of work done
n.m or Joule (J)
If body dose not move in one direction of force then
W.D = Component of force in the direction of motion * displacement or distance.
Work done by external force:
A)
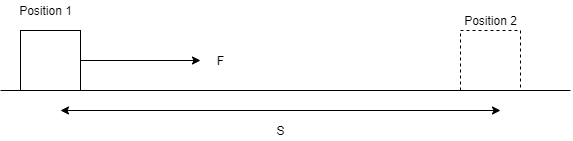
Consider an object at position (1) and subjected to force “f”.
Due to force f and object will be displaced from the position (1) to new position (2) at a distance ‘s’, then work done is given as
W.D = force * displacement
U = F * S ………. ( +ve if w.d is in the direction of the force )
B)
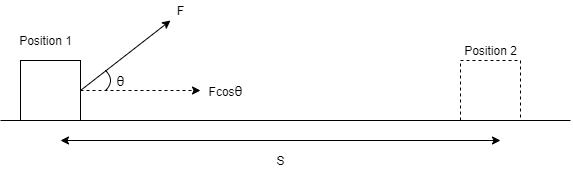
If an object is subjected to a force ‘F’ acting at an angle 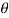 with horizontal, then object is displaced from position (1) to position (2) at distance ‘s’. Here the work is done due to component of force in direction of motion (displacement).
with horizontal, then object is displaced from position (1) to position (2) at distance ‘s’. Here the work is done due to component of force in direction of motion (displacement).
W.D = U = component of force in direction of displacement * displacement.
U = fcos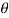 * s
* s
Work done by gravitational force gravity:

As shown in figure if object of mass ‘m’ is displaced from position (1) to position (2) then W.D IS given by,
W.D = weight * displacement in the direction of weight.
U = m.g * h
U = m.g.s sin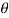
Therefore,
U = mg sin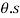
If block is moving from (1) to (2) i.e in the down direction (in the direction of the gravity) then W.D is +ve.
If block is moving from position (2) to (1) then it is against the gravity, so W.D is negative (-ve)
U = -mg sin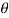 .s
.s
Work done due to fractional force:
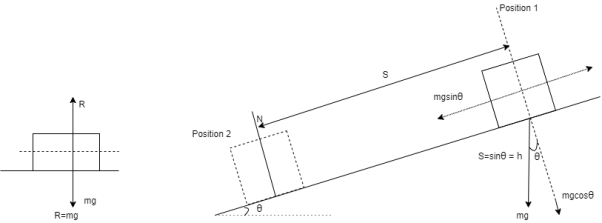
R=Normal Reaction
R=mg for horizontal plane
R=mg cos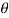 for inclined plane
for inclined plane
 Fraction force= Fr=Uk
Fraction force= Fr=Uk
 W.D.by friction force = Friction force
W.D.by friction force = Friction force displacement
displacement
 U=
U= 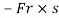
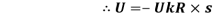
Uk= coefficient of kinetic friction
*Friction force(Fr) always act in the opposite direction of displacement .
Thus W.D is negative.
W.D.by friction force is always negative.
Use coefficient of kinetic friction Uk.
When object is moving on the horizontal plane then,
R= mg cos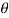
When object is moving on the inclined plane, then
R= mg. Cos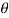
Work done by spring force
Consider spring with stiffness k as soon in figure with under formed length.
Let X1 = deformation of spring at position 1 of an object.
X2 = deformation of spring at position 1 of on object.
Then
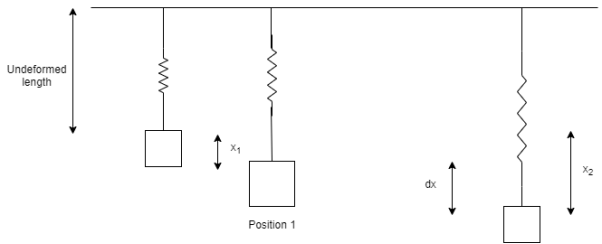
Spring force = F=k.x

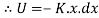
= 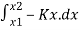
= 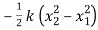
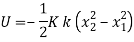
K : spring constant or stiffness = unit (N/m)
-ve sign indicates that spring force acts in the direction opposite to displacement
i.e. towards original position
Energy (energy of a particle)
The capacity to do work is called as energy
It is scaler quantity.
Type of energy:
1] Mechanical energy – Potential energy, Kinetic energy
2] Thermal energy
3] Electrical energy
Potential energy:
The energy possessed by a particle by virtue of its position is known as potential energy.
P.E.=m.g.h.
Kinetic energy:
The energy possessed by a particle due to its motion is called kinetic energy.
K.E. = 1/2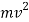 .
.
Work energy principal:
It state that “The total work done by all the forces acting on the particle during some displacement is equal to change in kinetic energy during that displacement.
From newton second law,
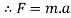
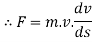
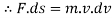
Integrate both side
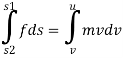
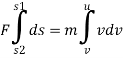
 = m.
= m.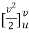
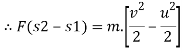
But s2-s1= s
F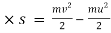
& F 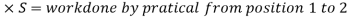
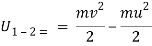
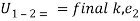 – intial k.
– intial k.
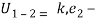 k.
k.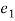
 change in kinetic energy.
change in kinetic energy.
k.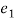 = kinetic energy of particle at postion 1
= kinetic energy of particle at postion 1
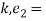 kinetic energy of particle at position 2
kinetic energy of particle at position 2
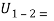 algebraic sum of w.d. By all force acting on the partical
algebraic sum of w.d. By all force acting on the partical
Conservative force:
If work done by a force is independent of the path followed by particle, then a force is known as conservation force.
Here w.d.=change of potential energy of practical.
Ex. Spring force, gravity force etc.
Non conservative force:
If work done by a force is dependent upon the followed by particle, then force is called as non-conservation force.
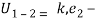 k.
k.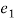
Ex. fractional force, viscous force etc.
Principal of conservation of Energy:
It states that “The total energy of a particle [P.E+ K.E.] remain constant during the displacement of particle from position 1 to position 2 under the action of conservation force .
From work energy principal:
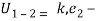 k.
k.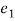 …………(1)
…………(1)
But
Under the action of conservation force,
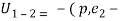 p.
p.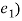 …………(2)
…………(2)
Equating 1&2
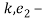 k.
k.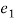 = -
= -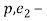 p.
p.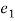
 k.
k.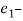 p.
p.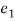
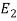 =
= 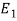
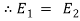 where,
where,
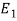 = k.
= k.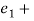 p.
p.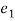 = total energy of position 1
= total energy of position 1
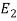 =
= 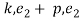 = total energy of position 2
= total energy of position 2
Where principal are involved, then above principal cannot be used.
Power:
It is rate doing work.
Power = 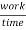
Power = 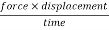
Power = force 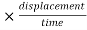
Power = force  velocity
velocity
Power 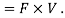
Efficiency:
The ratio of work done by machine to the work done on the machine same time is called as efficiency.
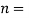 Outout power / Input power.
Outout power / Input power.
1] Linear momentum:
It is the total quantity of motion possess by a body or particle or object.
Linear momentum = mass  velocity
velocity
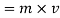 .
.
S.I. Unit: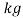 .m/sec
.m/sec
2] Angular momentum:
It is the product of mass moment of inertia of the body (I) and angular velocity of the body.
Angular movement= mass moment of inertia  angular speed
angular speed
Angular movement = Iw
SI unit is: kg.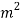 /sec
/sec
Impulse – momentum theorem:
It states that “when an unbalanced system of force is acting on the particle for short time interval, the impulse produced by all impulsive force is equal to change in momentum of particle.
From 2nd law of motion, we have
F=m.a.
F= m.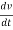
F.dt = m.dv
Integration both side between the position 1& 2,
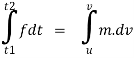
If force and mass are constant.
f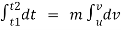
F(t)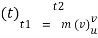
F [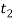 -
- 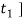 = m.[v- u ]
= m.[v- u ]
F 
But 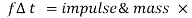 velocity = momentum
velocity = momentum
Impulse 1-2 = m1 –m2
Impulse = Final momentum - Initial momentum.
Impulse = change in momentum.
Where
Impulse = 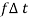 = f
= f 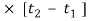
Final momentum = mv = m1
Initial momentum = mu = m2
Impulse in x direction = m (V x – U x)
Impulse in y direction = m (V y – U y)
Impulse in z direction = m (V z – U z)
Non – Impulsive force:
Any force which does not produces any kind of impulse, is called as non-impulsive force.
Ex. 1] force exerted by spring
2] force of acting reaction.
3] internal forces. Etc.
Conservation of momentum – principal:
We have impulse momentum theorem,
Impulse = final momentum – initial momentum.
But
When, 1] resultant of force is zero.
2] time interval 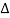 t is very very small,
t is very very small,
3] all external forces are non-impulsive,
Then
Impulse = 0.
Impulse momentum theorem can be written as,
0 = final momentum – initial momentum.
Final momentum =initial momentum.
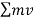 =
= 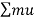 .
.
The total momentum remains constant.
i.e. Total momentum of particle is conservation.
Total momentum is conserved only in one direction.
Impact occurs when two bodies collide with each other in a very short period of time, resulting relatively huge (impulsive) forces to be exerted between the bodies.
Example: striking of a hammer on a nail, a golf club on a ball
There are two types on impact:
- Central Impact
Central impact occurs when the direction of motion of the mass centers of the two colliding particles is along a line passing through the mass centers of the particles. This line is called the line of impact which is perpendicular to plane of contact.
2. Oblique impact
When the motion of one or both of the particles make an angle with the line of impact, then the impact is said to be oblique impact
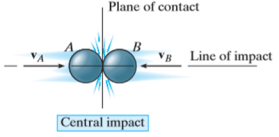
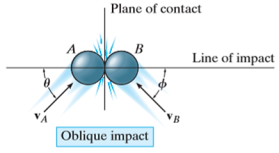
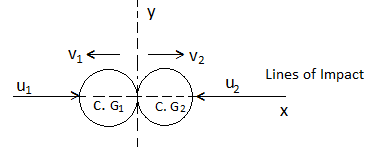
Consider two particles A and B involving the central impact of the shown in Fig. a
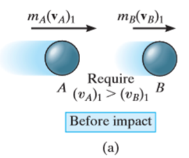
Let velocity of particle A is more than particle B.
During the collision the particles must be considered as deformable or no rigid particles. The particles will undergo a period of deformation such that they exert an equal but opposite deformation impulse 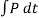 on each other.
on each other.
When the deformation becomes maximum the relative motion of particle will become zero. Hence, the particle will move with same velocity v.
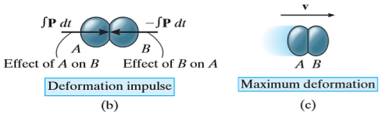
Then the particles will either return to their original shape or remain permanently deformed. This occurs after the period of restitution.
The equal but opposite restitution impulse 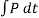 pushes the particles apart from each another, shown in fig d. In reality, the physical properties of any two bodies are such that the deformation impulse will always be greater than that of restitution.
pushes the particles apart from each another, shown in fig d. In reality, the physical properties of any two bodies are such that the deformation impulse will always be greater than that of restitution.
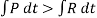
After the separation, the particles will have the final momenta as shown in Fig e.
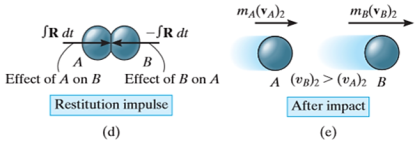
Momentum for the system of particles is conserved as during the collision the internal impulses of deformation and restitution cancel each other.
Hence,
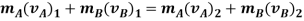
Applying impulse momentum equation for particle A during deformation phase (fig a, b and c), we get
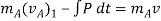
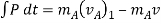
Now, applying impulse momentum equation for particle A during restitution phase (fig c, d and e), we get
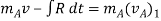

The ratio of the restitution impulse to the deformation impulse is called the coefficient of restitution.
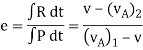
Similarly, Coefficient of restitution can be established for particle B.
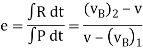
If velocity 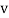 is unknown, then it can be eliminated from above equations and coefficient of restitution can be expressed in terms of the particle’s initial and final velocities
is unknown, then it can be eliminated from above equations and coefficient of restitution can be expressed in terms of the particle’s initial and final velocities
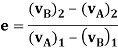
When, e = 1, the collision between the two particles is perfectly elastic. In perfectly elastic collision, the deformation impulse is equal and opposite to the restitution impulse. This is the ideal case and is impossible to achieve this case.
When e = 0, the collision between the two particles is perfectly plastic. In this case there is no restitution impulse so that after collision both particles couple or stick together and move with a common velocity.
When the velocities of the two colliding bodies and their mass centre lies on the line of impact, then it is called as direct central impact.
References:
1. Engineering Mechanics: F. L. Singer
2. Engineering Mechanics: Timoshenko & Young
3. Engineering Mechanics: Bear & Johnston
4. Engineering Mechanics: I. H. Shames
5. Engineering Mechanics: A. Nelson
Unit - 4
D'Alembert’s Principle
D’Alembert’s principle states that
For a system of mass of particles, a sum of difference of the force acting on system and time derivatives of the moment is zero when they projected onto any virtual displacement.
It is also known as Lagrange-d’Alembert principle, named after the French mathematician and physicist Jean le Rond d’Alembert. It is an alternative form of Newton’s second law of motion which states that Newton’s second law states that a acceleration of an object depends upon two variables – the net force acting on a object and the mass of the object. The acceleration of the body is directly proportional to the net forces acting on the body and inversely proportional to the mass of a body. This means that as force acting upon an object is increased, an acceleration of the object is increased. Likewise, as mass of an object is increased, the acceleration of the object is decreased. According to the 2nd law of motion, F = ma while it is represented as F – ma = 0 in D’Alembert’s law. So it is said that an object is in equilibrium when a real force is acting on it. Here, F is a real force while -ma is the fictitious force known as inertial force.
D’Alembert’s Principle Mathematical Representation
D’Alembert’s principle can be explained in mathematically the following way:
∑i(Fi−miai)δri=0
Where,
i is the integral used for a identification of variable corresponding to a particular particle in the system
Fi is a total applied force on the ith place
mi is a mass of the ith particles
ai is a acceleration of ith particles
miai is a time derivative representation
𝜹ri is a virtual displacement of ith particle
Derivation of D’Alembert Principle
Using D’Alembert’s mathematical formula, virtual work can be shown equal to the D’Alembert’s principle, which is equal to zero.
Following is the derivation:
Fi(T)=miai (total force on each particle)
Fi(T)=miai=0 (inertial force is moved left, representing quasi-static equilibrium)
δW=∑iF(T)i.δri−∑imiai.δri=0 (equated to virtual work )
δW=∑iFi.δri+∑iCi.δri−∑imiai.δri=0 (separation of applied force and constraint force)
δW=∑i(Fi−miai).δri=0 (final equation)
Examples of D’Alembert Principle
1D motion of rigid body: T – W = ma or T = W + ma where T is tension force of wire, W is weight of sample model and ma is the acceleration force.
The 2D motion of rigid body: For an object moving in an x-y plane the following is a mathematical representation: Fi= -mrc where Fi is a total force applied on a ith place, m mass of the body and arc is the position vector of the centre of mass of the body. This is the D’Alembert’s principle.
Applications
- Mass falling under gravity
- Parallel axis theorem
- Frictionless vertical hoop with a bead.
NUMERICALS ON D’-ALEMBERTS PRINCIPLE FOR CURVILENAR MOTION
1) A pendulum bob has a mass of 10 kg and released from rest when 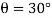 as shown in fig. Determine the tension in the chord at
as shown in fig. Determine the tension in the chord at 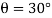 . Neglect the size of bob.
. Neglect the size of bob.
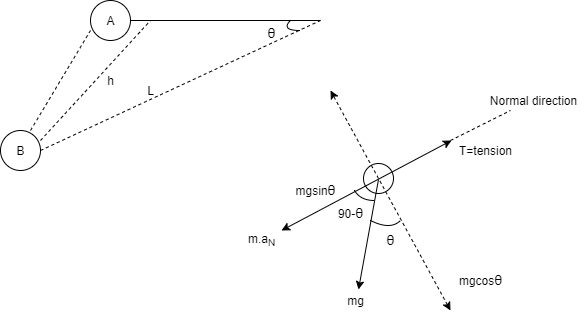
h = L sin
Consider the pendulum is initially at A and when it is released it occupies new position at B.
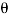 = angle displaced
= angle displaced
L = Length of string of pendulum = radius of curvature = 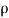 {rho}
{rho}
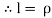
Now at point B apply D’- Alemberts Principle in normal direction ∑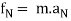
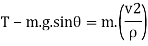
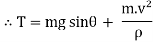
Here,
V = velocity at B = 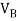
 Using equation of motion
Using equation of motion
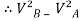 = 2gs
= 2gs
∴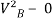 = 2gh
= 2gh
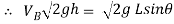
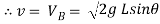
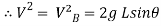 ……….. Put in equation 1
……….. Put in equation 1
We get,
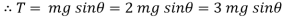
At
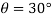
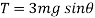
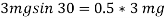 N
N
2) A 0.5 kg balls revolves in horizontal circle as shown in figure. If L = 1m and maximum allowable tension in the string is 100N determine maximum allowable speed and corresponding angle 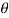 .
.
Consider the body diagram of ball as shown in figure
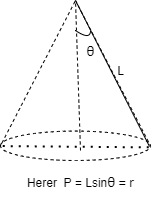
Apply D’-ALEMBERTS principle in x direction
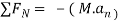
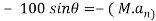
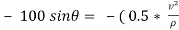 )
)
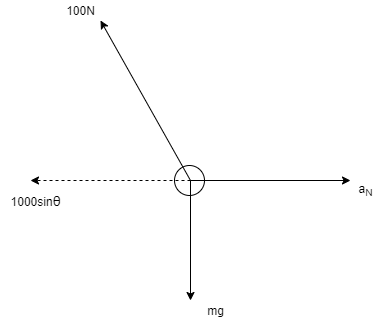
∴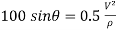

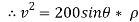
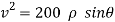 …………… (1)
…………… (1)
Apply D’- alemberts principle in y direction
∑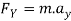
But as there is no velocity in y direction thus
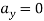
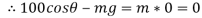
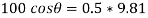
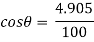
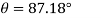 …………………………. Put in equation 1.
…………………………. Put in equation 1.
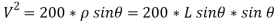
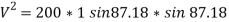
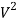 = 199.52
= 199.52
Therefore,
V= 14.12 m/s
This is max allowable speed.
Work and work done:
The work is said to be done when a force acts on a body and moves through certain distance.
W.D = Force * distance
U = F * S
Unit of work done
n.m or Joule (J)
If body dose not move in one direction of force then
W.D = Component of force in the direction of motion * displacement or distance.
Work done by external force:
A)
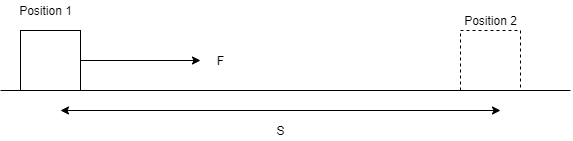
Consider an object at position (1) and subjected to force “f”.
Due to force f and object will be displaced from the position (1) to new position (2) at a distance ‘s’, then work done is given as
W.D = force * displacement
U = F * S ………. ( +ve if w.d is in the direction of the force )
B)
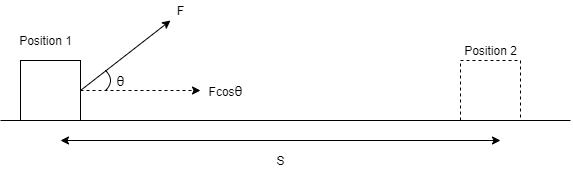
If an object is subjected to a force ‘F’ acting at an angle 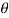 with horizontal, then object is displaced from position (1) to position (2) at distance ‘s’. Here the work is done due to component of force in direction of motion (displacement).
with horizontal, then object is displaced from position (1) to position (2) at distance ‘s’. Here the work is done due to component of force in direction of motion (displacement).
W.D = U = component of force in direction of displacement * displacement.
U = fcos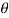 * s
* s
Work done by gravitational force gravity:
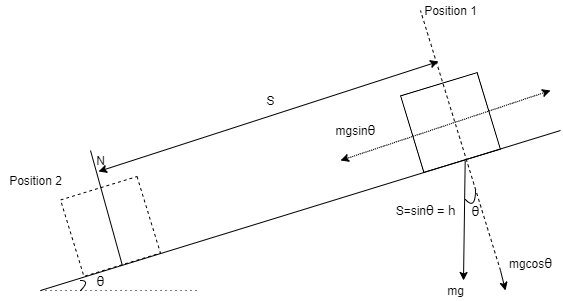
As shown in figure if object of mass ‘m’ is displaced from position (1) to position (2) then W.D IS given by,
W.D = weight * displacement in the direction of weight.
U = m.g * h
U = m.g.s sin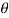
Therefore,
U = mg sin
If block is moving from (1) to (2) i.e in the down direction (in the direction of the gravity) then W.D is +ve.
If block is moving from position (2) to (1) then it is against the gravity, so W.D is negative (-ve)
U = -mg sin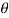 .s
.s
Work done due to fractional force:
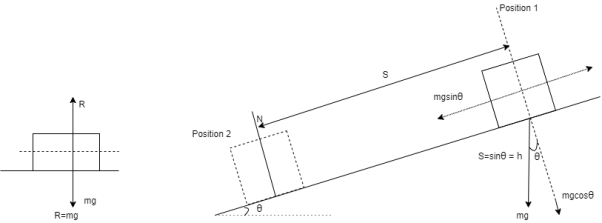
R=Normal Reaction
R=mg for horizontal plane
R=mg cos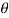 for inclined plane
for inclined plane
 Fraction force= Fr=Uk
Fraction force= Fr=Uk
 W.D.by friction force = Friction force
W.D.by friction force = Friction force displacement
displacement
 U=
U= 

Uk= coefficient of kinetic friction
*Friction force(Fr) always act in the opposite direction of displacement .
Thus W.D is negative.
W.D.by friction force is always negative.
Use coefficient of kinetic friction Uk.
When object is moving on the horizontal plane then,
R= mg cos
When object is moving on the inclined plane, then
R= mg. Cos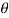
Work done by spring force
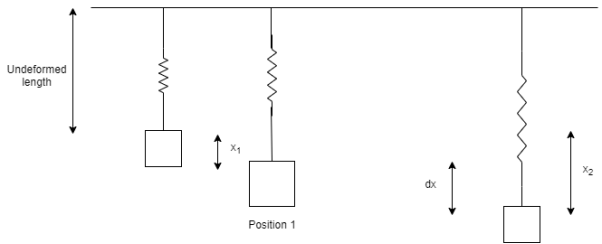
Consider spring with stiffness k as soon in figure with under formed length.
Let X1 = deformation of spring at position 1 of an object.
X2 = deformation of spring at position 1 of on object.
Then
Spring force = F=k.x

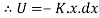
= 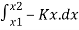
= 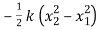
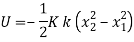
K : spring constant or stiffness = unit (N/m)
-ve sign indicates that spring force acts in the direction opposite to displacement
i.e. towards original position
Energy (energy of a particle)
The capacity to do work is called as energy
It is scaler quantity.
Type of energy:
1] Mechanical energy – Potential energy, Kinetic energy
2] Thermal energy
3] Electrical energy
Potential energy:
The energy possessed by a particle by virtue of its position is known as potential energy.
P.E.=m.g.h.
Kinetic energy:
The energy possessed by a particle due to its motion is called kinetic energy.
K.E. = 1/2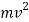 .
.
Work energy principal:
It state that “The total work done by all the forces acting on the particle during some displacement is equal to change in kinetic energy during that displacement.
From newton second law,
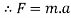
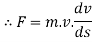
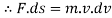
Integrate both side
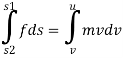
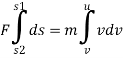
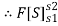 = m.
= m.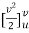
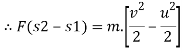
But s2-s1= s
F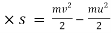
& F 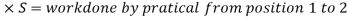
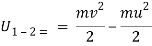
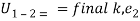 – intial k.
– intial k.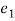
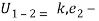 k.
k.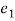
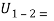 change in kinetic energy.
change in kinetic energy.
k.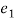 = kinetic energy of particle at postion 1
= kinetic energy of particle at postion 1
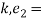 kinetic energy of particle at position 2
kinetic energy of particle at position 2
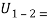 algebraic sum of w.d. By all force acting on the partical
algebraic sum of w.d. By all force acting on the partical
Conservative force:
If work done by a force is independent of the path followed by particle, then a force is known as conservation force.
Here w.d.=change of potential energy of practical.
Ex. Spring force, gravity force etc.
Non conservative force:
If work done by a force is dependent upon the followed by particle, then force is called as non-conservation force.
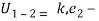 k.
k.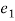
Ex. fractional force, viscous force etc.
Principal of conservation of Energy:
It states that “The total energy of a particle [P.E+ K.E.] remain constant during the displacement of particle from position 1 to position 2 under the action of conservation force .
From work energy principal:
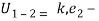 k.
k.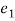 …………(1)
…………(1)
But
Under the action of conservation force,
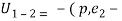 p.
p.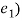 …………(2)
…………(2)
Equating 1&2
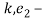 k.
k.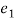 = -
= -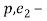 p.
p.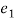
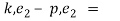 k.
k.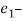 p.
p.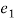
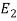 =
= 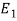
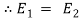 where,
where,
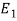 = k.
= k.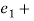 p.
p.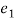 = total energy of position 1
= total energy of position 1
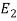 =
= 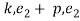 = total energy of position 2
= total energy of position 2
Where principal are involved, then above principal cannot be used.
Power:
It is rate doing work.
Power = 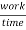
Power = 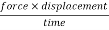
Power = force 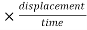
Power = force  velocity
velocity
Power 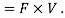
Efficiency:
The ratio of work done by machine to the work done on the machine same time is called as efficiency.
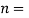 Outout power / Input power.
Outout power / Input power.
1] Linear momentum:
It is the total quantity of motion possess by a body or particle or object.
Linear momentum = mass  velocity
velocity
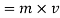 .
.
S.I. Unit: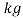 .m/sec
.m/sec
2] Angular momentum:
It is the product of mass moment of inertia of the body (I) and angular velocity of the body.
Angular movement= mass moment of inertia  angular speed
angular speed
Angular movement = Iw
SI unit is: kg.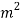 /sec
/sec
Impulse – momentum theorem:
It states that “when an unbalanced system of force is acting on the particle for short time interval, the impulse produced by all impulsive force is equal to change in momentum of particle.
From 2nd law of motion, we have
F=m.a.
F= m.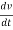
F.dt = m.dv
Integration both side between the position 1& 2,
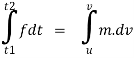
If force and mass are constant.
f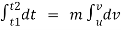
F(t)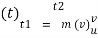
F [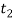 -
- 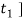 = m.[v- u ]
= m.[v- u ]
F 
But 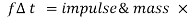 velocity = momentum
velocity = momentum
Impulse 1-2 = m1 –m2
Impulse = Final momentum - Initial momentum.
Impulse = change in momentum.
Where
Impulse = 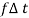 = f
= f 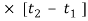
Final momentum = mv = m1
Initial momentum = mu = m2
Impulse in x direction = m (V x – U x)
Impulse in y direction = m (V y – U y)
Impulse in z direction = m (V z – U z)
Non – Impulsive force:
Any force which does not produces any kind of impulse, is called as non-impulsive force.
Ex. 1] force exerted by spring
2] force of acting reaction.
3] internal forces. Etc.
Conservation of momentum – principal:
We have impulse momentum theorem,
Impulse = final momentum – initial momentum.
But
When, 1] resultant of force is zero.
2] time interval 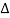 t is very very small,
t is very very small,
3] all external forces are non-impulsive,
Then
Impulse = 0.
Impulse momentum theorem can be written as,
0 = final momentum – initial momentum.
Final momentum =initial momentum.
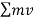 =
= 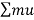 .
.
The total momentum remains constant.
i.e. Total momentum of particle is conservation.
Total momentum is conserved only in one direction.
Impact occurs when two bodies collide with each other in a very short period of time, resulting relatively huge (impulsive) forces to be exerted between the bodies.
Example: striking of a hammer on a nail, a golf club on a ball
There are two types on impact:
- Central Impact
Central impact occurs when the direction of motion of the mass centers of the two colliding particles is along a line passing through the mass centers of the particles. This line is called the line of impact which is perpendicular to plane of contact.
2. Oblique impact
When the motion of one or both of the particles make an angle with the line of impact, then the impact is said to be oblique impact
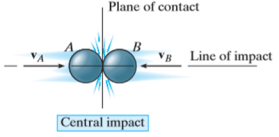

Consider two particles A and B involving the central impact of the shown in Fig. a
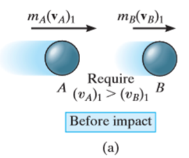
Let velocity of particle A is more than particle B.
During the collision the particles must be considered as deformable or no rigid particles. The particles will undergo a period of deformation such that they exert an equal but opposite deformation impulse 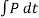 on each other.
on each other.
When the deformation becomes maximum the relative motion of particle will become zero. Hence, the particle will move with same velocity v.
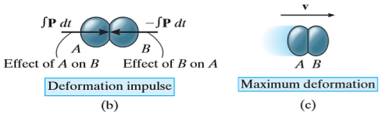
Then the particles will either return to their original shape or remain permanently deformed. This occurs after the period of restitution.
The equal but opposite restitution impulse 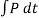 pushes the particles apart from each another, shown in fig d. In reality, the physical properties of any two bodies are such that the deformation impulse will always be greater than that of restitution.
pushes the particles apart from each another, shown in fig d. In reality, the physical properties of any two bodies are such that the deformation impulse will always be greater than that of restitution.
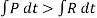
After the separation, the particles will have the final momenta as shown in Fig e.
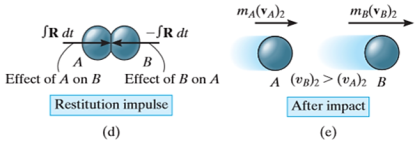
Momentum for the system of particles is conserved as during the collision the internal impulses of deformation and restitution cancel each other.
Hence,
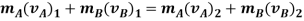
Applying impulse momentum equation for particle A during deformation phase (fig a, b and c), we get
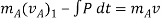
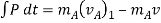
Now, applying impulse momentum equation for particle A during restitution phase (fig c, d and e), we get
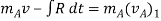
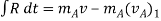
The ratio of the restitution impulse to the deformation impulse is called the coefficient of restitution.
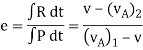
Similarly, Coefficient of restitution can be established for particle B.

If velocity 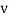 is unknown, then it can be eliminated from above equations and coefficient of restitution can be expressed in terms of the particle’s initial and final velocities
is unknown, then it can be eliminated from above equations and coefficient of restitution can be expressed in terms of the particle’s initial and final velocities
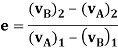
When, e = 1, the collision between the two particles is perfectly elastic. In perfectly elastic collision, the deformation impulse is equal and opposite to the restitution impulse. This is the ideal case and is impossible to achieve this case.
When e = 0, the collision between the two particles is perfectly plastic. In this case there is no restitution impulse so that after collision both particles couple or stick together and move with a common velocity.
When the velocities of the two colliding bodies and their mass centre lies on the line of impact, then it is called as direct central impact.
References:
1. Engineering Mechanics: F. L. Singer
2. Engineering Mechanics: Timoshenko & Young
3. Engineering Mechanics: Bear & Johnston
4. Engineering Mechanics: I. H. Shames
5. Engineering Mechanics: A. Nelson