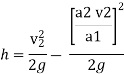Unit 2
Fluid Kinematics & Fluid Dynamics
Fluid kinematics is a branch of ‘Fluid Mechanics’ which deals with the study of velocity and acceleration of the particles of fluid in motion and then distribution in space without considering any force or energy involved.
Lagrangian method
In this method, the observer concentrates on the movement of a single particle.
The path taken by the particle and the changes in its velocity and acceleration is studied.
In the cartesian system, the position of the fluid particle in space (x, y, z) at any time t from its position (a, b, c) at time t=0 shall be given as,
x = f1(a, b, c, t)
y = f2(a, b, c, t)
z = f3(a, b, c, t)
The velocity and acceleration components (obtained by taking derivations with respect to time) are given by,
u = 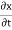
velocity components: v = 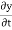
w = 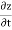
ax = 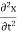
acceleration component: ay = 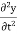
az = 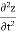
At any point, the resultant velocity or acceleration shall be the resultant of these components of the respective quantity at that point.
Resultant velocity, v = 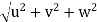
Acceleration a = 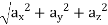
Similarly, other quantities like pressure, density, etc. can be found.
Eulerian Method
In the Eulerian method, the observer concentrates on a point in the fluid system. Velocity, acceleration, and other characteristics of the fluid at that particular point are studied.
This method is almost exclusively used a fluid mechanics, especially because of its mathematical simplicity. The velocity at any point (x, y, z) can be written as
u = f1(x, y, z, t)
v = f2(x, y, z, t)
w = f3(x, y, z, t)
The components of acceleration of the fluid particle can be worked out by partial differentiation as follows:
ax = 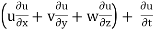
ay = 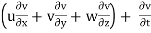
az = 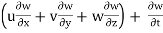
Now, resultant velocity: v = 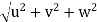
Acceleration a = 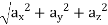
Let V is the resultant velocity at any point in a fluid flow.
Let u, v, and w are its components in x, y, and z directions.
The velocity components are functions of space coordinates and time. Mathematically, the velocity components are given as
u = f1(x, y, z, t)
v = f2(x, y, z, t)
w = f3(x, y, z, t)
and Resultant velocity, v = 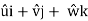
→ |v| = 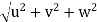
Let ax, ay, and az are the total acceleration in x, y, and z direction respectively. Then by the chain rule of differentiation, we have
ax = 
but 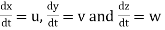
ax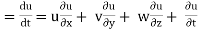
similarly, ay 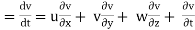
az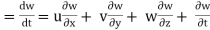
Acceleration vector a = axi + ayj + azk
= 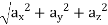
Local Acceleration and Connective Acceleration
Local acceleration is defined as the rate of increase of velocity with respect to time at a given point in a flow field.
The expression 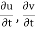 and
and  is known as local acceleration.
is known as local acceleration.
Connective acceleration is defined as the rate of change of velocity due to the change of position of fluid-particle in a fluid flow. The expression other than 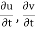 and
and 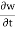 in the equation are known as connective acceleration.
in the equation are known as connective acceleration.
1) Steady and Unsteady flows
Steady flow – The type of flow in which the fluid characteristics like velocity, pressure, density, etc. at a point do not change with time is called steady flow.
Example: Flow-through prismatic or non-prismatic conduct at a constant flow rate Q m3/s is steady.
Mathematically, 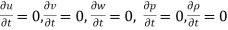
Unsteady flow: It is that type of flow in which the velocity, pressure,2 or density at a point change w.r.t. time.
E.g.: the flow in a pipe whose value is being opened or closed gradually.
Mathematically, 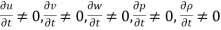
2) Uniform and Non-uniform Flows
Uniform flow – The type of flow, in which the velocity at any given time does not change with respect to space is called uniform flow.
E.g.: Flow through a straight prismatic conduit.
Mathematically, 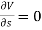 when t = constant
when t = constant
Non-uniform flow – It is the type of flow in which the velocity at any given time changes with respect to space.
E.g.: Flow-through non-prismatic conduit.
Mathematically, 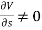 when t = constant
when t = constant
3) One, two & Three-dimensional flow
One dimensional flow – it is that type of flow in which the flow parameter such as velocity is a function of time and one space coordinate.
E.g.: Flow in a pipe where average flow parameters are considered for analysis.
Mathematically, u = f(x), v = 0 & w = 0
Two-dimensional Flow – The flow in which the velocity is a function of time and two rectangular space coordinates is called two-dimensional flow.
E.g.: Flow between parallel plates of infinite extent.
Mathematically, u = f1(x,y) v = f2(x,y) & w = 0
Three-dimensional flow – It is the type of flow in which the velocity is a function of time and three mutually perpendicular directions.
E.g.: Flow in a converging or diverging pipe or channel.
Mathematically, u = f1(x,y,z) v = f2(x,y,z) & w = f3(x,y,z)
4) Rotational and Irrotational Flows
Rotational Flow -A flow said to be rotational if the fluid particles while moving in the direction of flow rotate about their mass centers.
E.g.; Motion of liquid in a rotating tank.
Irrotational flow - A flow said to be rotational if the fluid particles while moving in the direction of flow do not rotate about their mass centers.
E.g.: Flow above a drain hole of a stationary tank or a water basin.
5) Laminar and Turbulent Flows
Laminar Flow – A Laminar flow is one in which paths taken by the individual particles do not cross one another and move along a well-defined path.
E.g.: Flow of blood in veins and arteries.
Turbulent Flow – A turbulent flow is that flow in which fluid particles move in a zig-zag way.
E.g.: High velocity flows in a conduit of large size.
6) Compressible & Incompressible Flow
Compressible Flow – It is that type of Flow in Which the density (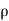 ) of the fluid changes from point to point.
) of the fluid changes from point to point.
E.g.: Flow of gases through orifices, nozzles, gas turbines, etc.
Mathematically, 
Incompressible Flow – It is the type of flow in which density is constant for the fluid flow. Liquids are generally considered flowing incompressible.
E.g.: subsonic aerodynamics.
Mathematically, 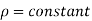
7) Subcritical, Critical & Supercritical Flow
Subcritical flow– When Froude’s number is less than one the flow is known as subcritical flow.
Mathematically, Fr 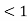
Critical flow– When Froude’s number is equal to one the flow is known as subcritical flow.
Mathematically, Fr 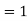
Supercritical flow– When Froude’s number is more than one the flow is known as subcritical flow.
Mathematically, Fr 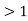
A streamline may be defined as an imaginary line within the flow so that the tangent at any point on it indicates the velocity at that point.
A stream tube is a fluid mass bounded by a group of streamlines. The contents of the stream tube are known as current filaments. Examples:- Pipes & nozzles.
A pathline is a path followed by a fluid particle in motion. A path line shows the direction of a particular particle as it moves ahead.
A streak line is a curve which gives an instantaneous time picture of the location of the fluid particles, which have passed through a given point.
E.g. : the path is taken by smoke coming out of the chimney.
The velocity potential is defined as a scalar function of space and time such that its negative derivative with respect to any direction given the fluid velocity in that direction.
A definite volume with fixed boundary shape in space along with fluid flow passage is called control volume & the boundary of his volume is known as the control surface.
The boundary of control volume may be extended up to such an extent that it includes the portion of the flow passage which is to be studied.
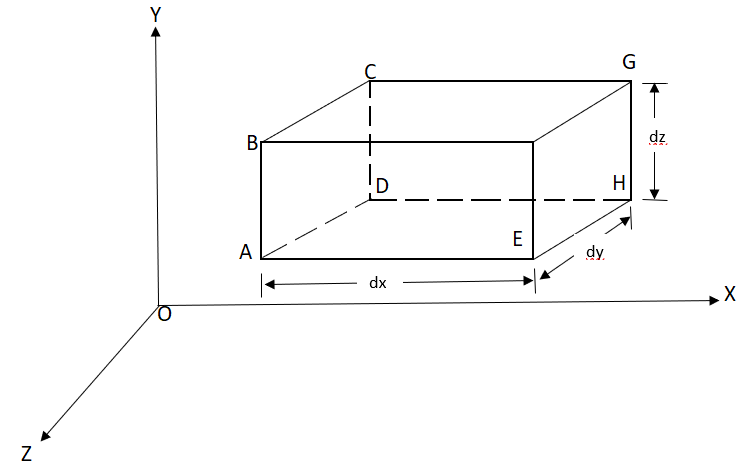
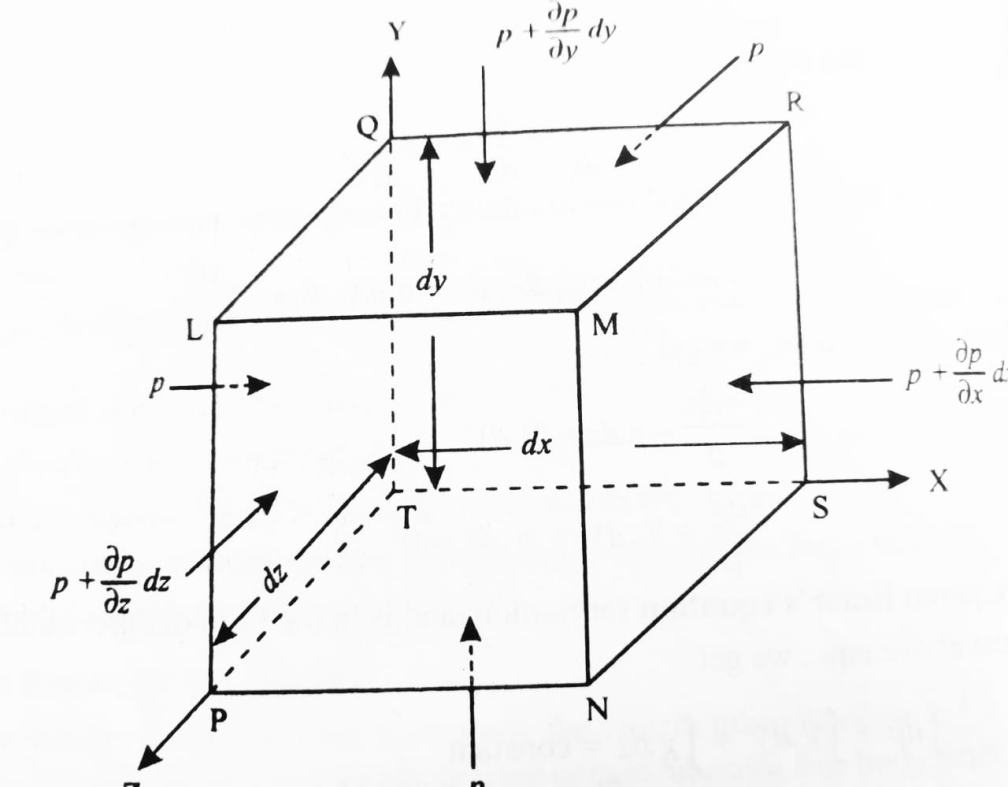
Considers a fluid element (control volume)- parallelepiped with sides dx, dy, and dz as shown in fig.
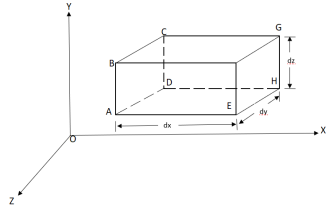
Let, 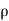 = Mass density of the fluid at a particular instant
= Mass density of the fluid at a particular instant
u, v, w= components of velocity of flow entering the three faces of the parallelepiped.
Rate of Mass of fluid entering the face ABCD
= 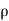 X velocity in x-direction X area of ABCD
X velocity in x-direction X area of ABCD
=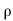 u dy dz
u dy dz
Rate of Mass of fluid leaving the lace FEGH
= 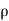 u dy dz +
u dy dz + 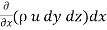
:. Mass accelerated per unit time, due to flow in x-direction
= 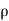 u dy dz –[
u dy dz –[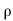 u+
u+ dx]dydz
dx]dydz
= - -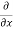 (
(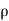 u) dx dy dz
u) dx dy dz
Similarly, the gain in fluid mass per unit time in the parallelepiped due to flow in Y and Z- direction.
= 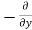 (
(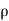 v) dx dy dz ……… (in Y- direction)
v) dx dy dz ……… (in Y- direction)
= 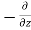 (
(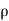 w) dx dy dz ……… (in Z- direction)
w) dx dy dz ……… (in Z- direction)
The total gain in fluid mass per unit for fluid along three co-ordinate axes
= -[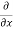 (
(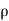 u) +
u) +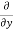 (
(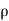 v) +
v) + 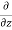 (
(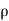 w)] dx dy dz …………1
w)] dx dy dz …………1
Rate of change of mass of the parallelepiped (control volume)
= 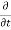 (
(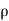 dx, dy dz) ………………….. 2
dx, dy dz) ………………….. 2
From Equation 1& 2
-[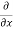 (
( u) +
u) + (
( v) +
v) +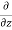 (
(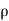 w)] dx dy dz =
w)] dx dy dz = 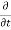 (
(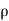 dx dy dz)
dx dy dz)
Simplification and rearrangement of teams would reduce the above expression to
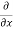 (
(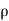 u) +
u) + 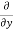 (
(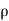 v) +
v) + 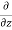 (
(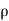 w) +
w) + 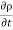 =0
=0
This eq. is the general equation of continuity in three dimensions and applies to any type of flow and for any fluid whether compressible as incompressible
For steady flow (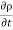 =0) incompressible fluids (
=0) incompressible fluids (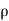 = constant) the equation reduces to
= constant) the equation reduces to
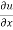 +
+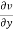 +
+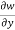 =0
=0
For two-dimensional flow eq. reduce to
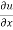 +
+ 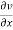 =0
=0
Potential Function
The velocity potential is defined as the scaler function of space and time such that its negative derivative with respect to any directions gives the fluid velocity in that direction.
It is denoted by Ø (phi)
Thus, mathematically the velocity potential is defined as
Ø = f(x, y,z,t)
And Ø = f(x, y, z)
u = -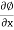
v = -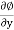
w = -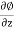
where u, v, and w are the components of velocity in the x, y, and z directions respectively.
The negative sign signifies that Ø decreases with an increase in the values of x, y, and z. in other words, it indicates that the flow is always in the direction of decreasing Ø.
Stream function –
It is defined as the scalar function of space and time such that its partial derivative with respect to any direction gives the velocity component at right angles to that direction.
It is denoted by Ψ (psi) and defined only for two-dimensional flow.
Mathematically, for ready flow, it is defined as Ψ = f(x, y) such that
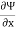 = v
= v
And 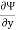 = -u
= -u
Rotational component (wz) can be given as-
It shows that Phi exits then, flow will be irrotational.
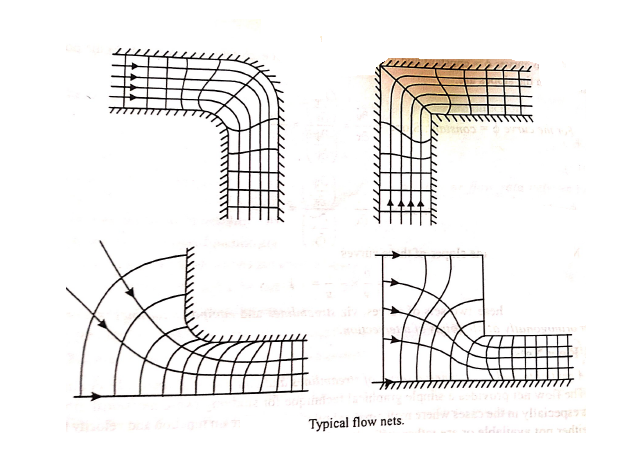
Methods of drawing flow nets
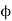
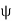
2. Graphical method
3. Electrical Analogy Method
4. Hydraulic Models
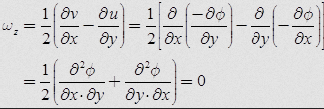
Use of flow nets
1) To determine the streamlines and equipotential lines.
2) To determine the quantity of seepage and upward lift pressure below the hydraulic structure.
3) To determine the velocity and pressure distribution for given boundaries of flow (provided the velocity distribution and pressure at any reference section are known).
4) To determine the design of the outlets for their streamlining.
Limitations of flow nets
1) The flow net analysis cannot be applied to the region close to the boundary the effects of viscosity are predominant.
2) In case of a flow of a fluid past a solid body, while the flow net gives a fairly accurate picture of the flow pattern for the upstream part of a solid body, it can give little information concerning the flow conditions at the rare because of separation & eddies.
The motion of the fluid element is influenced by the following forces
The net pressure force in X direction = p dydz – 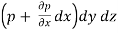
= 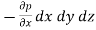
2. Gravity or body force
Let B be the body force per unit mass of fluid having components Bx, By & Bz
In x, y & z directions respectively.
Then, body force acting on the parallelepiped in the direction of X coordinate is = Bx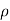 dx dydz
dx dydz
3. Inertia force
The inertia force acting on the fluid mass, along the X coordinates is given by,
Mass x acceleration = 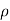 dx dydz
dx dydz
Consider the steady flow of an ideal fluid along the shown tube.
Separate a small element of fluid of cross-sectional area dA and length ds from stream tube as a free body from the during fluid.
Fig. shows such a small element LM of fluid of cross Section area dA and length ds.
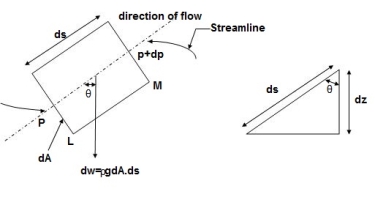
Let, p= Pressure of the elements at L
p + dp = Pressure on the element at M,and
V=Velocity of the fluid element.
The external forces tending to accelerate the fluid elements in the direction of streamline are as follows:
= -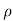 .g.dA.ds.cos
.g.dA.ds.cos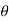
=-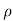 .g.dA.ds (dz/ds) (:. Cos
.g.dA.ds (dz/ds) (:. Cos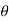 = dz/ds)
= dz/ds)
= -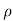 .g.dA.dz
.g.dA.dz
Mass flow of the fluid element = 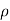 .dA.ds
.dA.ds
The Acceleration of the fluid element
a = dv/dt = dv/ds * ds/dt = v. dv/ds
Now , according to Newton’s second law of motion, force= mass x acceleration.
:. – dp. dA.-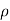 .g.dA.dz=
.g.dA.dz= 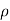 .dA.ds x v.dv/ds
.dA.ds x v.dv/ds
Dividing both sides by 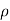 .dA, we get
.dA, we get
-dp/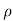 - g.dz= v.dv
- g.dz= v.dv
Or dp/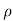 + v.dv + g.dz=0
+ v.dv + g.dz=0
This is the required Euler’s equation for motion.
Integrating the Euler’s equation of motion we get
1/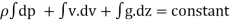
p/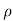 + v2 /2 + gz = constant
+ v2 /2 + gz = constant
dividing by g, we get
p/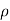 g + v2/2g + z = constant
g + v2/2g + z = constant
p/w + v2/2g + z = constant
or in other words, 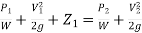 +
+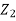
which proves Bernoulli’s equation.
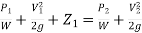 +
+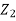 + hL
+ hL
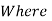 hL = Loss of energy between sections 1 & 2.
hL = Loss of energy between sections 1 & 2.
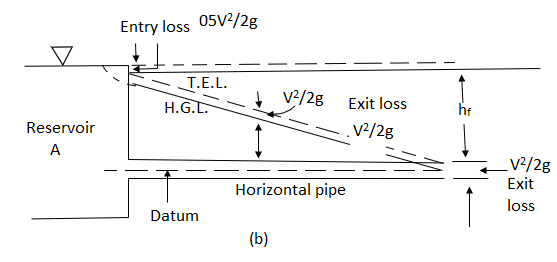
The concept of hydraulic gradient line and total energy line is quite useful in the study of the flow of fluid in pipes.
Total energy Line (T.E.L. or E.G.L)
Total head = 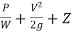
Hydraulic gradient Line (H.G.L)
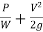
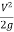
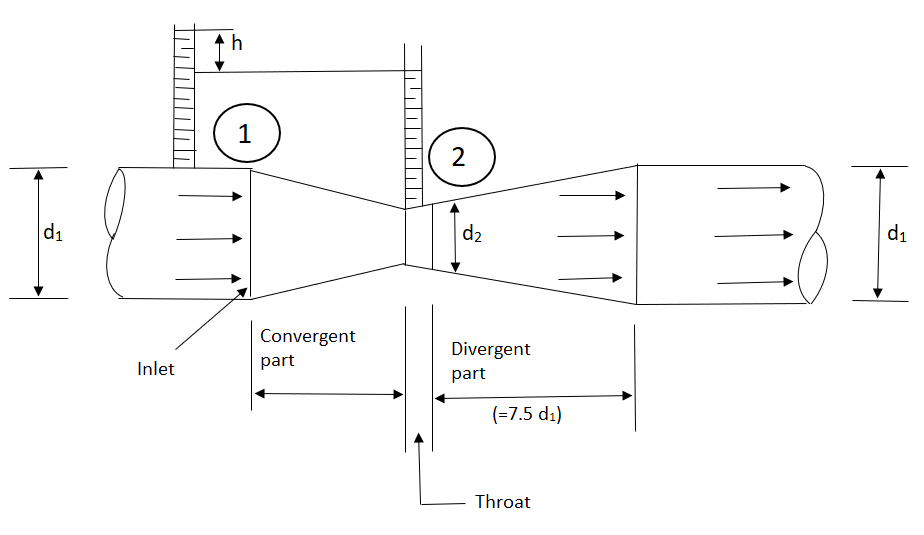
A venturimeter is a device used for measuring the rate of a flow of a fluid flowing through a pipe it consists of three parts:
i) A short converging part, ii) Throat, and (iii)Diverging Part
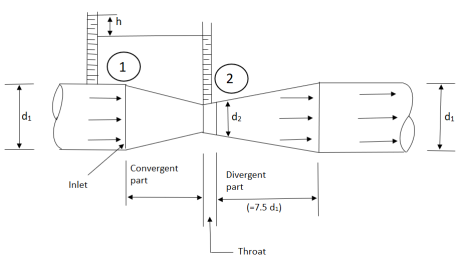
It is based on the principle of Bernoulli’s equation.
The expression for Rate of flow through venturimeter
Consider a venturimeter fitted in a horizontal pipe through which a fluid is following as shown in fig.
Let d1 = diameter at inlet or at section(1)
P1= pressure at section (1)
V1 = velocity of fluid at section (1)
a1= area of section (1) =π/4. d2
and d2, P2, V2, a2 are corresponding values at section (2)
Applying Bernoulli’s equation at section (1) and (2) we get
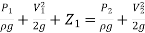 +
+
As the pipe is horizontal hence z1 = z2
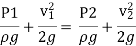
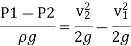
But 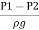 is the difference of pressure heads at section land 2 and it is equal to h
is the difference of pressure heads at section land 2 and it is equal to h
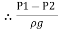 =h
=h
Substituting this value of  in the above eqn, we get.
in the above eqn, we get.
 h =
h = 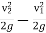 ………….. (1)
………….. (1)
Now applying continuity equation at section 1 and 2
a1 v1 = a2 v2
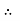 v1 =
v1 = 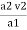
Substituting this value of v1 in equation 1
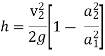
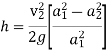
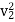 =
= 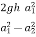
Q = a2v2
=a2 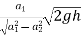
= 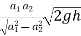
The above equation gives the discharge under ideal conditions and is called, theoretical discharge Actual discharge will be less than theoretical discharge.
Qact =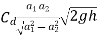
Where Cd = co-efficient of venturimeter and its value is less than 1.
Orifice Meters or an Orifice plate.
Construction-
Working-
The discharge through a rotameter is given by:

Q = Volume flow rate
Cd = coefficient of discharge.
Aann = Annular area between float and tube
Vfl = Volume of float.
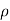 fl = Density of float material
fl = Density of float material
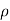 f = Density of fluid, and
f = Density of fluid, and
Af = Maximum cross-sectional area of the fluid.
As the flow area Aann is a function of the height of float in the tube, the flow rate scale can be engraved on the tube corresponding to a particular float.
Advantages
1) Simpler in operating.
2) Handling and installation are easy.
3) A wide variety of corrosive fluids can be handled.
4) Low cost.
Limitations
1) Mounted vertically, limited to small pipe sizes and capacities.
2) Less accurate compared to Venturimeter and orifice meter.
P1= intensity of pressure at point (1)
V1 = velocity of flow at (1)
P2 = pressure at point (2)
V2 = velocity at point (2), which is zero
H = depth of the tube in the liquid.
h = rise of liquid in the tube above the fire Surface
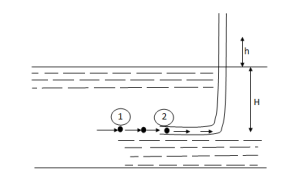
Applying Bernoulli’s equation at points (1) and (2)
We get
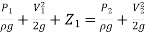 +
+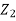
But Z1 = Z2 as points (1) and (2) are the same line and V2 =0
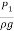 = pressure Lead at (1) = H
= pressure Lead at (1) = H
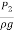 = pressure head at (2) = (h+H)
= pressure head at (2) = (h+H)
Substituting these values, we get
H+ 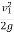 = (h+H)
= (h+H)
:. h= 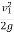 or V1 =
or V1 = 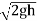
This is theoretical velocity.
Actual velocity is given by (V1)act= Cv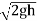
Where Cv= Co-efficient of pitot-tube
:. Velocity at any point V= Cv
Reference books:
1. Engineering Fluid Mechanics by R. J. Garde and A.J Mirajgaonkar, Pub: SCITECH Publications( India )Pvt.Ltd, Chennai
2. Fluid Mechanics and its Applications, Vijay Gupta, Santosh K Gupta, New Age international pvt. Ltd, New Delhi,
3. Fluid Mechanics, Fundamentals, and applications by Yunus. A Cengel and John.M Cimbala, Mc Graw Hill International, New Delhi.
4. Fluid Mechanics by Streeter, Wylie, and Bedford – Pub: McGraw Hill International, New Delhi.
5. Open Channel Hydraulics by Ven Tee Chow, Pub: Mcgraw- Hill Book Company- Koga.
6. A Text-Book of Fluid Mechanics and Hydraulic Machines- by Dr. R K Rajput Pub: S Chand and Co Ltd. New Delhi