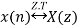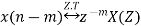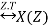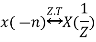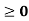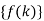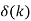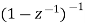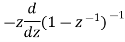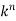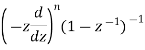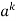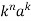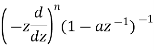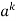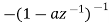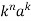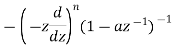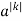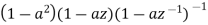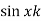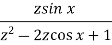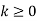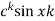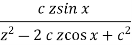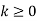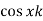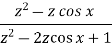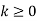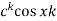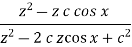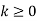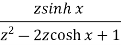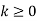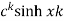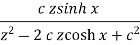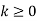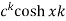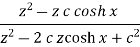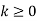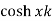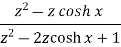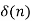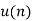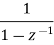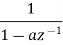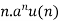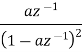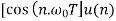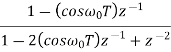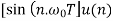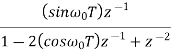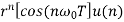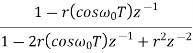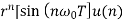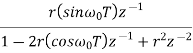Unit-2
Fourier transform & Z – Transform
|
The Fourier integral of f(x) is-
 ………. (1)
………. (1)
Which can be written as-
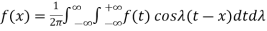 …………… (2)
…………… (2)
Because 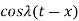 is an even function of
is an even function of 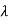 . Also since
. Also since 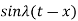 is an odd function of
is an odd function of  then we have-
then we have-
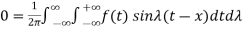 ……… (3)
……… (3)
Multiply (3) by ‘i’ and add to (2)-
We get-
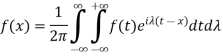
Which is the complex form of the Fourier integral.
Example 1:
Using the complex form, find the Fourier series of the function
f(x) = sinx = 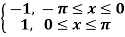
Solution:
We calculate the coefficients 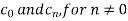
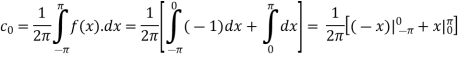
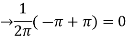

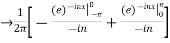 =
= 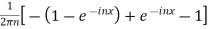
=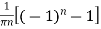
Hence the Fourier series of the function in complex form is
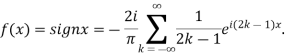
We can transform the series and write it in the real form by renaming it as
n=2k-1,n=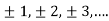
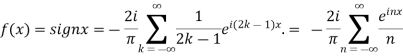
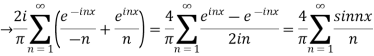
= 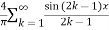
Example 2:
Using complex form find the Fourier series of the function f(x) = x2, defined on the interval [-1,1]
Solution:
Here the half-period is L=1. Therefore, the coefficient c0 is,
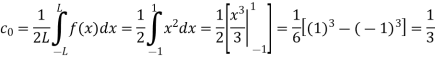
For n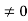
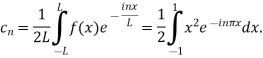
Integrating by parts twice, we obtain
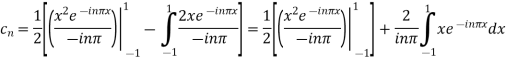
= 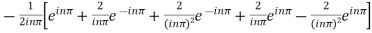
= 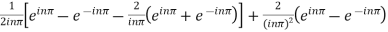
=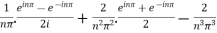 .
.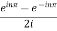
= 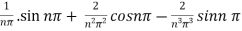 .
.
Fourier integral theorem
If f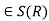 , then
, then
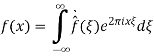
Proof. We first claim that

Let 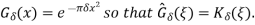 By the multiplication formula we get
By the multiplication formula we get
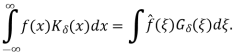
Since 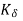 is a good kernel, n the first integral goes to f(0) as
is a good kernel, n the first integral goes to f(0) as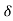 tends to 0. Since the
tends to 0. Since the
The second integral converges to 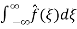 as
as 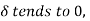 our claim is proved.
our claim is proved.
In general , let F(y)=f(y+x) so that

Example:1
Find the Fourier integral representation of the function
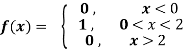
Solution:
The graph of the function is shown in the below figure satisfies the hypothesis of
Theorem -1. Hence from Eqn,(5) and (6), we have
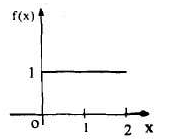
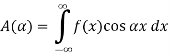
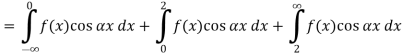
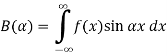
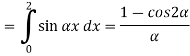
Substituting these coefficients in Eqn. (4) we obtain
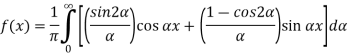
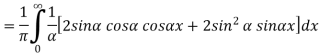
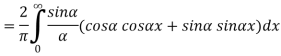
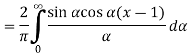
This is the Fourier integral representation of the given function.
Example:2
Find the Fourier integral representation of the function
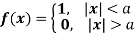
Solution:
The graph of the given function is shown in the below figure. Clearly, the given function f(x) is an even function. We represent f(x) by the Fourier cosine integral. We obtain
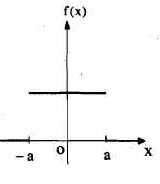
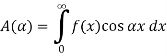
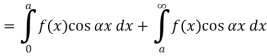
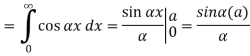
And thus,
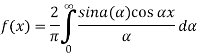
 The following notations are used to find the Fourier sine and cosine integrals.
The following notations are used to find the Fourier sine and cosine integrals.
If the function f(x) is even 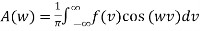

B(w)= 0

 If the function f(x) is odd
If the function f(x) is odd 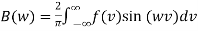

Example:1
Find the Fourier cosine integral of  , where x>0, k>0 hence show that
, where x>0, k>0 hence show that
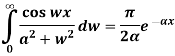
Solution:
The Fourier cosine integral of f(x) is given by:
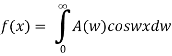
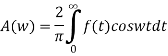
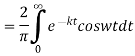
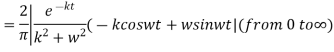
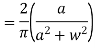
Example:2
Find the half –range sine series of the function
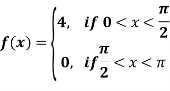
Solution: xx
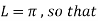
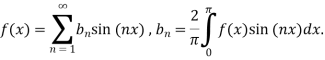
Where
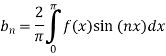
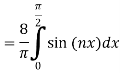
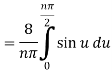
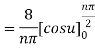
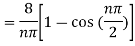
In mathematics, a Fourier transform (FT) is a mathematical transform that decomposes a function (often a function of time, or a signal) into its constituent frequencies, such as the expression of a musical chord in terms of the volumes and frequencies of its constituent notes. The term Fourier transform refers to both the frequency domain representation and the mathematical operation that associates the frequency domain representation to a function of time.
The Fourier transform of a function of time is a complex-valued function of frequency, whose magnitude (absolute value) represents the amount of that frequency present in the original function, and whose argument is the phase offset of the basic sinusoid in that frequency. The Fourier transform is not limited to functions of time, but the domain of the original function is commonly referred to as the time domain. There is also an inverse Fourier transform that mathematically synthesizes the original function from its frequency domain representation, as proven by the Fourier inversion theorem
The function-
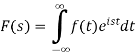
Is called the Fourier transform of the function f(x).
The function f(x) given below is called inverse Fourier transform of F(s)-
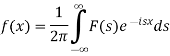
Properties of Fourier transform-
Property-1: Linear property- If F(s) and G(s) are two Fourier transforms of f(x) and g(x) respectively, then-
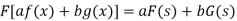
Where a and b are the constants.
Property-2: Change of scale- If F(s) is a Fourier transform of f(x), then
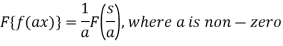
Property-3: Shifting property- If F(s) is a Fourier transform of f(x), then
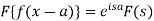
Property-4: Modulation- If F(s) is a Fourier transform of f(x), then
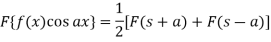
Example: Find the Fourier transform of-
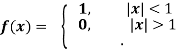
Hence evaluate 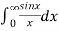
Sol. As we know that the Fourier transform of f(x) will be-
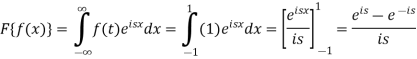
So that-
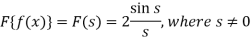
For s = 0, we get- F(s) = 2
Hence by the inverse formula, we get-
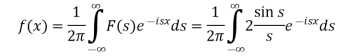
Putting x = 0, we get
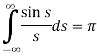
So-
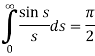
Example: Find the Fourier transform of 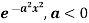
Sol. As we know that the Fourier transform of f(x) will be-
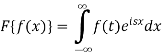
So that-
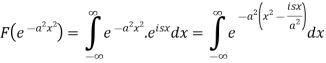
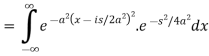
Now put 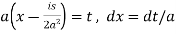
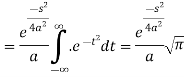
So that-
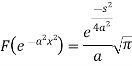
Finite Fourier sine and cosine transforms-
The finite Fourier sine transform of f(x), in 0<x<c, is given by-
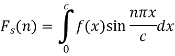
Where n is an integer.
And the function f(x) is called the inverse finite Fourier sine transform of 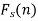 which is defined by-
which is defined by-
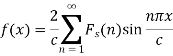
The finite Fourier cosine transform of f(x), in 0<x<c, is given by-
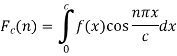
Where n is an integer.
And the function f(x) is called the inverse finite Fourier cosine transform of 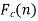 which is defined by-
which is defined by-
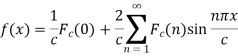
Example-1: Find the Fourier sine transform of 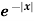
Sol. Here x being positive in the interval (0, ∞)
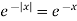
Fourier sine transform of 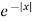 will be-
will be-
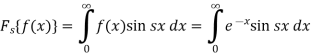
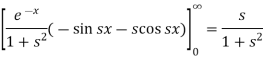
Example-2: Find the Fourier cosine transform of-
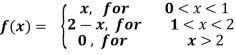
Sol. We know that the Fourier cosine transform of f(x)-
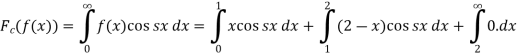
= 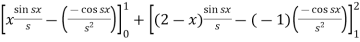
= 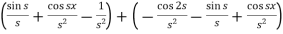
= 
Example-3: Find the Fourier sine transform of 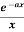
Sol. Let 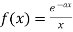
Then the Fourier sine transform will be-
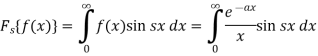
Now suppose,
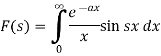
Differentiate both sides with respect to x, we get-
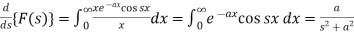 ……. (1)
……. (1)
On integrating (1), we get-
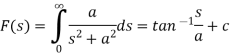
In mathematics and signal processing, the Z-transform converts a discrete-time signal, which is a sequence of real or complex numbers, into a complex frequency-domain representation. It can be considered as a discrete-time equivalent of the Laplace transform. This similarity is explored in the theory of time-scale calculus.
Analysis of continuous-time LTI systems can be done using z-transforms. It is a powerful mathematical tool to convert differential equations into algebraic equations.
The bilateral (two-sided) z-transform of a discrete-time signal x(n) is given as
Z.T[x(n)] = X(Z) = 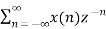
The unilateral (one sided) z-transform of a discrete time signal x(n) is given as
Z.T[x(n) = X(Z) = 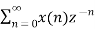
Z-transform may exist for some signals for which Discrete-Time Fourier Transform (DTFT) does not exist.
Definition
The Z-transform can be defined as either a one-sided or two-sided transform.
The bilateral or two-sided Z-transform of a discrete-time signal x(n) is the formal power series X(z) defined as
X (z) = Z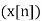 =
= 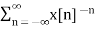
where n is an integer and z is, in general, a complex number:
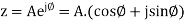

where A is the magnitude of z,j is the imaginary unit and 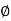 is the complex argument (also referred to as angle or phase) in radians.
is the complex argument (also referred to as angle or phase) in radians.
Alternatively, in cases where x[n] is defined only for 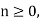 the single-sided or unilateral Z-transform is defined as
the single-sided or unilateral Z-transform is defined as
X (z) = Z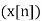 =
= 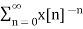
In signal processing, this definition can be used to evaluate the Z-transform of the unit impulse response of a discrete-time causal system.
An important example of the unilateral Z-transform is the probability-generating function, where the component x[n] is the probability that a discrete random variable takes the value n, and the function X(z) is usually written as X(s) in terms of s = x-1. The properties of Z-transforms (below) have useful interpretations in the context of probability theory.
Standard properties
Z-transform properties:
Z-Transform has the following properties:
If x(n)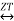 X(Z)
X(Z)
And
y(n)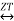 Y(Z)
Y(Z)
Then linearity property states that
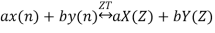
Then the time-shifting property states that
Multiplication by Exponential Sequence Property
If x(n) 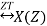
Then multiplication by an exponential sequence property is
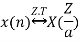
3. Time Reversal Property
Then time reversal property states that
Differentiation in Z-Domain OR Multiplication by n Property
If 
Then multiplication by n or differentiation in z-domain property states that,
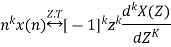
If 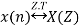
And
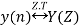
Then convolution property states that
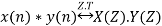
If 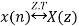
And
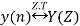
Then co-relation property states that
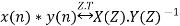
Region of Convergence (ROC) of Z-Transform
The range of variation of z for which z-transform converges is called the region of convergence of z-transform.
Properties of ROC of Z-Transforms
Some standard formulae-
1. 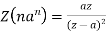
2. 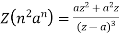
3. 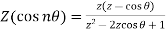
4. 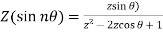
5.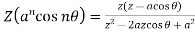
6. 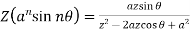
Example-1: Find Z-transform of the following functions-
(i) 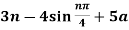
(ii) 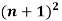
Sol. (i) 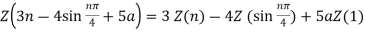
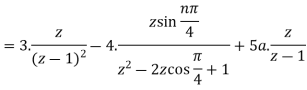
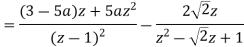
(ii) 
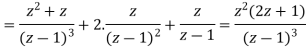
Example-2: Find Z-transform of the following functions-
(i) 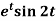
(ii) 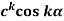
Sol. (i) As we know that-
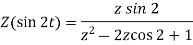
So that-
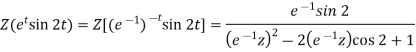
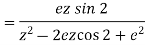
(ii) we know that-
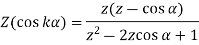
So that-
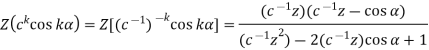
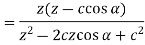
The ZT of important sequences are given in the following table-
Sequence k | ZT |
|
| F(z) |
|
| 1 |
|
U(k) |
|
|
k |
| |z|>1 |
|
| |z|>1 |
|
| |z|>|a| |
|
| |z|>|a| |
|
| |z|<|a| |
|
| |z|<|a| |
|
| |a|<|z|<1/|a| |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Inverse ZT-
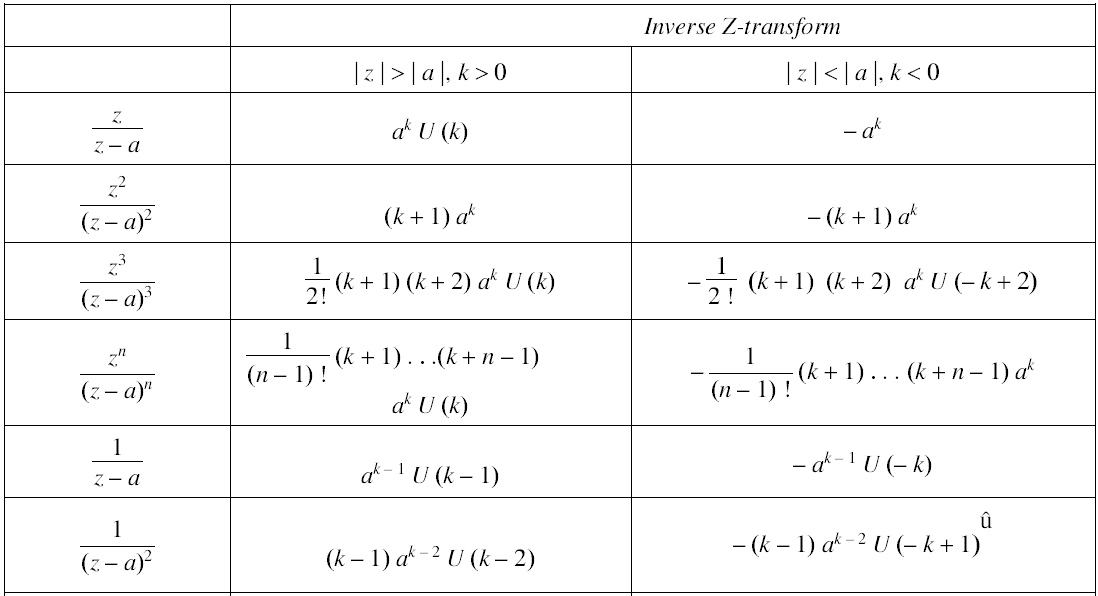
sequence | z-transformation |
| 1 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Example 1:
Find the z-transformation of the following left-sided sequence
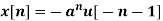
Solution:
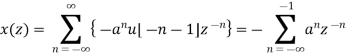
= 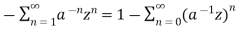
= 1- 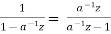
= 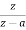
If 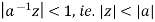
Example 2:
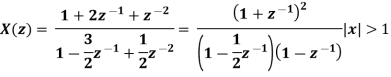
Solution:
Long division method to obtain 
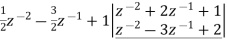 2
2

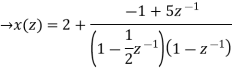
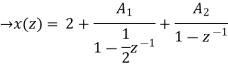
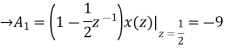
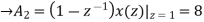
Now x(z) can be written as,
X(z) = 2-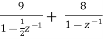
Working procedure to solve the linear difference equation by ZT-
1. Take the ZT of both sides of the differential equations.
2. Transpose all terms without U(z) to the right
3. Divide by the coefficients of U(z)
4. Express this function in terms of the Z-transform of known function and take the inverse ZT of both sides.
This will give 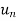 as a function of n, is the required solution.
as a function of n, is the required solution.
Example 1:
Solve the differential equation 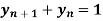 by the z-transformation method.
by the z-transformation method.
Solution:
Given,
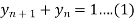
Let y(z) be the z-transform of 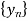
Taking z-transforms of both sides of eq(1) we get,
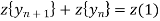
ie. 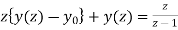
using the given condition, it reduces to
(z+1)y(z) = 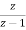
Ie.
Y(z) = 
Or Y(Z) = 
On taking inverse Z-transforms, we obtain

Example 2:
Solve 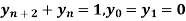 using z-transforms
using z-transforms
Solution:
Consider,
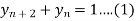
Taking z-transforms on both sides, we get
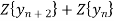 =
=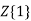
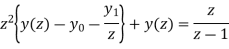
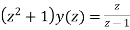 or
or
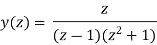
Now,
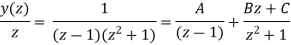
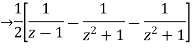
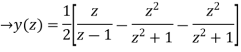
Using inverse z-transform we obtain,
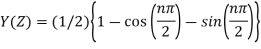
Example-3:
Solve the following by using Z-transform
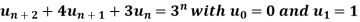
Sol. If 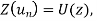 then
then 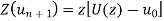
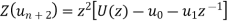
And
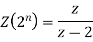
Now taking the Z-transform of both sides, we get
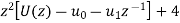 z[
z[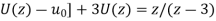
It becomes-

So,
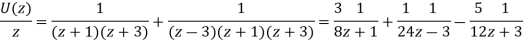
Now-

On inversion, we get-
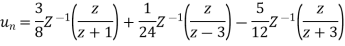
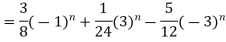
References
1. Erwin Kreyszig, Advanced Engineering Mathematics, 9thEdition, John Wiley & Sons, 2006.
2. N.P. Bali and Manish Goyal, A textbook of Engineering Mathematics, Laxmi Publications.
3. Higher engineering mathematic, Dr. B.S. Grewal, Khanna publishers