undefined | unit 6 optimum design

MSD

UNIT 6

Optimum Design

6.1 Objectives of optimum design

For a design problem a large number of solutions are available which basically fulfil the functional requirement. Optimum design helps to choose the best alternative of the all available keeping a certain target in mind like minimizing weight or maximum strength or minimum cost. Following are the main objectives

To select the best possible option from the available alternatives keeping the target of maximizing a desirable effect or minimizing the undesirable effect

To reach the best solution without doing the repetitive work of detail analysis and evaluation of each alternative

6.2 Adequate design & Optimum design.

Adequate design:

It is the selection of material and values for independent GP’s for a mechanical element. For eg: Shaft in Gear Box

Material selected from list

Diameter is evaluated based on strength and rigidity considerations.

By changing materials number of feasible design can be obtained called as adequate designs.

Optimum Design:

It is the selection of material and values for independent GP’s with the explicit objective of either minimizing the most significant UE or maximizing the most significant FR’s making certain in the design procedure

Objective of minimizing weight keeping the material cost within specific limit.

From number of adequate designs a single design is selected, giving minimum weight with cost within specified limits.

6.3 Design parameters

The design parameter that make the design complete and successful are classified into three groups

1) Functional requirement parameters:The condition required to be satisfied by mechanical element for which the machine/mechanism has to function satisfactorily.

Characteristics

Not always limited to specific value, but have feasible range

Individual FRP’s are dependent or independent on each other

FRP’s include undesirable effect parameter

2) Material Parameters:It describes the material with values.

Characteristics

Values of MP’s are unique

Individual MP’s depend on each other

3) Geometrical parameter:It is defined as the geometry of mechanical element.

Characteristics

It is desirable to select independent GP that uniquely define geometry.

Independent of each other.

6.4 Johnsons Method of Optimum Design.

Johnson's method involves developing three major design equations and solving these equations. These three equations are

1) Primary design equation (PDE): It is most important equation which outlines the most significant functional requirement to be maximized or the most significant undesirable effect which is to be minimized. For example in design of a tensile bar the primary target of minimum weight, then the equation for weight is the PDE.

2) Secondary design equation (SDE) The secondary or subsidiary design equation are the important equation other than the primary design equation. For example in case of tensile bar design the equation of tensile stress is the SDE.

3) Limit Equation (LE): The limit equations are the important design equation that define the satisfactory ranges of certain design parameters. Like the limiting value of stress, or torsional stiffness etc.

Following steps are followed in optimum design using Johnsons Method for normal specifications

Select independent GP(geometrical parameters)

Decide the basis of optimum design (like weight, cost, strength etc.) and Write P.D.E

Write S.D.E (Expressing FR’s +UE’s) other than quantity to be optimized.

Write LE. Indicate loose limit if any

Classify parameters in PDE, SDE and LE’s

Combine S.D.E’s with P.D.E's by eliminating only US and UL parameter.

Find variation of optimum design quantity wrt each independent parameter in developed PDE

Using L.E, determine and substitute the optimum value foe each independent parameter on P.D.E

Write final P.D.E in terms of parameter groups (FRP’s with UE’s + MP’s + GP’s)

Select optimum material from comparison table for various materials

Finally optimum values of geometrical parameters are obtained by solving SDE’s

6.5 Design procedure for helical spring

Diameter of wire:

Shear stress

Wahl’s stress factor

Also refer for k value from Data hand book

Where d = diameter of spring wire ‘generally varies from 4 to 12 for general use

Mean Diameter of Coil

Mean coil diameter D = cd

Outer diameter of coil Do= D d

Inner diameter of coil Di= D - d

Number of coil or turns

Axial Deflection

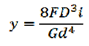
where i = Number of active turns or coils

Free length

Where,

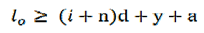
y = Maximum deflection

Clearance ‘a’ = 25% of maximum deflection or a = xdi, for x value refer figure in DHB Assume squared and ground end

∴Number of additional coil n = 2

Stiffness or Rate of spring

Pitch

6.6 Design of Shafts

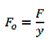
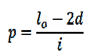
Shafts are designed on the basis of strength or rigidity or both. Design based on strength is to ensure that stress at any location of the shaft does not exceed the material yield stress. Design based on rigidity is to ensure that maximum deflection and maximum twist of the shaft is within the allowable limits.

In designing shafts on the basis of strength, the following cases may be considered:

Shafts subjected to torque

Shafts subjected to bending moment

Shafts subjected to combination of torque and bending moment

Shafts Subjected to Torque

Maximum shear stress developed in a shaft subjected to torque is given by,

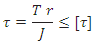
where T = Twisting moment acting upon the shaft,

J = Polar moment of inertia

= for solid shafts

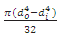= for hollow shafts

r= Distance from neutral axis to the outer most fiber = d/2

Shafts Subjected to Bending Moment

Maximum bending stress developed in a shaft is given by,

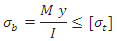
where M = Bending Moment

I = Moment of inertia

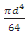= for solid shafts

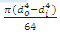= for hollow shafts.

y = Distance from neutral axis to the outer most fiber = d / 2

Shafts Subjected to Combination of Torque and Bending Moment

When the shaft is subjected to combination of torque and bending moment, principal stresses are calculated and then different theories of failure are used. Bending stress and torsional shear stress can be calculated using the above relations.

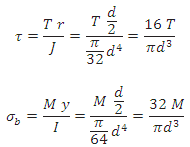
Maximum shear stress is given by,

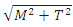is called equivalent torque, such that

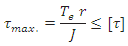
Maximum principal stress is given by,

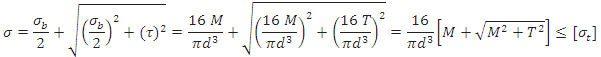
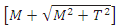is called equivalent bending moment, such that

6.7 Design formulas of pressure vessel

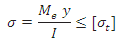
In this section, it is assumed that the cylinder is subjected to only internal pressure. Consider an elemental ring of radius r and radial thickness dr. (sr) and (st) are radial and tangential stresses respectively. Considering the equilibrium of vertical forces

2σtdr + 2(r+dr)(σr+dσr) = 2rσr

Neglecting the term (dr x dσr), the above expression is written as

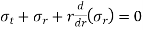(a)

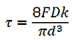
It is further assumed that the axial stress (σ1) is uniformly distributed over the cylinder wall thickness. Therefore, the strain (ε1) isconstant.

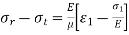(b)

where E is the modulus of elasticity and (m) is Poisson’s ratio. The right-hand side of Eq. (b) is constant and is denoted by (–2Cl ).

σr - σt =–2Cl (c)

Adding Eqs. (a) and(c),

Multiplying both sides of the above equation by (r),

(d)

Since,

(e)

From (d) and (e),

Integrating with respect to r,

or,

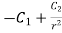= (f)

Substituting the above value in the Eq. (c),

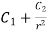= (g)

The constants C1 and C2 are evaluated from the following two boundary conditions:

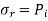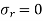when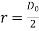

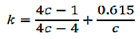
Substituting the constants in Eqs. (f) and (g),

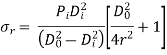
The negative sign is introduced in theexpression for (σr) since it denotes compressivestress.

At the inner surface of the cylinder,

and the stresses are given by,

σr = –Pi

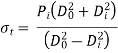
At the outer surface of the cylinder,

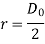
and the stresses are given by

σr = 0

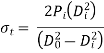
The variation of principal stresses (σr) and (σt) across the cylinder thickness is shown in above fig

The principal stress in axial direction (s1) is assumed to be uniform over the cylinder wall thickness. Considering equilibrium of forces in the axial direction,

6.8 Redundancy

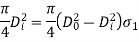
In engineering, redundancy is the duplication of critical components or functions of a system with the intention of increasing reliability of the system, usually in the form of a backup or fail-safe, or to improve actual system performance

The two functions of redundancy are passive redundancy and active redundancy. Both functions prevent performance decline from exceeding specification limits without human intervention using extra capacity.

Passive redundancy uses excess capacity to reduce the impact of component failures. One common form of passive redundancy is the extra strength of cabling and struts used in bridges. This extra strength allows some structural components to fail without bridge collapse. The extra strength used in the design is called the margin of safety.

Eyes and ears provide working examples of passive redundancy.

Active redundancy eliminates performance declines by monitoring the performance of individual devices, and this monitoring is used in voting logic. The voting logic is linked to switching that automatically reconfigures the components. Error detection and correction and the Global Positioning System (GPS) are two examples of active redundancy.

Electrical power distribution provides an example of active redundancy.