UNIT 4
Properties of Pure substances & Thermodynamics of Vapour Cycle
A substance that has a homogeneously fixed chemical composition is called a pure substance.
In thermodynamics, Steam is considered as a pure substance.
So, in this module, we are going to study about the various processes and properties of steam.
Formation of steam

The above diagram shows the phase change occurring when water from and at 0°C is heated till is converted to steam and further to superheat it.
Temperature is plotted as ordinate and Enthalpy as abscissa.
Phase Change
hg = hf + hfg
hsup = hg + Cp (tsup - tsat)
tsup = temperature of superheated steam
tsat = temperature of saturation of steam
Cp = Specific heat of water at constant pressure.
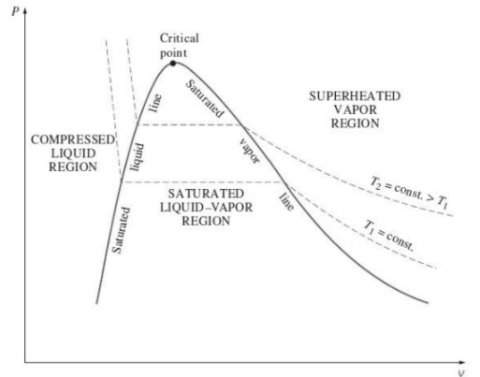
In order to understand the change in properties of water and steam during the phase change process, we have to study the property diagrams.
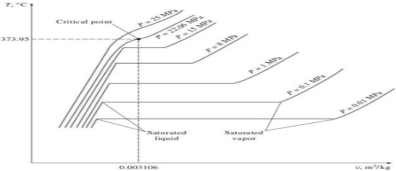
Critical Point
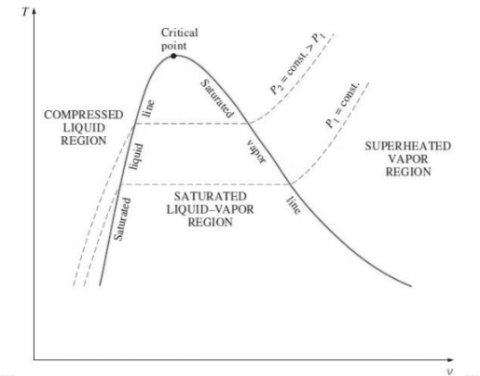
2. P-v diagram for pure substance:
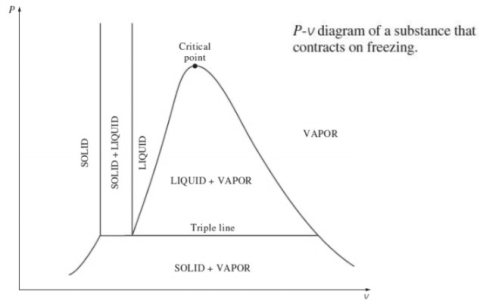
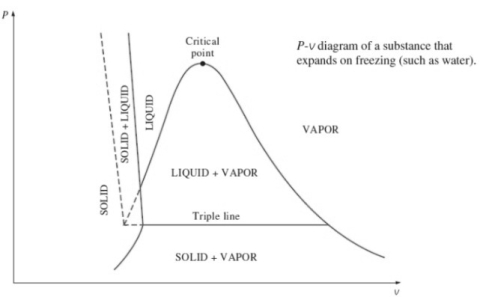
Triple point
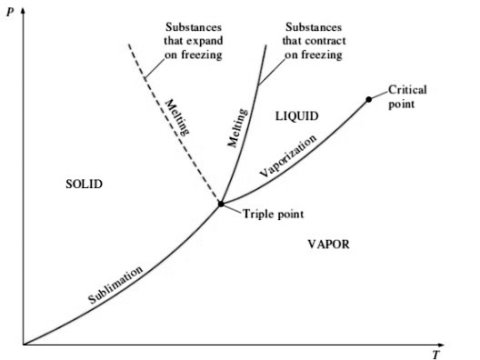
3. P-T diagram for pure substance:
For a wide range, it is very difficult and complex to keep track of various relationships of thermodynamic properties.
Hence, these are tabulated to make it easier for engineers to find various values.
For water, these are called as STEAM TABLES.
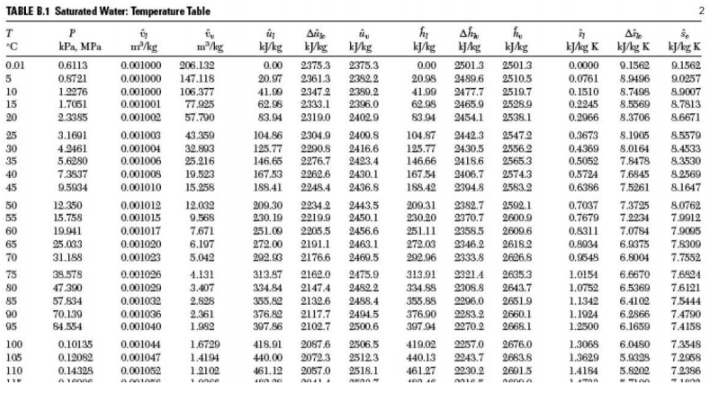
Figure shows the temperature table for saturated water.
There are 3 notations used.
It was prepared by Dr. Mollier. It shows relation between enthalpy and entropy or water at various pressures. It is very useful. The steam tables are actually tabulated version of Mollier diagram.
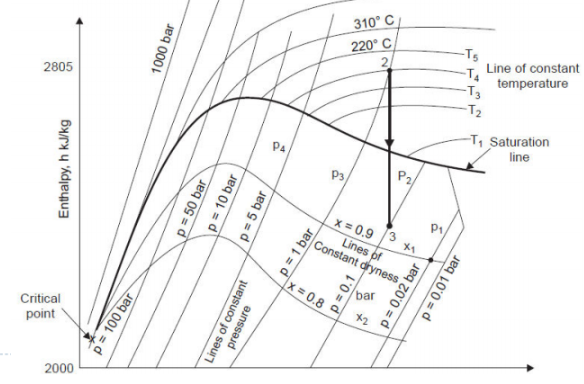
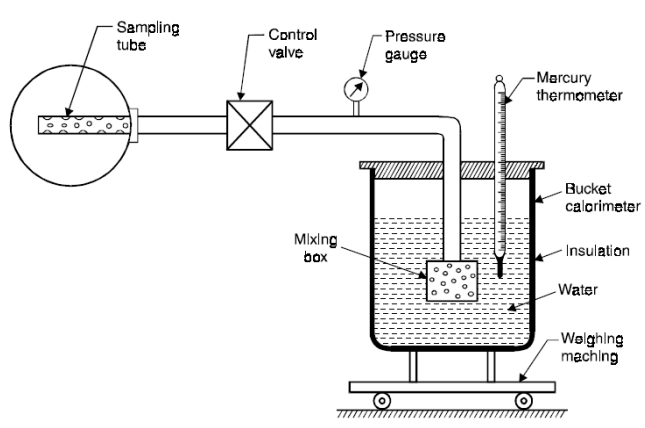
Dryness fraction is defined ratio of mass of dry steam to total mass of mixture of wet steam.
It is denoted by ‘x’.
It is very important property of steam and needs to be determined before the steam is fed to the boiler.
The dryness fraction of steam can be measured by using the following calorimeters:
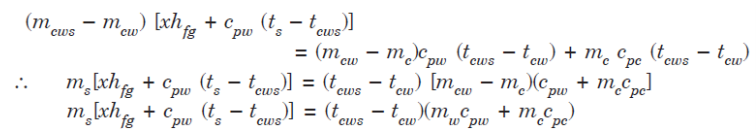
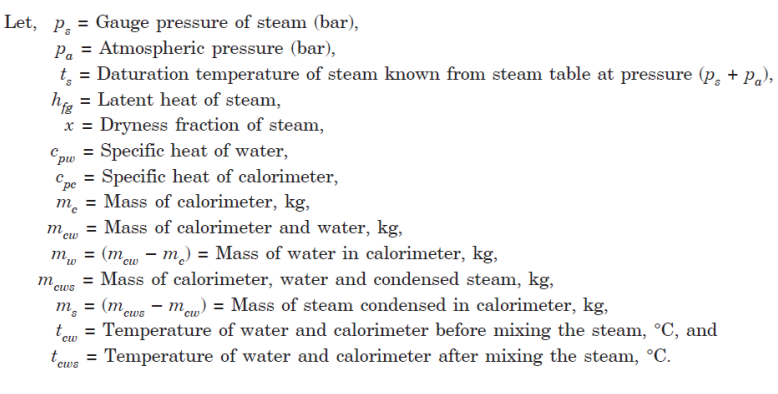
2. Throttling Calorimeter
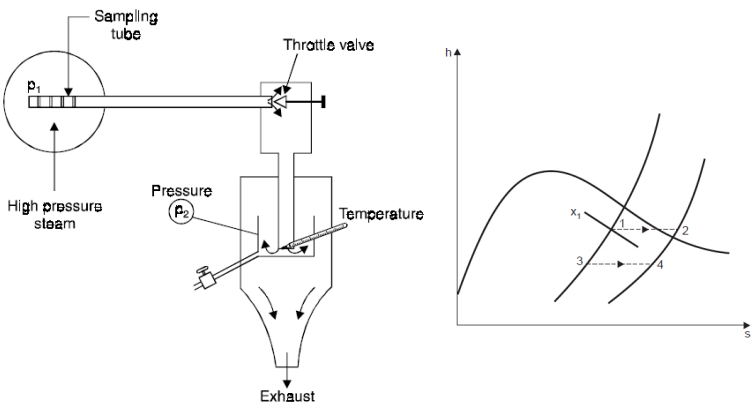
h2 = h1 = hf1 + x1hfg1
x1 = (h2 - hf1) / hfg1
3. Separating and Throttling Calorimeter
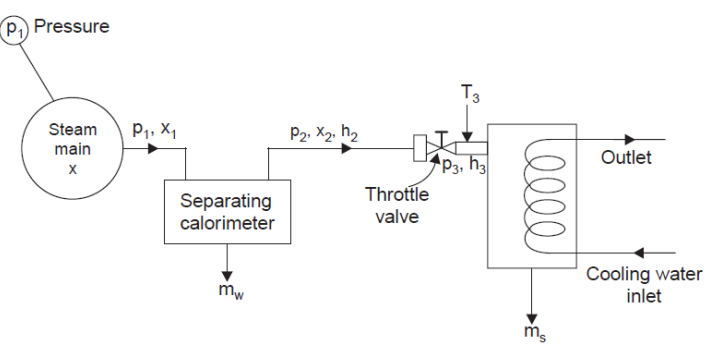
x1 = (mass of dry vapour) / total mass = (x2.ms) / (mw + ms)
h3 = h2 = hf2 + x2.hfg2
x2 = (h3 – hf2) / hfg2
In a previous module, we have seen the limitations of Carnot Cycle.
The actual steam power plants work on Rankine Cycle.
Simple Rankine Cycle:
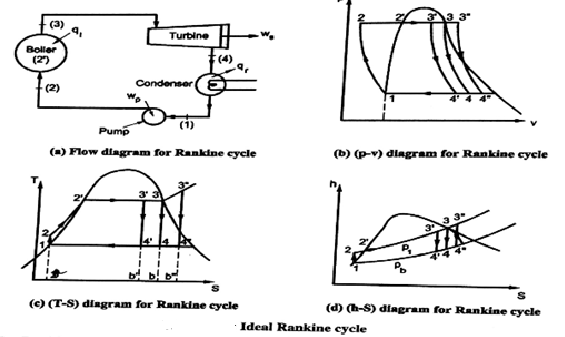
The above diagrams illustrate the various processes of a simple Rankine Cycle applied to a simple steam powered power plant.
Let us the various processes.
Analysis of Rankine Cycle
Assume 1 kg of steam in the cycle and applying SFEE to various processes, we get,
for Process 1-2
Pump work = h2 - h1 = ʃ-V dp
for Process 2-3
Heat supplied in boiler (qi) = h3 - h2
For Process 3-4
Work done in turbine = WT= h3 - h4
For Process 4-1
Heat rejected in condenser (qr) = h4 – h1
Efficiency of Rankine Cycle (η)
Efficiency of Rankine Cycle η = (Shaft work) / (Heat supplied)
= WT – WP / qi
= {(h3 - h4) - (h2 - h1)} / h3 - h2
Methods of improving efficiency of Rankine Cycle
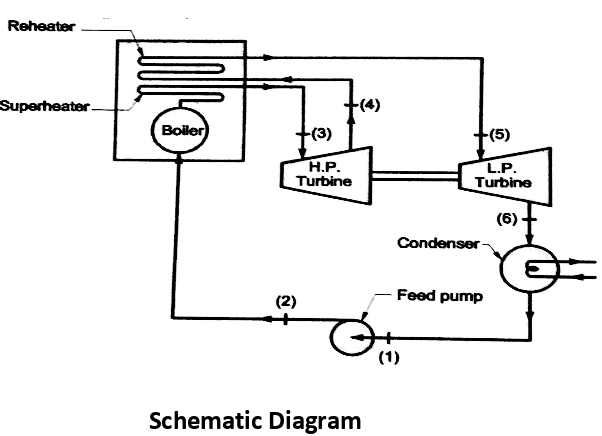
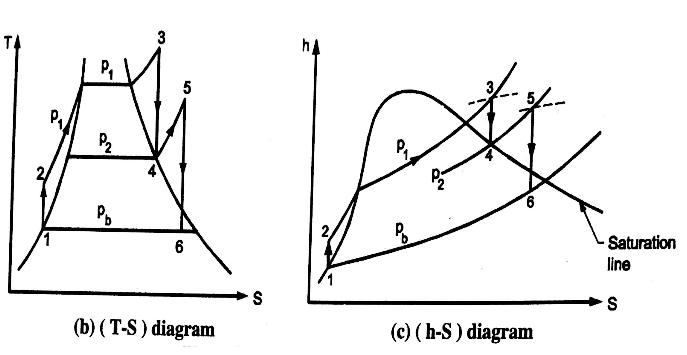
Analysis of Rankine cycle with reheat
Efficiency of reheat cycle for two stage turbines with one reheater in between the stage can be computed as
WT = WT(HP) + WT(LP)
= (h3 – h4) + (h5 – h6)
Wp = (h2 - h1)
Heat Supplied = Heat supplied in boiler and reheater = (h3 - h2) + (h5 - h4)
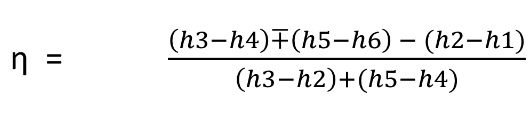
Advantages
Disadvantages