UNIT 3
Kinematic Analysis of Mechanisms: Graphical Method
Figure.3.1 Relative Velocity Method
Now contemplate the body B occupation AN inclined direction as shown in Fig. 3.2 (a). The relative speed of A with regard to B could also be obtained by the law of tetragon of velocities or triangle law of velocities.
• Take any fixed-point o and draw vector oa to represent. Now contemplate the body B occupation AN inclined direction as shown in Fig. 3.2 (a). The relative speed of A with regard to B could also be obtained by the law of tetragon of velocities or triangle law of velocities.
• Take any fixed-point o and draw vector oa to represent vA in magnitude and direction to some appropriate scale. • Similarly, draw vector ob to represent vB in magnitude and direction to a similar scale. Then vector ba represents the relative speed of A with regard to B as shown in Fig. 3.2 (b). within the similar manner as mentioned on top of, the relative speed of A with regard to B, A in magnitude and direction to some appropriate scale.
• Similarly, draw vector ob to represent vB in magnitude and direction to a similar scale. Then vector ba represents the relative speed of A with regard to B as shown in Fig. 3.2 (b). within the similar manner as mentioned on top of, the relative speed of A with regard to B,
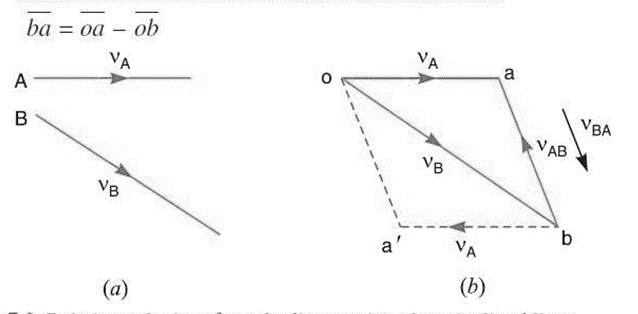
Figure.3.2. Relative Velocities of two bodies Moving along Inclined Lines
• Consider 2 points A and B on a rigid link as shown in Fig.(a).
• Let the purpose B moves with relation to A, with Associate in Nursing angular speed of ω rad/s, and let α rad/s2 be the angular acceleration of the link AB.
• Acceleration of a particle whose speed changes each in magnitude and direction at any instant has the subsequent 2 components:
1. The centripetal or radial part, that is perpendicular to the rate of the particle at the given instant.
2. The tangential part, that is parallel to the rate of the particle at the given instant.
• Thus, for a link AB, the rate of purpose B with relation to A (i.e., VBA) is perpendicular to the link AB as shown in Fig. 8.1 (a).
Since purpose B moves with relation to A with Associate in Nursing angular speed of ω rad/s, so a centripetal or radial part of the acceleration of B with relation to A,
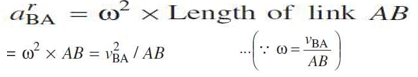
Figure.3.3 Acceleration Analysis
Rest discussion we have done in previous section 2.
Key Takeaways:
Displacement, velocity, and acceleration analysis by relative velocity method, relative velocity of two bodies moving along inclined lines.
The velocity of a link by the instantaneous method can be calculated by the following,
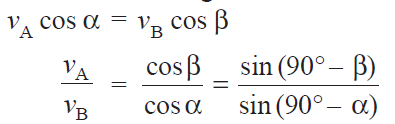
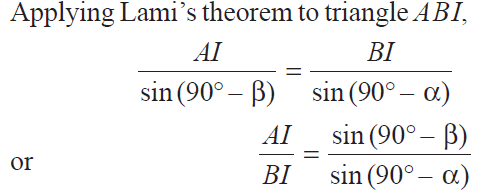
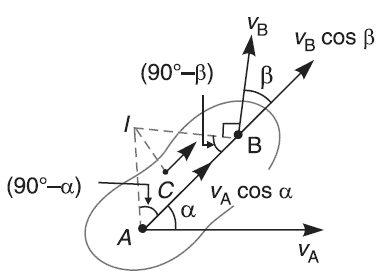
Figure.3.4. Instantaneous Center Velocity
Extension of Rigid Body: The extension of a rigid body refers to the operation of theoretically extending the body to fill all space. By this operation every point in space becomes a point of the body and as a result, has a velocity associated with it. Since this is not an actual extension of the body, a theoretical extension does not influence how the actual body moves-it simply follows the motions of the actual body.
Instantaneous Center of Velocity (ICV): Any point on a rigid body or on its extension that has zero velocity is called the Instantaneous Center of Velocity of the body. Assuming one knows the ICV of a body, one can calculate the velocity of any point A on the body using the equation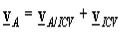 
and recognizing that be definition 
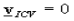. This gives,
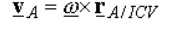
In figure 3.5 we can easily analyze the instantaneous velocity with respect to the angular velocity.
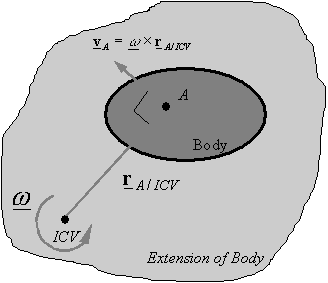
Figure.3.5. Extension of Body
In 2-D motion, if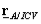 
is in the plane of motion and 
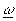is perpendicular to this plane, then one can use the scalar relation.
Methods of finding the ICV: Given the velocity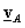 
of point A on a rigid body and the angular velocity of the rigid body one can use the above equation to find the distance 
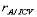between point A and the ICV. One can then draw a line perpendicular to the velocity and passing through A, and move along this line a distance 
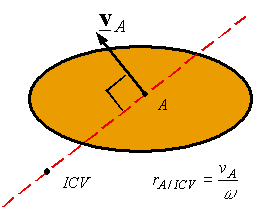to get to the ICV. The side on which the ICV is can be determined by the direction of the angular velocity.
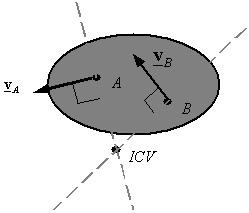
Figure.3.6. ICV Diagram
Given the rate of points A and B on a rigid body one will notice the ICV by drawing a line perpendicular to and spending through A, and by drawing a line perpendicular to and spending through B. one in every of the subsequent 3 cases can result
• The lines run across at one point: the purpose of the intersection is that the ICV. The angular speed may be calculated once the ICV is decided exploitation the rate of either purpose and its corresponding distance from the ICV.
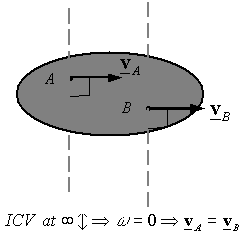
Figure.3.7. ICV Intersection at One Point
The lines are parallel (they intersect at infinity): The ICV is at the time, and also the angular rate is zero since time times zero is that the solely means one will get velocities aside from infinite. Therefore, the body is in pure translation, and also the rate of the 2 points should be constant.
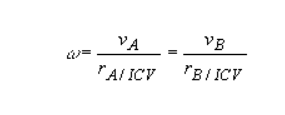
Figure.3.8. Lines are parallel (ICV)
The two lines fall on top of each other: One can find the location of the ICV using the proportionality of velocity and distance from the ICV to create similar triangles. This follows from,
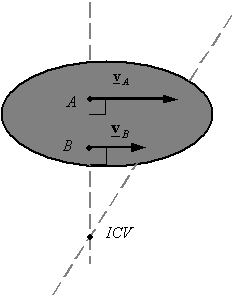
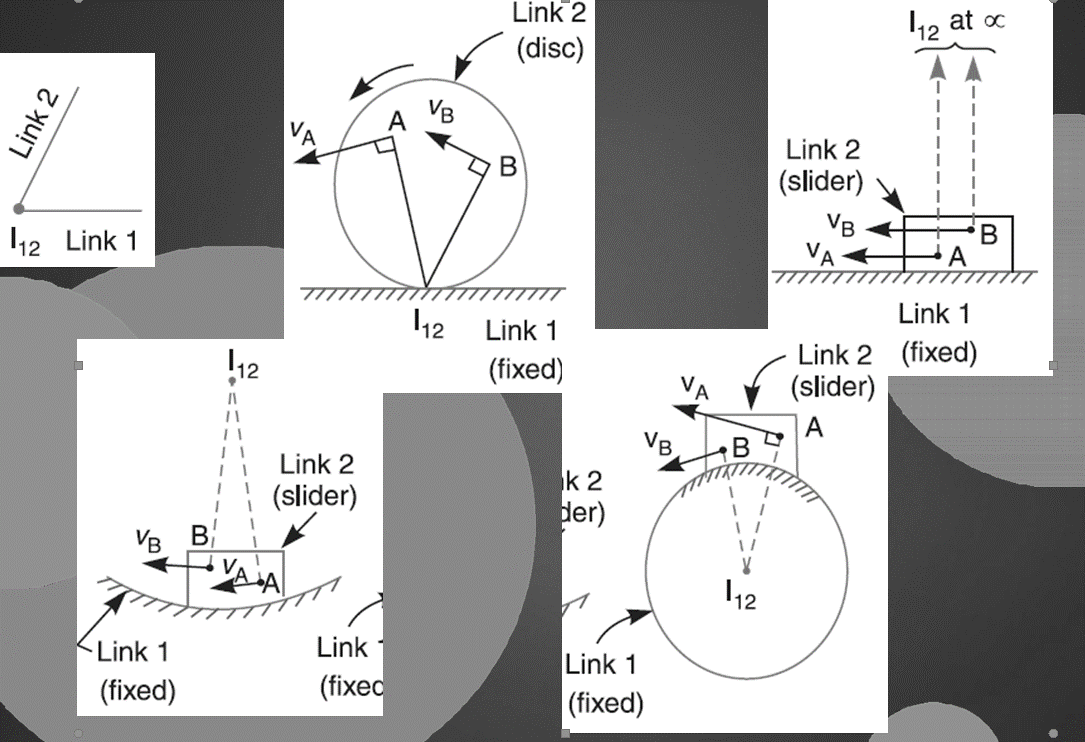
Figure.3.9. Two Lines on Top of Each Other (ICV)
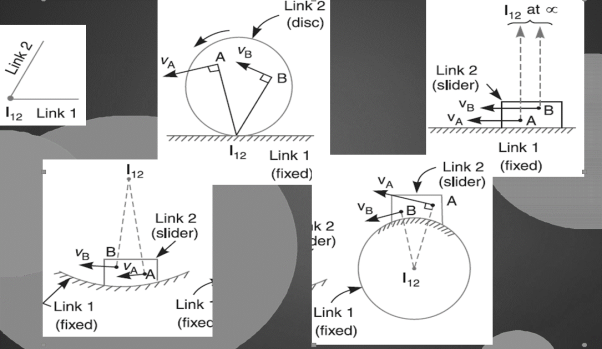
Figure.3.10. Location of Instantaneous Centers for Different Velocity Points
Key Takeaways:
Instantaneous centre of velocity, Extension of Rigid body, Calculation instantaneous centre by relative velocity method, various location of instantaneous centres for different velocity points.
If 3 bodies have plane motion relative to at least one another, then their area unit 3 instant centers, and therefore the 3 instant centers all lie on the identical line. this can be Kennedy’s theorem.
Using Circle Diagrams for Locating Instant Centers
1. Locate (i.e., identify) primary instant centers. E.G., pin connections, slippery bodies, and rolling bodies with no slippy.
2. Draw a circle and mark equally spaced ticks to represent every link within the mechanism.
3. Draw solid lines distinguishing every of the familiar primary instant centers.
4. Draw broken lines between every purpose (i.e., link) to represent the remaining instant centers to be found.
5. Apply Kennedy's theorem to search out the unknown instant centers.
« Back
EXAMPLE: Four-bar Linkage
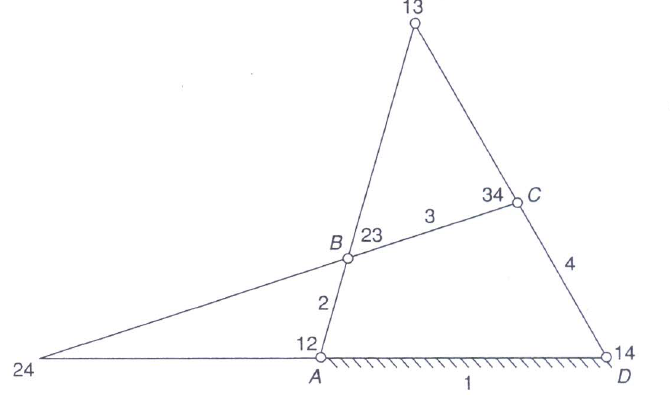
Figure.3.11. Four bar Linkage and Circle Diagram
Key Takeaways:
Kennedy’s theorem, basic understanding, Four bar linkage, and circle diagram.
When the angular rate of a link is understood and it's needed to search out the angular rate of another link, find their common I-center. the speed of this I-center relative to a hard and fast third link is that the same whether or not the I-center is taken into account on the primary or the second link. First, contemplate the I-center to be thought of on the primary link and procure the speed on the I-center. Then contemplate the I-center to air the second link and realize its angular rate. as an example, if it's needed to search out the angular rate of link four once the angular rate of link two is understood of a four-link mechanism is understood, find the I-center 24. Imagine link two to be within the kind of a flat disc containing purpose 24 and revolving regarding twelve or A.
Then
V24= w2 (24-12)
Now imagine link 4 to be large enough to contain point 24 and revolving about 14 or D. Then
V24= w4 (24-14)
or
W4=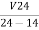 
or
= 
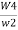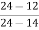
The above equation is known as the angular velocity theorem. In other words, the angular velocity ratio of two links relative to a third link is inversely proportional to the distance of their common I-center from their respective center of rotation. In the above case point, 12 and 14 lie on the same side of 24 on lines 24-14, and the direction of rotation of the two links (2 and 4) is the same i.e clockwise or counterclockwise. Had they been on the opposite side of the common I-center the direction would have been opposite.
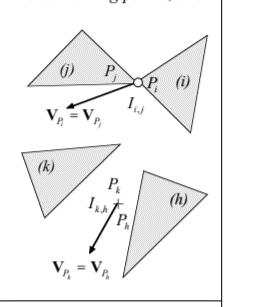
Figure.3.12. Instantaneous center of six Link
Key Takeaways:
Angular velocity ratio theorem, Instantaneous centre of six links.
The instant center of velocities may be a straightforward graphical methodology for activity speed analysis on mechanisms. the strategy provides a visual understanding of however speed vectors are connected. Tools: ruler, right angle, protractor. The instant center of velocities between 2 links is that the location at those 2 coinciding points, one on every link, has identical velocities. The most obvious instant center of velocities or just the moment center (IC), between 2 links that are fastened to every alternative is that the purpose at the center of the pin joint. for instance, the center of the pin joint between links i and j is viewed as 2 coinciding points, Pi on link i and Pj on link j, that have a similar velocity. the moment center between these 2 links is denoted as ii,j or ii,j . the moment center of velocities might not be settled at intervals the physical boundaries of a link. As shown within the second figure, the IC between links k and h, ik,h, is found on imagined extensions of each link.
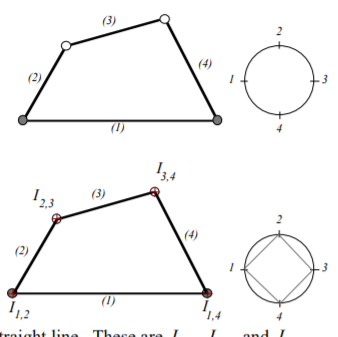
Figure.3.13. Instantaneous Center
Instant Centers of a Four-bar
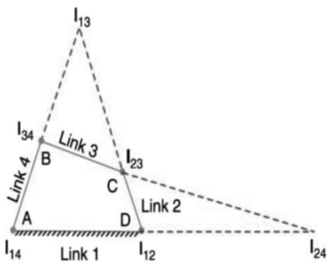
A four-bar mechanism has six instant centers despite the scale or orientation of the links. For clerking functions in locating the IC’s, we tend to draw a circle and place link indices on the circle in any desired order. This clerking procedure might not be necessary for a four-bar, however becomes helpful once mechanisms with a larger variety of links area unit thought-about. (1) (2) (3) (4) one a pair of three-four Since pin joints area unit instant centers, for a four-bar with four-pin joints, four IC’s area unit right away known. every found IC is marked on the circle as a line drawn between the 2 corresponding link indices. These four IC’s area units actual (not imaginary) pin joints. to seek out the opposite IC’s, we tend to apply Kennedy’s rule. The IC’s between links a pair of, three and four should lie on a line. This area unit I2,3, I3,4, and I2,4. Since we tend to have already got I2,3 and I3,4, we tend to draw a line through them; I2,4 should even be on this line. The IC’s between links one, a pair of and four should lie on a line. This area unit I1,2, I1,4, and I2,4. Since we tend to have already got I1,2 and I1,4, we tend to draw a line through them; I2,4 should even be on this line. The intersection of those 2 lines is I2,4.
Figure.3.14. ICR for Four Bar
The number of instantaneous centers in a constrained kinematic chain is equal to the number of possible combinations of two links.
N=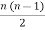 
Types of Instantaneous centers are:
1) Fixed instantaneous centers
2) Permanent instantaneous centers
3) Neither fixed nor permanent instantaneous centers
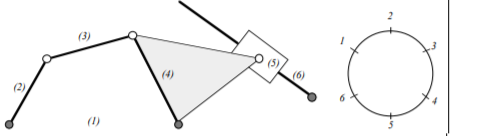
Figure.3.15. Four bar Mechanism
The above figure shows a fixed instantaneous center for a four-bar mechanism
Instant Centers of a Six-bar
In this example, we consider a six-bar mechanism containing a four-bar and an inverted slider-crank that share one link and one pin joint. A circle is constructed with link indices 1 – 6. We first find the six IC’s that belong to the four-bar.
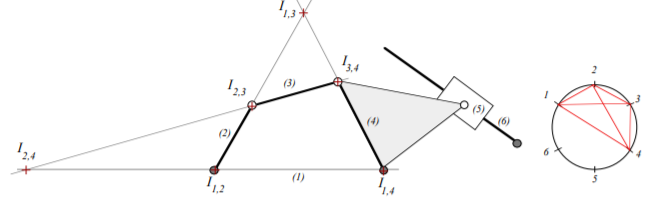
Figure.3.16 Six Link Bar
We first find the six IC’s that belong to the four-bar.
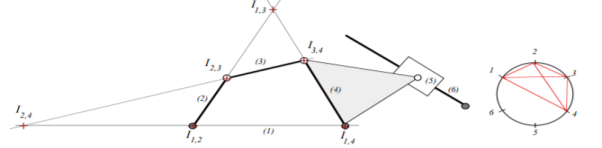
Figure.3.17 Analysis of Six Link Mechanism
Key Takeaways:
Analysis of mechanism by ICR method, Instant centre of four-bar, Instant centres of six-bar mechanism, analysis of six link mechanism.
Coriolis acceleration is generated by the eastward rotation of the earth around the N-S axis.
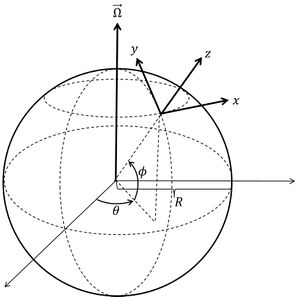
Figure. 3.18: Definition of x, y, z axes on the rotating earth.
This acceleration will be thought of as a strictly kinematic impact by noting that point derivation in an exceedingly rotating frame introduces a term associated with rotation of the axes of reference [1]:
(dr⃗ /dt) mounted +Ω⃗ mounted, where wherever indicates the position of a fluid parcel on the rotating earth (r⃗ =0 at the earth's center), u⃗ ≡dr⃗ /dt is that the rate on the rotating earth, Ω⃗ the rotation vector on the N-S rotation axis. The radial earth rotation frequency Ω≈7.292110−5s−1. The acceleration then follows from (d2r⃗ /dt2) mounted / dt+Ω⃗ mounted +Ω⃗ mounted +Ω⃗ × (Ω⃗ ×r⃗). The term du⃗ /dt is that the acceleration on the rotating earth, 2Ω⃗ ×u⃗ is that the Coriolis acceleration and also the and also the × (Ω⃗ ×r⃗) is the part of the force salaried by earth's attraction and adjustment of the equilibrium ocean surface slope. If no forces area unit functioning on the fluid and within the absence of friction, (d2r⃗ /dt2) mounted frame=0. In order to seek out a lot of usual formulas for the Coriolis acceleration we tend to use a system of axes on the rotating earth as indicated in figure one, wherever is that the angle indicating meridian (expressed in radians), ϕ the elevation angular unit angle indicating latitude and R is that the earth radius. during this system the vector parts area unit u⃗ = (u=R cosϕ dθ/dt, v=R dϕ /dt,0), Ω⃗ = (0, Ω cosϕ, Ω sinϕ). The Coriolis acceleration is then given by: du/dt=fv, dv/dt=−fu, f=2Ωsinϕ. The Coriolis acceleration will be derived in an exceedingly a lot of intuitive means if we tend to think about fluid velocities (u, v) a lot smaller than the Earth's surface rotation rate U=R Ω cosϕ. because of the rotation of the planet, a force, perpendicular to the earth's rotation axis, acts on ocean waters. This force is just partially salaried by earth's attraction; it's a part acting on the surface of the planet towards the equator, see figure a pair of.
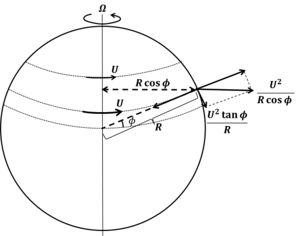
Figure.3.19.: The tangential component of the centrifugal force.
If water is at rest this tangential component of the centrifugal force is balanced by a sea surface slope. When seawater is brought into motion, this equilibrium is broken and water motion experiences Coriolis acceleration. A current u in east-direction will experience an acceleration toward the equator as a result of the increased tangential component of the centrifugal force:
dv/dt=−sinϕ[(U+u)2−U2]/Rcosϕ≈−fu with f=2Ωsinϕ.
The approximation is very accurate because in natural situations |u|<<|U|.
When fluid is traveling northward along the earth's surface with velocity v=R dϕ/dt, it experiences a decrease of surface rotation velocity U. The principle of angular momentum conservation implies that the fluid will accelerate to conserve its angular momentum. This can be expressed as
d[(U+u) Rcosϕ]/dt=d[ΩR2cos2ϕ+uRcosϕ]/dt=0.
From this equation we find du/dt=fv, assuming again that |u|<<|U|.
When fluid parcels square measure set in motion, they, therefore, expertise associate degree acceleration to the correct on the hemisphere (ϕ>0) associate degreed an acceleration to the left on the hemisphere. as a result of f is tiny (typically on the order of 10-4), the earth's rotation hardly influences currents that fluctuate with higher frequencies, like wave revolution. However, the influence on stationary currents or slowly varied currents, like the wind-driven flow and recurrent event motion, is important (see Coriolis and recurrent event motion in shelf seas). this is often the case in wide basins, with a dimension of the order or larger than |u⃗ +v⃗ |/f. A fluid moving freely (no external or resistance forces) in a very wide basin (no water surface inclination), with initial speed V, can describe a circular motion thanks to Coriolis acceleration: u=V sinft, v=V cosft. The fluid follows an associate degree mechanical phenomenon circle with radius V/f. This flow pattern is typically discovered once a robust watercourse jet enters a good basin.
Key Takeaways:
Coriolis component of acceleration, understanding of concepts and different velocity formula.
Reference Books:
2. Bevan T, “Theory of Machines”, Third Edition, Longman Publication |
|
3. Paul E. Sandin, “Robot Mechanisms and Mechanical Devices Illustrated”, Tata McGraw Hill Publication
|
4. Ghosh Malik, “Theory of Mechanism and Machines”, East-West Pvt. Ltd.
|
5. M.P. Groover, “Automation, production systems and computer-integrated manufacturing”, Prentice-Hall of India Pvt. Ltd
