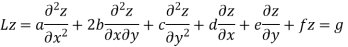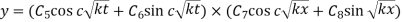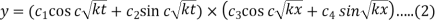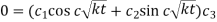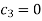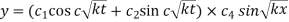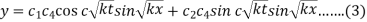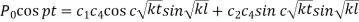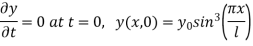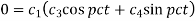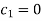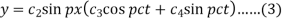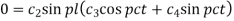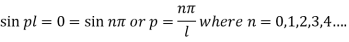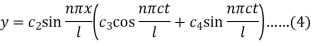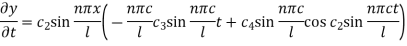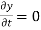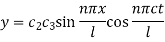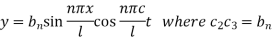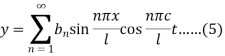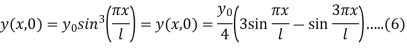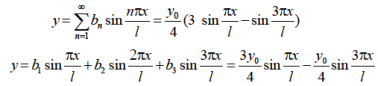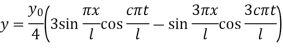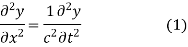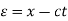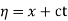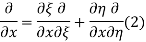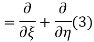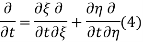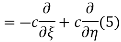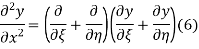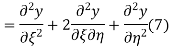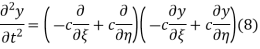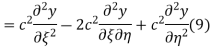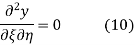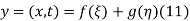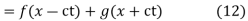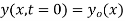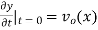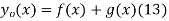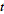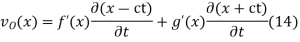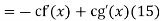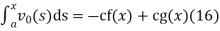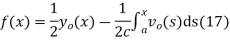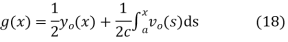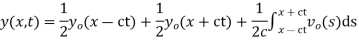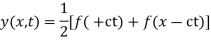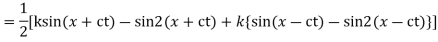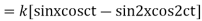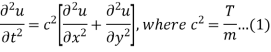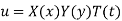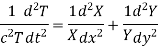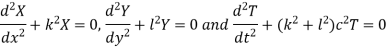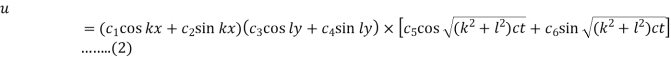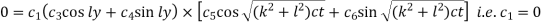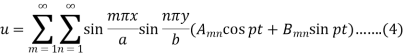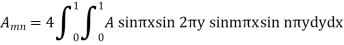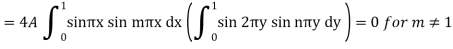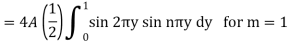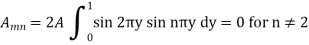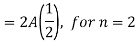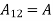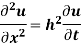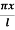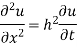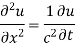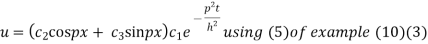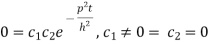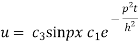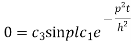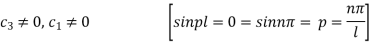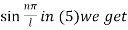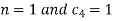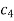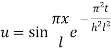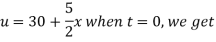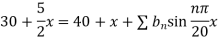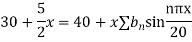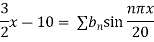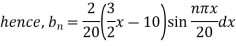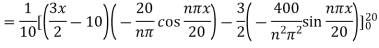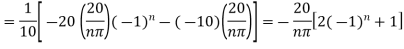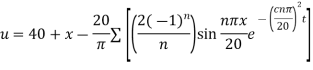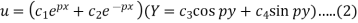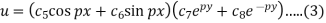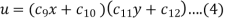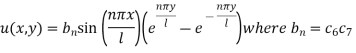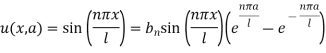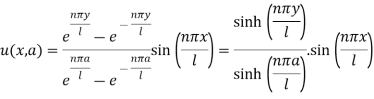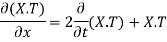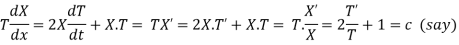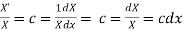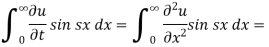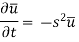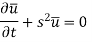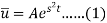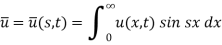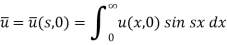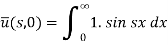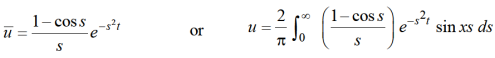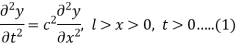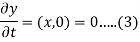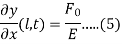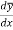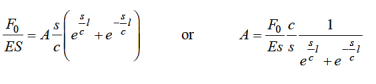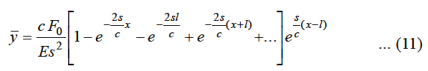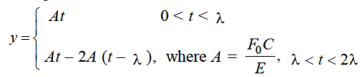Unit-6
Applications of partial differential equations (PDE)
The second order PDE in two independent variables of the form
|
Here a, b, c, d, e, f and g are the functions of the independent variables x and y.
The principal part of the operator L, can be given as-
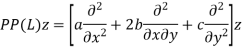
The equation is classified as-
Hyperbolic if- 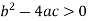
Parabolic if- 
Elliptic if- 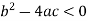
Here 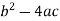 is the discriminant of the operator L.
is the discriminant of the operator L.
Example: Classify the following PDEs into hyperbolic, parabolic or elliptic.
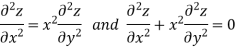
Sol. In the first PDE, a = 1, b = 0 and c = 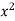
So that-
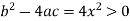
Thus, we can say that the given PDE is hyperbolic.
Now in second PDE,
A = 1, b = 0 and c = 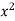
So that-
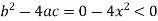
Therefore, the second PDE is elliptic.
Modeling of vibrating string-
Suppose a elastic string of length ‘l’ is stretched between two points O and T.
Let the string is released from rest and allowed to vibrate, perpendicular to its length.
|
Let the displacement at the point P(x, y) is ‘y’ at any time, then the wave equation-
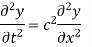
We get the solution of the wave equation
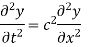
By using the method of separation of variables-
Here we have-

Suppose y = XT where X is a function of x only and T is the function of t.
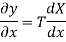
Since T and X are functions of a singe variable only.
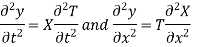
Put these values in the equations given above-
We get-
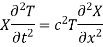
On separating the variables, we get-
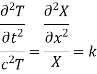
Here the variables x and y are independent, each side is constant-
So that-
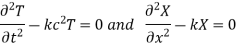
Here auxiliary equations are-
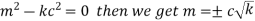
And
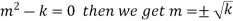
Situation-1: if k>0 then-
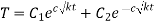
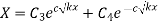
Situation-2: when k<0 then-
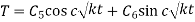
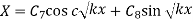
Situation-3: when k = 0, then-
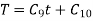
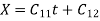
Here we are dealing with the wave motion (k<0).
y = TX
|
Example: Find the solution of the wave equation-
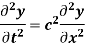
In such a way that 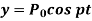 where
where 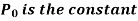 when x = l and y = 0 when x = 0.
when x = l and y = 0 when x = 0.
Sol.
We have-
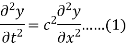
Here the solution of the wave equation is-
Put y = 0, when x = 0
|
Equation (2) becomes-
Put y =
|
Now equating the coefficient of sin and cos on both sides-
We get-
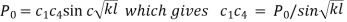
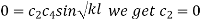
And p = 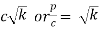
Equation (3) becomes-
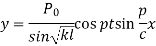

Example: A tightly stretched string with points x = 0 and x = l is initially in a position given by 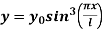 .
.
Suppose it is released from rest from this position then find out the displacement y(x,t)
Sol.
Let the equation of the vibrating string be-
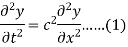
The initial conditions are-
y(0,t) = 0 and y(l, t) = 0
The solution of the equation (1) is-
Now y(0, t) = 0 gives-
Therefore (2) becomes-
And y(l,t) gives-
So that-
Put the value of ‘p’ in equation (3), we get-
|
Then-
|
Since
Then equation (4) turns to-
|
But it is given that-
|
On putting t = 0 in equation (5) , we have-
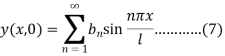
Now equation (6) and (7), we get-
|
Comparing the coefficients-

Here equation (5) reduces to-
|
D'Alembert's solution of the wave equation The method of d'Alembert provides a solution to the one-dimensional wave equation
that models vibrations of a string. The general solution can be obtained by introducing new variables
Using (4) and (5) to compute the left and right sides of (3) then gives
respectively, so plugging in and expanding then gives
This partial differential equation has general solution
where f and g are arbitrary functions, with f representing a right-traveling wave and g a left-traveling wave. The initial value problem for a string located at position
Taking the derivative with respect to
and integrating gives
Solving (13) and (16) simultaneously for f and g immediately gives
so plugging these into (13) then gives the solution to the wave equation with specified initial conditions as
|
Example. Find the deflection of a vibrating string of unit length having fixed ends with initial velocity zero and initial deflection f (x)=k (sinx –sin2x) Solution. By d’Alembert’s method, the solution is
i.e., the given boundary corrections are satisfied. Wave equation in two dimensions- The equation
Is the wave equation in two dimensions. Solution of wave equation in two dimensions (rectangular membrane)- Let us assume that the solution of the above equation (1) is of the form-
Put these values in the equation and dividing by XYT, we get-
|
If each member is a constant then this can hold good.
Now choosing the constants suitably, we get-
|
Hence the solution of equation (1) is-
|
Now let the membrane is rectangular and it is stretched between the lines x = 0, x = a, y = 0, y = b.
Then the condition u = 0 when x = 0 gives-
|
Then putting 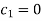 in (2) and applying the condition u = 0, when x = a,we get-
in (2) and applying the condition u = 0, when x = a,we get-
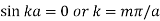
Now applying the conditions u = , when y = 0 and y = b, we get
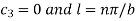
Therefore, the solution (2) becomes-
|
Where 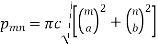
Choosing the constant 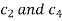 so that
so that 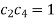
We can write the general solution of the equation-1 as-
|
If the membrane starts from rest from the initial position u = f(x,y)
Which means-
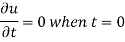
Then equation 3 gives-

Also using the condition u = f(x, y) when t = 0, we get-
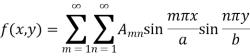
Which is the double Fourier series.
Now multiply the both sides by  and integrating from x = 0 to x = a and y = 0 to y = b,
and integrating from x = 0 to x = a and y = 0 to y = b,
Every term on the right except one, become 0, therefore we get-
|
This is called the generalized Euler’s formula.
Example: Find the deflection u(x,y,t) of the square membrane with a = b = 1 and c = 1. If the initial velocity is zero and the initial deflection is f(x, y) = 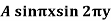 .
.
Sol.
Here taking a = b = 1 and f(x, y) = 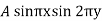 in equation above (5)-
in equation above (5)-
We get-
|
Also from equation (3) above-
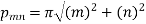
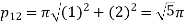
Therefore from equation (4),
The solution will be-
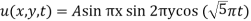
One dimensional heat flow
Suppose heat flow along a bar of uniform cross section in the direction perpendicular to the cross section. Take one end of the bar as origin and the direction of the heat flow is along x axis.
Let the temperature of the bar at any time t at a point x distance from the origin be u(x,t). Then the equation of one-dimensional heat flow is
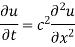
Example. A rod of length 1 with insulated sides is initially at a uniform temperature u. Its ends are suddenly cooled to 0° Celsius and are kept at that temperature. Prove that the temperature function u (x, t) is given by
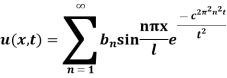
Where 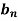 is determined from the equation.
is determined from the equation.
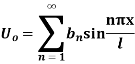
Solution. Let the equation for the conduction of heat be
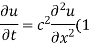
Let us assume that u = XT, where X is a function of x alone and T that of t alone.
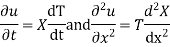
Substituting these values in (1) we get 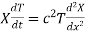
i.e. 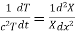
Let each side be equal to a constant 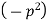
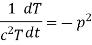
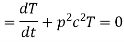
And 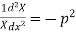
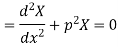
Solving (3) and (4) we have
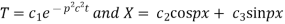
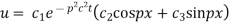
Putting x = 0, u = 0 in (5), we get
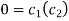

(5) becomes 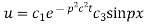
Again putting x = l, u =0 in (6), we get
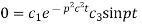
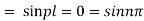
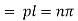
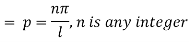
Hence (6) becomes |
This equation satisfies the given conditions for all integral values n. Hence taking n = 1, 2, 3,…, the most general solution is
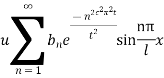
By initial conditions 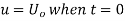
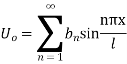
Example 2. Find the solution of
For which u (0, t) = u (l.t) =0 =sin Solution.
In example 10 the given equation was
On comparing (1) and (2) we get Thus solution of (1) is
On putting x =0 u =0 in (3) we get
(3) reduced to
On putting x = l and u =0 in (4) we get
Now (4) is reduced to
On putting t = 0, u =
This equation will be satisfied if
On putting the values of
|
Example 3. The ends A and B of a rod 20 cm long having the temperature at 30 degree Celsius and at 80 degree Celsius until steady state prevails. The temperature of the ends are changed to 40 degree Celsius and 60 degree Celsius respectively. Find the temperature distribution in the rod at time t.
Solution. The initial temperature distribution in the rod is
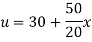
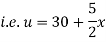
And the final distribution (i.e. steady state) is
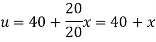
To get u in the intermediate period, reckoning time from the instant when the end temperature were changed we assumed
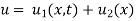
Where 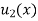 is the steady state temperature distribution in the rod (i.e. temperature after a sufficiently long time) and
is the steady state temperature distribution in the rod (i.e. temperature after a sufficiently long time) and 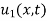 is the transient temperature distribution which tends to zero as t increases.
is the transient temperature distribution which tends to zero as t increases.
Thus, 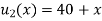
Now  satisfies the one-dimensional heat flow equation
satisfies the one-dimensional heat flow equation

Hence u is of the form
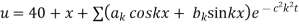
Since 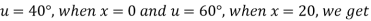
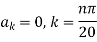
Hence 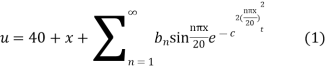
Using the initial condition i.e.
|
Putting this value of 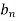 n (1), we get
n (1), we get
|
Two-dimensional heat flow-
Let us consider the heat flow in a metal plate of uniform thickness, in the direction parallel to length and breadth of the plate.
Let u(x, y) be the temperature at any point (x, y) of the plate at time t is given as-
|
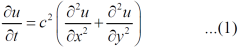
In the steady state, u doesn’t change with t,

Equation (1) becomes-
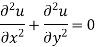
Which is known as Laplace’s equation in two dimensions.
Example:
Laplace equation in two dimensions
The equation-
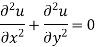
Is known as the Laplace’s equation in two dimensions.
Solution of Laplace’s equation-
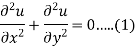
Let
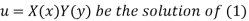
Put the value in (1), we get-
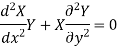
Separating the variables-
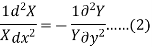
Since x and y are the independent variables, equation (2) can hold good only if each side of (2) is equal to a constant (k),
Then (2) leads the ordinary differential equation-
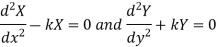
On solving these equations, we get-
- When k is positive and it is equals to
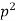 , say
, say
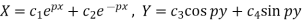
2. When k is negative and it is equals to 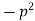 , say
, say
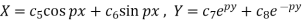
3. When k is zero-
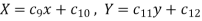
Example: Solve the Laplace’s equation 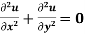 subject to the conditions u(0, y) = u(l, y) = u(x, 0) = 0 and u(x, a) = sin n
subject to the conditions u(0, y) = u(l, y) = u(x, 0) = 0 and u(x, a) = sin n
Sol.
The three possible solutions of Laplace’s equation-
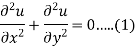
are-
|
We need to solve equation (1) satisfying the following boundary conditions-
u(0, y) ........... (5)
u(l, y) = 0........(6)
u(x, 0) = 0 ..........(7)
and u(x, a) = sin n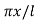 ...... (8)
...... (8)
using (5), (6) and (2), we get-
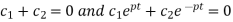
Solving these equations, we get-
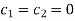
Which leads to trivial solution.
Similarly we get a trivial solution by using (5), (6) and (4).
Hence the solution for the present problem is solution (3).
Now using (5) in (3), we get-
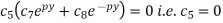
Therefore, equation (3) becomes-
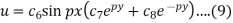
Using (6), we get-
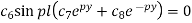
Therefore either-
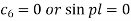
If we take 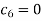 then we get a trivial solution.
then we get a trivial solution.
Thus sin pl = 0 whence 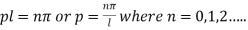
Equation (9) becomes-
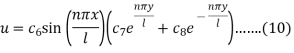
Using (6), we have 0 = 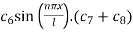
i.e.
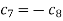
Thus the solution suitable for this problem is-
Now using the condition (8)-
|
We get-
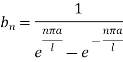
Hence the required solution is-
|
Method of separation of variables
In this method, we assume that the dependent variable is the product of two functions, each of which involves only one of the independent variables. So to ordinary differential equations are formed.
Example: Using the method of separation of variables, solve
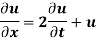
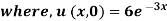
Solution. 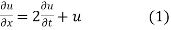
Let, u = X(x). T (t). (2)
Where X is a function of x only and T is a function of t only.
Putting the value of u in (1), we get
(a) |
On integration log X = cx + log a = log 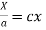
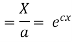
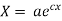
(b) 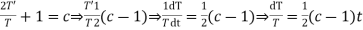
On integration 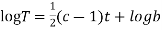
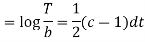
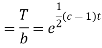
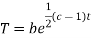
Putting the value of X and T in (2) we have
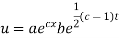
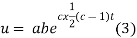
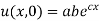
But, 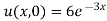
i.e. 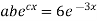
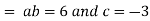
Putting the value of a b and c in (3) we have
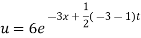
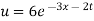
Which is the required solution.
Example. Use the method of separation of variables to solve equation
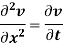
Given that v = 0 when t→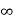 as well as v =0 at x = 0 and x = 1.
as well as v =0 at x = 0 and x = 1.
Solution. 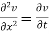
Let us assume that v = XT where X is a function of x only and T that of t only
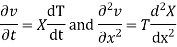
Substituting these values in (1), we get
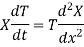
Let each side of (2) equal to a constant 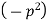
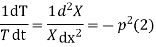
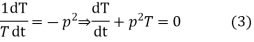
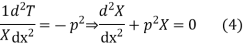
Solving (3) and (4) we have
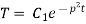
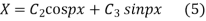
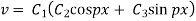
Putting x = 0, v = 0 in (5) we get

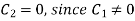
On putting the value of 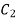 in (5) we get
in (5) we get
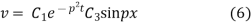
Again putting x = l, v= 0 in (6) we get
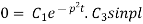
Since 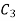 cannot be zero.
cannot be zero.
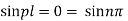
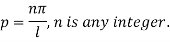
Inputting the value of p in (6) it becomes
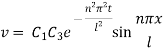
Hence, 
This equation satisfies the given condition for all integral values of n. Hence taking n = 1, 2, 3,… the most general solution is
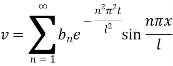
Example. Using the method of separation of variables, solve 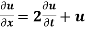 Where
Where 
Solution. Assume the given solution 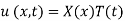
Substituting in the given equation, we have
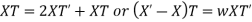
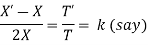
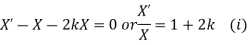
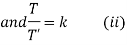
Solving (i) 
From (ii) 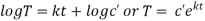
Thus 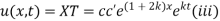
Now, 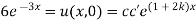
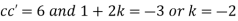
Substituting these values in (iii) we get
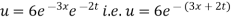
Which is the required solution
Example: Solve the equation-
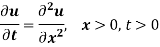
Subject to the conditions-
- u = 0 when x = 0, t>0
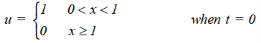
- U(x,t) is bounded.
Sol.
Here we apply Fourier sine transform-
We get-
|
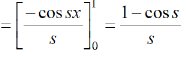 ...... (2)
...... (2)
Put the value of 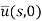 in equation (1), we get
in equation (1), we get
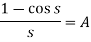
So that-
|
Wave equation-
The wave equations is given as-
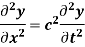
The solution of wave equation by Laplace transform-
Example: A uniform rod whose length is ‘l’ at rest in its equilibrium position with the end x = 0 fixed. At t = 0, a constant force Find the motion of the rod for t>0. Sol. Suppose the displacement in the rod is y(x,t). The equation of motion is given by-
Subject to the conditions- y(x,0) = 0 ..... (2)
y(0,t) = 0 ...... (4)
Here E is the Young’s modulus. Now applying Laplace transform on (1),we get-
We know that equation (6) is an ordinary differential equation and its solution is-
Put y = 0 and x = 0 from (2) in (7),we get- 0 = A + B B = -A Then (7) becomes-
Laplace transform of (3) is-
Differentiating equation (8) w.r. t x-
Put the value of
|
Put the value of A in (8),we get-
|
Putting x = l in (11),
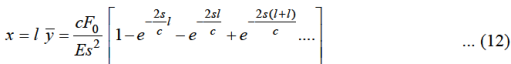
Now applying inversion transform on (12),we get-
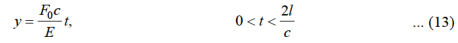
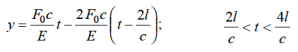
Putting 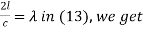
|
Which is the required answer.
References
1. Erwin Kreyszig, Advanced Engineering Mathematics, 9thEdition, John Wiley & Sons, 2006.
2. N.P. Bali and Manish Goyal, A textbook of Engineering Mathematics, Laxmi Publications.
3. Higher engineering mathematic, Dr. B.S. Grewal, Khanna publishers