Unit - 5

Expansion of Functions and Indeterminate forms

5.1 Maclaurin's theorem

Maclaurin’s Series Expansions

Statement:-

Maclaurin’s series of f(x) at x = 0 is given by,

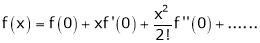
Expansion of some standard functions

i)      f(x) = ex then

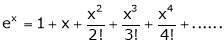
Proof:-

Here

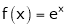
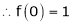
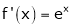
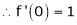
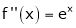
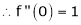
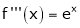
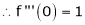
By Maclaurin’s series we get,

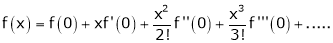
i.e.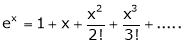

Note that

1. Replace x by –x we get

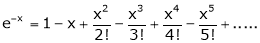
2.     f(x) = sin x then

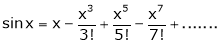
Proof:

Let (x) = sin x

Then by Maclaurin’s series,

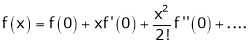    … (1)

Since

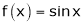
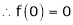
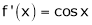
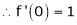
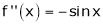
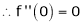
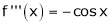
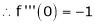
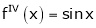
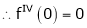
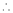By equation (i) we get,

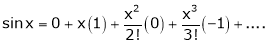
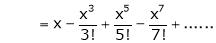
3. 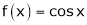    Then

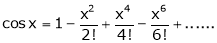
Proof:

Let f(x) = cos x

Then by Maclaurin’s series,

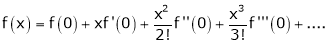   … (1)

Since

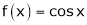
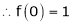
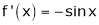
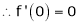
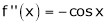
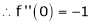
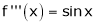
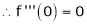
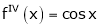
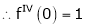
From Equation (1)

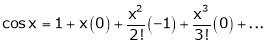
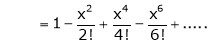
4. 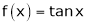      then

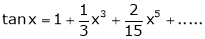
Proof:

Here f(x) = tan x

By Maclaurin’s expansion,

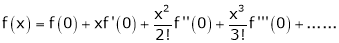  … (1)

Since

…..

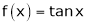
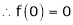
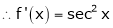
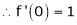
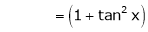
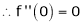
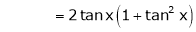
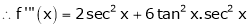
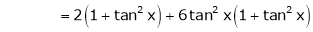
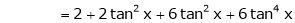
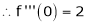
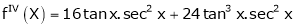
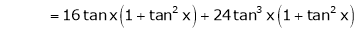
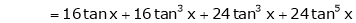
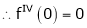
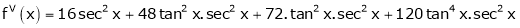
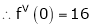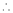By equation (1)

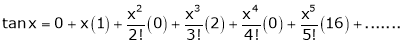
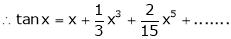
5. 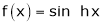    Then

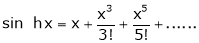
Proof:-

Here f(x) = sin hx.

By Maclaurin’s expansion,

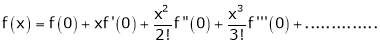  (1)

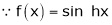
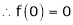
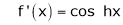
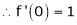
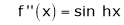
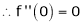
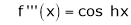
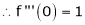
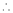By equation (1) we get,

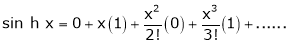
6. 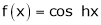    . Then

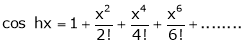
Proof:-

Here f(x) = cos hx

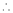By Maclaurin’s expansion

 (1)

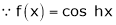
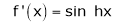
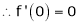
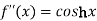
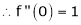
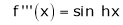
By equation (1)

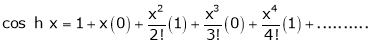
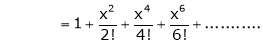
7.     f(x) = tan hx

Proof:

Here f(x) = tan hx

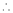By Maclaurin’s series expansion,

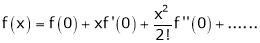    … (1)

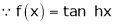
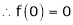
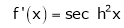
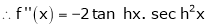
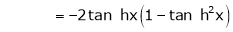
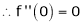
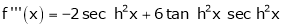
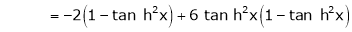
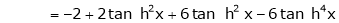
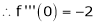
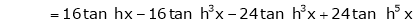
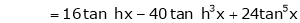
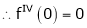
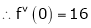
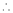By equation (1)

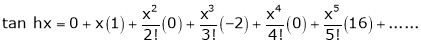
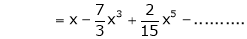
8. 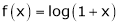       then

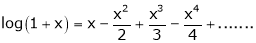
Proof:-

Here f(x) = log (1 + x)

By Maclaurin’s series expansion,

  … (1)

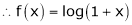
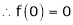
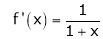
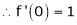
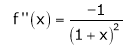
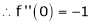
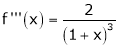
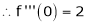
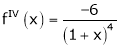
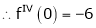
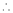By equation (1)

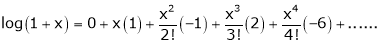
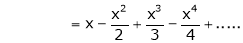
9.    

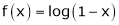
In above result we replace x by -x

Then

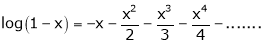
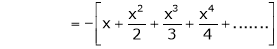
10. Expansion of tan h-1x

We know that

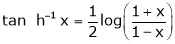
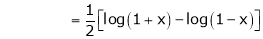
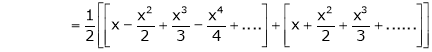
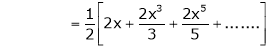
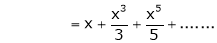
Thus

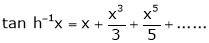
11. Expansion of (1 + x)m

Proof:-

Let f(x) = (1 + x)m

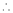By Maclaurin’s series.

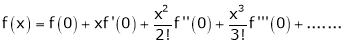  … (1)

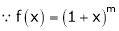
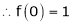
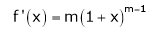
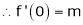
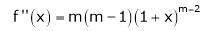
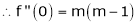
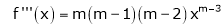
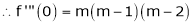
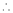By equation (1) we get,

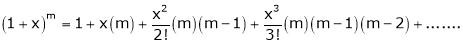
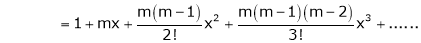
Note that in above expansion if we replace m = -1 then we get,

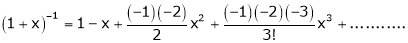
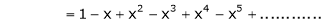
Now replace x by -x in above we get,

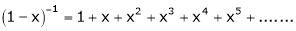
Expand by, Maclaurin’s theorem

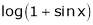
Solution:

Here f(x) = log (1 + sin x)

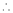By Maclaurin’s Theorem,

   … (1)

……..

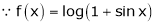
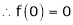
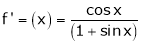
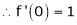
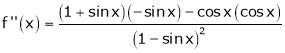
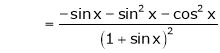
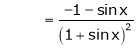
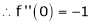
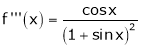
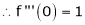
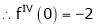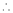equation (1) becomes,

5.2 Taylor's theorem

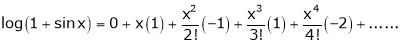
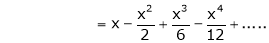
Taylor’s Series Expansion:-

a)     The expansion of f(x+h) in ascending power of x is

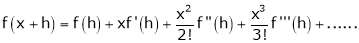
b)    The expansion of f(x+h) in ascending power of h is

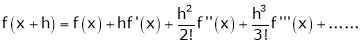
c)     The expansion of f(x) in ascending powers of (x-a) is,

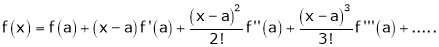
Using the above series expansion we get series expansion of f(x+h) or f(x).

Expansion of functions using standard expansions

Expand 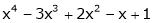in power of (x – 3)

Solution:

Let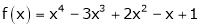

Here a = 3

Now by Taylor’s series expansion,

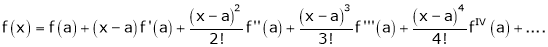 … (1)

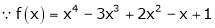
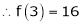
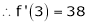
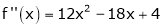
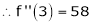
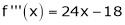
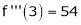
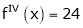
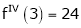
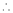equation (1) becomes.

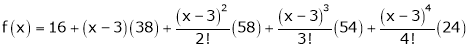
Using Taylors series method expand

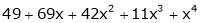in powers of (x + 2)

Solution:

Here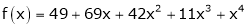

a = -2

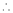By Taylors series,

   … (1)

Since

,  , …..

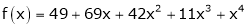
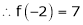
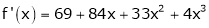
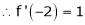
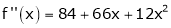
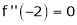
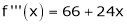
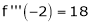
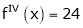
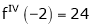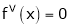Thus equation (1) becomes

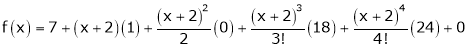
Expand 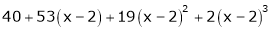in ascending powers of x.

Solution:

Here

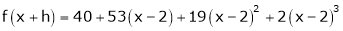
i.e.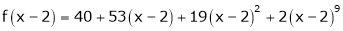

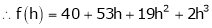
Here h = -2

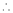By Taylors series,

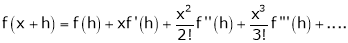    … (1)

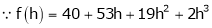
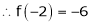
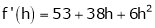
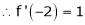
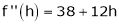
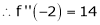
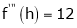
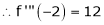
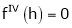
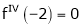
equation (1) becomes,

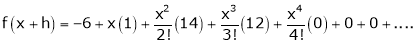
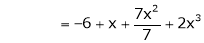
Thus

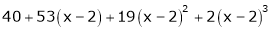
Expand 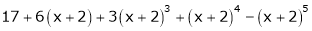in powers of x using Taylor’s theorem,

Solution:

Here

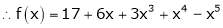
i.e.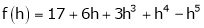

Here

h = 2

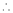By Taylors series

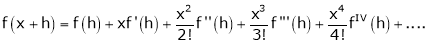  … (1)

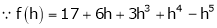
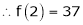
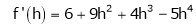
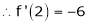
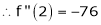
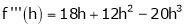
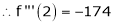
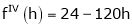
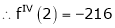
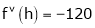
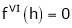
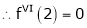
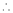By equation (1)

5.3 Indeterminate forms and L' Hospital's rule

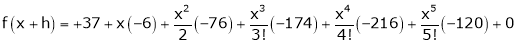
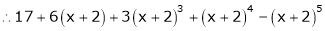
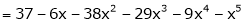
L – Hospital rule for and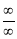

Statement:

If 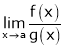 takes eithe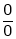r or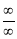

Indeterminate form, then

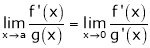Provided limit is exist

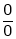If again takes either or .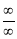

Then 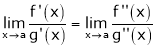; limit is exist

We continue the procedure until the limit is exist.

Exercise 1

Evaluate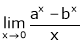

Solution:

Let

   …

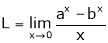By L – Hospital rule,

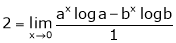
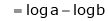
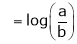
Exercise 2

Evaluate

Solution:

Let

   …

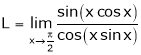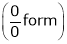
By L – Hospital rule

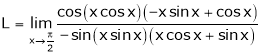
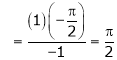
Exercise 3

Evaluate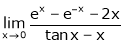

Solution:

Let

  …

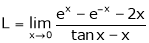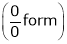
By L – Hospital rule

   …

  …

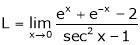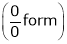
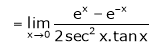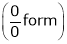
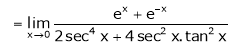
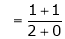
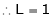
Find the value of a, b if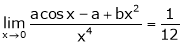

Solution:

Let

   …

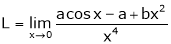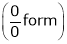
By L – Hospital rule

   …

   …

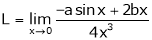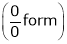
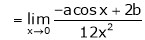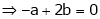    … (1)

    …

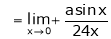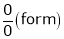
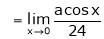
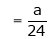
But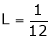

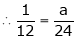
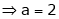
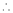From equation (1)

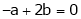
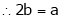
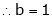
Evaluate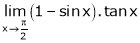

Solution:

Let

   …

    …

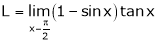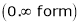
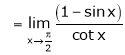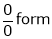
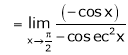    (By L – Hospital Rule)

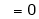
Evaluate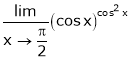

Solution:

Let

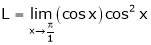   … 0o form

Taking log on both sides we get,

  …

   …

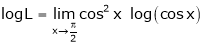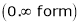
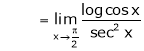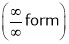
By L – Hospital Rule

i.e.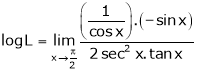

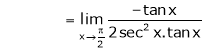
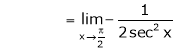
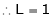
Evaluate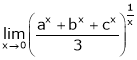

Solution:

Let

   …

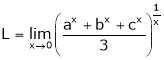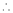Taking log on both sides,

  …

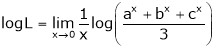
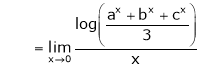By L – Hospital rule,

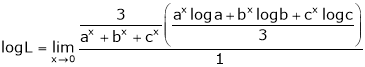
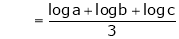
i.e.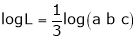

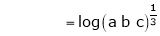
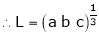
Evaluate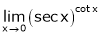

Solution:

Let

    …

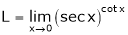Taking log on both sides, we get

    …

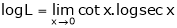
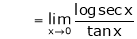By L – Hospital Rule,

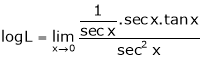
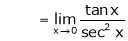
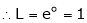
Reference Books:

1. Advanced Engineering Mathematics by Erwin Kreyszig, Wiley India Pvt. Ltd.

2. Advanced Engineering Mathematics by H. K. Dass, S. Chand, New Delhi.

3. A text book of Engineering Mathematics Volume I by Peter V. O’Neil and Santosh K.Sengar, Cengage Learning.

4. Mathematical methods of Science and Engineering by Kanti B. Datta, Cengage Learning.

5. Numerical methods by Dr. B. S. Grewal, Khanna Publishers, Delhi.

6. A text book of Engineering Mathematics by N. P. Bali, Iyengar, Laxmi Publications (P) Ltd.,New Delhi.