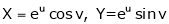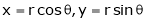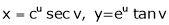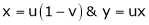Unit 6

Partial Differentiation

6.1 Partial derivatives: Introduction

Partial Differentiation

If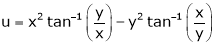

Prove that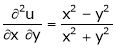

Partial differentiation of function of function

If z = f(u) and 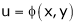. Then z becomes a function of x & y. In this case z becomes a function of function of x & y.

i.e.

Then

,

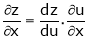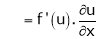
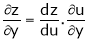
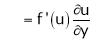
Similarly

If

Then z becomes a function of function of x, y & z.

…………….

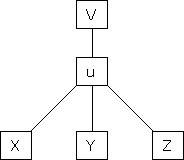
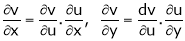
1. If where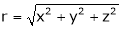

Prove that

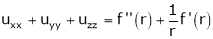
2.     If V = 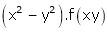show that

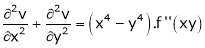
3.     If 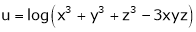show that

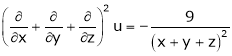
4.     If 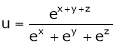then prove that

6.2 Total derivatives

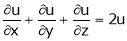
In mathematics, the total derivative of a function 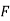 f {\displaystyle f}  at a point is the best linear approximation near this point of the function with respect to its arguments. Unlike partial derivatives, the total derivative approximates the function with respect to all of its arguments, not just a single one. In many situations, this is the same as considering all partial derivatives simultaneously. The term "total derivative" is primarily used when f {\displaystyle f} is a function of several variables, because when f {\displaystyle f} is a function of a single variable, the total derivative is the same as the derivative of the function.

"Total derivative" is sometimes also used as a synonym for the material derivative in fluid mechanic

A total derivative of a multivariable function of several variables, each of which is a function of another argument, is the derivative of the function with respect to said argument. It is equal to the sum of the partial derivatives  with respect to each variable times the derivative of that variable with respect to the independent variable.

6.3 Differentiation of implicit function

In mathematics, an implicit equation is a relation of the form 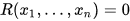R ( x 1 , … , x n ) = 0 {\displaystyle R(x_{1},\ldots ,x_{n})=0} where R {\displaystyle R} Ris a function of several variables (often a polynomial). For example, the implicit equation of the unit circle is x 2 + y 2 − 1 = 0 {\displaystyle x^{2}+y^{2}-1=0} .

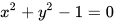An implicit function is a function that is defined implicitly by an implicit

Equation, by associating one of the variables (the value) with the others

(the arguments). Thus, an implicit function for y {\displaystyle y} yin the context of the unit circle is defined implicitly by 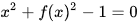x 2 + f ( x ) 2 − 1 = 0 {\displaystyle x^{2}+f(x)^{2}-1=0} . This implicit equation defines f {\displaystyle f} f as a function of 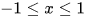x {\displaystyle x} y only if − 1 ≤ x ≤ 1 {\displaystyle -1\leq x\leq 1} and one considers only non-negative (or non-positive) values for the values of the function.

The implicit function theorem provides conditions under which some kinds of relations define an implicit function, namely relations defined as the indicator function of the zero set of some continuously differentiable multivariate function.

EXAMPLE 1:

Example 2:

6.4 Euler's theorem on homogeneous function of two variables

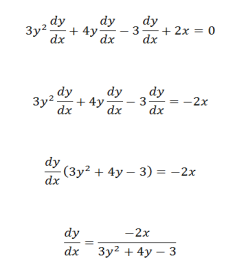
A polynomial in x & y is said to be Homogeneous expression in x & y of degree n. If the degree of each term in the expression is same & equal to n.

e.g.

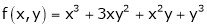is a homogeneous function of degree 3.

To find the degree of homogeneous expression f(x, y).

1. Consider
2. Put . Then if we get .

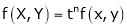Then the degree of is n.

Ex.

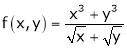
Consider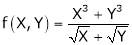

Put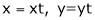

.

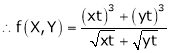
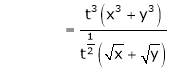
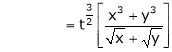
Thus degree of f(x, y) is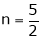

Note that

If 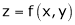be a homogeneous function of degree n then z can be written as

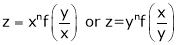
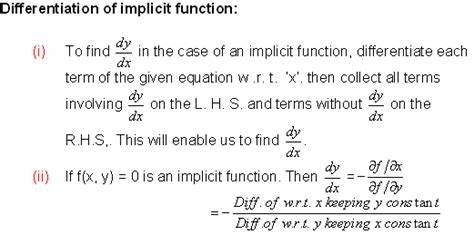
Differentiation of Implicit function

Suppose that we cannot find y explicitly as a function of x. But only implicitly through the relation f(x, y) = 0.

Then we find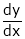

Since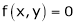

diff. P. w.r.t. x we get

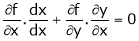
i.e.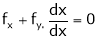

Similarly,

It f (x, y, z) = 0 then z is called implicit function of x, y. Then in this case we get

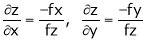
Ex.

Find 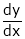if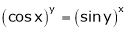

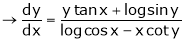
Ex. Find 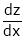. If , &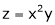

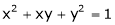
Ex. If , where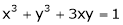

Find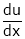

Ex. If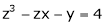

Then find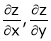

Eulers Theorem on Homogeneous functions:

Statement:

If 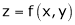be a homogeneous function of degree n in x & y then,

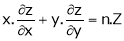
Deductions from Eulers theorem

1. If 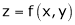be a homogeneous function of degree n in x & y then,

.

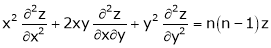2.     If 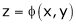be a homogeneous functions of degree n in x & y and also 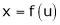then,

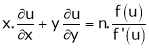
And

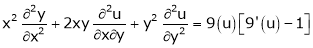
Where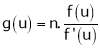

Ex.

If 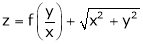, find the value of

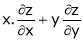
Ex.

If then find the value of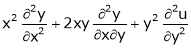

Ex. If 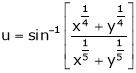then prove

That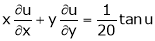

Ex. If 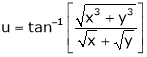the prove that

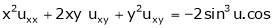
Ex. If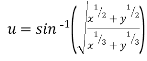  then show

That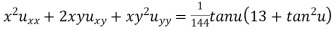

6.5 Jacobian and its Properties

Jacobians, Errors and Approximations, maxima and minima

Jacobians

If u and v be continuous and differentiable functions of two other independent variables x and y such as

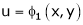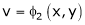, then we define the determine

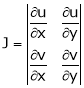as Jacobian of u, v with respect to x, y

Similarly ,

JJ’ = 1

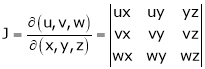
Actually Jacobins are functional determines

Ex.

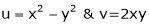1.   Calculate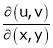
2. If
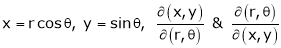
3. If

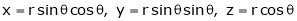
ST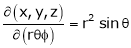

4.      find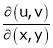

5.     If and 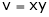, find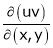

6.    

7.     If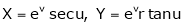  

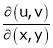
8.     If , ,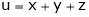

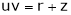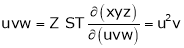
JJ1 = 1

If ,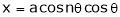

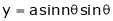
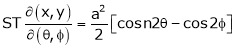
JJ1=1

Jacobian of composite function (chain rule)

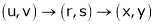
Then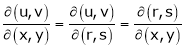

Ex.

1. If

Where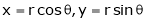

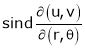
2.     If 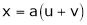 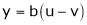 and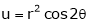

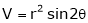
Find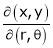

3.     If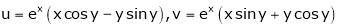

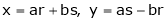
Find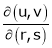

Jacobian of Implicit function

Let u1, u2 be implicit functions of x1, x2 connected by f1, f2 such there

,

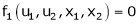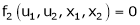
Then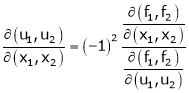

Similarly,

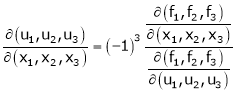
Ex.

If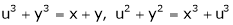

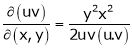
If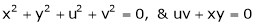

Find

Partial derivative of implicit functions

Consider four variables u, v, x, y related by implicit function.

,

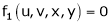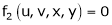
Then

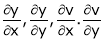
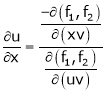
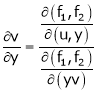
Ex.

If 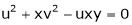and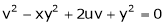

Find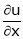

If 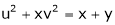and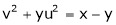

Find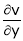

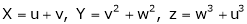
Find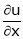

If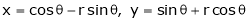

Find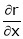

6.6 Maxima and Minima of functions of two variables

When a function has one variable we know how to find the maxima and minima of the function by differentiating and equating to zero to find the points. But when a function has more than two variables, we use partial differentiation to find the maxima and minima. Where $\lambda $ is Lagrange multiplier.

Maxima and Minima of Functions of Two Variables:

Example 1:

If . . . f ( x , y ) . . . Has a maximum or minimum at point ( a , b ) [ i.e. . . . ( a , b ) . . . Is a point of inflection ], then

f x = 0 . . . And . . . f y = 0 , . . . . . . At ( a , b )

Now put . . . δ f ( a , b ) . . . = . . . f ( a + h , b + k ) – f ( a , b )

So at a maximum or minimum

δ f . . . ≈ . . . h 2 f x x + k 2 f y y + 2 h k f x y

So f has a maximum at ( a , b ) if . . . f x = 0 . . . And . . . f y = 0 , . . . And . . . δ f < 0 for all small h and k . Similarly it has a maximum if . . . δ f > 0 for all small h and k . In particular . . . f xx < 0 . . . And . . . f yy < 0 . . . For a maximum ( putting h = 0 and k = 0 respectively) and . . . f xx > 0 . . . And . . . f yy > 0 . . . For a minimum

The approximation for δ f can be written:

δ f . . . ≈ . ( ( h f xx + k f xy ) 2 – k 2 ( f xy 2 – f xx f yy ) ) ⁄ 2 f xx ,providing . . . f xx ≠ 0.

Or . .   δ f . . . ≈ . . . ( Θ 2 – k 2 Δ ) / 2 f xx , . . . Where . . . Θ = ( h f xx + k f xy ) ,

And . . . Δ = ( f xy 2 – f xx f yy ) .

Note that . . . ( Θ 2 – k 2 Δ ) . . . Is positive if Δ is zero or negative, and that Δ is not dependant on the values of h and k . So for a point of inflection we have:

• Δ ≤ 0 . . . And . . . f xx > 0 . . . ⇒ . . . δ f > 0 . . . ⇒ . . . Minimum.
• Δ ≤ 0 . . . And . . . f xx < 0 . . . ⇒ . . . δ f < 0 . . . ⇒ . . . Maximum.
• Δ > 0 . . . Then the value of δ f is dependant on h and k , so this is a saddle-point.

What if f xx = 0 ? . . . Note that the original approximation for δ f is symmetic in x , y , h and k . So we can write

δ f . . . ≈ . . . ( Λ 2 – h 2 Δ ) / 2 f yy , . . . Where . . . Λ = ( k f yy + h f xy ) , . . . Δ same as above.

And note that if . . . f xx = 0 . . . Then Δ is positive, so we will have a saddle-point. A completely symmetric argument holds for f yy = 0.